Slopes and Areas Frequently we will want to





- Slides: 12

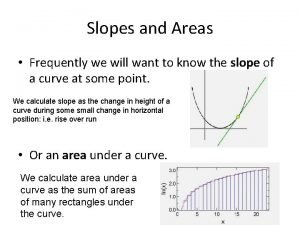
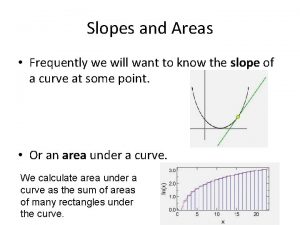
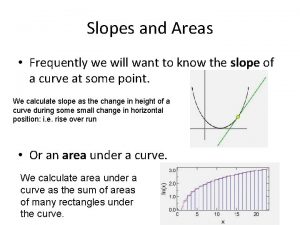
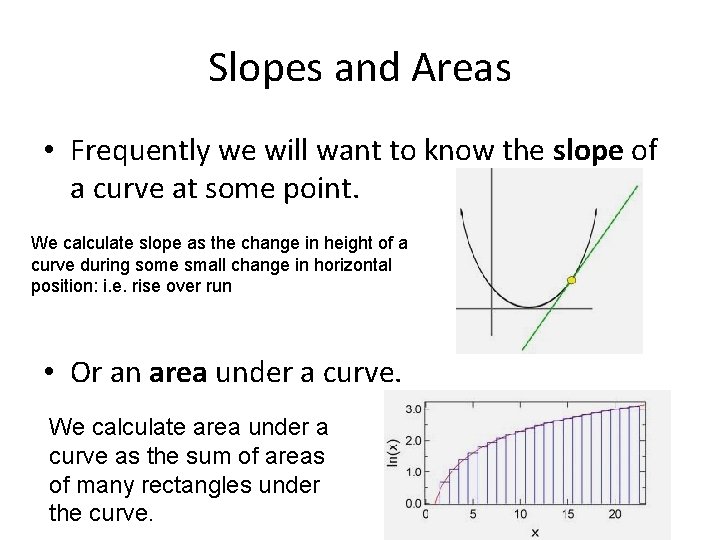
Slopes and Areas • Frequently we will want to know the slope of a curve at some point. We calculate slope as the change in height of a curve during some small change in horizontal position: i. e. rise over run • Or an area under a curve. We calculate area under a curve as the sum of areas of many rectangles under the curve.

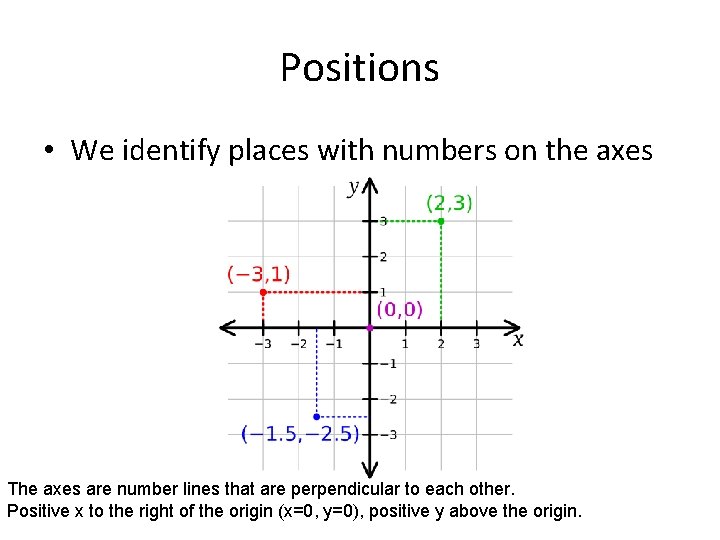
Review: Axes • When two things vary, it helps to draw a picture with two perpendicular axes to show what they do. Here are some examples: y x x y varies with x Here we say “ y is a function of x”. t x varies with t Here we say “x is a function of t”.

Positions • We identify places with numbers on the axes The axes are number lines that are perpendicular to each other. Positive x to the right of the origin (x=0, y=0), positive y above the origin.

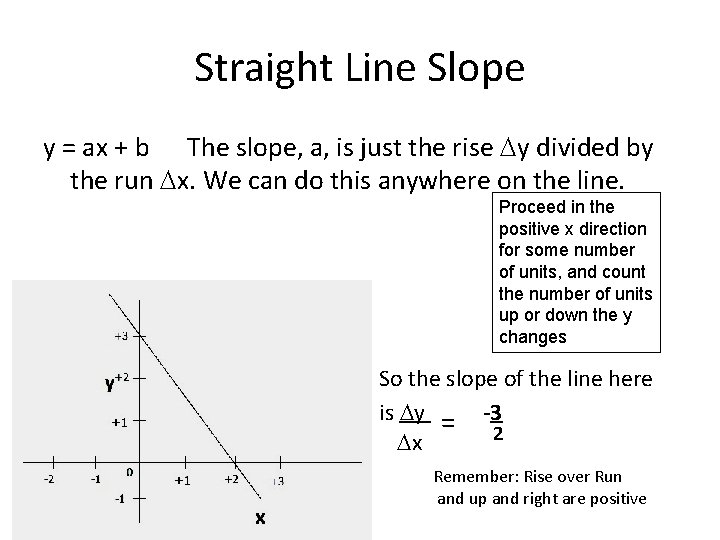
Straight Lines • Sometimes we can write an equation for how one variable varies with the other. For example a straight line can be described as y = ax + b Here, y is a position on the line along the y-axis, x is a position on the line along the xaxis, a is the slope, and b is the place where the line hits the y-axis

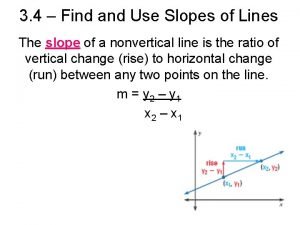
Straight Line Slope y = ax + b The slope, a, is just the rise Dy divided by the run Dx. We can do this anywhere on the line. Proceed in the positive x direction for some number of units, and count the number of units up or down the y changes So the slope of the line here is Dy = -3 2 Dx Remember: Rise over Run and up and right are positive

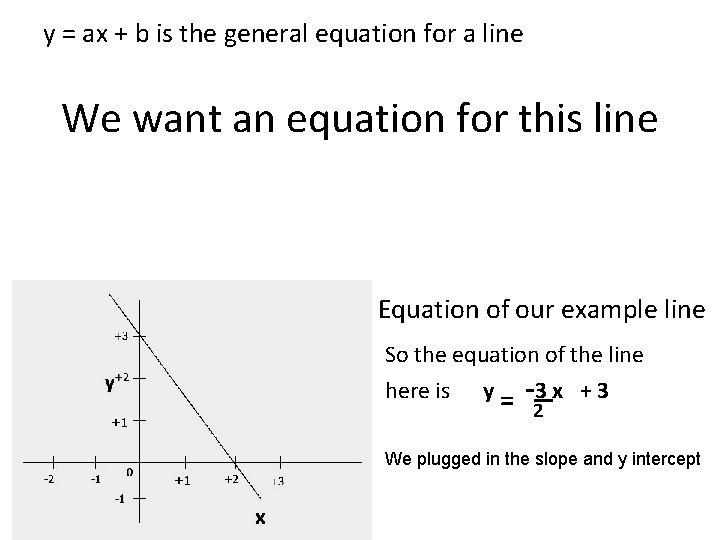
y- intercept y = ax + b is our equation for a line b is the place where the line hits the y-axis The intercept b is y = +3 when x = 0 for this line

y = ax + b is the general equation for a line We want an equation for this line Equation of our example line So the equation of the line here is y = -3 x + 3 2 We plugged in the slope and y intercept

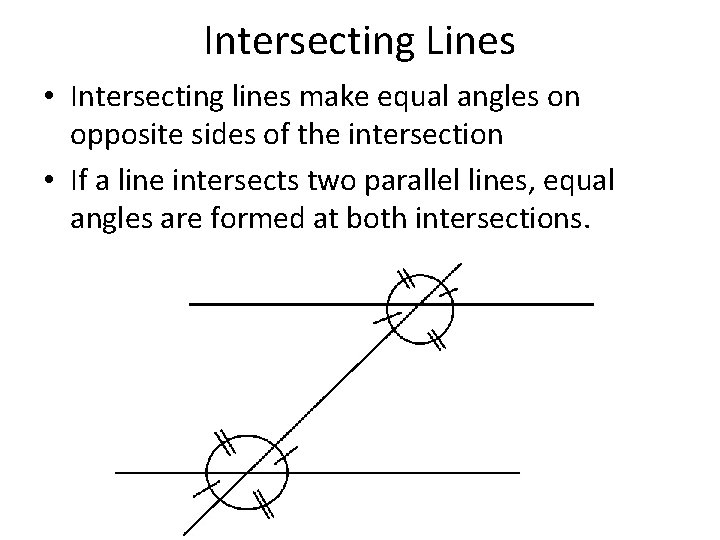
Intersecting Lines • Intersecting lines make equal angles on opposite sides of the intersection • If a line intersects two parallel lines, equal angles are formed at both intersections.

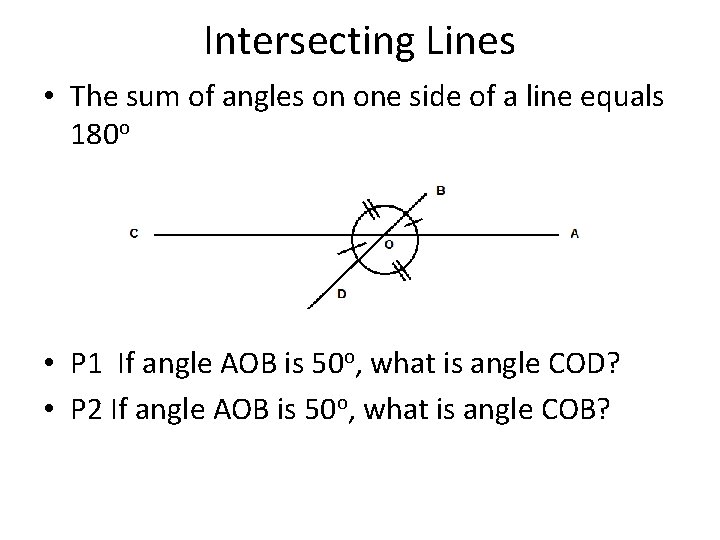
Intersecting Lines • The sum of angles on one side of a line equals 180 o • P 1 If angle AOB is 50 o, what is angle COD? • P 2 If angle AOB is 50 o, what is angle COB?

Sum of angles in a Triangle • The sum of angles in a triangle equals 180 o • Notice this is a right triangle, because one of the angles (X 0 Y) is 90 o • P 3 if angle X 0 Y is 90 o, and angle 0 XY is 60 o, what is angle 0 YX?

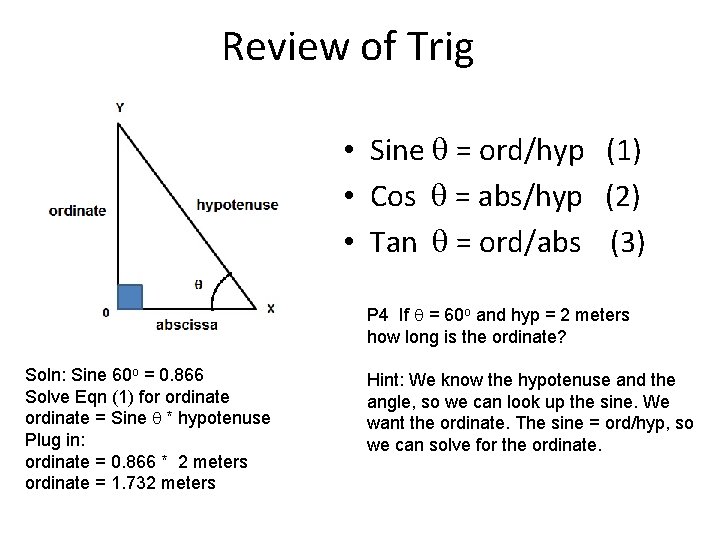
Review of Trig • Sine q = ord/hyp • Cos q = abs/hyp • Tan q = ord/abs P 4 If q = 60 o and hyp = 2 meters how long is the ordinate? Hint: We know the hypotenuse and the angle, so we can look up the sine. We want the ordinate. The sine = ord/hyp, so we can solve for the ordinate.

Review of Trig • Sine q = ord/hyp (1) • Cos q = abs/hyp (2) • Tan q = ord/abs (3) P 4 If q = 60 o and hyp = 2 meters how long is the ordinate? Soln: Sine 60 o = 0. 866 Solve Eqn (1) for ordinate = Sine q * hypotenuse Plug in: ordinate = 0. 866 * 2 meters ordinate = 1. 732 meters Hint: We know the hypotenuse and the angle, so we can look up the sine. We want the ordinate. The sine = ord/hyp, so we can solve for the ordinate.
 Slope of parallel lines
Slope of parallel lines Parallel and perpendicular lines worksheet doc
Parallel and perpendicular lines worksheet doc Slopes of parallel and perpendicular lines assignment
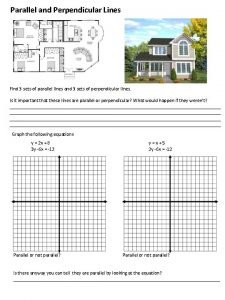
Slopes of parallel and perpendicular lines assignment 3 sets of parallel lines
3 sets of parallel lines Slopes of parallel and perpendicular lines lesson 8-1
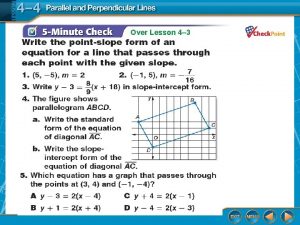
Slopes of parallel and perpendicular lines lesson 8-1 If line a contains q(5 1)
If line a contains q(5 1) What slope is perpendicular to 5/8
What slope is perpendicular to 5/8 Find and use slopes of lines
Find and use slopes of lines How often do you exercise
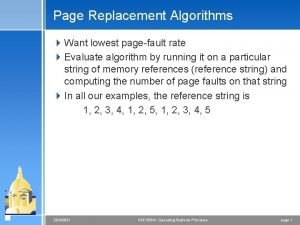
How often do you exercise Page replacement algorithm online calculator
Page replacement algorithm online calculator Habitual past action with would
Habitual past action with would Very often often sometimes rarely never
Very often often sometimes rarely never Tolerates no challenge to his authority
Tolerates no challenge to his authority