Lorentz Transformation 2 2012 1 1 Now we





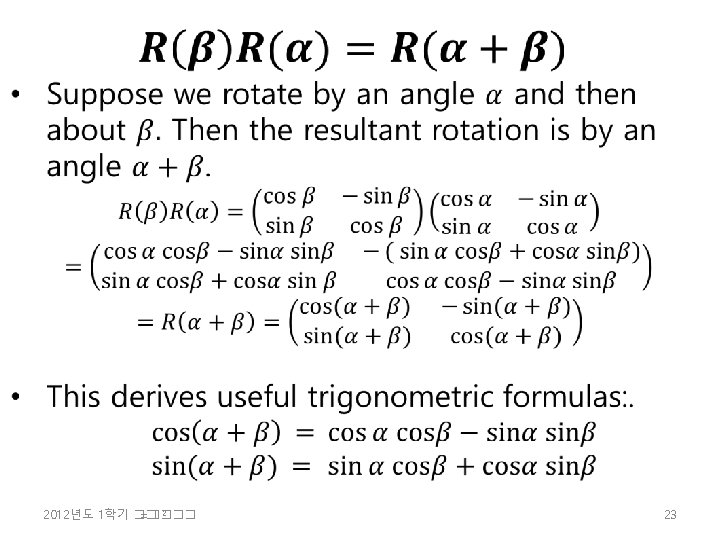

- Slides: 26

Lorentz Transformation 2 2012년도 1학기 �� =���� 1

Now we know that … • According to Michelson-Morley Experiment, the speed of light is invariant. • 2 important consequences: time dilation and length contraction. • We believe that there must be a linear transformation that relates the space-time coordinates of any two inertial reference frame. • The transformation rules are called Lorentz transformation. • This week, we derive the transformation. 2 2012년도 1학기 �� =���� 2

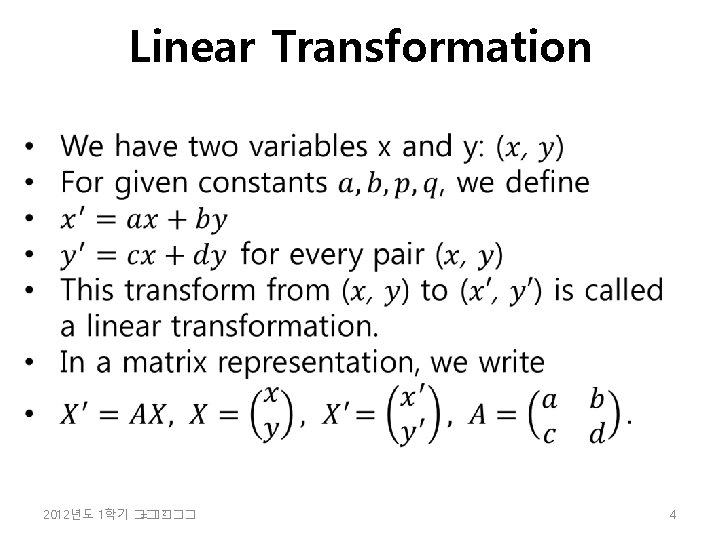
Linear Transformation 2 2012년도 1학기 �� =���� 3

Linear Transformation 2 2012년도 1학기 �� =���� 4

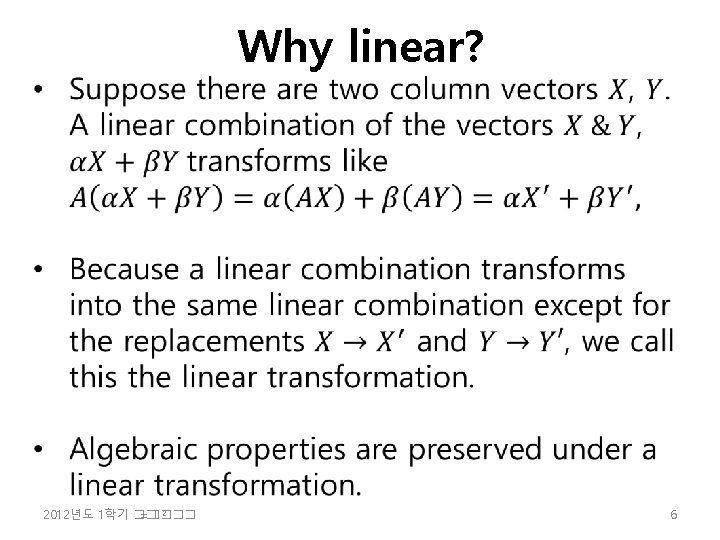
Linear Transformation 2 2012년도 1학기 �� =���� 5


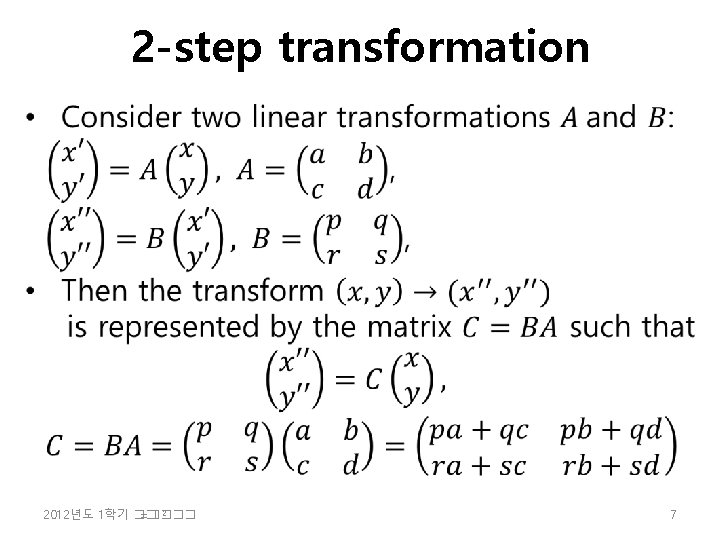
2 -step transformation 2 2012년도 1학기 �� =���� 7

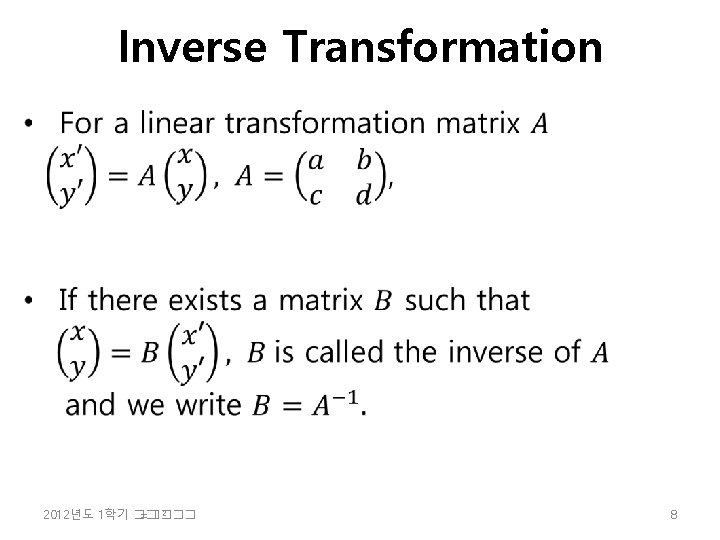
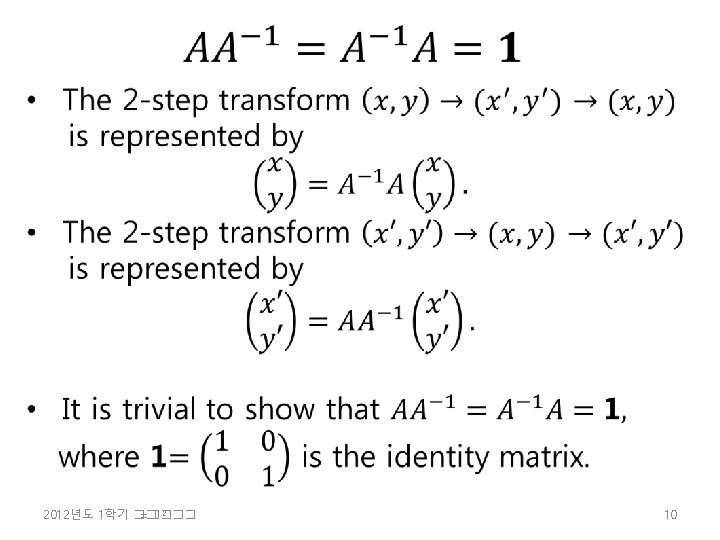
Inverse Transformation 2 2012년도 1학기 �� =���� 8

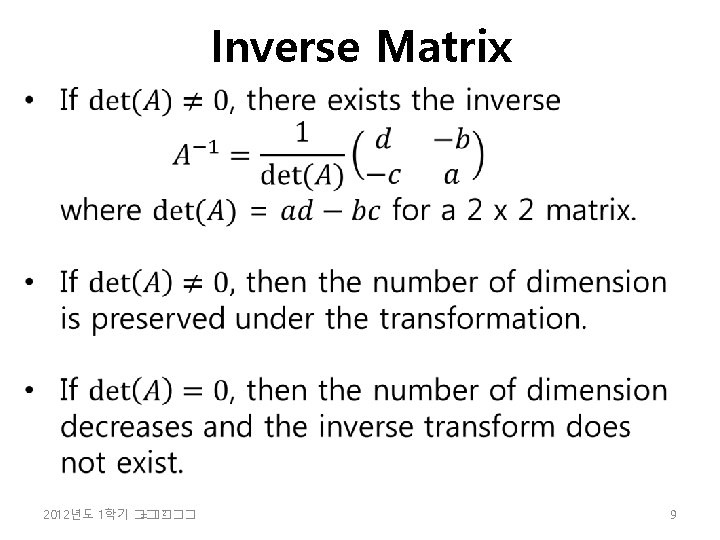
Inverse Matrix 2 2012년도 1학기 �� =���� 9


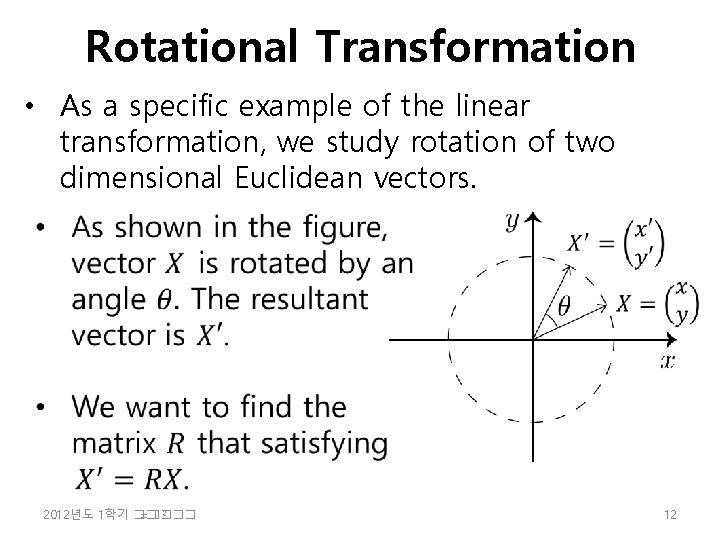
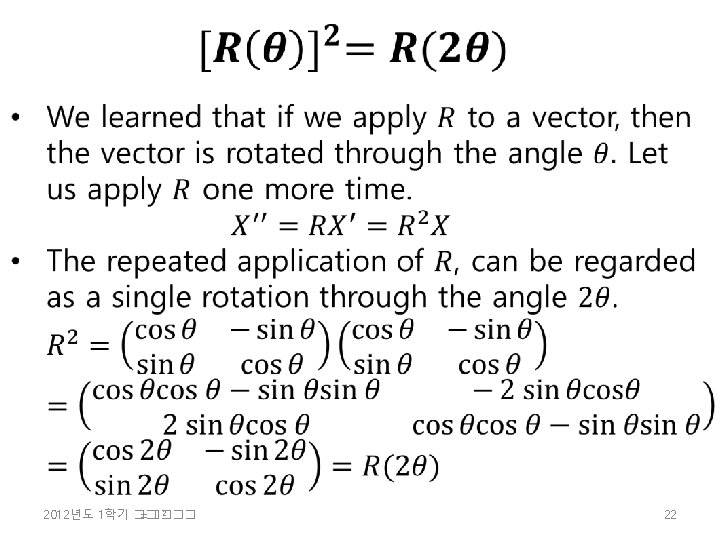
Rotational Transformation 2 2012년도 1학기 �� =���� 11

Rotational Transformation • As a specific example of the linear transformation, we study rotation of two dimensional Euclidean vectors. 2 2012년도 1학기 �� =���� 12

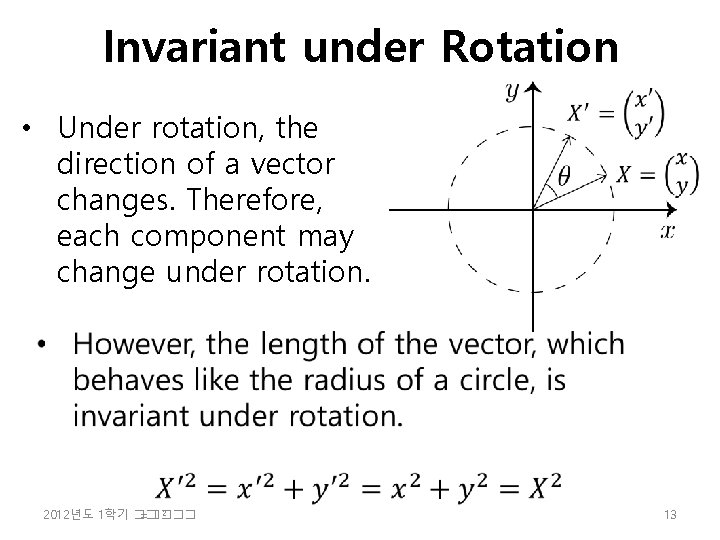
Invariant under Rotation • Under rotation, the direction of a vector changes. Therefore, each component may change under rotation. 2 2012년도 1학기 �� =���� 13

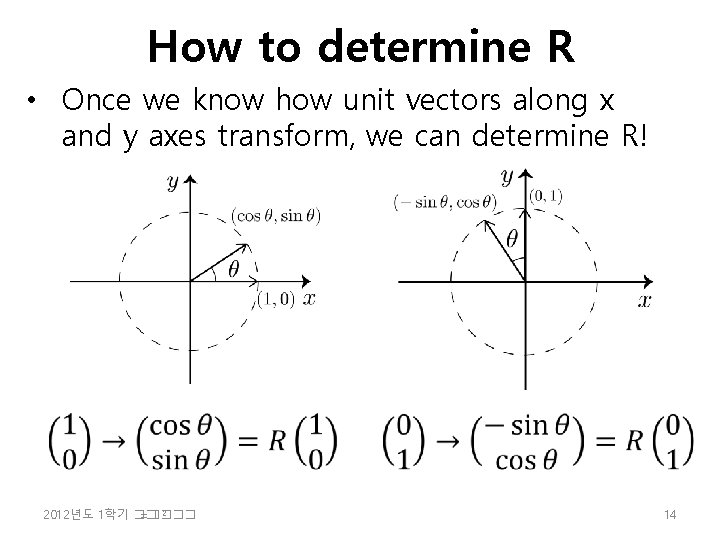
How to determine R • Once we know how unit vectors along x and y axes transform, we can determine R! 2 2012년도 1학기 �� =���� 14

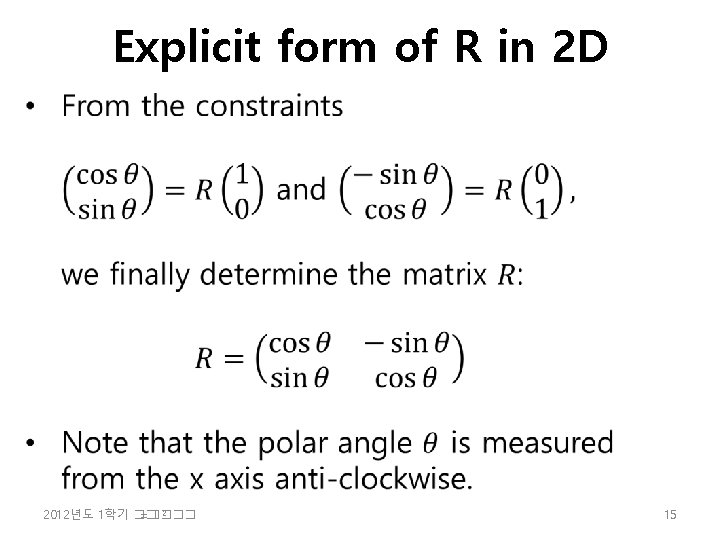
Explicit form of R in 2 D 2 2012년도 1학기 �� =���� 15

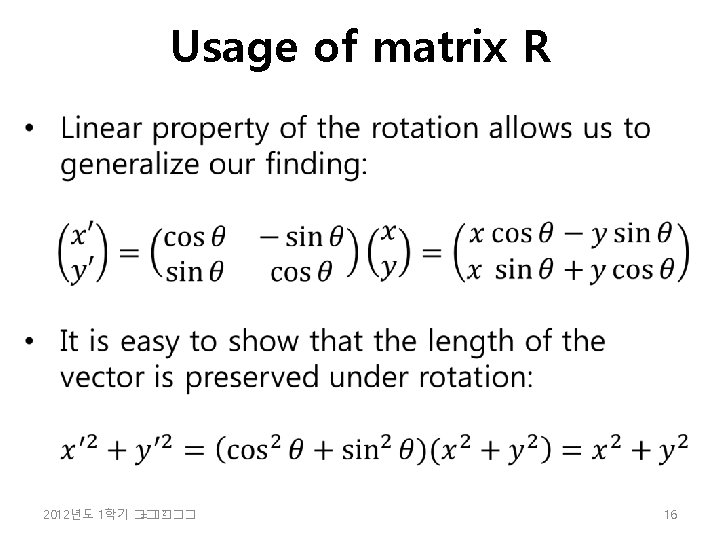
Usage of matrix R 2 2012년도 1학기 �� =���� 16

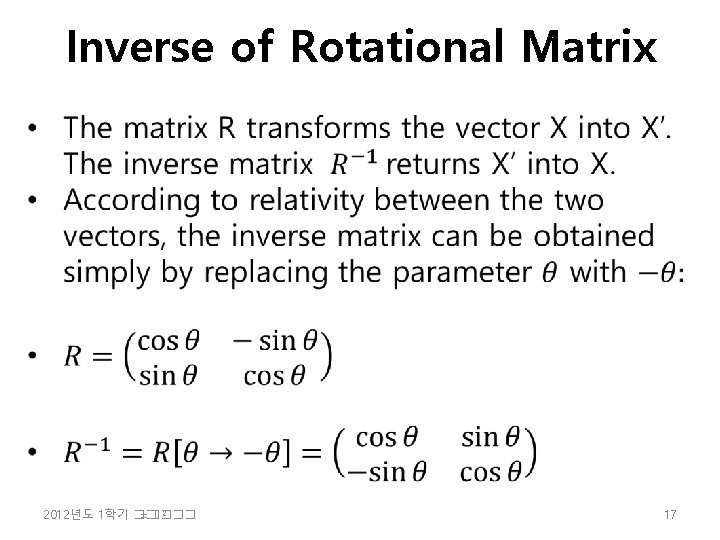
Inverse of Rotational Matrix 2 2012년도 1학기 �� =���� 17

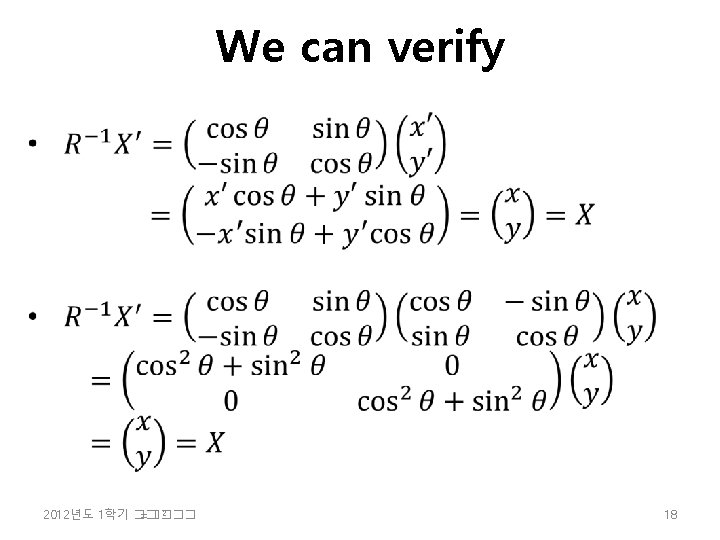
We can verify 2 2012년도 1학기 �� =���� 18

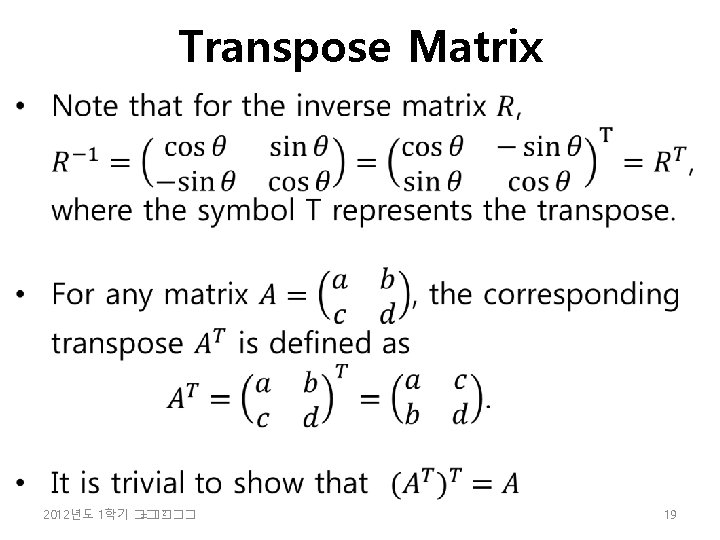
Transpose Matrix 2 2012년도 1학기 �� =���� 19

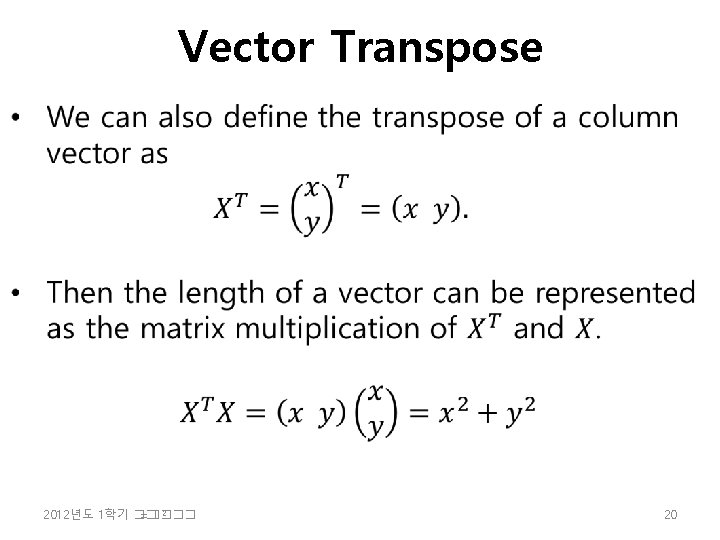
Vector Transpose 2 2012년도 1학기 �� =���� 20

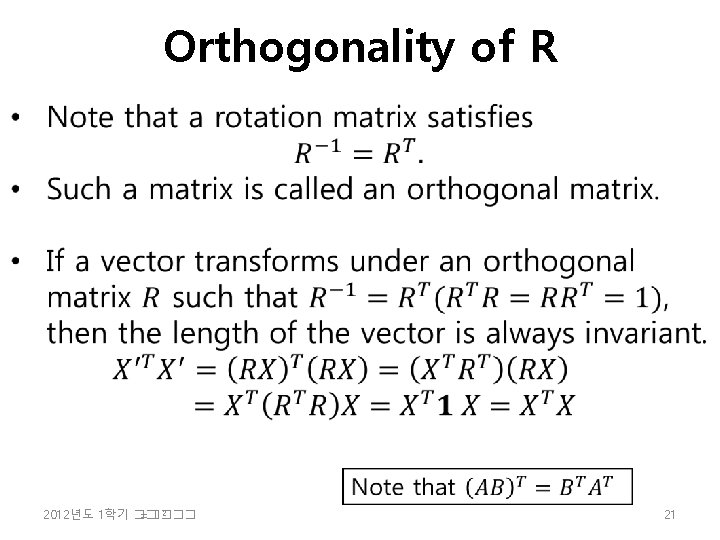
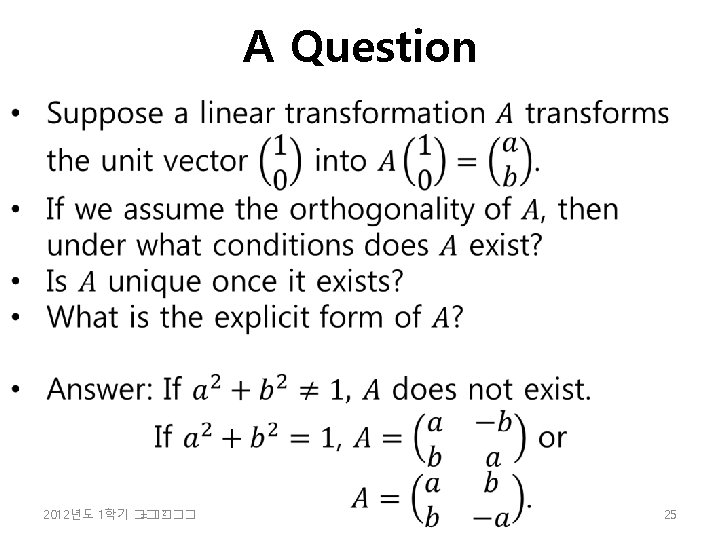
Orthogonality of R 2 2012년도 1학기 �� =���� 21

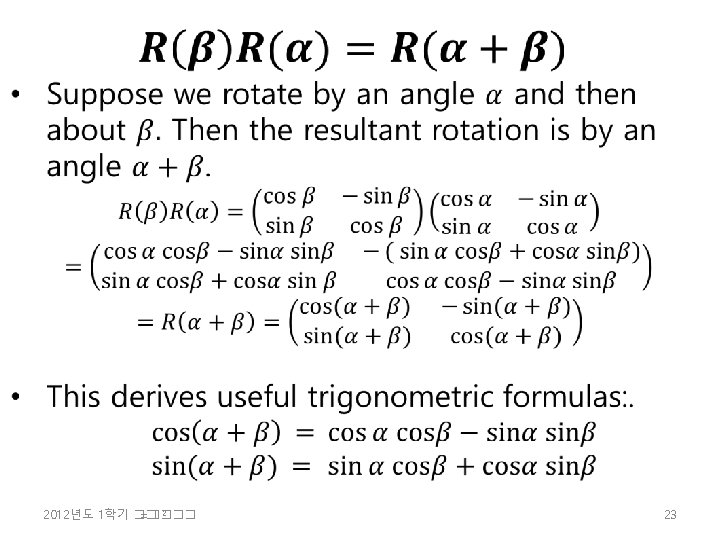



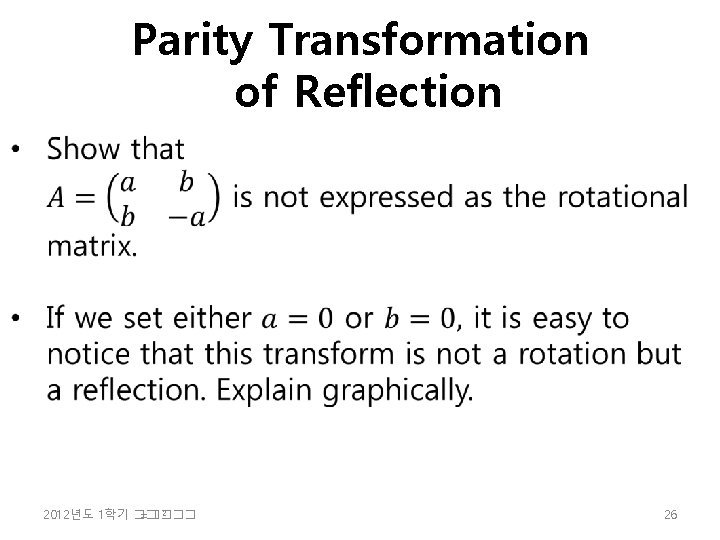
Parity Transformation of Reflection 2 2012년도 1학기 �� =���� 26
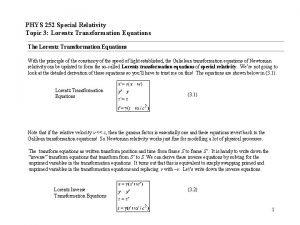
 Inverse lorentz transformation equation
Inverse lorentz transformation equation Proof of lorentz transformation
Proof of lorentz transformation Lorentz transformation equation derivation
Lorentz transformation equation derivation Lorentz transformation matrix
Lorentz transformation matrix Lorentz transformation equation
Lorentz transformation equation Now i see it now you don't
Now i see it now you don't Lorentz
Lorentz Pengertian gaya lorentz
Pengertian gaya lorentz Diagramme de lorentz
Diagramme de lorentz Intervallo invariante
Intervallo invariante Lorentz angle
Lorentz angle Manyetik tork
Manyetik tork Fanny lorentz
Fanny lorentz Ampere's law solenoid
Ampere's law solenoid Verso forza di lorentz
Verso forza di lorentz Lorentz angle
Lorentz angle Densità di energia campo magnetico
Densità di energia campo magnetico Jelaskan transformasi galileo
Jelaskan transformasi galileo Lorentz
Lorentz Psk lorentz
Psk lorentz Equazioni di lorentz
Equazioni di lorentz Gaya lorentz
Gaya lorentz Lorentz force
Lorentz force Legge di faraday
Legge di faraday What is lorentz factor
What is lorentz factor Lorentz force derivation class 12
Lorentz force derivation class 12 Lorentz
Lorentz