Elastic Properties of Solids Topics Discussed in Kittel

Elastic Properties of Solids Topics Discussed in Kittel, Ch. 3, pages 73 -85

Hooke's “Law” • A property of an ideal spring of spring constant k is that it takes twice as much force to stretch the spring twice as far. That is, if it is stretched a distance x, the restoring force is given by F = - kx. The spring is then said to obey Hooke's “Law” • An elastic medium is one in which a disturbance can be analyzed in terms of Hooke’s “Law” forces. Consider the propagation of a mechanical wave (disturbance) in a solid. • We are interested in the case of very long wavelengths, when the wavelength is much, much larger than the interatomic spacing: >> a so that the solid can be treated as a continuous elastic medium & the fact that there atoms on a lattice is irrelevant to the wave propagation.

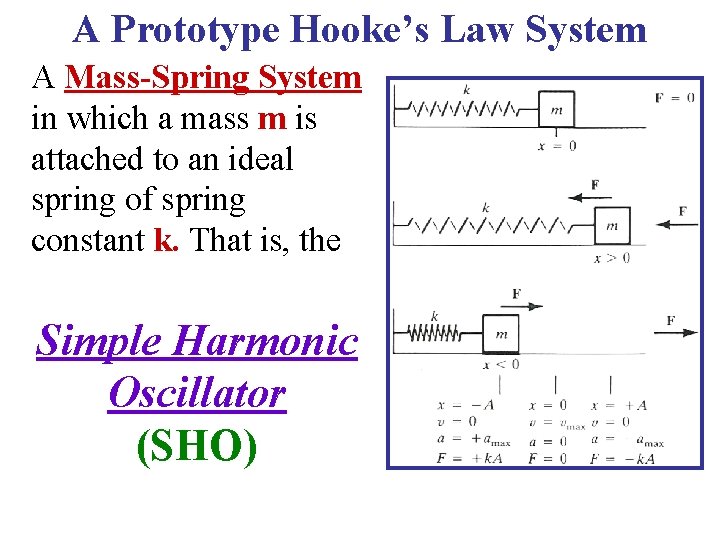
A Prototype Hooke’s Law System A Mass-Spring System in which a mass m is attached to an ideal spring of spring constant k. That is, the Simple Harmonic Oscillator (SHO)

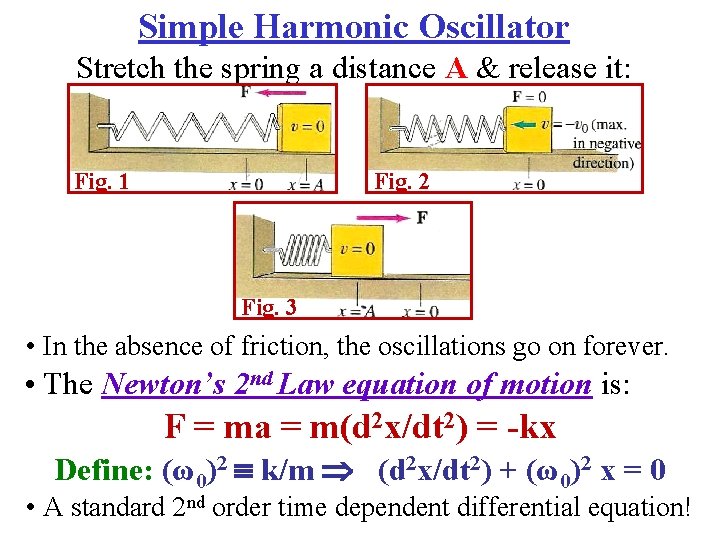
Simple Harmonic Oscillator Stretch the spring a distance A & release it: Fig. 1 Fig. 2 Fig. 3 • In the absence of friction, the oscillations go on forever. • The Newton’s 2 nd Law equation of motion is: F = ma = m(d 2 x/dt 2) = -kx Define: (ω0)2 k/m (d 2 x/dt 2) + (ω0)2 x = 0 • A standard 2 nd order time dependent differential equation!

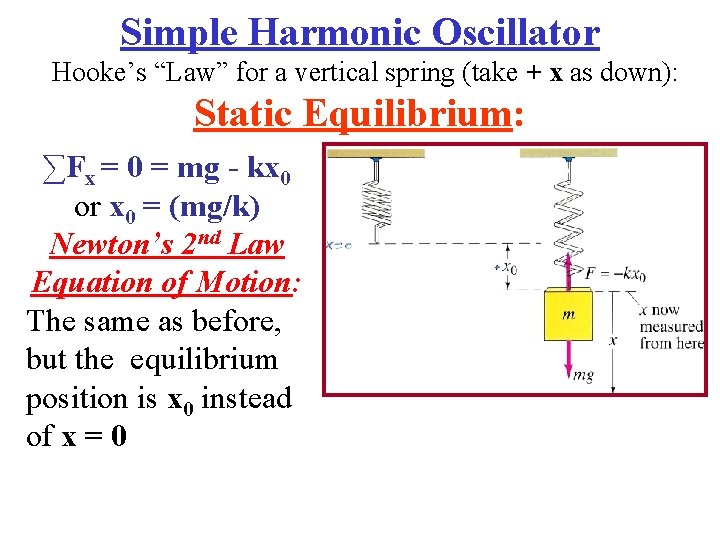
Simple Harmonic Oscillator Hooke’s “Law” for a vertical spring (take + x as down): Static Equilibrium: ∑Fx = 0 = mg - kx 0 or x 0 = (mg/k) Newton’s 2 nd Law Equation of Motion: The same as before, but the equilibrium position is x 0 instead of x = 0

• An Elastic Medium is defined to be one in which a disturbance from equilibrium obeys Hooke’s “Law” so that a local deformation is proportional to an applied force. • If the applied force gets too large, Hooke’s “Law” no longer holds. If that happens the medium is no longer elastic. This is called the Elastic Limit. • The Elastic Limit is the point at which permanent deformation occurs, that is, if the force is taken off the medium, it will not return to its original size and shape.

Sound Waves • Sound waves are mechanical waves which propagate through a material medium (solid, liquid, or gas) at a speed which depends on the elastic & inertial properties of the medium. There are 2 types of wave motion for sound waves: Longitudinal and Transverse

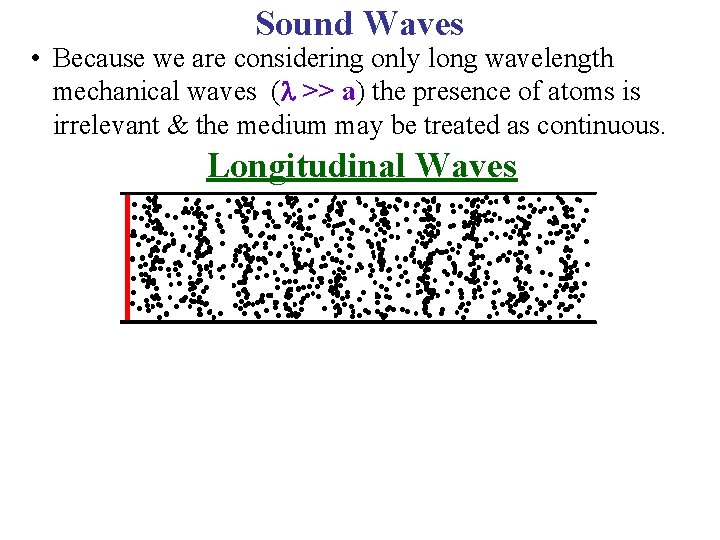
Sound Waves • Because we are considering only long wavelength mechanical waves ( >> a) the presence of atoms is irrelevant & the medium may be treated as continuous. Longitudinal Waves

Sound Waves • Because we are considering only long wavelength mechanical waves ( >> a) the presence of atoms is irrelevant & the medium may be treated as continuous. Longitudinal Waves Transverse Waves

• Sound waves propagate through solids. This tells us that wavelike lattice vibrations of wavelength long compared to the interatomic spacing are possible. • The detailed atomic structure is unimportant for these waves & their propagation is governed by the macroscopic elastic properties of the crystal. • So, the reason for discussing sound waves is that they correspond to the low frequency, long wavelength limit of the more general lattice vibrations we have been considering up to now. • At a given frequency and in a given direction in a crystal it is possible to transmit 3 different kinds of sound waves, differing in their direction of polarization and in general also in their velocity.

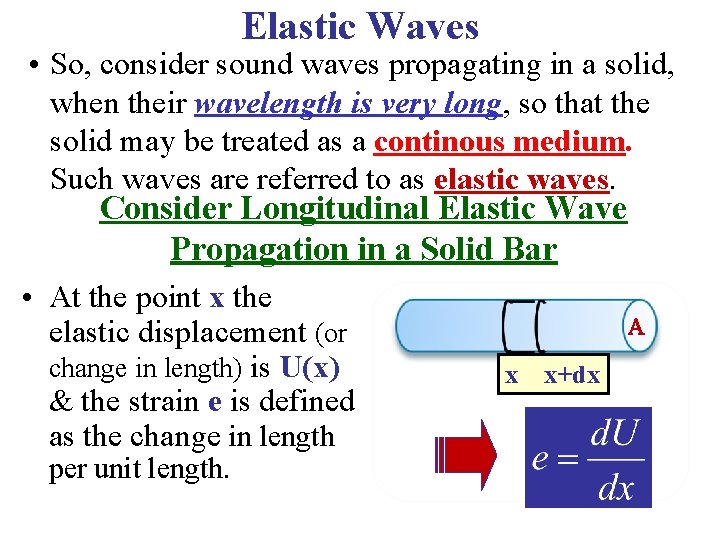
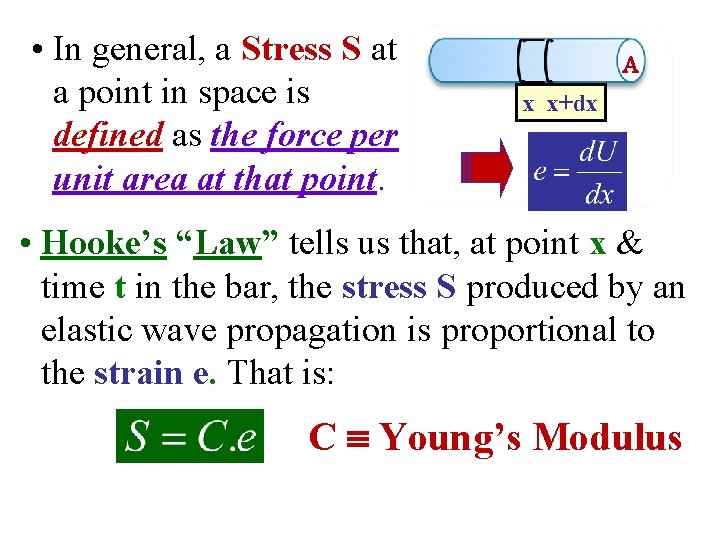
Elastic Waves • So, consider sound waves propagating in a solid, when their wavelength is very long, so that the solid may be treated as a continous medium. Such waves are referred to as elastic waves. Consider Longitudinal Elastic Wave Propagation in a Solid Bar • At the point x the A elastic displacement (or change in length) is U(x) x x+dx & the strain e is defined as the change in length per unit length.

• In general, a Stress S at a point in space is defined as the force per unit area at that point. A x x+dx • Hooke’s “Law” tells us that, at point x & time t in the bar, the stress S produced by an elastic wave propagation is proportional to the strain e. That is: C Young’s Modulus

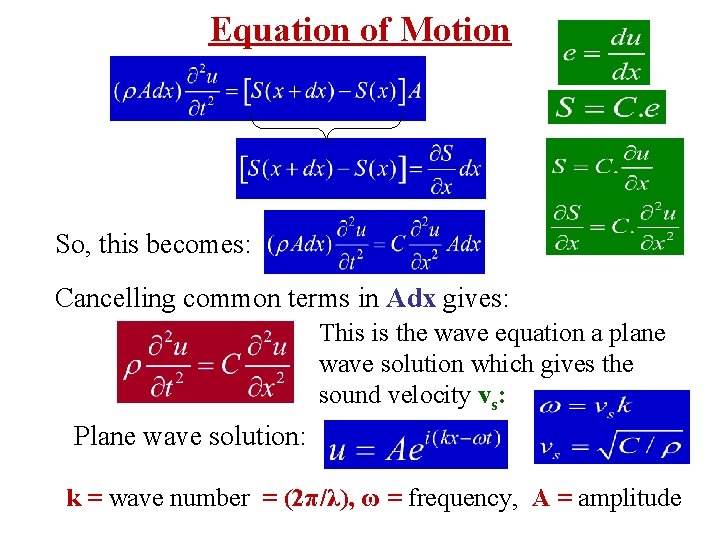
• To analyze the dynamics of the bar, choose an arbitrary segment of length dx as shown above. Use Newton’s 2 nd Law to write for the motion of this segment, A x x+dx C Young’s Modulus Mass Acceleration = Net Force resulting from stress

Equation of Motion So, this becomes: Cancelling common terms in Adx gives: This is the wave equation a plane wave solution which gives the sound velocity vs: Plane wave solution: k = wave number = (2π/λ), ω = frequency, A = amplitude

• Unlike the case for the discrete lattice, the dispersion relation ω(k) in this long wavelength limit is the simple equation: • At small λ (k → ∞), scattering from discrete atoms occurs. • At long λ (k → 0), (continuum) no scattering occurs. • When k increases the sound velocity decreases. • As k increases further, the scattering becomes greater since the strength of scattering increases as the wavelength decreases, and the velocity decreases even further.

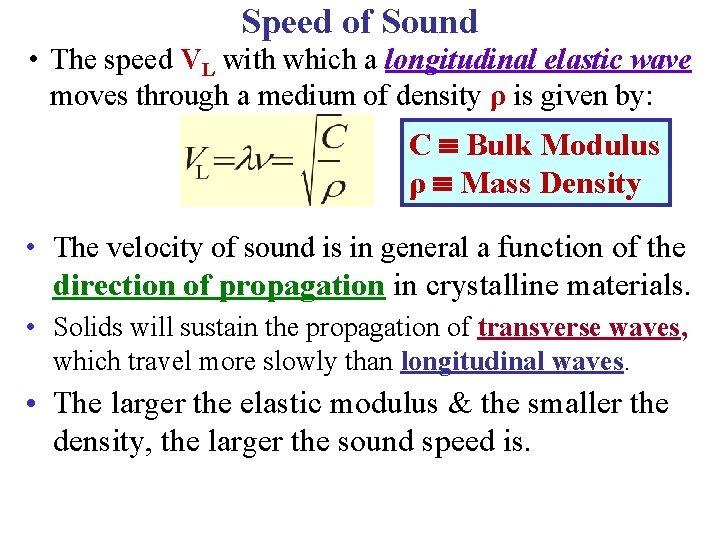
Speed of Sound • The speed VL with which a longitudinal elastic wave moves through a medium of density ρ is given by: C Bulk Modulus ρ Mass Density • The velocity of sound is in general a function of the direction of propagation in crystalline materials. • Solids will sustain the propagation of transverse waves, which travel more slowly than longitudinal waves. • The larger the elastic modulus & the smaller the density, the larger the sound speed is.

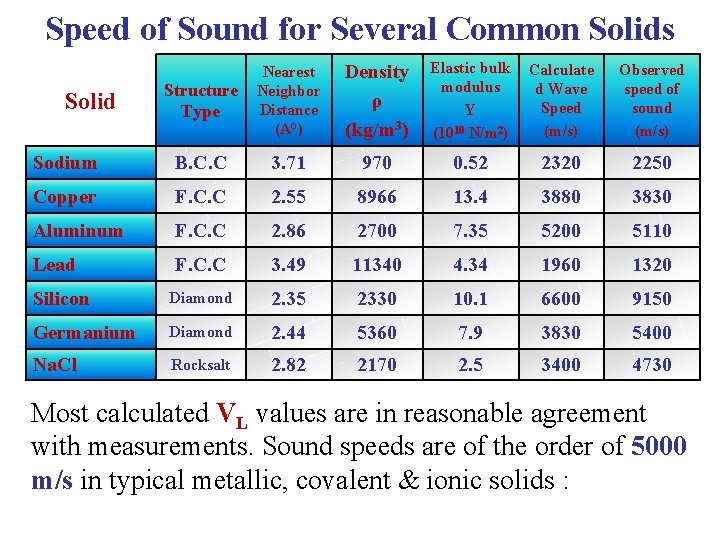
Speed of Sound for Several Common Solids Structure Type Nearest Neighbor Distance (A°) Density ρ (kg/m 3) Elastic bulk modulus Y (1010 N/m 2) Calculate d Wave Speed (m/s) Observed speed of sound (m/s) Sodium B. C. C 3. 71 970 0. 52 2320 2250 Copper F. C. C 2. 55 8966 13. 4 3880 3830 Aluminum F. C. C 2. 86 2700 7. 35 5200 5110 Lead F. C. C 3. 49 11340 4. 34 1960 1320 Silicon Diamond 2. 35 2330 10. 1 6600 9150 Germanium Diamond 2. 44 5360 7. 9 3830 5400 Na. Cl Rocksalt 2. 82 2170 2. 5 3400 4730 Solid Most calculated VL values are in reasonable agreement with measurements. Sound speeds are of the order of 5000 m/s in typical metallic, covalent & ionic solids :