Viscosit Equazioni di Navier Stokes Viscous stresses are

Viscosità • Equazioni di Navier Stokes

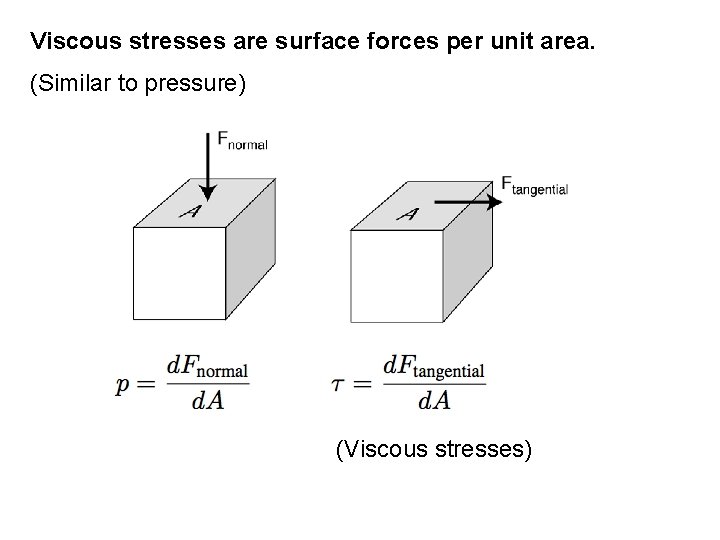
Viscous stresses are surface forces per unit area. (Similar to pressure) (Viscous stresses)

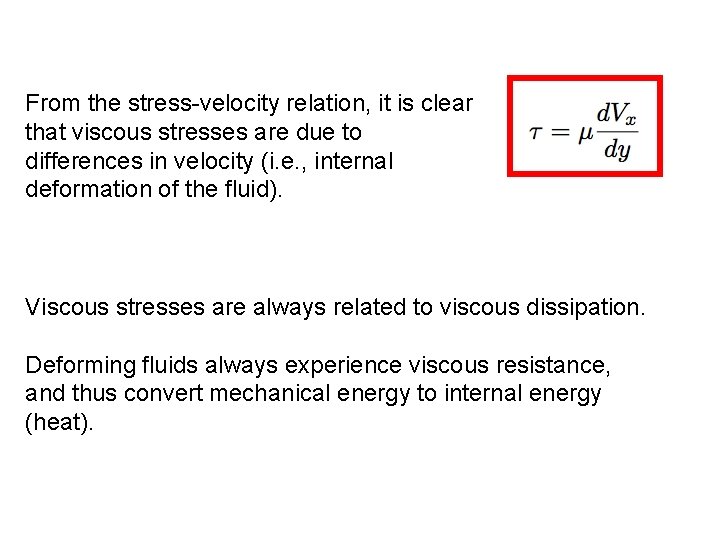
From the stress-velocity relation, it is clear that viscous stresses are due to differences in velocity (i. e. , internal deformation of the fluid). Viscous stresses are always related to viscous dissipation. Deforming fluids always experience viscous resistance, and thus convert mechanical energy to internal energy (heat).

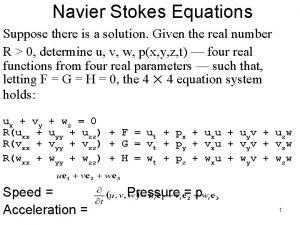
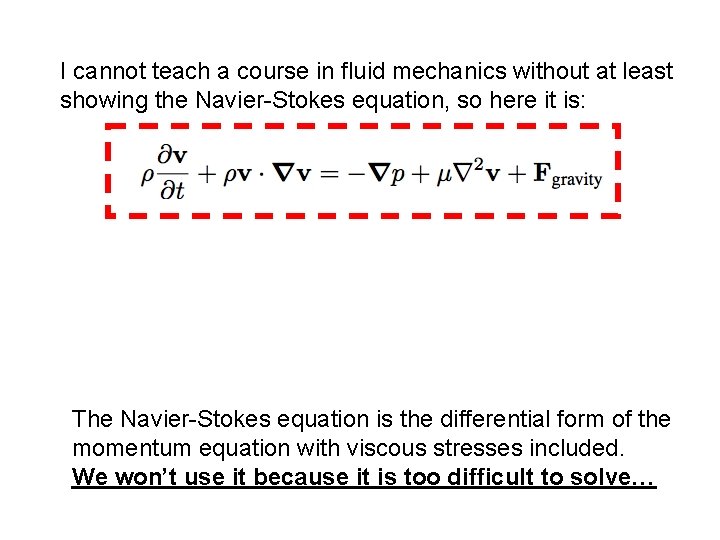
I cannot teach a course in fluid mechanics without at least showing the Navier-Stokes equation, so here it is: The Navier-Stokes equation is the differential form of the momentum equation with viscous stresses included. We won’t use it because it is too difficult to solve…

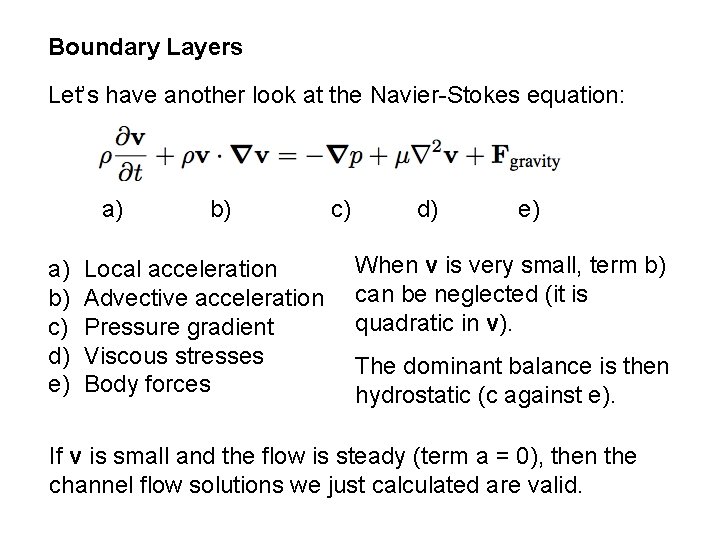
Boundary Layers Let’s have another look at the Navier-Stokes equation: a) a) b) c) d) e) b) Local acceleration Advective acceleration Pressure gradient Viscous stresses Body forces c) d) e) When v is very small, term b) can be neglected (it is quadratic in v). The dominant balance is then hydrostatic (c against e). If v is small and the flow is steady (term a = 0), then the channel flow solutions we just calculated are valid.

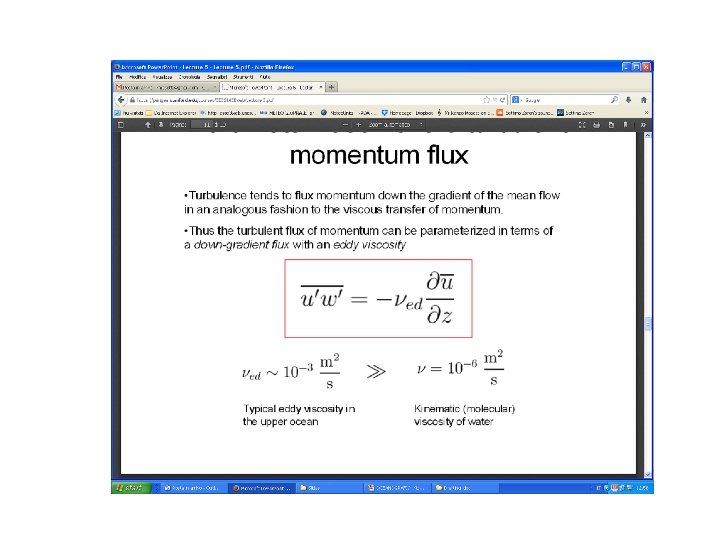
However, when v is larger, term b) can no longer be left out. Term b) is the term that leads to turbulence. Near the boundaries, v is small and term b) may be neglected (laminar flow).

A bit of scaling (we skipped this in chapter 8) Introduce typical scales for the variables, such as: 1) Length scale L = pipe diameter (or radius…) 2) Distance over which velocity changes. 2) Velocity scale V = mean flow velocity (or maximum…) Concentrating on terms b) and d), we find b) d)

To compare the terms, we take the ratio of b) to d): This estimate of the relative importance of the two terms has great relevance and has its own name: Reynolds number: When Re is very small, term b) can be neglected Laminar flow! When Re is very large, term d) can be neglected Fully turbulent flow! When Re is around 1, both terms are important.

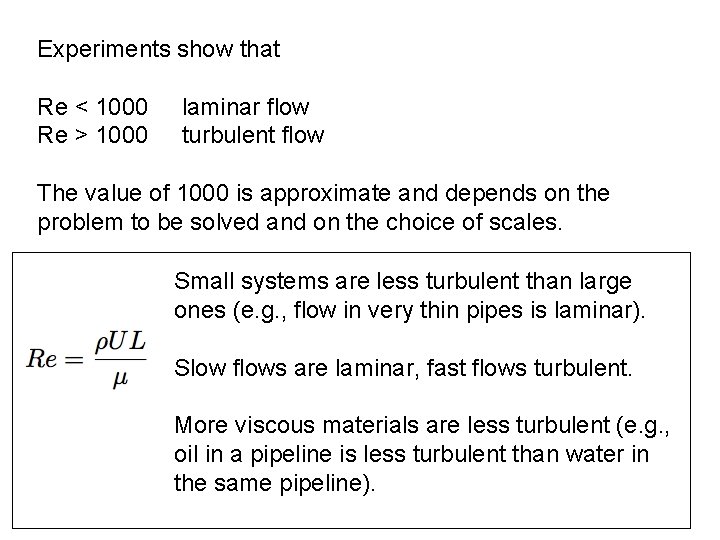
Experiments show that Re < 1000 Re > 1000 laminar flow turbulent flow The value of 1000 is approximate and depends on the problem to be solved and on the choice of scales. Small systems are less turbulent than large ones (e. g. , flow in very thin pipes is laminar). Slow flows are laminar, fast flows turbulent. More viscous materials are less turbulent (e. g. , oil in a pipeline is less turbulent than water in the same pipeline).