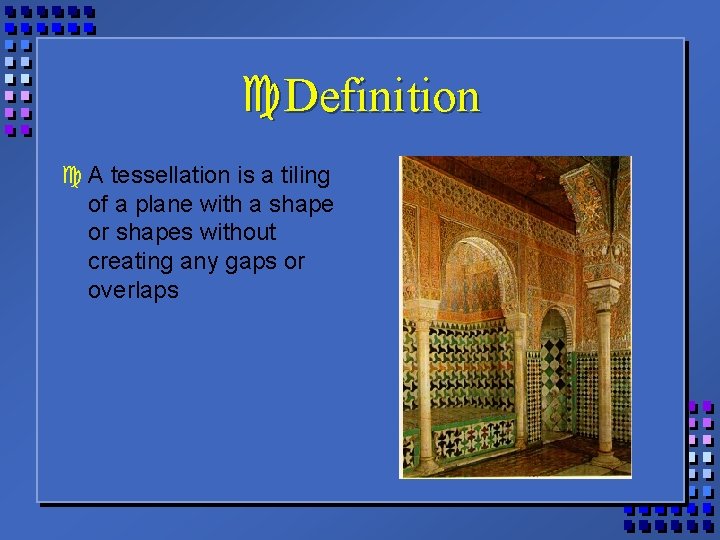
TESSELLATIONS c Definition c A tessellation is a

TESSELLATIONS

c. Definition c A tessellation is a tiling of a plane with a shape or shapes without creating any gaps or overlaps

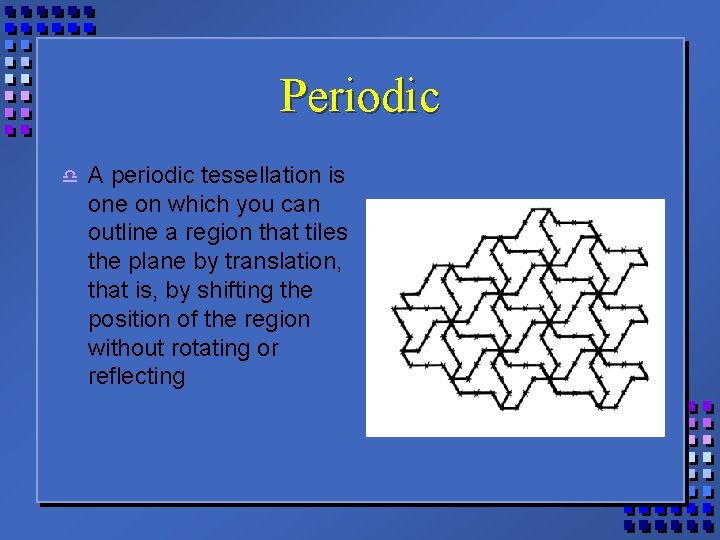
Periodic d A periodic tessellation is one on which you can outline a region that tiles the plane by translation, that is, by shifting the position of the region without rotating or reflecting

Periodic: Regular d d d A regular tessellation is a tiling in which only one shape is used The shape must have congruent sides and congruent angles There exists exactly three regular tessellations

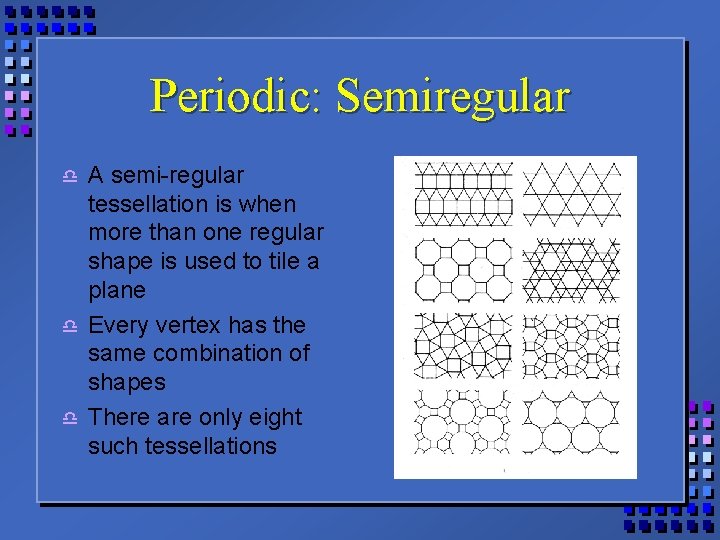
Periodic: Semiregular d d d A semi-regular tessellation is when more than one regular shape is used to tile a plane Every vertex has the same combination of shapes There are only eight such tessellations

Periodic: Not Semiregular d The vertices do not have the same combination of shapes A B A (3. 4. 6. 4) B (3. 3. 4)

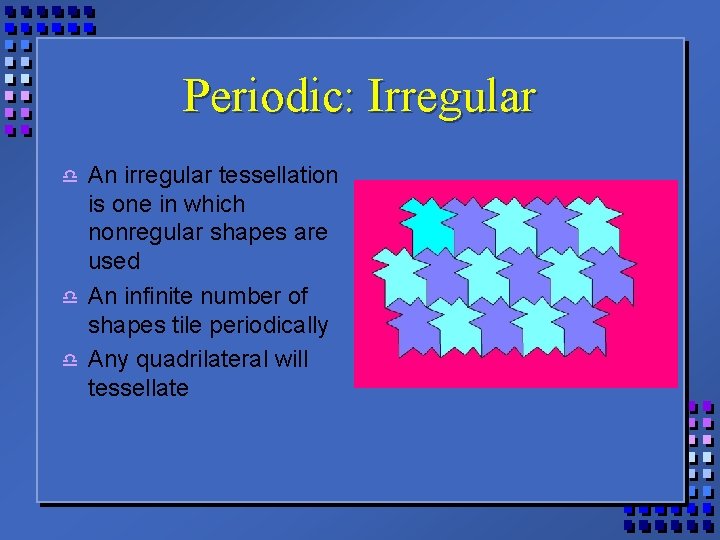
Periodic: Irregular d d d An irregular tessellation is one in which nonregular shapes are used An infinite number of shapes tile periodically Any quadrilateral will tessellate

Escher d M. C. Escher, the Dutch artist, is famous for his many pictures of periodic tilings with shapes that resemble living things

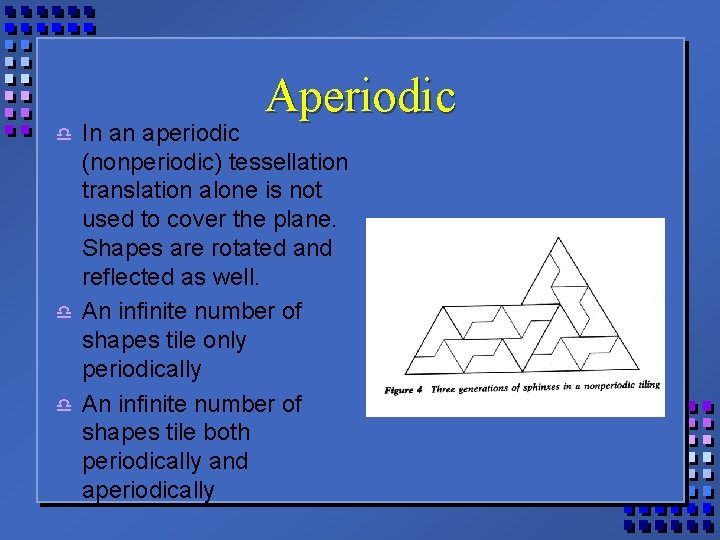
Aperiodic d d d In an aperiodic (nonperiodic) tessellation translation alone is not used to cover the plane. Shapes are rotated and reflected as well. An infinite number of shapes tile only periodically An infinite number of shapes tile both periodically and aperiodically

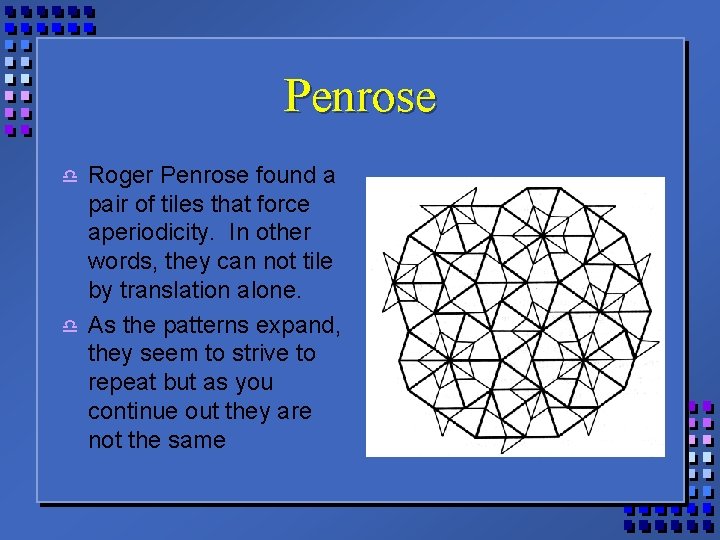
Penrose d d Roger Penrose found a pair of tiles that force aperiodicity. In other words, they can not tile by translation alone. As the patterns expand, they seem to strive to repeat but as you continue out they are not the same

Tessellations d d Periodic – Regular – Semiregular – Not Semiregular – Irregular Aperiodic

Credits Produced by Dottie Feinstein © 1997 (2007) Conti, Shrines of Power, HBJ Press, 1978 Gardner, Penrose Tiles to Trapdoor Ciphers, W. H. Freeman & Co. , 1989 Tessellation Poster: Teaching Notes, Dale Seymour, 1987

For more information see http: //www. Science. U. com/geometry/articles/tiling/ http: //library. thinkquest. org/