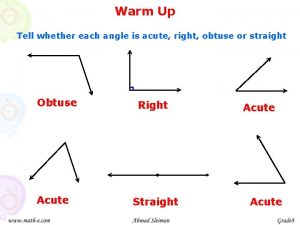
Complementary and Supplementary Angles Complementary Angles Two angles

Complementary Angles • Two angles are complementary if the sum of their angles equals

Example of Complementary Angles • What is the complementary angle of 43*? – SOLUTION:

How can I remember that? • Draw the C in Complementary C • Since

Another way to remember… • Just remember this phrase: “It is always RIGHT to

Click on the link below to manipulate different angles that are Supplementary. http: //www.

Supplementary Angles • Two angles are supplementary if the sum of their angles equals

Example of Supplementary Angles • What is the supplementary angle of 143*? – SOLUTION:

How can I remember that? • Draw the S in Supplementary S • Since

Slides: 10

Download presentation

Complementary and Supplementary Angles

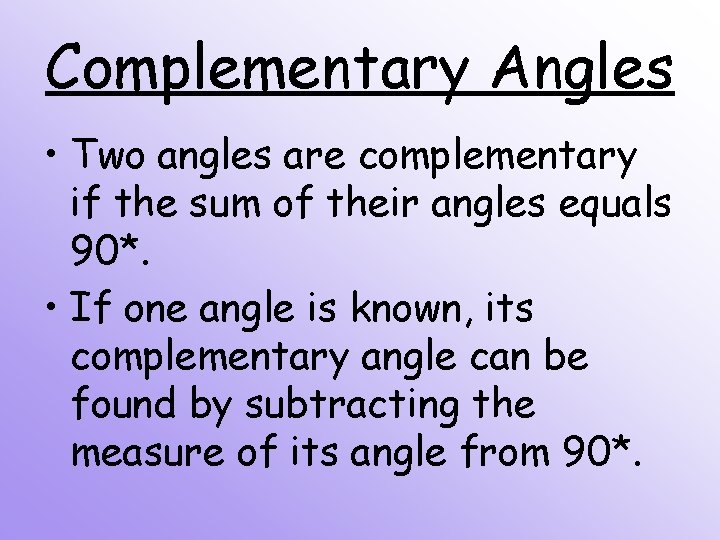
Complementary Angles • Two angles are complementary if the sum of their angles equals 90*. • If one angle is known, its complementary angle can be found by subtracting the measure of its angle from 90*.

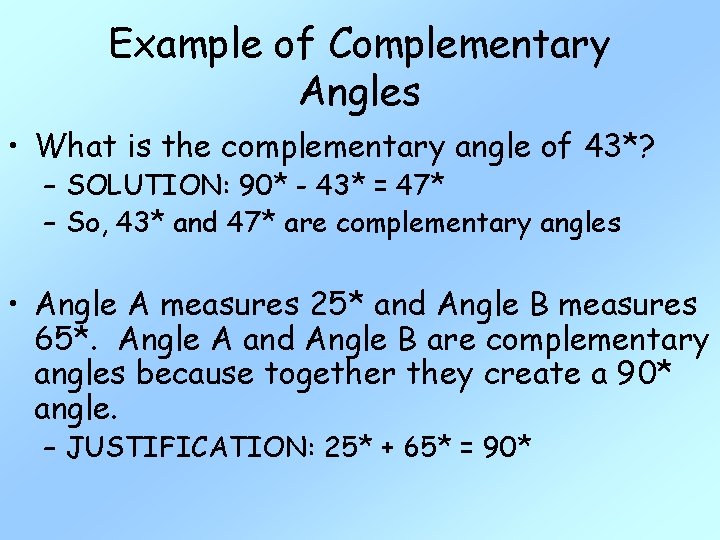
Example of Complementary Angles • What is the complementary angle of 43*? – SOLUTION: 90* - 43* = 47* – So, 43* and 47* are complementary angles • Angle A measures 25* and Angle B measures 65*. Angle A and Angle B are complementary angles because together they create a 90* angle. – JUSTIFICATION: 25* + 65* = 90*

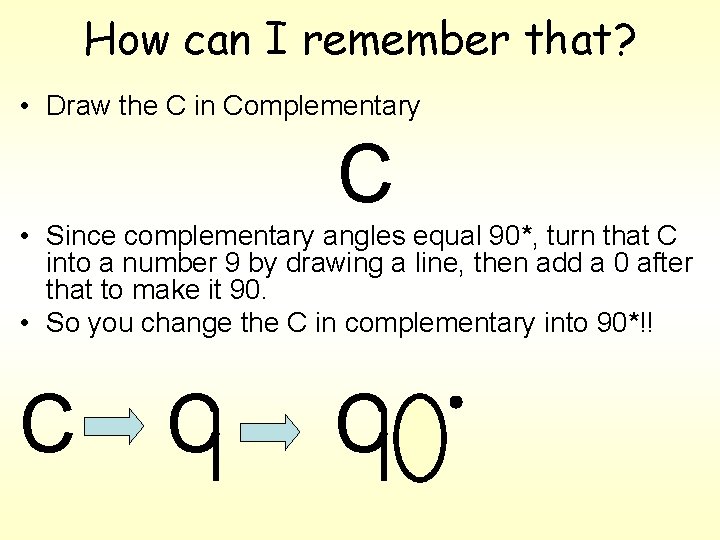
How can I remember that? • Draw the C in Complementary C • Since complementary angles equal 90*, turn that C into a number 9 by drawing a line, then add a 0 after that to make it 90. • So you change the C in complementary into 90*!! C C C

Another way to remember… • Just remember this phrase: “It is always RIGHT to give COMPLIMENTS” • A RIGHT angle is 90* and COMPLIMENT and COMPLEMENTARY sound alike

Click on the link below to manipulate different angles that are Supplementary. http: //www. mathopenref. com/a nglecomplementary. html

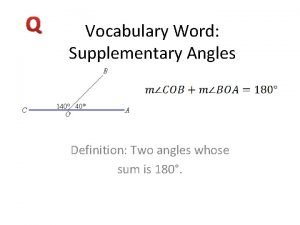
Supplementary Angles • Two angles are supplementary if the sum of their angles equals 180*. • If one angle is known, its supplementary angle can be found by subtracting the measure of its angle from 180*.

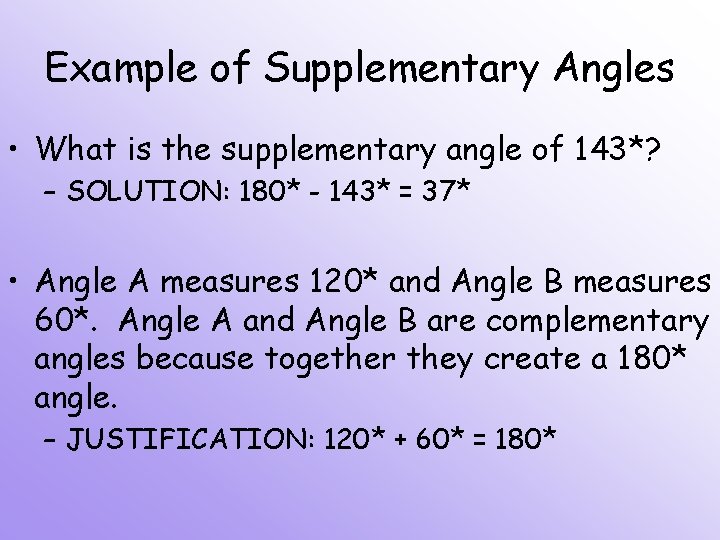
Example of Supplementary Angles • What is the supplementary angle of 143*? – SOLUTION: 180* - 143* = 37* • Angle A measures 120* and Angle B measures 60*. Angle A and Angle B are complementary angles because together they create a 180* angle. – JUSTIFICATION: 120* + 60* = 180*

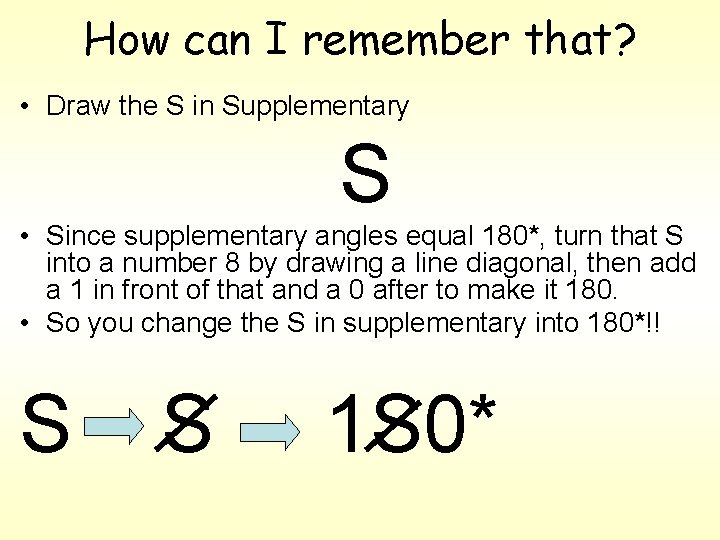
How can I remember that? • Draw the S in Supplementary S • Since supplementary angles equal 180*, turn that S into a number 8 by drawing a line diagonal, then add a 1 in front of that and a 0 after to make it 180. • So you change the S in supplementary into 180*!! S S 1 S 0*

Click on the link below to manipulate different angles that are Supplementary. http: //www. mathopenref. com/angl esupplementary. html