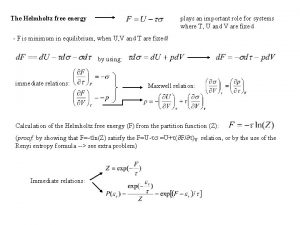
The Helmholtz free energy plays an important role

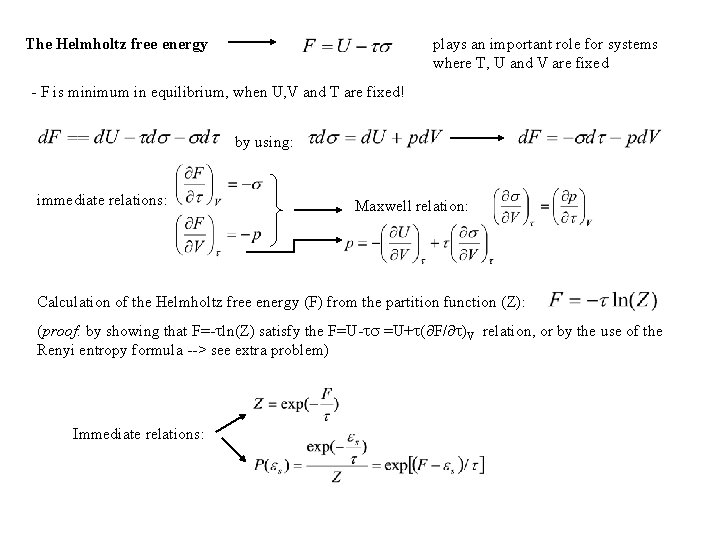
The Helmholtz free energy plays an important role for systems where T, U and V are fixed - F is minimum in equilibrium, when U, V and T are fixed! by using: immediate relations: Maxwell relation: Calculation of the Helmholtz free energy (F) from the partition function (Z): (proof: by showing that F=- ln(Z) satisfy the F=U- =U+ ( F/ )V relation, or by the use of the Renyi entropy formula --> see extra problem) Immediate relations:

. One atom in a box Ideal gas: A first look We first calculate the partition function (Z 1) of one atom of mass M free to move in a cubical box of volume V=L 3 Wave functions of possible states: Energies of possible states: where (nx , ny , nz : positive integers) after performing the integrals: We can introduce the so called quantum concentration, which is rouhgly the concentration of one atom in a cube of side equal to thermal average de Broglie wavelength. ( ) Whenever n<<n. Q --> classical regime. An ideal gas is defined as a gas of nonintearcting atoms in the classical regime! Average internal energy of one particle Thermal average occupancy of one state: (for the classical regime, this must be <<1!)

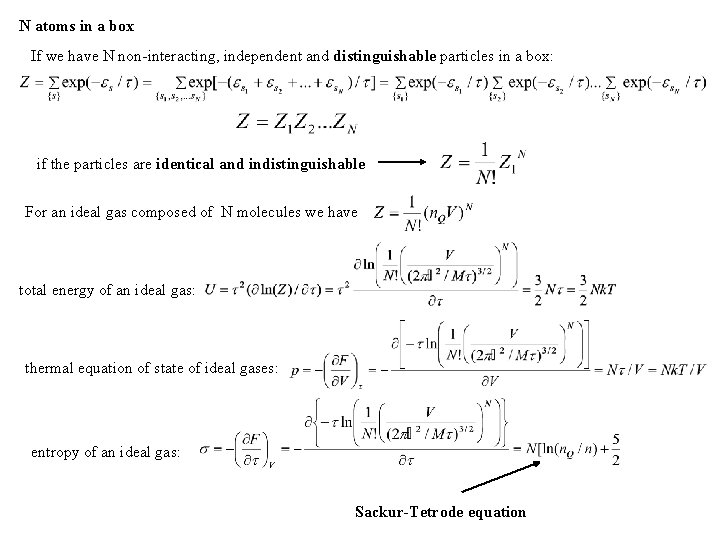
N atoms in a box If we have N non-interacting, independent and distinguishable particles in a box: if the particles are identical and indistinguishable For an ideal gas composed of N molecules we have total energy of an ideal gas: thermal equation of state of ideal gases: entropy of an ideal gas: Sackur-Tetrode equation

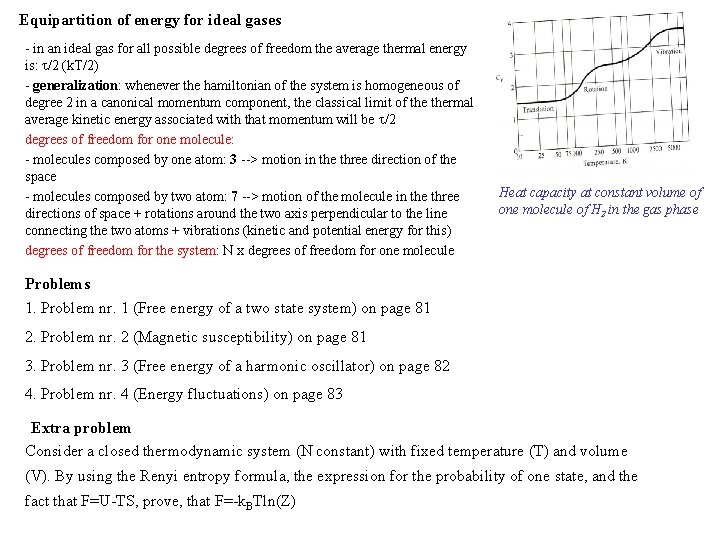
Equipartition of energy for ideal gases - in an ideal gas for all possible degrees of freedom the average thermal energy is: /2 (k. T/2) - generalization: whenever the hamiltonian of the system is homogeneous of degree 2 in a canonical momentum component, the classical limit of thermal average kinetic energy associated with that momentum will be /2 degrees of freedom for one molecule: - molecules composed by one atom: 3 --> motion in the three direction of the space - molecules composed by two atom: 7 --> motion of the molecule in the three directions of space + rotations around the two axis perpendicular to the line connecting the two atoms + vibrations (kinetic and potential energy for this) degrees of freedom for the system: N x degrees of freedom for one molecule Heat capacity at constant volume of one molecule of H 2 in the gas phase Problems 1. Problem nr. 1 (Free energy of a two state system) on page 81 2. Problem nr. 2 (Magnetic susceptibility) on page 81 3. Problem nr. 3 (Free energy of a harmonic oscillator) on page 82 4. Problem nr. 4 (Energy fluctuations) on page 83 Extra problem Consider a closed thermodynamic system (N constant) with fixed temperature (T) and volume (V). By using the Renyi entropy formula, the expression for the probability of one state, and the fact that F=U-TS, prove, that F=-k. BTln(Z)