Engineering Economics I Interest and Equivalence Engineering Economics

Engineering Economics I Interest and Equivalence

Engineering Economics • Engineering Economics is the application of standard set of equivalence equations in determining the relative economic value of a small set of alternative capital investments that differ in their time streams of costs and benefits. • As a civil engineer you may need to determine the financial feasibility of the project or the most economical design alternative. • The information covered here is needed for the (FE) and the (PE) exams.

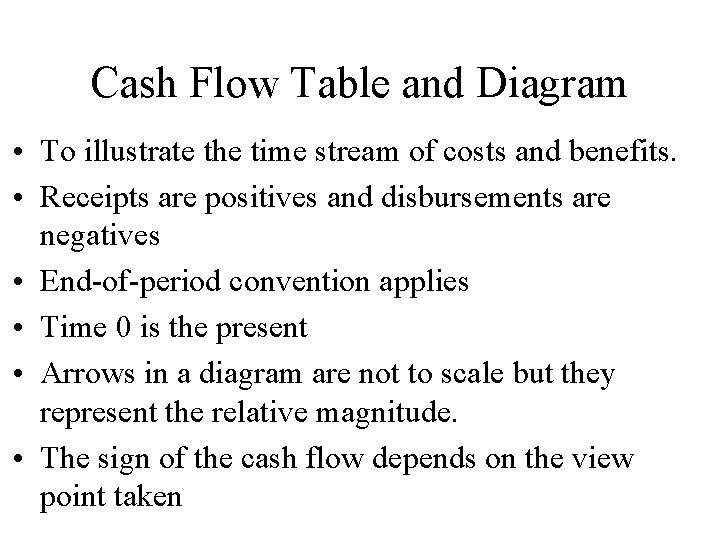
Cash Flow Table and Diagram • To illustrate the time stream of costs and benefits. • Receipts are positives and disbursements are negatives • End-of-period convention applies • Time 0 is the present • Arrows in a diagram are not to scale but they represent the relative magnitude. • The sign of the cash flow depends on the view point taken

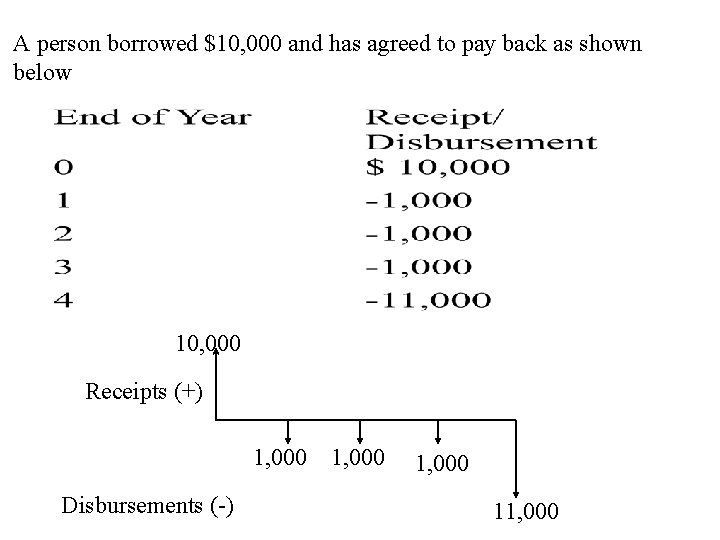
A person borrowed $10, 000 and has agreed to pay back as shown below 10, 000 Receipts (+) 1, 000 Disbursements (-) 1, 000 11, 000

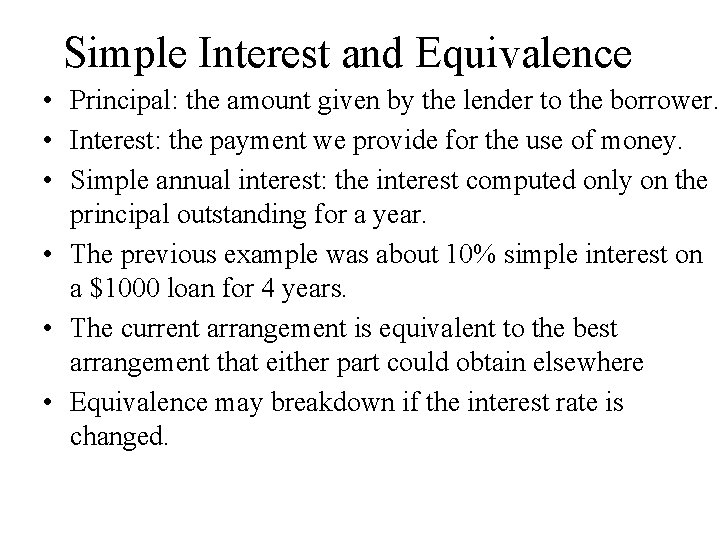
Simple Interest and Equivalence • Principal: the amount given by the lender to the borrower. • Interest: the payment we provide for the use of money. • Simple annual interest: the interest computed only on the principal outstanding for a year. • The previous example was about 10% simple interest on a $1000 loan for 4 years. • The current arrangement is equivalent to the best arrangement that either part could obtain elsewhere • Equivalence may breakdown if the interest rate is changed.

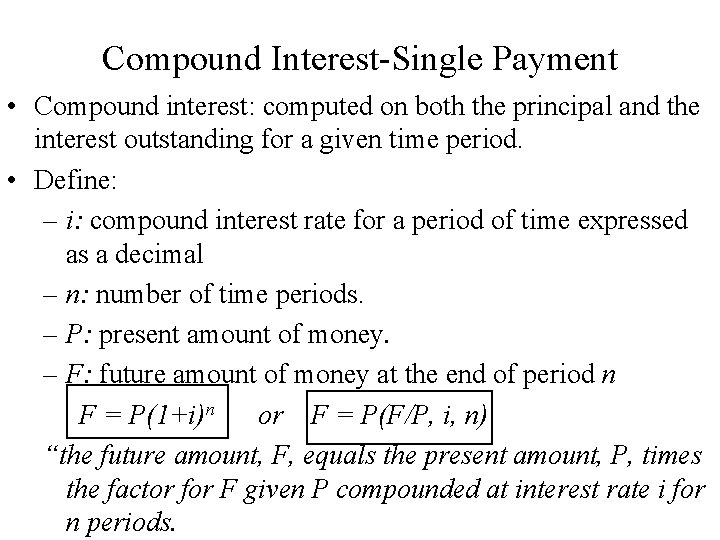
Compound Interest-Single Payment • Compound interest: computed on both the principal and the interest outstanding for a given time period. • Define: – i: compound interest rate for a period of time expressed as a decimal – n: number of time periods. – P: present amount of money. – F: future amount of money at the end of period n F = P(1+i)n or F = P(F/P, i, n) “the future amount, F, equals the present amount, P, times the factor for F given P compounded at interest rate i for n periods.

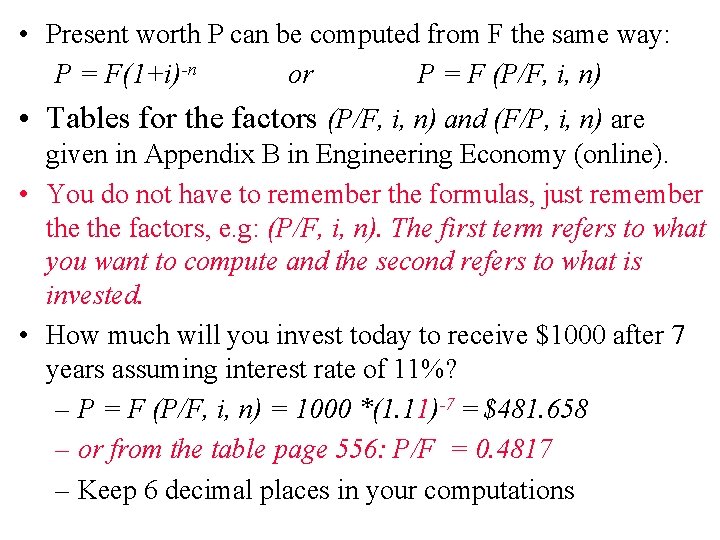
• Present worth P can be computed from F the same way: P = F(1+i)-n or P = F (P/F, i, n) • Tables for the factors (P/F, i, n) and (F/P, i, n) are given in Appendix B in Engineering Economy (online). • You do not have to remember the formulas, just remember the factors, e. g: (P/F, i, n). The first term refers to what you want to compute and the second refers to what is invested. • How much will you invest today to receive $1000 after 7 years assuming interest rate of 11%? – P = F (P/F, i, n) = 1000 *(1. 11)-7 = $481. 658 – or from the table page 556: P/F = 0. 4817 – Keep 6 decimal places in your computations

• Rule of 72: – if (i X n = 72), future factor is » 2, money will double • Nominal and effective interest rate – the nominal interest rate for a time period ignores the effect of any subperiod compounding. – effective interest rate takes into account the accumulation of interest: 4% computed quarterly means that the interest is computed and added to the principal every three months. – if r is the nominal interest rate per time period; and m: number of compound subperiods per time period. then effective annual interest rate iy = (1+ )m - 1 – What is the effective annual interest rate of 7% nominal interest compounded monthly? the answer is 7. 23%

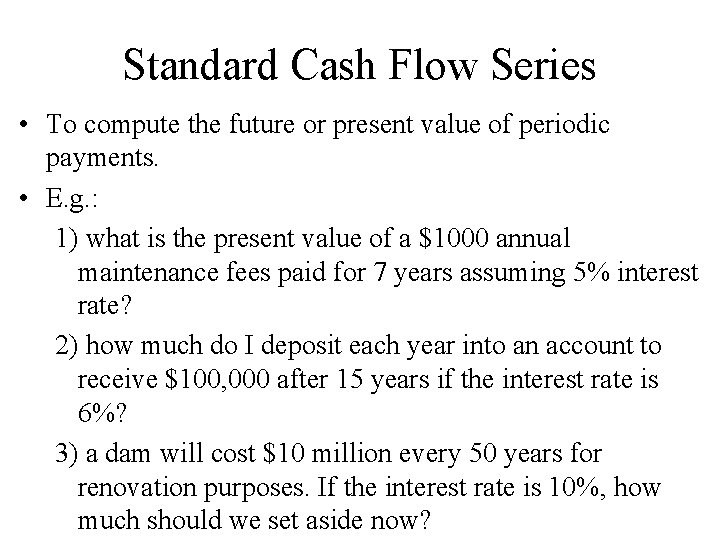
Standard Cash Flow Series • To compute the future or present value of periodic payments. • E. g. : 1) what is the present value of a $1000 annual maintenance fees paid for 7 years assuming 5% interest rate? 2) how much do I deposit each year into an account to receive $100, 000 after 15 years if the interest rate is 6%? 3) a dam will cost $10 million every 50 years for renovation purposes. If the interest rate is 10%, how much should we set aside now?

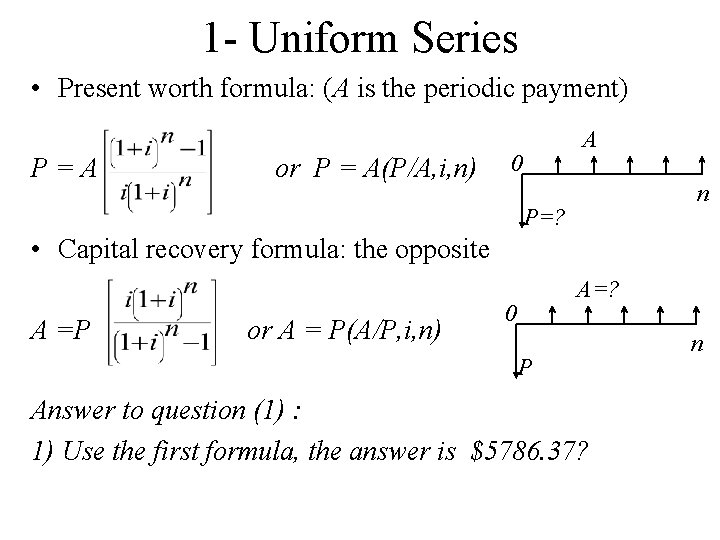
1 - Uniform Series • Present worth formula: (A is the periodic payment) P=A or P = A(P/A, i, n) 0 A n P=? • Capital recovery formula: the opposite A =P or A = P(A/P, i, n) 0 A=? P Answer to question (1) : 1) Use the first formula, the answer is $5786. 37? n

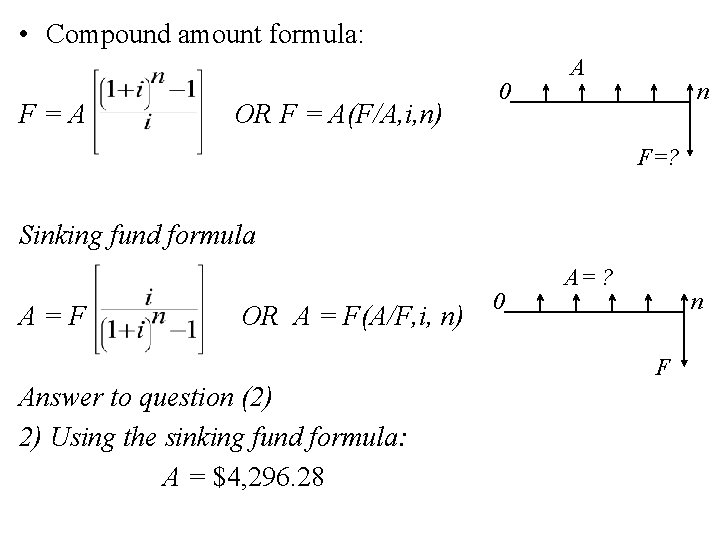
• Compound amount formula: F=A OR F = A(F/A, i, n) 0 A n F=? Sinking fund formula A=F OR A = F(A/F, i, n) 0 A= ? n F Answer to question (2) 2) Using the sinking fund formula: A = $4, 296. 28

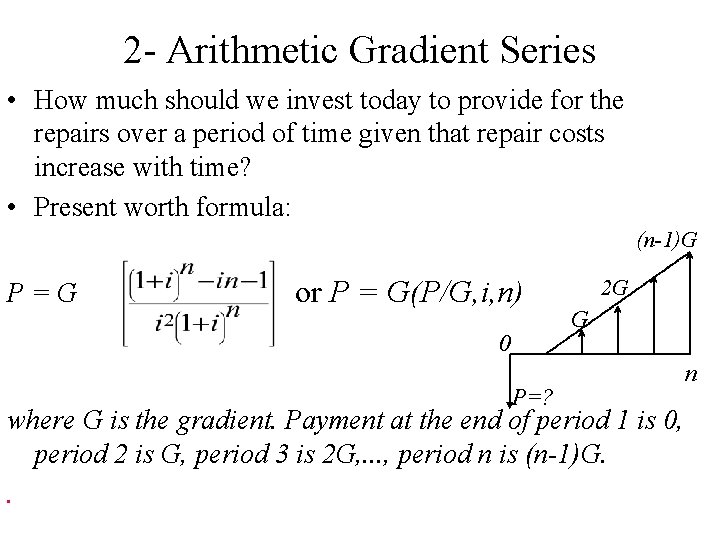
2 - Arithmetic Gradient Series • How much should we invest today to provide for the repairs over a period of time given that repair costs increase with time? • Present worth formula: (n-1)G P=G or P = G(P/G, i, n) 0 P=? 2 G G where G is the gradient. Payment at the end of period 1 is 0, period 2 is G, period 3 is 2 G, . . . , period n is (n-1)G. . n

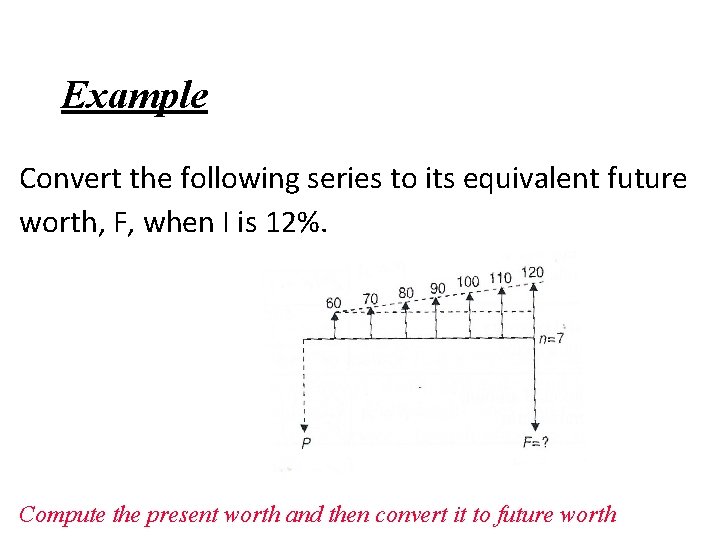
Example Convert the following series to its equivalent future worth, F, when I is 12%. Compute the present worth and then convert it to future worth

3 - Capitalized Cost • When it is necessary to assume that a certain structure or service will provide for ever. E. g. . : highways, pipelines, and dams. • The goal is to compute the amount of money that need to be set aside now to provide a fixed payment at certain intervals, for repairs ? • If we know the annual amount needed (A), the present investment P = A/ i. . . . (1) • If we know the amount needed each set of years (F), 10 million every 50 years for example, we first compute the annual payment A = F(A/F, i, n) then use formula (1) • Example 13 -8

Example A dam initially costs $30 million and must be renovated every 50 years at a cost of $10 million. Interest is 8%. Find the capitalized cost sufficient to construct and maintain the project in perpetuity.