Work and Energy Work Physics definition of Work

Work and Energy

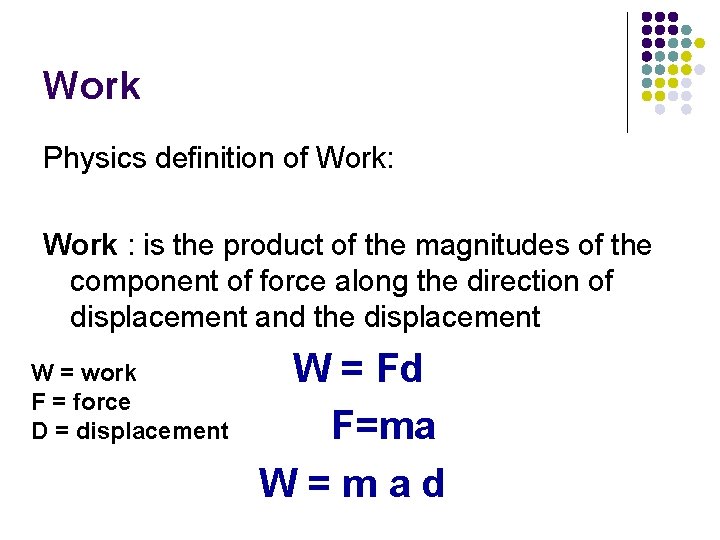
Work Physics definition of Work: Work : is the product of the magnitudes of the component of force along the direction of displacement and the displacement W = work F = force D = displacement W = Fd F=ma W=mad

Work displacement force WORK! displacement Work is done only when components of a force are parallel to a displacement. NO WORK! force

Is Work Happening? l l l A tug of war that is evenly matched A student carries a bucket of water along a horizontal path while walking at a constant velocity. A Crane lifting a car. A person holding a heavy chair at arm’s length for several minutes. A train engine pulling a loaded boxcar initially at rest.

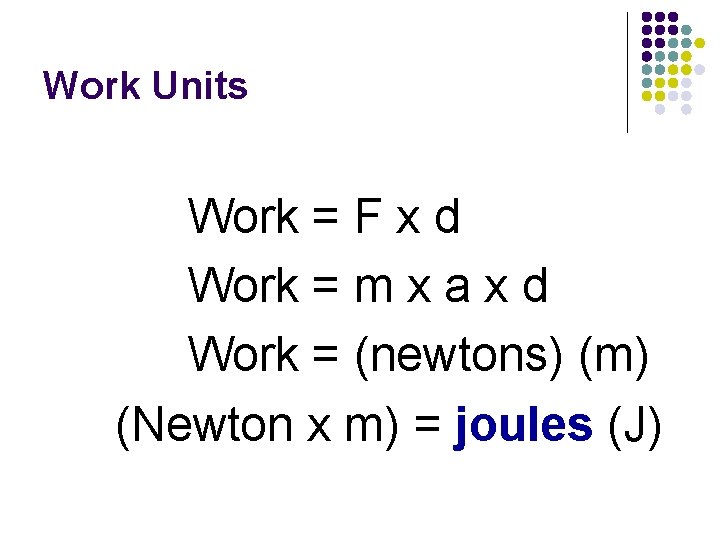
Work Units Work = F x d Work = m x a x d Work = (newtons) (m) (Newton x m) = joules (J)

Work Problem 1 A tugboat pulls a ship with a constant horizontal net force of 5. 00 x 103 N. How much work is done if the ship is pulled a distance of 3. 00 km? W=Fxd = 5. 00 x 10 3 N ( 3000 m) = 1. 5 x 107 Nm or J

Work Problem 2 If 2. 0 J of work is done raising a 180 g apple, how far is it lifted? W =Fd F = mg = 0. 18 kg(9. 8 m/s 2) = 1. 76 N W = Fd 2 J = (1. 76 N)d d = 1. 1 m

Work Problem 3 A weight lifter lifts a set of weights a vertical distance of 2. 00 m. If a constant net force of 350 N is exerted on the weights, what is the net work done on the weights? W = Fd W = 350 N x 2. 00 m = 700 Nm or 700 J

Sample Problem 4 What work is done by a forklift raising 583 kgs of frozen turkeys 1. 2 m? W = Fd F = 583 kg (9. 8 m/s 2) = 5713. 4 N W = 5713. 4 N ( 1. 2 m) = 6856 Nm or 6856 J

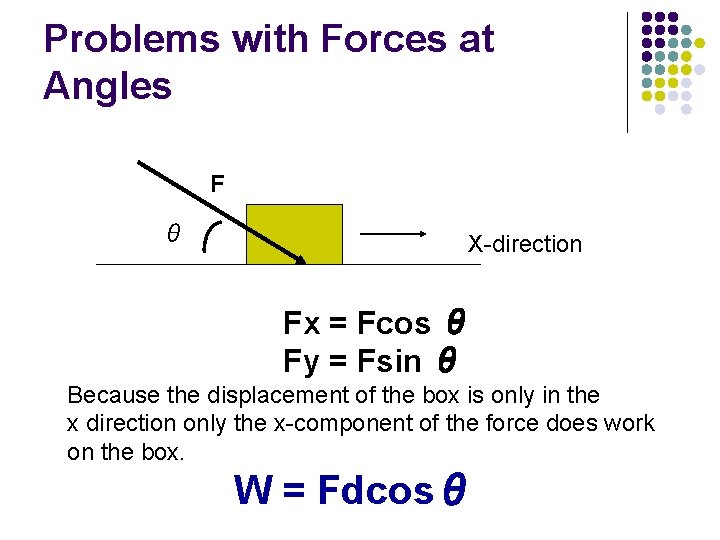
Problems with Forces at Angles F θ X-direction Fx = Fcos θ Fy = Fsin θ Because the displacement of the box is only in the x direction only the x-component of the force does work on the box. W = Fdcosθ

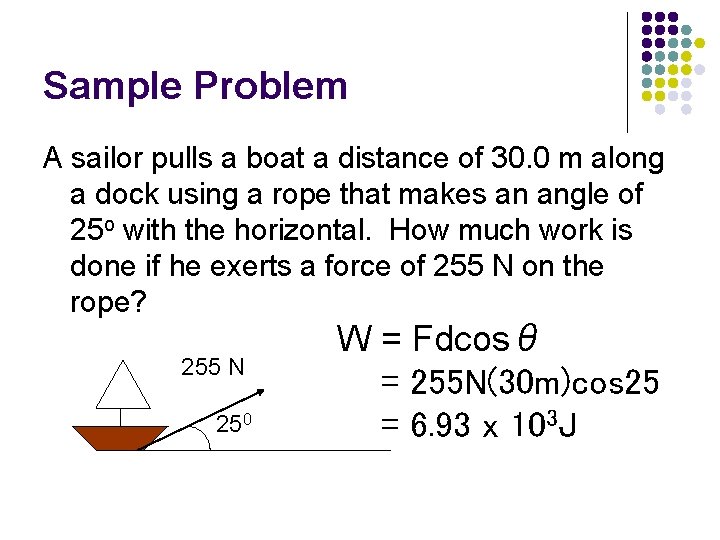
Sample Problem A sailor pulls a boat a distance of 30. 0 m along a dock using a rope that makes an angle of 25 o with the horizontal. How much work is done if he exerts a force of 255 N on the rope? 255 N 250 W = Fdcosθ = 255 N(30 m)cos 25 = 6. 93 x 103 J

Sliding up an Incline l l What we calculated was. . . For sliding an object up an incline. . W = Fd W = (mg sinθ) d

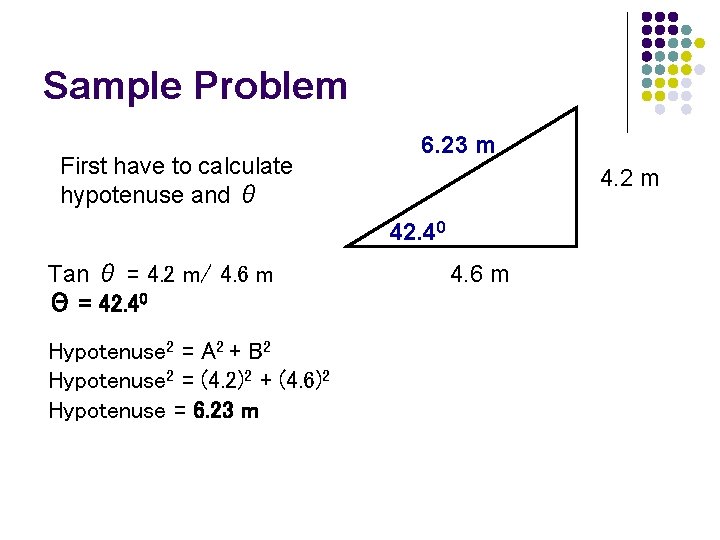
Sample Problem An airline passenger carries a 215 N suitcase up stairs, a displacement of 4. 20 m vertically and 4. 60 m horizontally. How much work does the passenger do? 4. 2 m 4. 6 m

Sample Problem First have to calculate hypotenuse and θ 6. 23 m 4. 2 m 42. 40 Tan θ = 4. 2 m/ 4. 6 m Θ = 42. 40 Hypotenuse 2 = A 2 + B 2 Hypotenuse 2 = (4. 2)2 + (4. 6)2 Hypotenuse = 6. 23 m 4. 6 m

Sample Problem 23 6. m Suitcase weighs 215 N 4. 2 m 42. 40 4. 6 m F║ = mg sinθ = 215 sin 42. 4 = 145 N W = Fd = 145 N ( 6. 23 m) = 899 Nm = 903 J This should equal the force of the suitcase moving it vertically 4. 2 m W = 215 N ( 4. 2 m) = 903 J

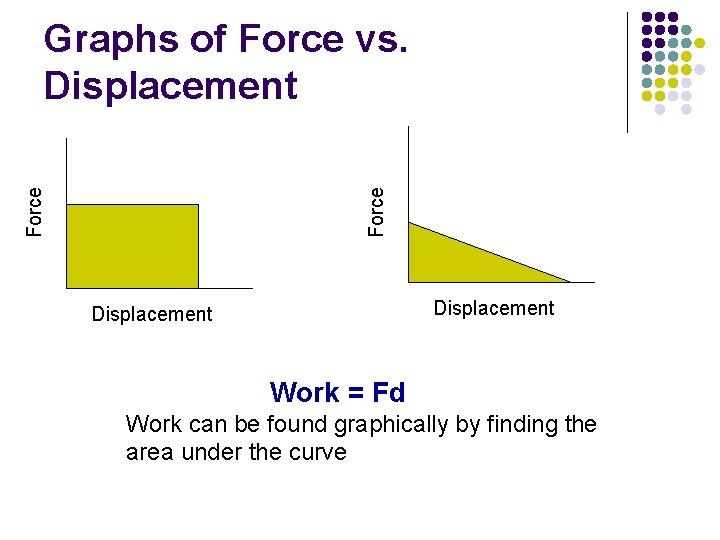
Force Graphs of Force vs. Displacement Work = Fd Work can be found graphically by finding the area under the curve

Homework l Do Work/Energy/Power worksheet #1 -4