Warmup 1112022 Day 6 1112022 Homework Questions 1112022










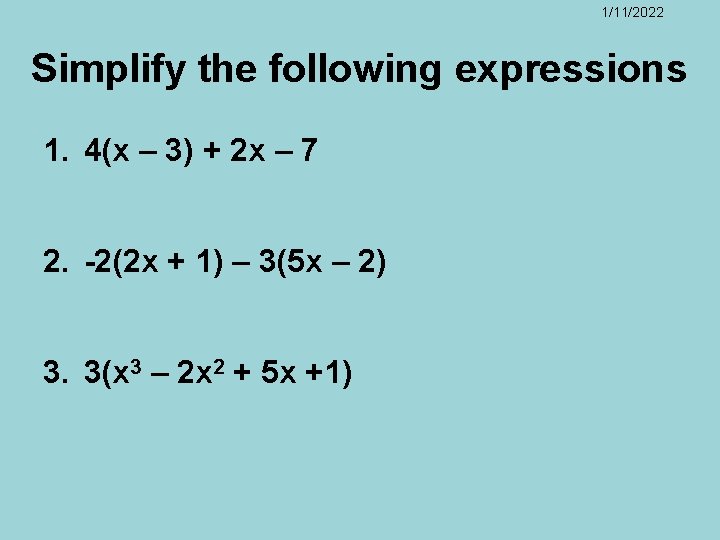



- Slides: 23

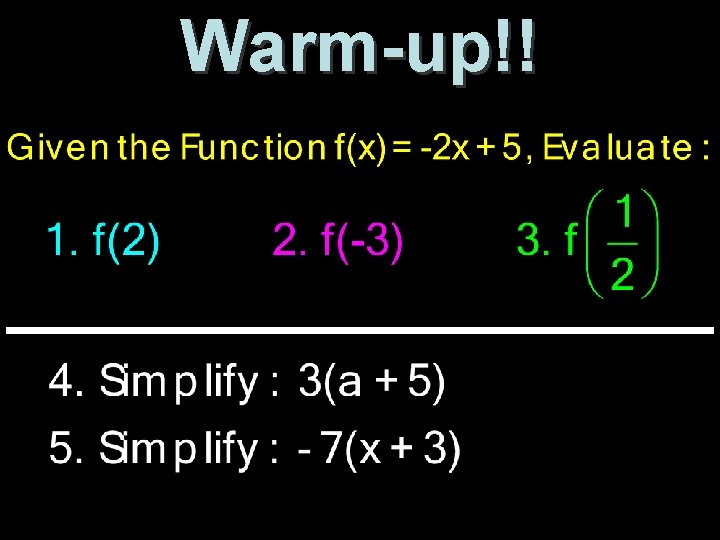
Warm-up!! 1/11/2022 Day 6

1/11/2022 Homework Questions? ? ?

1/11/2022 Day 6 Are you ready for the Skills Check? ?

1/11/2022 State the Domain and Range

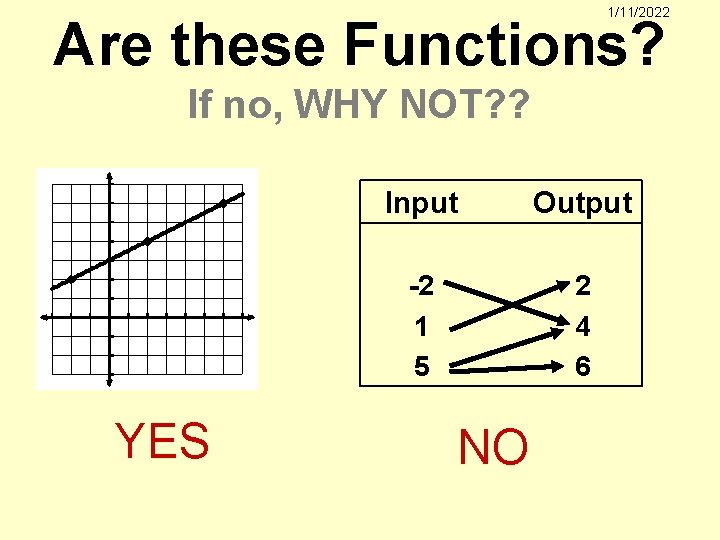
1/11/2022 Are these Functions? If no, WHY NOT? ? YES Input Output -2 1 5 2 4 6 NO

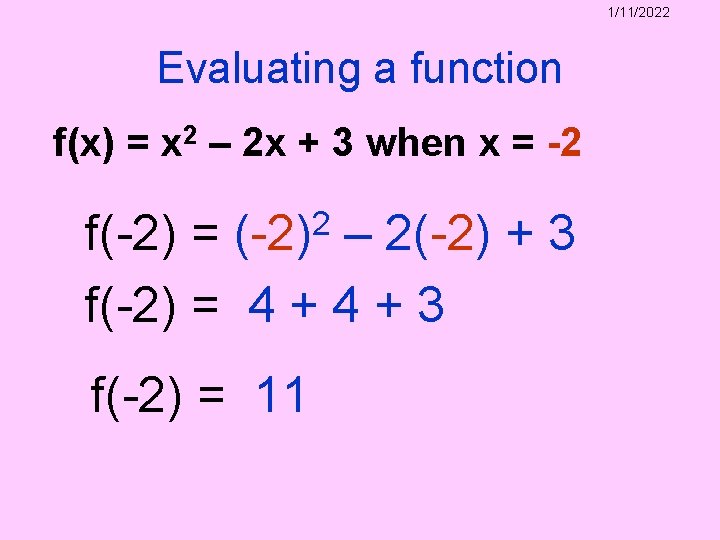
1/11/2022 Evaluating a function f(x) = x 2 – 2 x + 3 when x = -2 2 (-2) f(-2) = – 2(-2) + 3 f(-2) = 4 + 3 f(-2) = 11

1/11/2022 Day 6 Skills Check

GSE Algebra I UNIT QUESTION: How can we use realworld situations to construct and compare linear and exponential models and solve problems? Standard: MGSE. APR. 1 – add and subtract polynomials. Today’s Question: How do we combine like terms?

Adding and Subtracting Polynomials

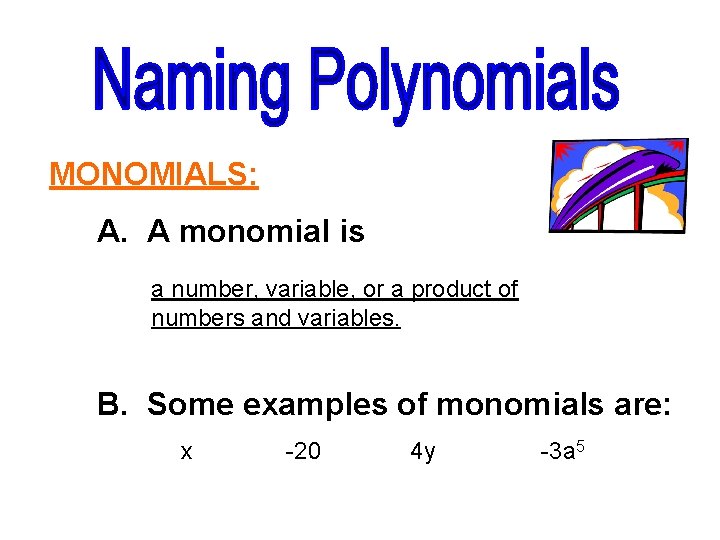
MONOMIALS: A. A monomial is a number, variable, or a product of numbers and variables. B. Some examples of monomials are: x -20 4 y -3 a 5

POLYNOMIALS: A. A polynomial is the ____ monomial or the sum ______ of monomials. B. An example of a polynomial in one variable, x, would be x 3+6 x 2+12 x+8 C. How many MONOMIALS are there in the above polynomial? 4

The _____exponent in the polynomial largest determines the DEGREE OF A POLYNOMIAL in one variable. A. Example: The degree of – 7 x + 9 – 2 4 x 2 is ____ because ___ 2 is the largest exponent in the polynomial. B. Example: Find the degree of the following polynomial: x 4 + 6 x 3 + 7 x 5 + 12 x 5

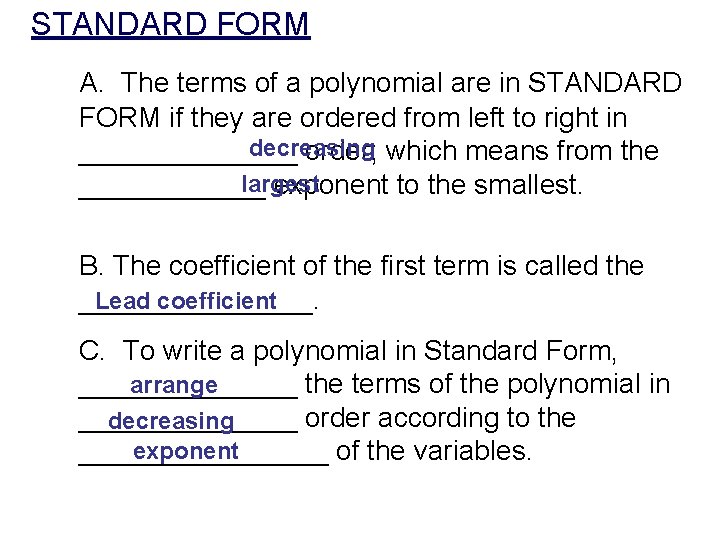
STANDARD FORM A. The terms of a polynomial are in STANDARD FORM if they are ordered from left to right in decreasing _______ order; which means from the largest ______ exponent to the smallest. B. The coefficient of the first term is called the Lead coefficient ________. C. To write a polynomial in Standard Form, arrange _______ the terms of the polynomial in _______ order according to the decreasing exponent ________ of the variables.

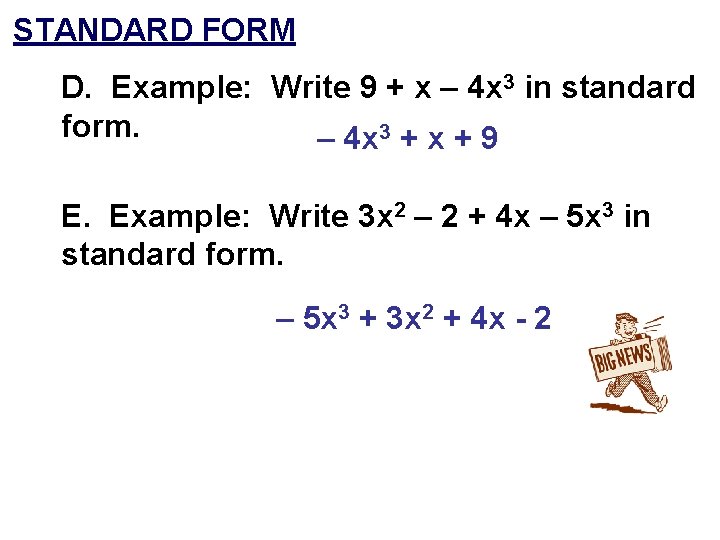
STANDARD FORM D. Example: Write 9 + x – 4 x 3 in standard form. – 4 x 3 + x + 9 E. Example: Write 3 x 2 – 2 + 4 x – 5 x 3 in standard form. – 5 x 3 + 3 x 2 + 4 x - 2

Some polynomials have SPECIAL NAMES that are determined by the following: degree A. Their _____ or B. Their _____ of terms number C. Look at the chart for an explanation.

Polynomial # of Name by # DEGREE Name by Term of Terms Degree s 12 1 Monomial 0 Constant 8 x 1 Monomial 1 Linear 4 x 2 + 3 2 Binomial 2 Quadratic 5 x 3 + x 2 2 Binomial 3 Cubic 3 x 2 – 4 x + 6 3 Trinomial 2 Quadratic

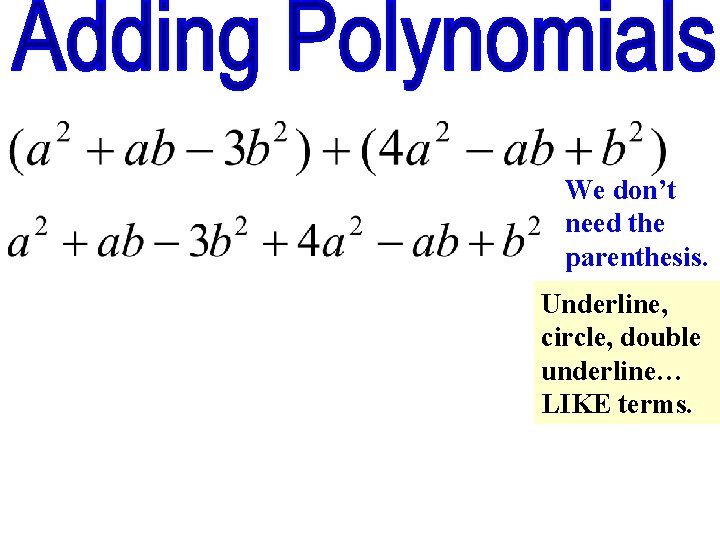
We don’t need the parenthesis. Underline, circle, double underline… LIKE terms.

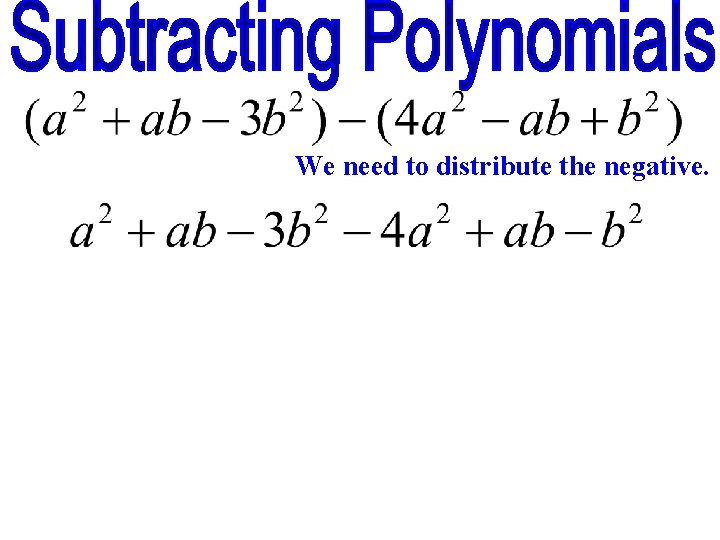
We need to distribute the negative.
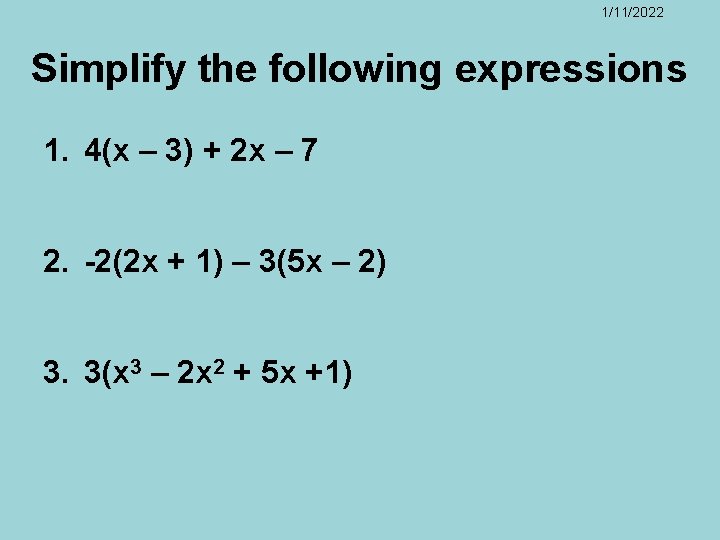
1/11/2022 Simplify the following expressions 1. 4(x – 3) + 2 x – 7 2. -2(2 x + 1) – 3(5 x – 2) 3. 3(x 3 – 2 x 2 + 5 x +1)

Evaluating Functions

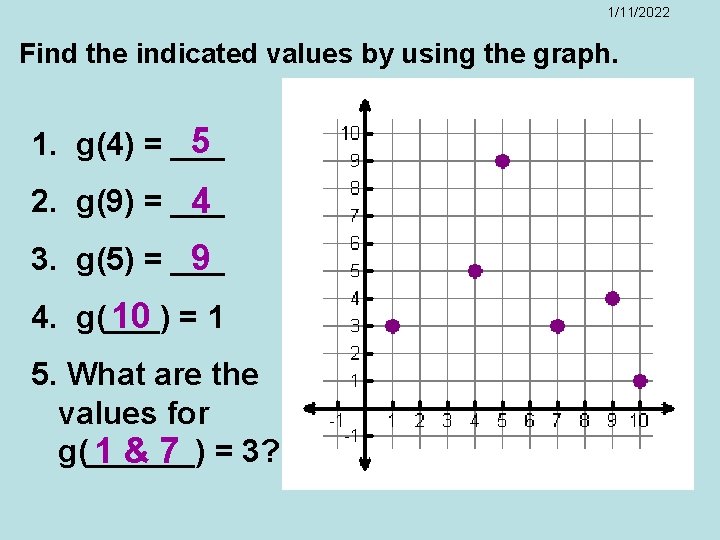
1/11/2022 Find the indicated values by using the graph. 5 1. g(4) = ___ 2. g(9) = ___ 4 3. g(5) = ___ 9 4. g(___) 10 = 1 5. What are the values for g(______) 1 & 7 = 3?

1/11/2022 Find the indicated values by looking in the table. 15 1. h(6) = ___ x h(x) 2. h(2) = ___ 7 0 3 3. h(___) 0 =3 2 7 4. h(___) 4 = 11 4 11 5 5. h(1) = ___ 6 15 9 6. h(3) = ___ 7. h(___) 5 = 13

1/11/2022 Practice WS