Vector Scalar Quantities Characteristics of a Scalar Quantity

Vector & Scalar Quantities

Characteristics of a Scalar Quantity o o Only has magnitude Requires 2 things: 1. A value 2. Appropriate units Ex. Mass: 5 kg Temp: 21° C Speed: 65 mph

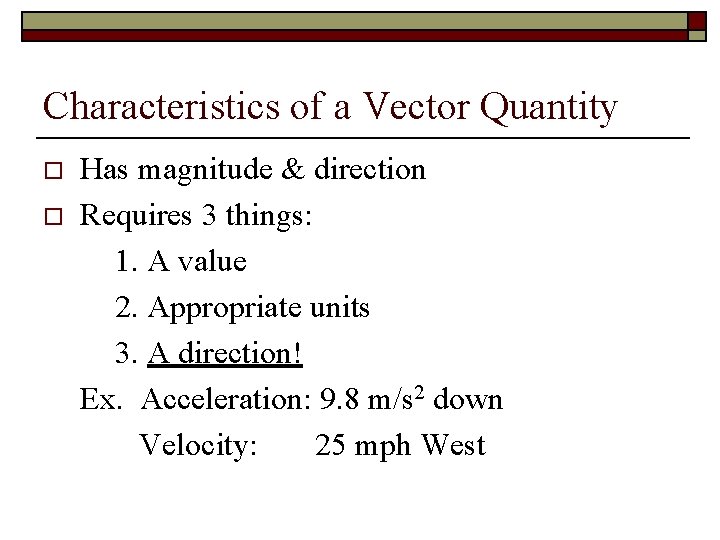
Characteristics of a Vector Quantity o o Has magnitude & direction Requires 3 things: 1. A value 2. Appropriate units 3. A direction! Ex. Acceleration: 9. 8 m/s 2 down Velocity: 25 mph West

More about Vectors o A vector is represented on paper by an arrow 1. the length represents magnitude 2. the arrow faces the direction of motion 3. a vector can be “picked up” and moved on the paper as long as the length and direction its pointing does not change

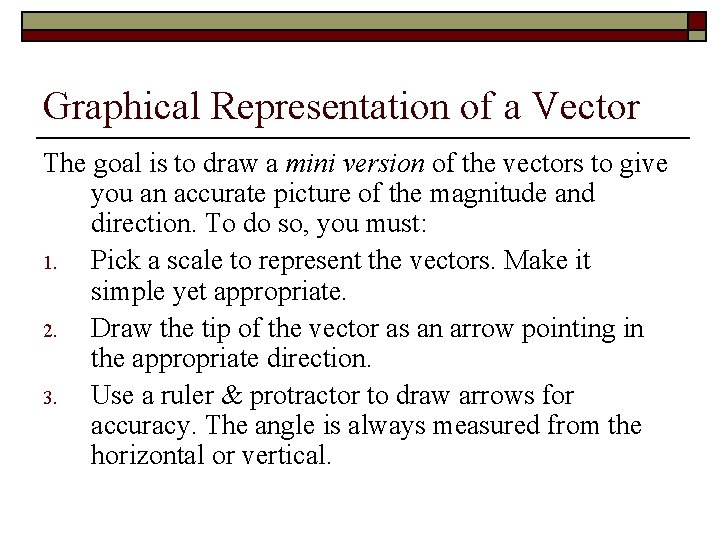
Graphical Representation of a Vector The goal is to draw a mini version of the vectors to give you an accurate picture of the magnitude and direction. To do so, you must: 1. Pick a scale to represent the vectors. Make it simple yet appropriate. 2. Draw the tip of the vector as an arrow pointing in the appropriate direction. 3. Use a ruler & protractor to draw arrows for accuracy. The angle is always measured from the horizontal or vertical.

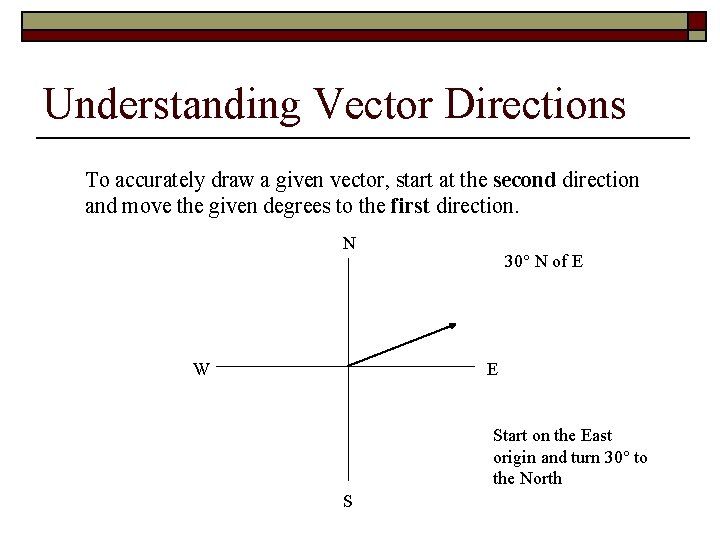
Understanding Vector Directions To accurately draw a given vector, start at the second direction and move the given degrees to the first direction. N W 30° N of E E Start on the East origin and turn 30° to the North S

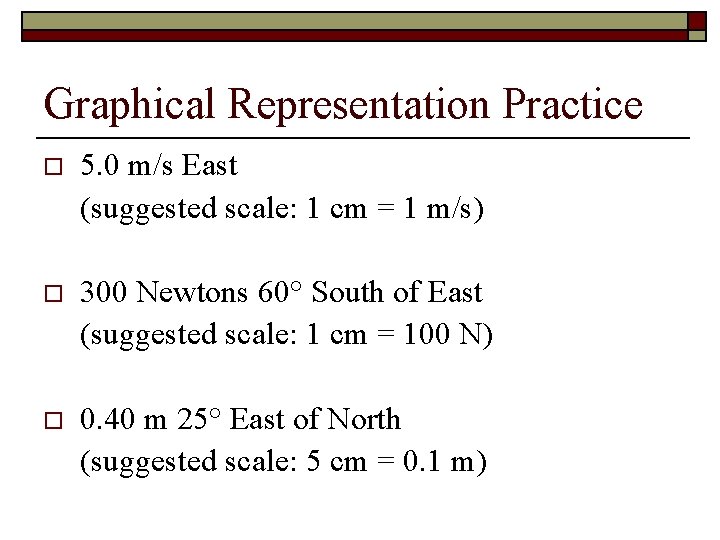
Graphical Representation Practice o 5. 0 m/s East (suggested scale: 1 cm = 1 m/s) o 300 Newtons 60° South of East (suggested scale: 1 cm = 100 N) o 0. 40 m 25° East of North (suggested scale: 5 cm = 0. 1 m)

Graphical Addition of Vectors 1. 2. 3. 4. Tip-To-Tail Method Pick appropriate scale, write it down. Use a ruler & protractor, draw 1 st vector to scale in appropriate direction, label. Start at tip of 1 st vector, draw 2 nd vector to scale, label. Connect the vectors starting at the tail end of the 1 st and ending with the tip of the last vector. This = sum of the original vectors, its called the resultant vector.

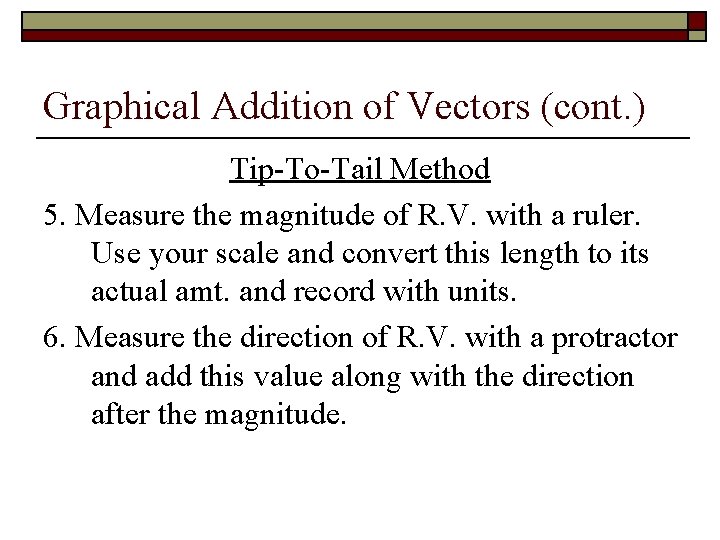
Graphical Addition of Vectors (cont. ) Tip-To-Tail Method 5. Measure the magnitude of R. V. with a ruler. Use your scale and convert this length to its actual amt. and record with units. 6. Measure the direction of R. V. with a protractor and add this value along with the direction after the magnitude.

Graphical Addition of Vectors (cont. ) 5 Km Scale: 1 Km = 1 cm 3 Km Resultant Vector (red) = 6 cm, therefore its 6 km.

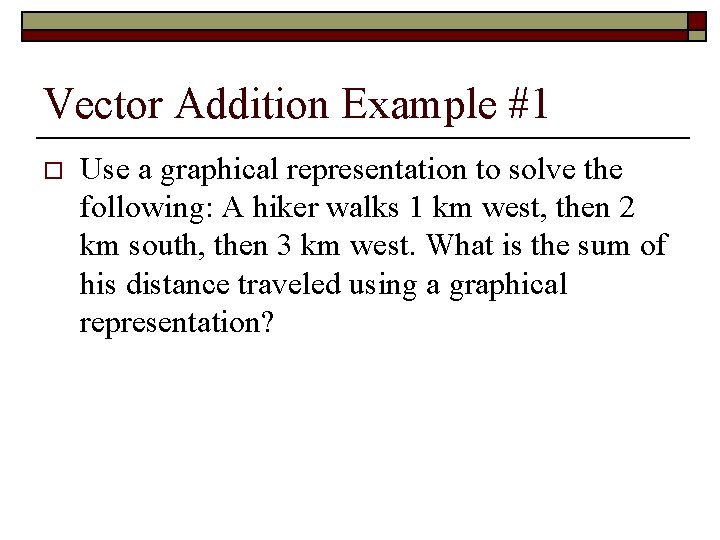
Vector Addition Example #1 o Use a graphical representation to solve the following: A hiker walks 1 km west, then 2 km south, then 3 km west. What is the sum of his distance traveled using a graphical representation?

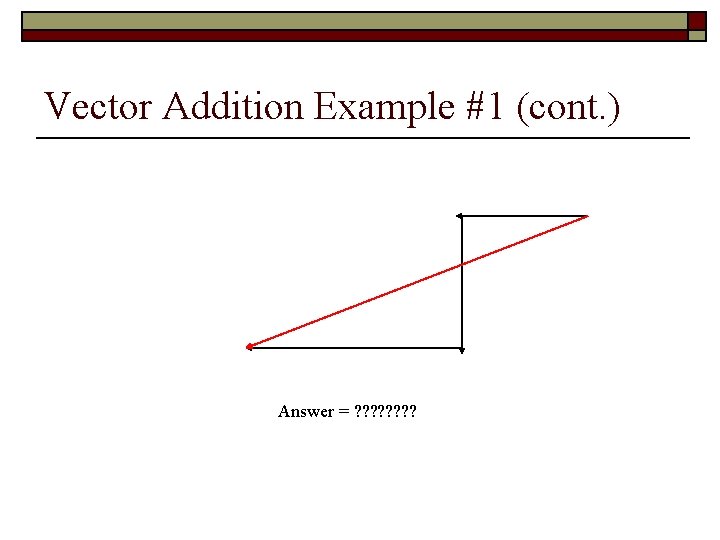
Vector Addition Example #1 (cont. ) Answer = ? ? ? ?

Vector Addition Example #2 o Use a graphical representation to solve the following: Another hiker walks 2 km south and 4 km west. What is the sum of her distance traveled using a graphical representation? How does it compare to hiker #1?

Vector Addition Example #2 (cont. ) Answer = ? ? ? ?

Mathematical Addition of Vectors o Vectors in the same direction: Add the 2 magnitudes, keep the direction the same. Ex. + = 3 m E 1 m E 4 m E

Mathematical Addition of Vectors o Vectors in opposite directions Subtract the 2 magnitudes, direction is the same as the greater vector. Ex. 4 m S + 2 m N = 2 m S

Mathematical Addition of Vectors o Vectors that meet at 90° Resultant vector will be hypotenuse of a right triangle. Use trig functions and Pythagorean Theorem.

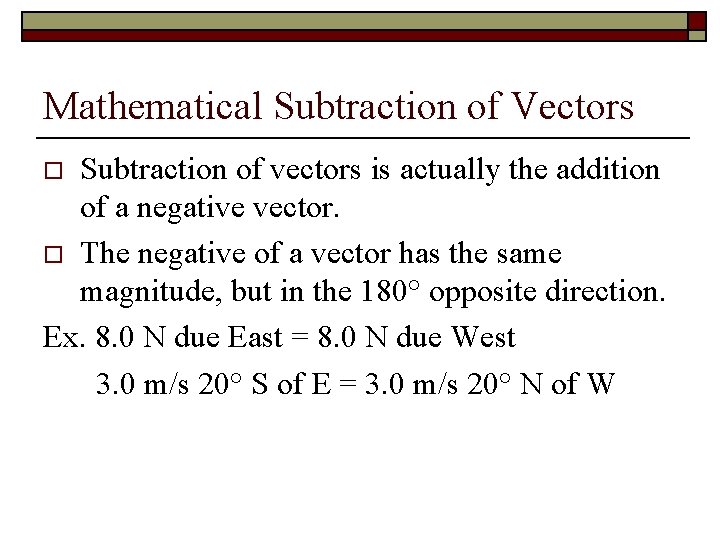
Mathematical Subtraction of Vectors Subtraction of vectors is actually the addition of a negative vector. o The negative of a vector has the same magnitude, but in the 180° opposite direction. Ex. 8. 0 N due East = 8. 0 N due West 3. 0 m/s 20° S of E = 3. 0 m/s 20° N of W o

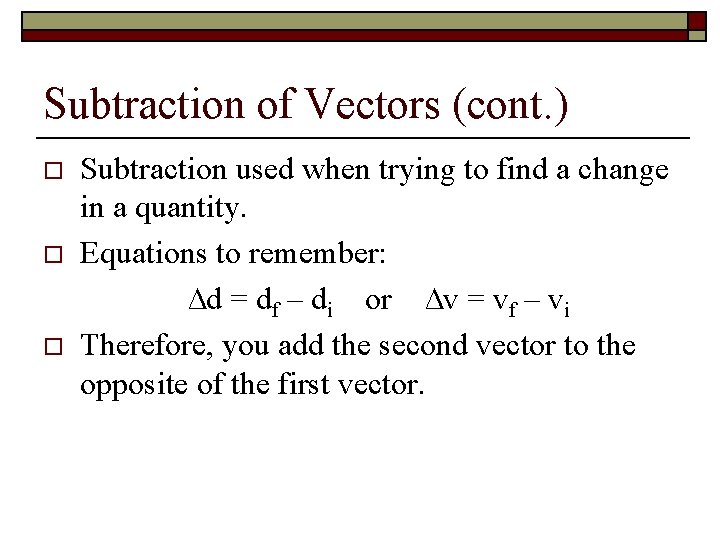
Subtraction of Vectors (cont. ) o o o Subtraction used when trying to find a change in a quantity. Equations to remember: ∆d = df – di or ∆v = vf – vi Therefore, you add the second vector to the opposite of the first vector.

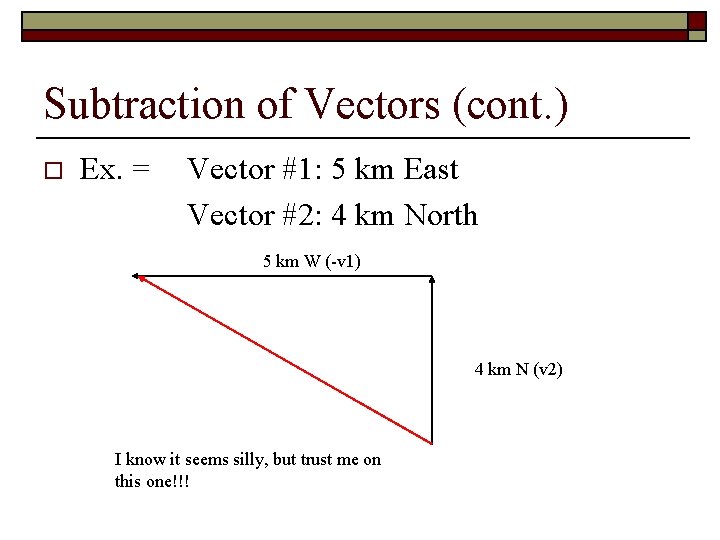
Subtraction of Vectors (cont. ) o Ex. = Vector #1: 5 km East Vector #2: 4 km North 5 km W (-v 1) 4 km N (v 2) I know it seems silly, but trust me on this one!!!

Component Method of Vector Addition o Treat each vector separately: 1. To find the “X” component, you must: Ax = Acos Θ 2. To find the “Y” component, you must: Ay = Asin Θ 3. Repeat steps 2 & 3 for all vectors

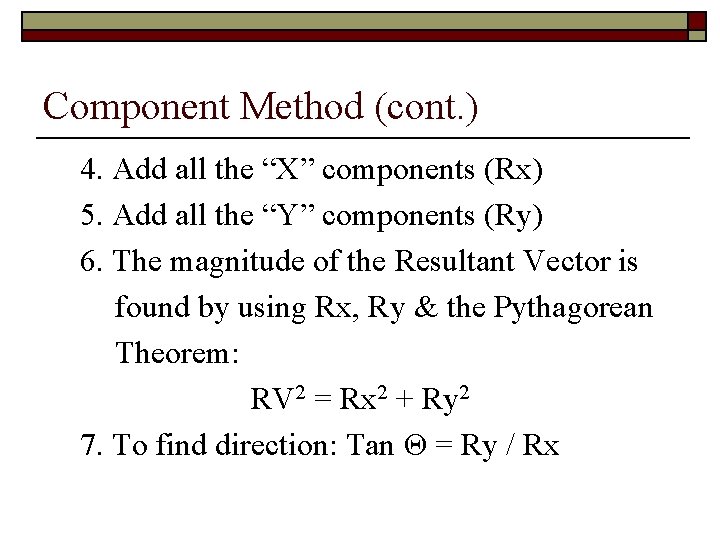
Component Method (cont. ) 4. Add all the “X” components (Rx) 5. Add all the “Y” components (Ry) 6. The magnitude of the Resultant Vector is found by using Rx, Ry & the Pythagorean Theorem: RV 2 = Rx 2 + Ry 2 7. To find direction: Tan Θ = Ry / Rx

Component Method (cont. ) Ex. #1 V 1 = 2 m/s 30° N of E V 2 = 3 m/s 40° N of W (this is easy!) Find: Magnitude & Direction Magnitude = 2. 96 m/s Direction = 78° N of W

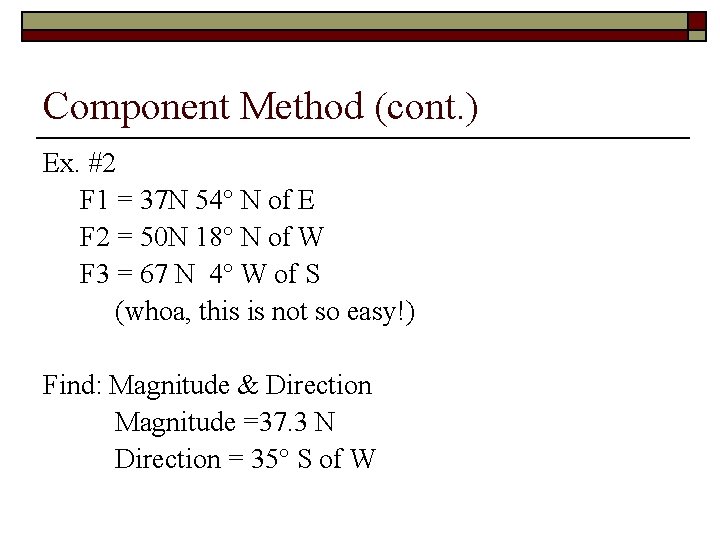
Component Method (cont. ) Ex. #2 F 1 = 37 N 54° N of E F 2 = 50 N 18° N of W F 3 = 67 N 4° W of S (whoa, this is not so easy!) Find: Magnitude & Direction Magnitude =37. 3 N Direction = 35° S of W