UNIT 1 Introduction to Science LETS REVIEW LAB

UNIT 1 Introduction to Science

LET’S REVIEW LAB SAFETY Safety Rap Video Safety Video

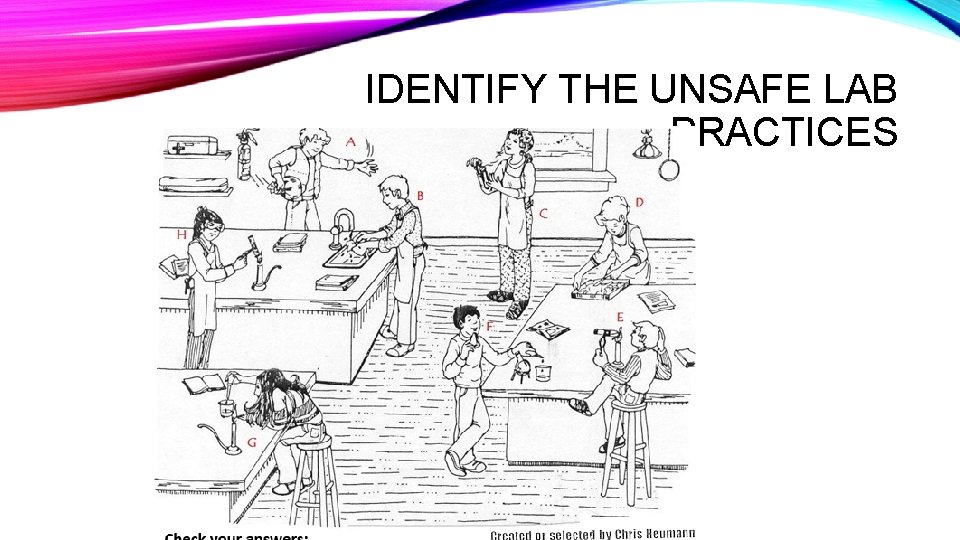
IDENTIFY THE UNSAFE LAB PRACTICES

SCIENTIFIC METHOD

STEPS OF THE SCIENTIFIC METHOD • Bozman Explains • Variables • Dependent • Independent

MYTHBUSTERS • http: //www. discovery. com/tv-shows/mythbusters/videos/poo-power/ • http: //www. discovery. com/tv-shows/mythbusters/videos/running-in-the-rainminimyth/ • http: //www. discovery. com/tv-shows/mythbusters/videos/busters-drop-to-doom/

SHOWING DATA USING GRAPHS

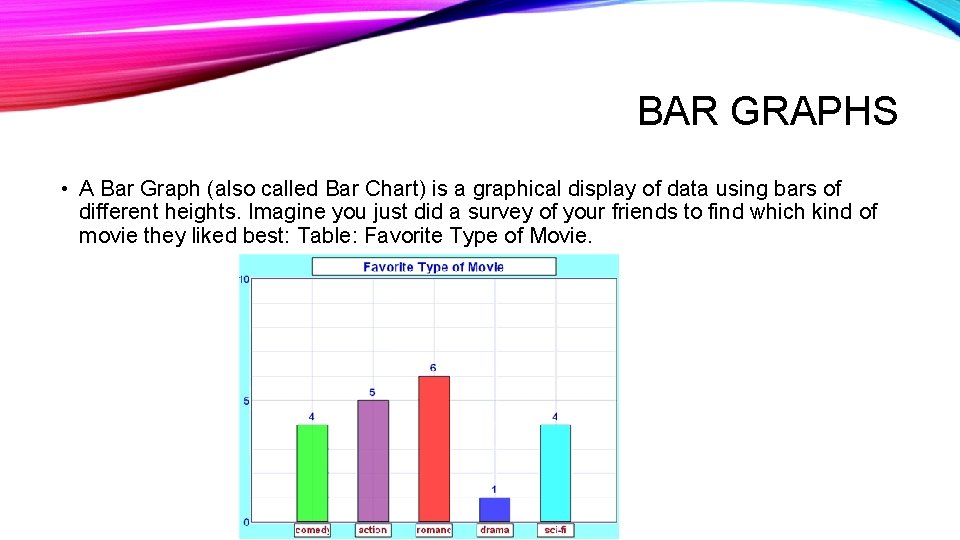
BAR GRAPHS • A Bar Graph (also called Bar Chart) is a graphical display of data using bars of different heights. Imagine you just did a survey of your friends to find which kind of movie they liked best: Table: Favorite Type of Movie.

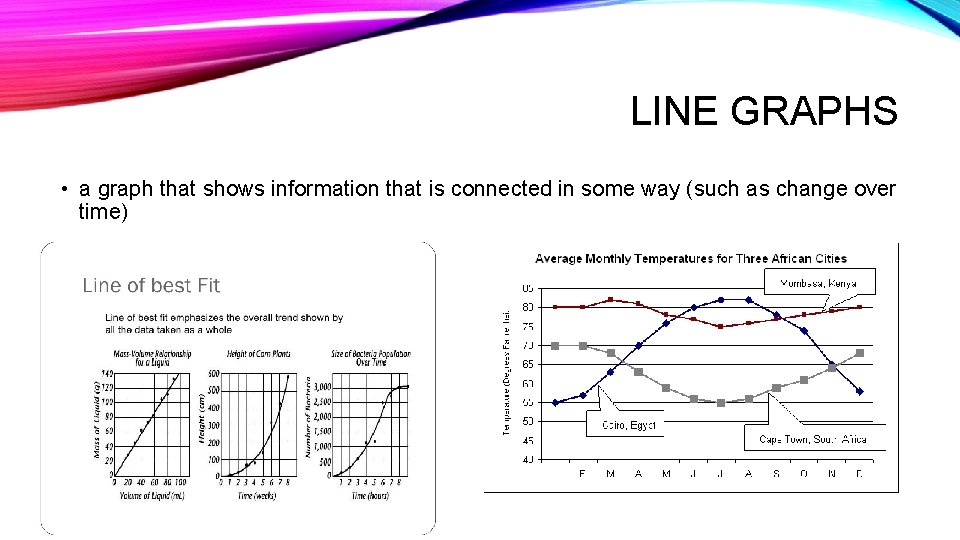
LINE GRAPHS • a graph that shows information that is connected in some way (such as change over time)

LAB EQUIPMENT

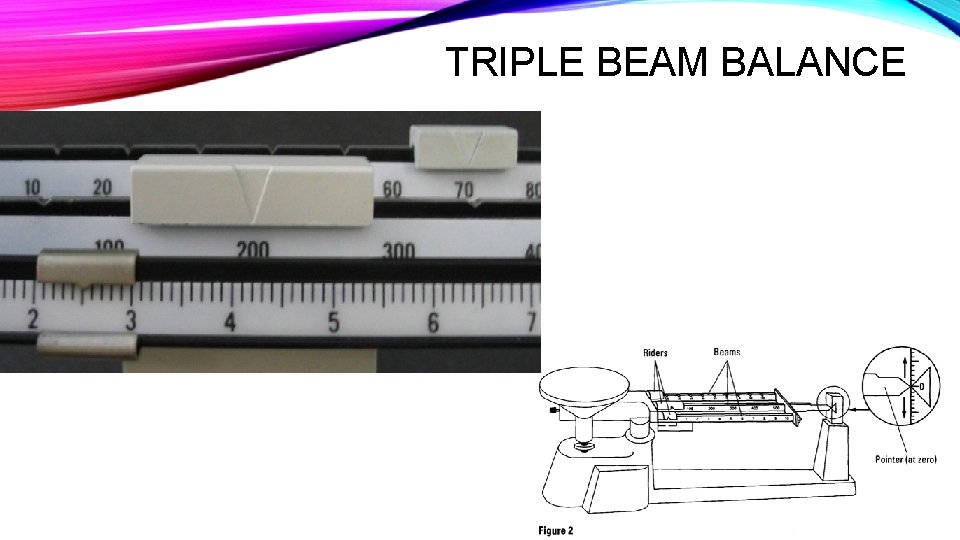
TRIPLE BEAM BALANCE

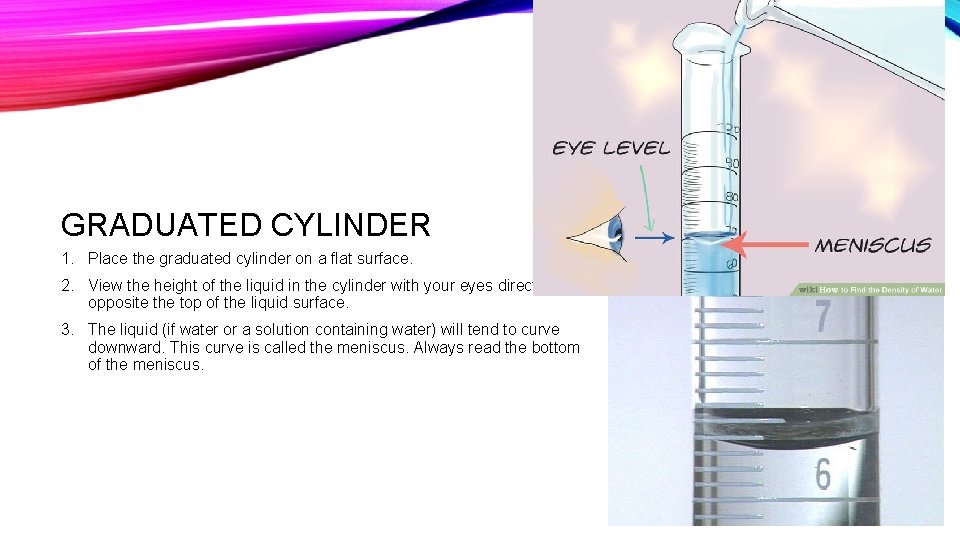
GRADUATED CYLINDER 1. Place the graduated cylinder on a flat surface. 2. View the height of the liquid in the cylinder with your eyes directly opposite the top of the liquid surface. 3. The liquid (if water or a solution containing water) will tend to curve downward. This curve is called the meniscus. Always read the bottom of the meniscus.

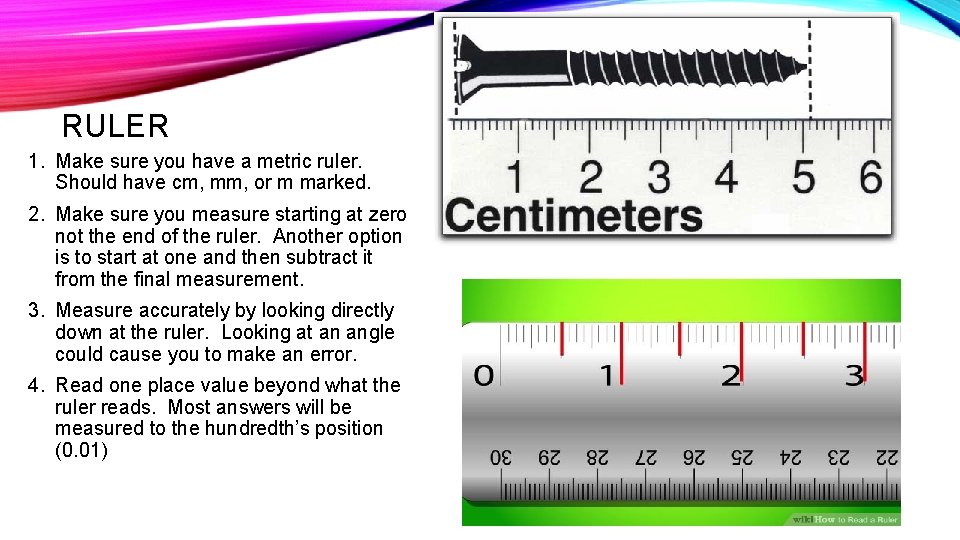
RULER 1. Make sure you have a metric ruler. Should have cm, mm, or m marked. 2. Make sure you measure starting at zero not the end of the ruler. Another option is to start at one and then subtract it from the final measurement. 3. Measure accurately by looking directly down at the ruler. Looking at an angle could cause you to make an error. 4. Read one place value beyond what the ruler reads. Most answers will be measured to the hundredth’s position (0. 01)

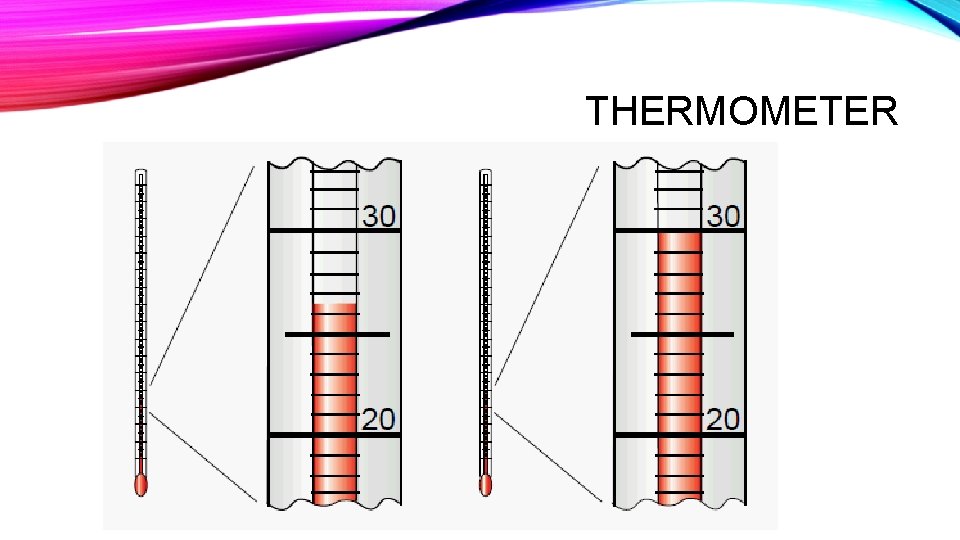
THERMOMETER

MEASUREMENT

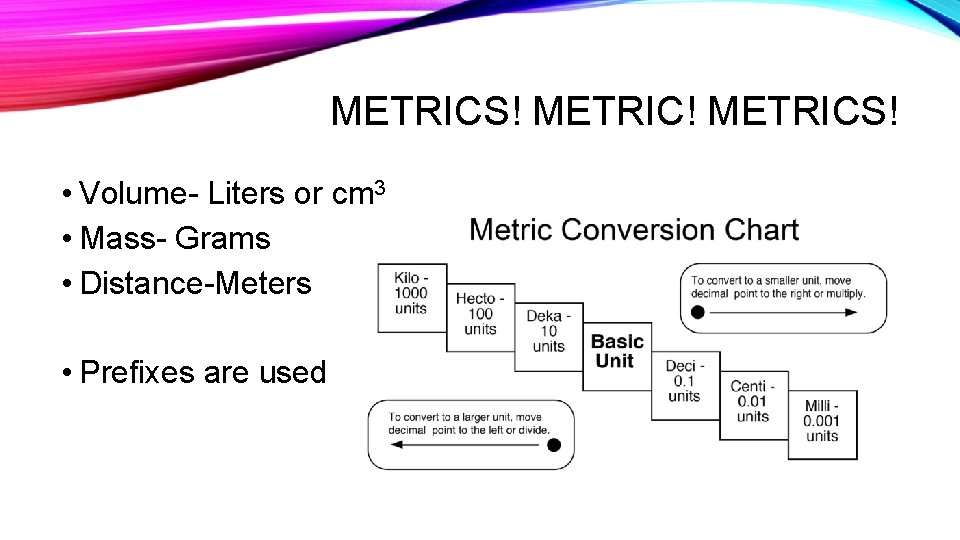
METRICS! • Volume- Liters or cm 3 • Mass- Grams • Distance-Meters • Prefixes are used

DIMENSIONAL ANALYSIS Converting Units

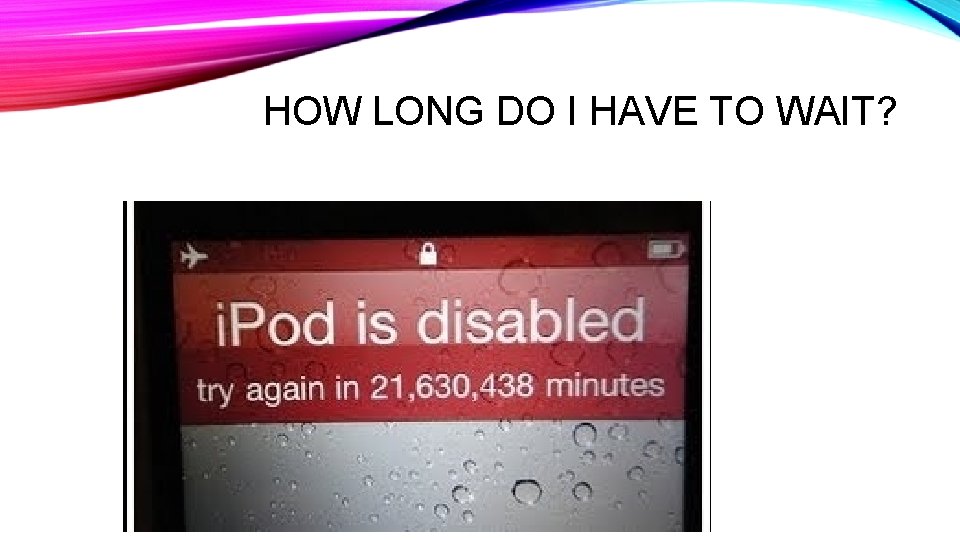
HOW LONG DO I HAVE TO WAIT?

LET’S REVIEW THE METRIC SYSTEM In General: Example: • Kilo = 1000 of base • Kilometer = 1000 m • Deci = 0. 1 of base • Meter = base unit • Centi = 0. 01 of base • Decimeter = 0. 1 m • Milli = 0. 001 of base • Centimeter = 0. 01 m • Millimeter = 0. 001 m

CONVERTING UNITS OF MEASUREMENT • You convert units of measurement in your everyday life. • How would you convert from minutes to seconds? • 1 minute = 60 seconds • Doing unit conversions in chemistry is no different, we just call it dimensional analysis. • Hint: Unit conversions are exact numbers, so the only sig figs you count are the ones in your original number • In chemistry, we use conversion factors, a ratio used to convert from unit to another. • I will give you non-metric conversion factors. Ex grams to pounds • As a conversion factor, minutes to seconds would be written like this: 60 seconds 1 minute

STEPS FOR CONVERTING UNITS 1. Identify the unit you are starting in, and identify the unit you need to convert to. 2. Choose the appropriate conversion factor(s) to get from your starting unit to your ending unit. 3. Set-up your problem, so your units are easily compared and canceled 4. Multiply the top, multiply the bottom, divide the top by the bottom

MINUTES TO SECONDS How many seconds are in 5 minutes? 1. Starting units: minutes, ending units: seconds 2. Conversion factor: 60 seconds/ 1 minute 5 minute 60 seconds 3. Set-up: 1 minute 4. Multiply top: 5 x 60 = 300, Multiply bottom: 1, Divide top by bottom: 300/1 = 300 seconds, this answer already has the correct number of sig figs

METRIC CONVERSIONS How many meters are in 300 centimeters? 1. Starting units: centimeters, Ending units: meters 2. Conversion factor: 0. 01 m = 1 cm, or 100 cm = 1 m 3. Set-up: 300 centimeter 100 centimeter 4. Multiply top: 300 x 1 = 300, Multiply bottom: 100, Divide top by bottom: 300/100= 3 meters, this answer already has the correct number of sig figs

2 CONVERSION PROBLEMS • For some dimensional analysis problems you will have to use 2 conversion factors to get to an answer. But you still use the same steps! Let’s look at an example: • How many seconds are in 4 hours? 1. Starting units: hours, Ending units: seconds 2. Conversion factors: 60 minutes = 1 hour, 60 seconds = 1 minute 3. Set-up: 4. 0 hours 60 minutes 60 seconds 1 hour 1 minute 4. Multiply the top: 4 x 60 = 14400, Multiply the bottom: 1 x 1 = 1, Divide top by bottom: 14400/1 = 14400 seconds, sig fig answer 14000 seconds

2 CONVERSION PROBLEMS How many yards are in 250 centimeters? Hint: 1 m = 1. 094 yds 1. Starting units: centimeters, Ending units: yards 2. Conversion Factors: 100 cm = 1 m, 1 m = 1. 094 yds 3. Set-up: 250. 0 cm 1 m 1. 094 yds 100 cm 1 m 4. Multiply the top: 250 x 1. 094 = 273. 5, Multiply the bottom: 100 x 1 = 100, Divide the top by the bottom: 273. 5/100 = 2. 735 yards, this answer already has the correct number of sig figs

PRACTICE TIME! • Try practicing dimensional analysis on your own on the worksheet. This worksheet will be a part of your homework packet.

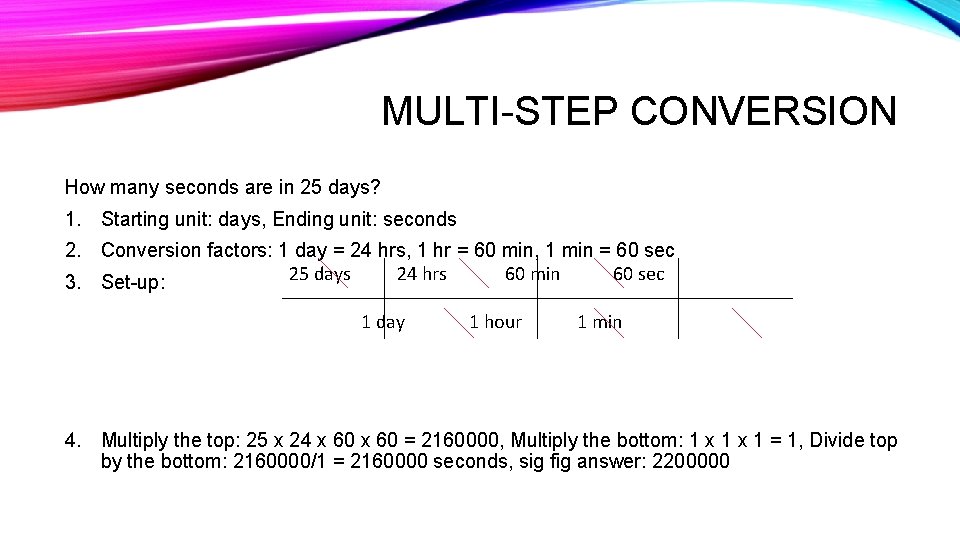
MULTI-STEP CONVERSION How many seconds are in 25 days? 1. Starting unit: days, Ending unit: seconds 2. Conversion factors: 1 day = 24 hrs, 1 hr = 60 min, 1 min = 60 sec 25 days 24 hrs 60 min 60 sec 3. Set-up: 1 day 1 hour 1 min 4. Multiply the top: 25 x 24 x 60 = 2160000, Multiply the bottom: 1 x 1 = 1, Divide top by the bottom: 2160000/1 = 2160000 seconds, sig fig answer: 2200000

WHAT ABOUT MEASUREMENTS WITH 2 UNITS? • Some measurements, like density (g/m. L), have two units. You can still use dimensional analysis to convert one or both of the units present. • You will still use the same steps, but your set-up will be longer and you have to be more careful about canceling your units. • For your conversion factors, I recommend separating them into the units you need to use for the top unit and the bottom unit • Let’s look at a few examples

2 UNIT CONVERSIONS Race cars routinely travel at an average speed of 225 mi/hr. What is the speed in km/hr? Hint: 1 mi = 1760 yd, 1 m = 1. 094 yd 1. Starting units: mi/hr, Ending units: km/hr, Note: hours is the same in both 2. Conversion factors: 1 mi = 1760 yd, 1 m = 1. 094 yd, 1000 m = 1 km 3. Set-up: 225 mi 1760 yd 1 m 1 km hr 1 mi 1. 094 yd 1000 m 4. Multiply top: 26. 2 mi x 1760 yd x 1 m x 1 km = 46112, Multiply bottom: 1 mi x 1. 094 yd x 1000 m = 1094, Divide top by bottom: 46112/1094 = 42. 1 km/hr (correct sig figs)

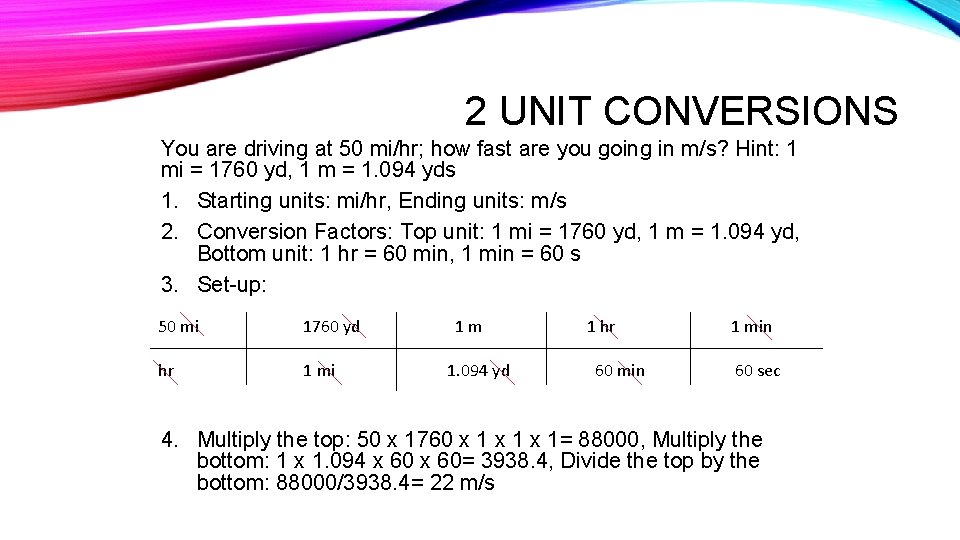
2 UNIT CONVERSIONS You are driving at 50 mi/hr; how fast are you going in m/s? Hint: 1 mi = 1760 yd, 1 m = 1. 094 yds 1. Starting units: mi/hr, Ending units: m/s 2. Conversion Factors: Top unit: 1 mi = 1760 yd, 1 m = 1. 094 yd, Bottom unit: 1 hr = 60 min, 1 min = 60 s 3. Set-up: 50 mi 1760 yd hr 1 mi 1 m 1. 094 yd 1 hr 60 min 1 min 60 sec 4. Multiply the top: 50 x 1760 x 1 x 1= 88000, Multiply the bottom: 1 x 1. 094 x 60= 3938. 4, Divide the top by the bottom: 88000/3938. 4= 22 m/s

LET’S PRACTICE • This worksheet is great practice for the skills we learned today and yesterday. • Use this worksheet to gauge your understanding of dimensional analysis • This worksheet will be a part of your homework packet due on the day of the test.