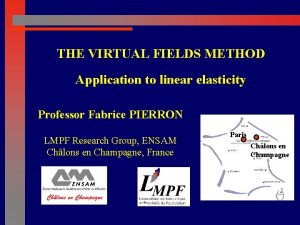
THE VIRTUAL FIELDS METHOD Introduction and Overview Professor









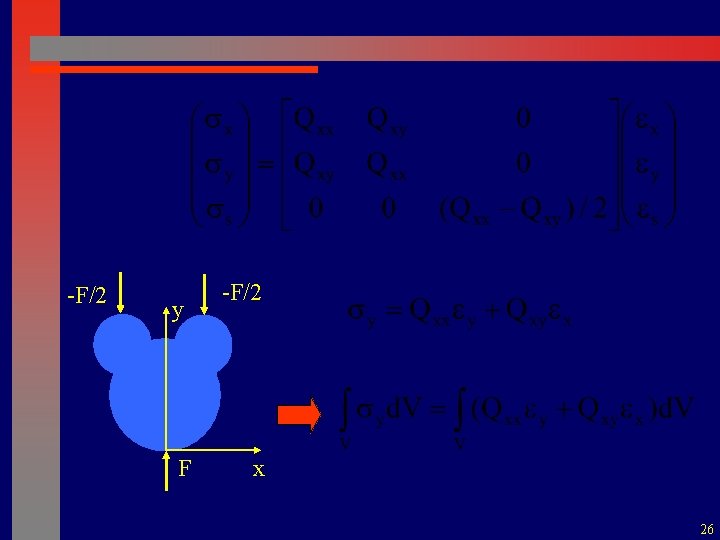







- Slides: 62

THE VIRTUAL FIELDS METHOD Introduction and Overview Professor Fabrice PIERRON LMPF Research Group, ENSAM Châlons en Champagne, France Paris Châlons en Champagne

A bit of history

A bit of history 1989 – First paper in “Comptes rendus de l’académie des sciences” (principle) 1990 – Ph. D thesis of Michel GRÉDIAC (thin anisotropic plates, including experiments) 1994 – First collaboration between FP & MG (anisotropic in-plane properties, shear) 1996 – 98: First application in dynamics (vibration of thin plates, exp. & num. ) 1998 – 2000: Series of work on in-plane elastic stiffnesses of composites (exp. & num. ) 2001: first attempt at a non-linear law (anisotropic) 2002 – 04: Significant progress on virtual fields selection in elasticity (special virtual fields, minimization of noise effects) 3

A bit of history 2003: First application in vibration with damping (thin plates) 2005 – 06: Convincing experimental results (in-plane anisotropic composite stiffnesses) 2006 – First application to elasto-plasticity 2006: Theoretical framework in elasticity (relation between FEMU and VFM) 2006: Optimisation of test configuration (with Airbus UK) 2006: First application on heterogeneous materials – stiffness contrast in impacted composite plates (with Bristol Univ. ) 4

A bit of history 2007: Application to elastography (MRI) 2007: First application to viscoplasticity (coll. M. A. Sutton) 2007: First application to heterogeneous plasticity (FSW joints) Ongoing Application to 3 D bulk measurements (composites, biomechanics), project with Loughborough university (Prof. J. M. Huntley, Dr P. D. Ruiz) Optimisation of virtual fields in plasticity Friction Stir Welds (collaboration with ONERA, France) Development of a user-oriented software: CAMFIT 5

The principle of virtual work

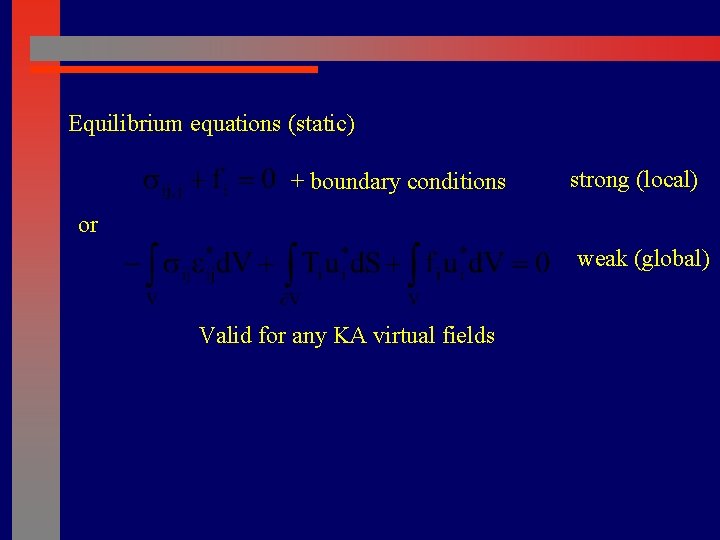
Equilibrium equations (static) + boundary conditions strong (local) or weak (global) Valid for any KA virtual fields

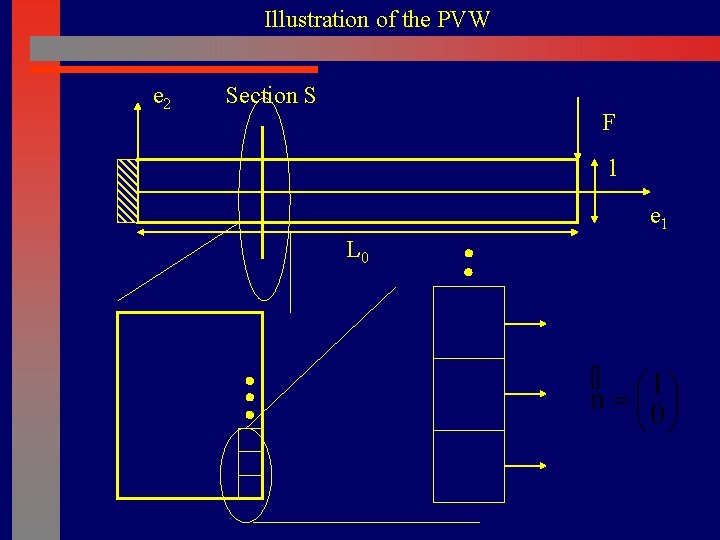
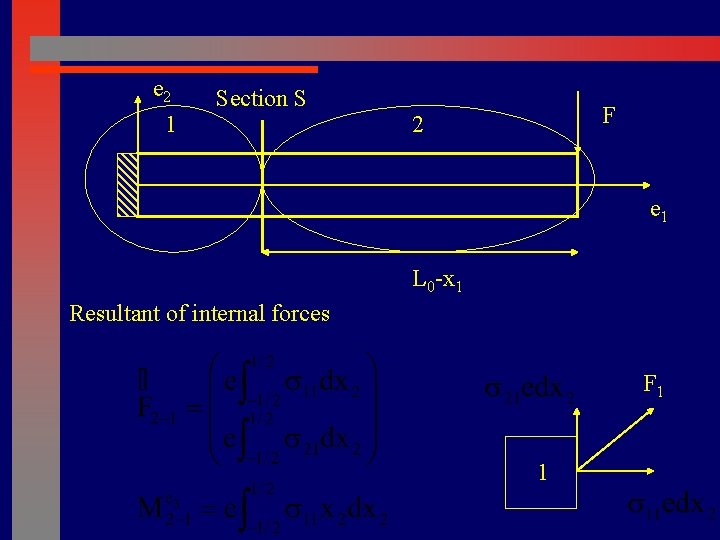
Illustration of the PVW e 2 Section S F l e 1 L 0

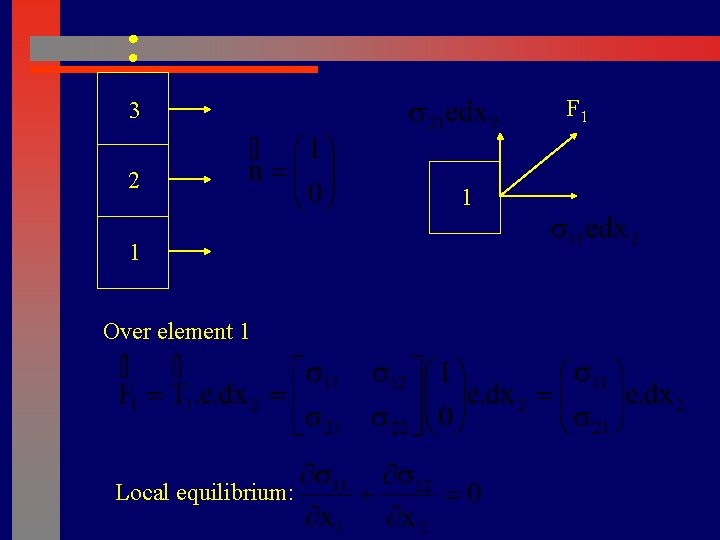
F 1 3 2 1 Over element 1 Local equilibrium: 1

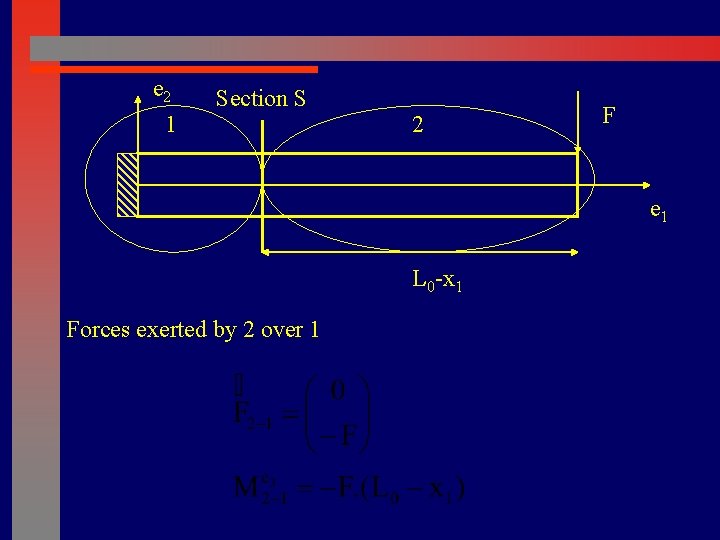
e 2 1 Section S 2 F e 1 L 0 -x 1 Forces exerted by 2 over 1

e 2 1 Section S F 2 e 1 L 0 -x 1 Resultant of internal forces F 1 1

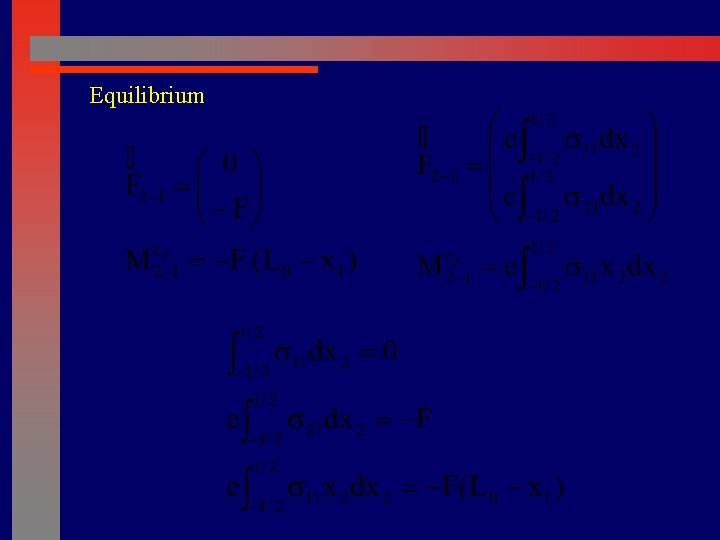
Equilibrium

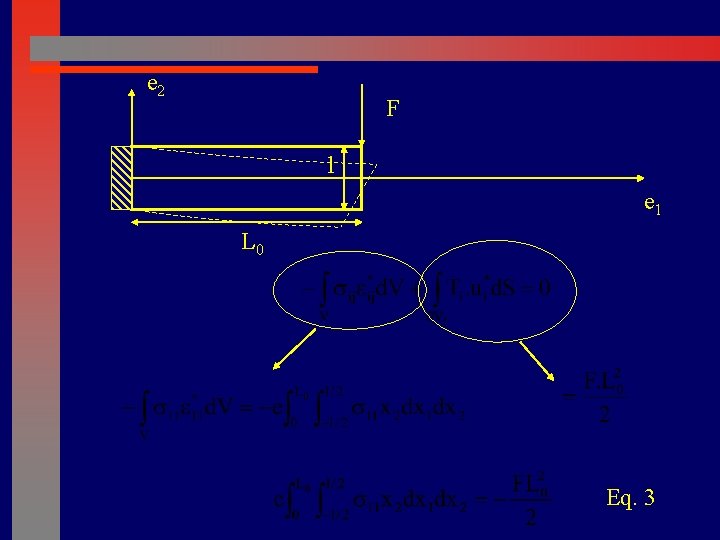
Valid over any section S of the beam: integration over x 1 Eq. 2 Eq. 3

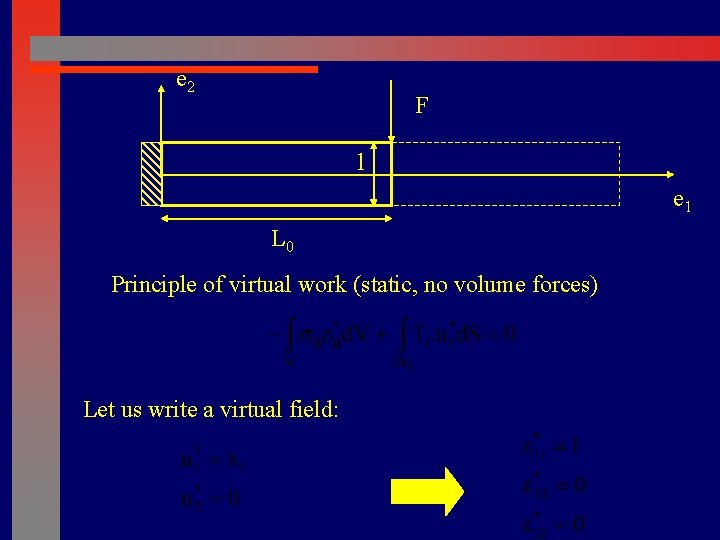
e 2 F l e 1 L 0 Principle of virtual work (static, no volume forces) Let us write a virtual field:

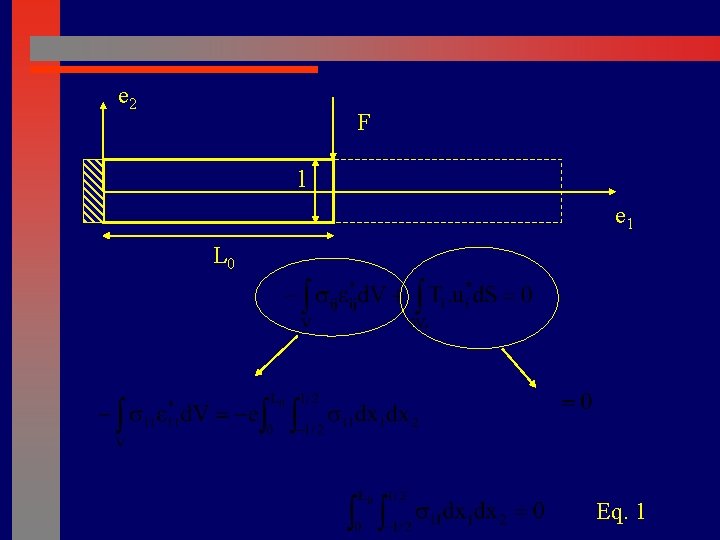
e 2 F l e 1 L 0 Eq. 1

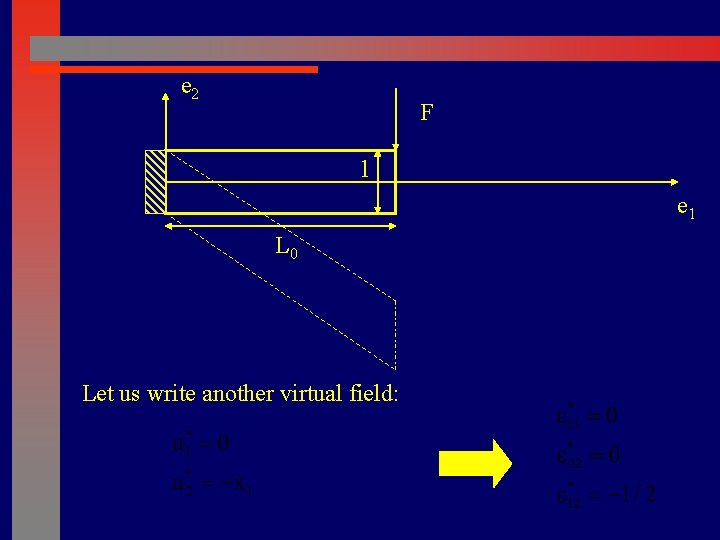
e 2 F l e 1 L 0 Let us write another virtual field:

e 2 F l e 1 L 0 Eq. 2

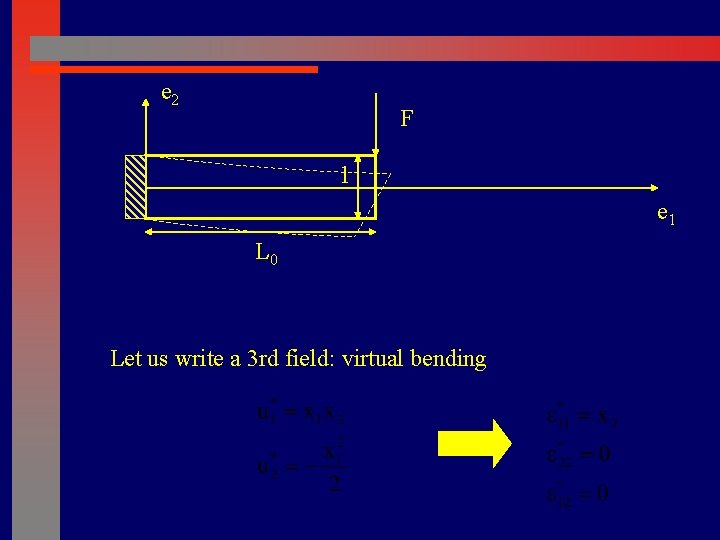
e 2 F l e 1 L 0 Let us write a 3 rd field: virtual bending

e 2 F l e 1 L 0 Eq. 3

The Virtual Fields Method

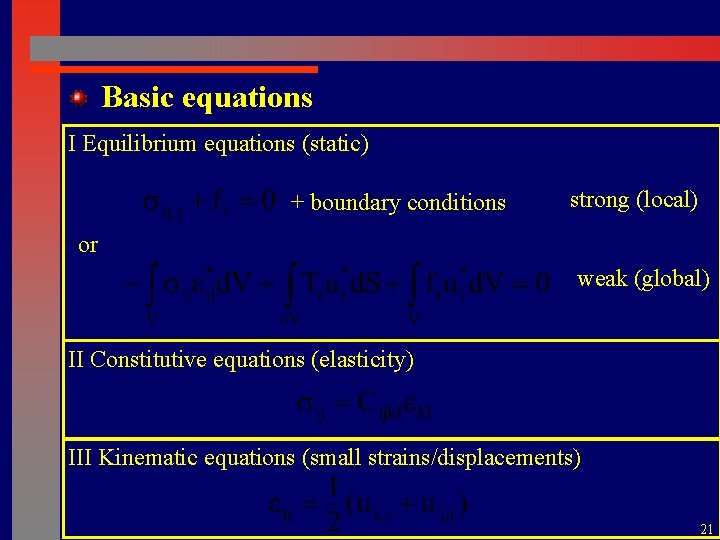
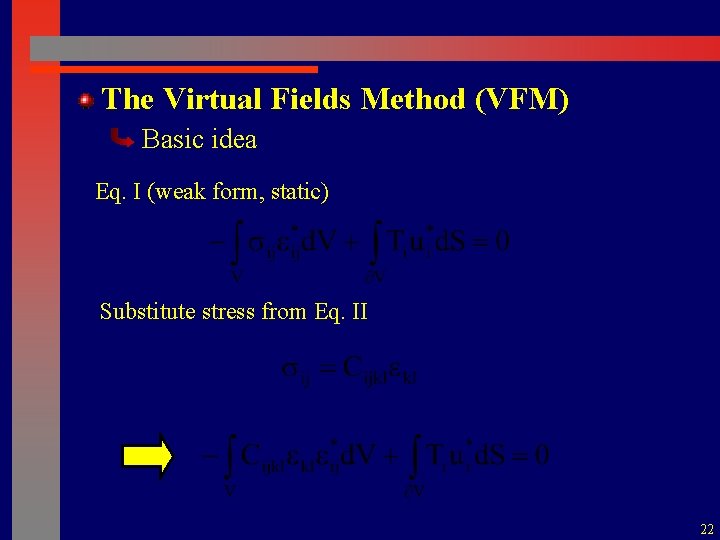
Basic equations I Equilibrium equations (static) + boundary conditions strong (local) or weak (global) II Constitutive equations (elasticity) III Kinematic equations (small strains/displacements) 21

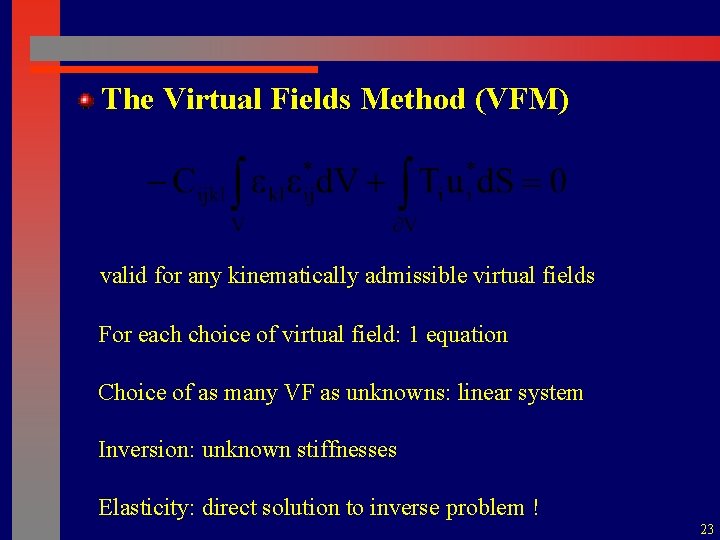
The Virtual Fields Method (VFM) Basic idea Eq. I (weak form, static) Substitute stress from Eq. II 22

The Virtual Fields Method (VFM) valid for any kinematically admissible virtual fields For each choice of virtual field: 1 equation Choice of as many VF as unknowns: linear system Inversion: unknown stiffnesses Elasticity: direct solution to inverse problem ! 23

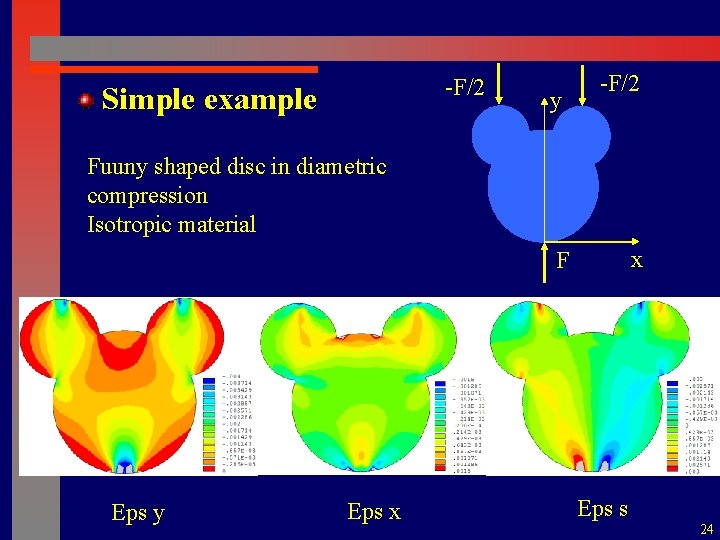
-F/2 Simple example y -F/2 Fuuny shaped disc in diametric compression Isotropic material x F Eps y Eps x Eps s 24

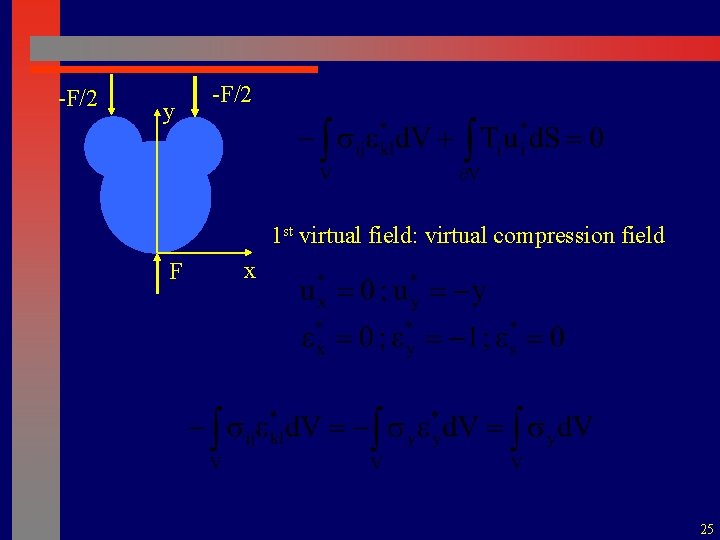
-F/2 y -F/2 1 st virtual field: virtual compression field F x 25
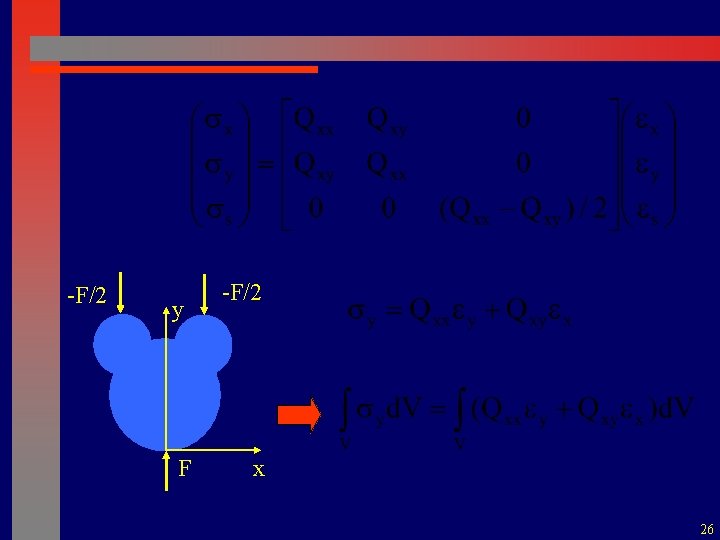
-F/2 y F -F/2 x 26

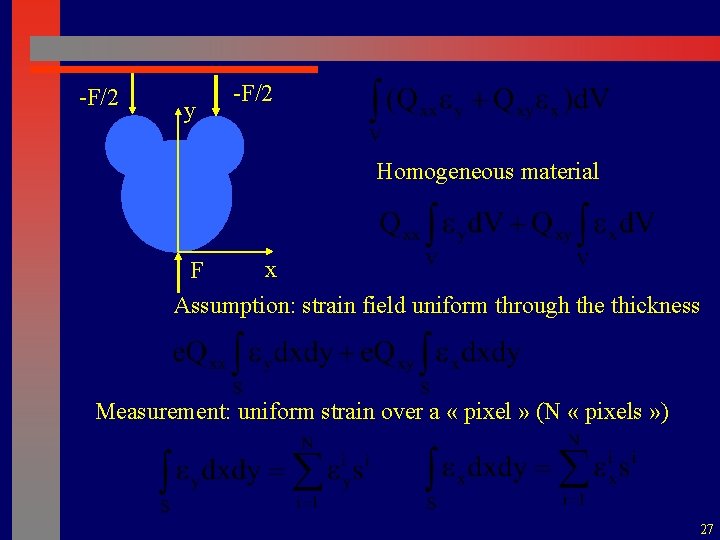
-F/2 y -F/2 Homogeneous material x F Assumption: strain field uniform through the thickness Measurement: uniform strain over a « pixel » (N « pixels » ) 27

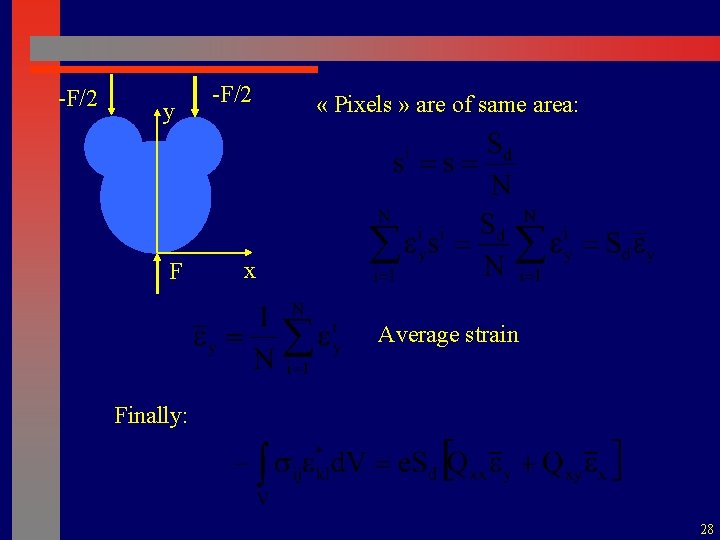
-F/2 y F -F/2 « Pixels » are of same area: x Average strain Finally: 28

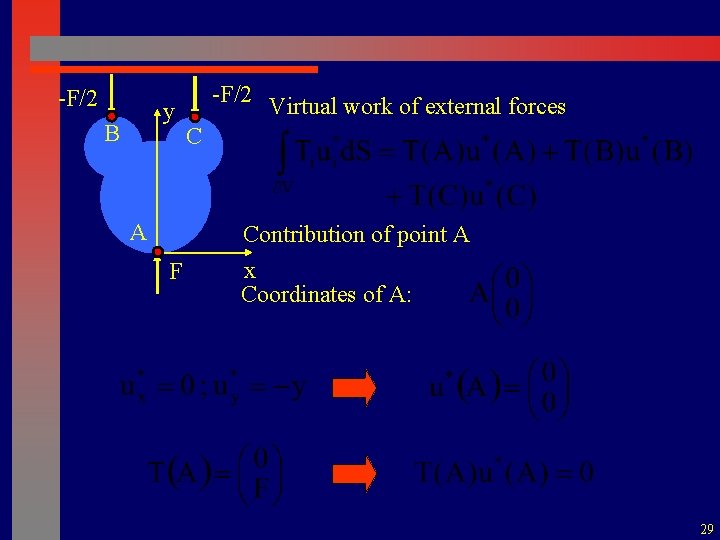
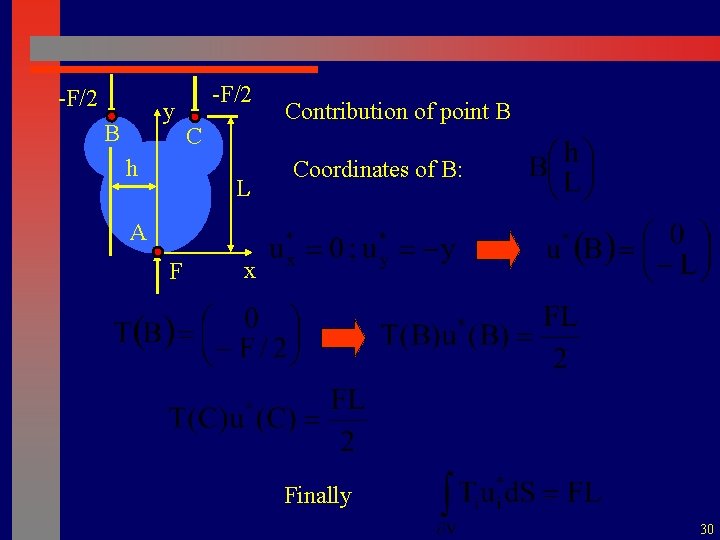
-F/2 y B A -F/2 Virtual work of external forces C Contribution of point A F x Coordinates of A: 29

-F/2 y B h -F/2 C L Contribution of point B Coordinates of B: A F x Finally 30

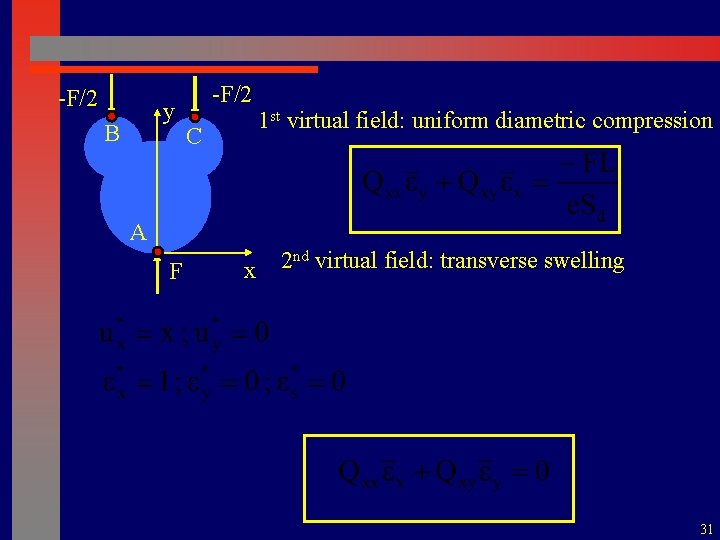
-F/2 y B -F/2 C 1 st virtual field: uniform diametric compression A F x 2 nd virtual field: transverse swelling 31

-F/2 y F -F/2 Finally x Direct solution To inverse problem !!! 32

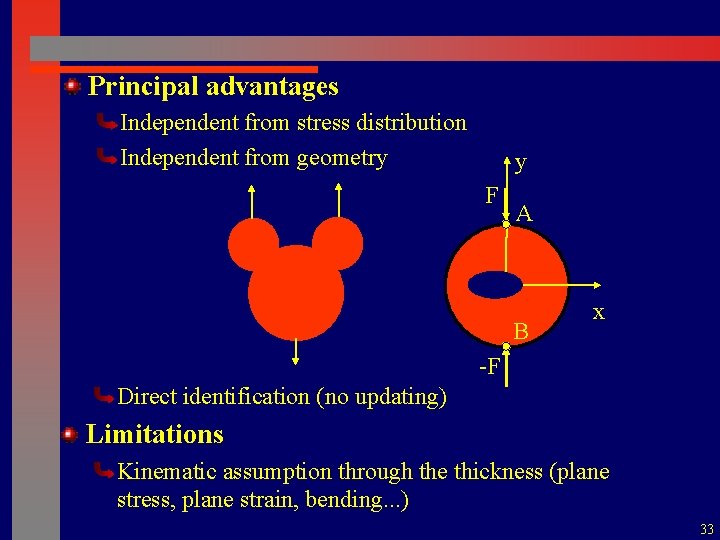
Principal advantages Independent from stress distribution Independent from geometry y F A B x -F Direct identification (no updating) Limitations Kinematic assumption through the thickness (plane stress, plane strain, bending. . . ) 33

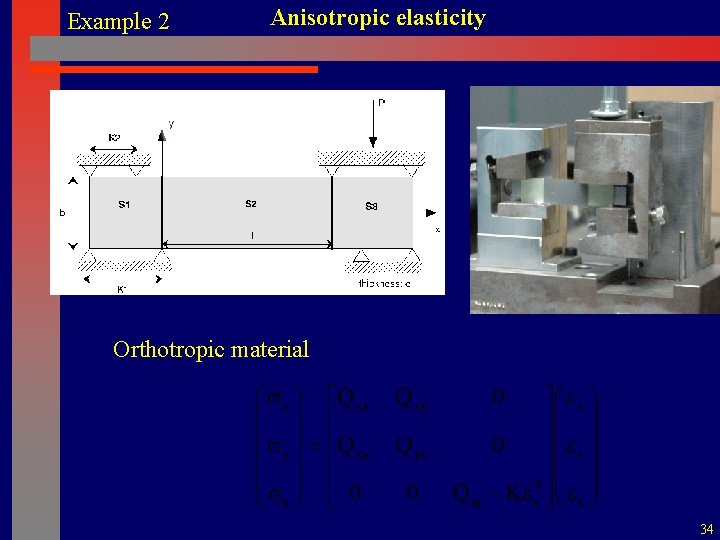
Example 2 Anisotropic elasticity Orthotropic material 34

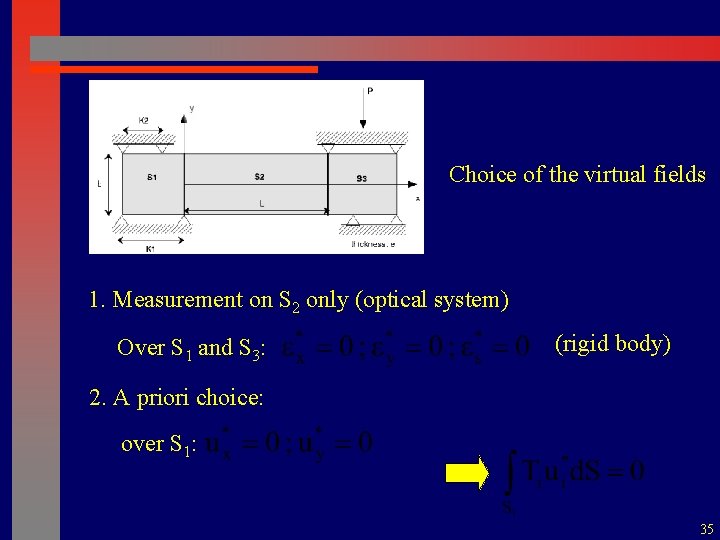
Choice of the virtual fields 1. Measurement on S 2 only (optical system) Over S 1 and S 3: (rigid body) 2. A priori choice: over S 1: 35

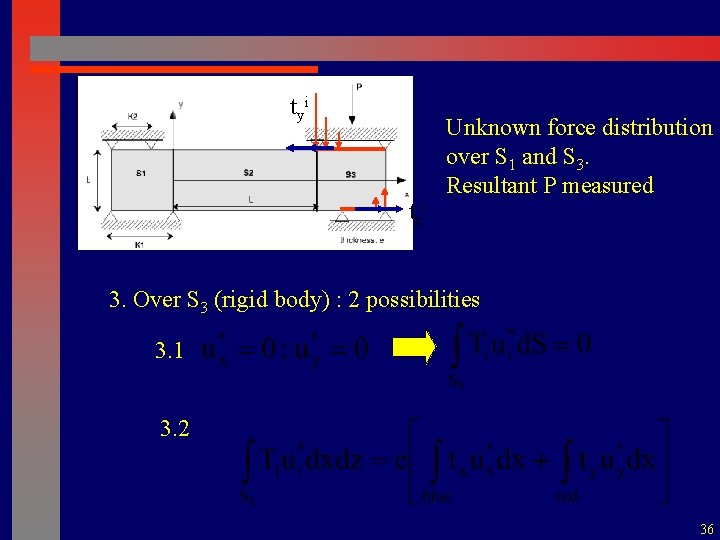
tyi txi Unknown force distribution over S 1 and S 3. Resultant P measured 3. Over S 3 (rigid body) : 2 possibilities 3. 1 3. 2 36

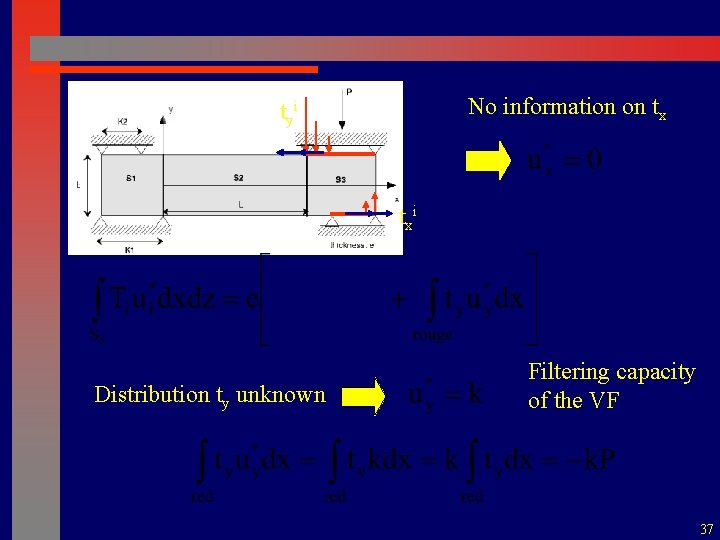
No information on tx tyi txi Distribution ty unknown Filtering capacity of the VF 37

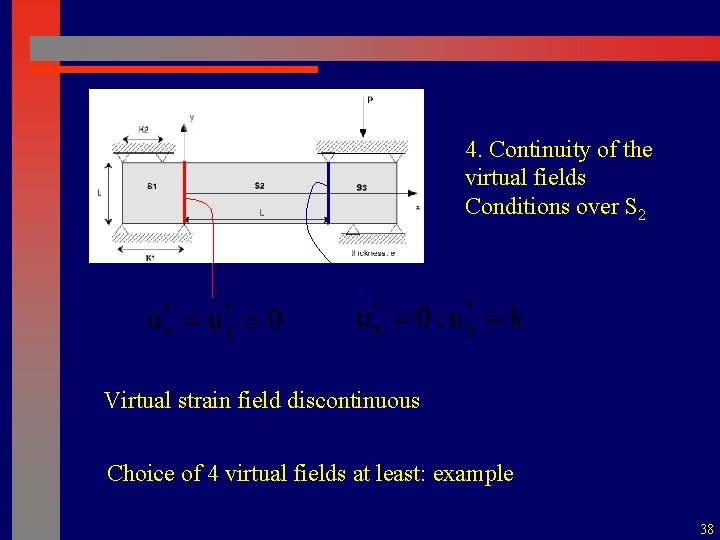
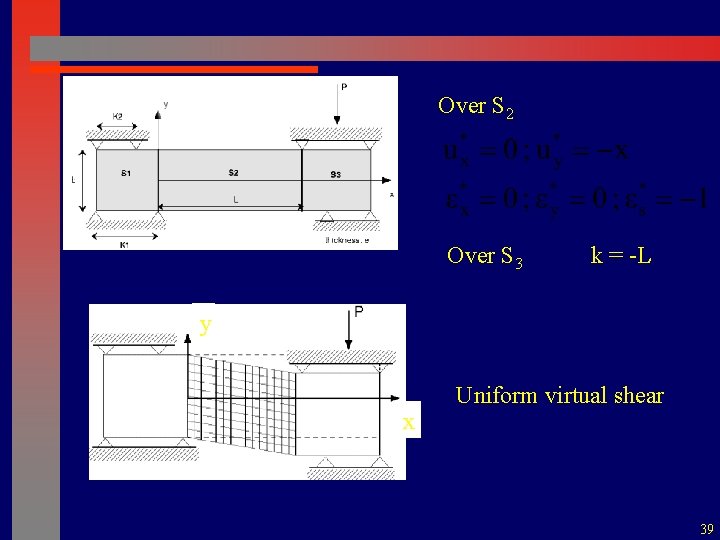
4. Continuity of the virtual fields Conditions over S 2 Virtual strain field discontinuous Choice of 4 virtual fields at least: example 38

Over S 2 Over S 3 k = -L y x Uniform virtual shear 39

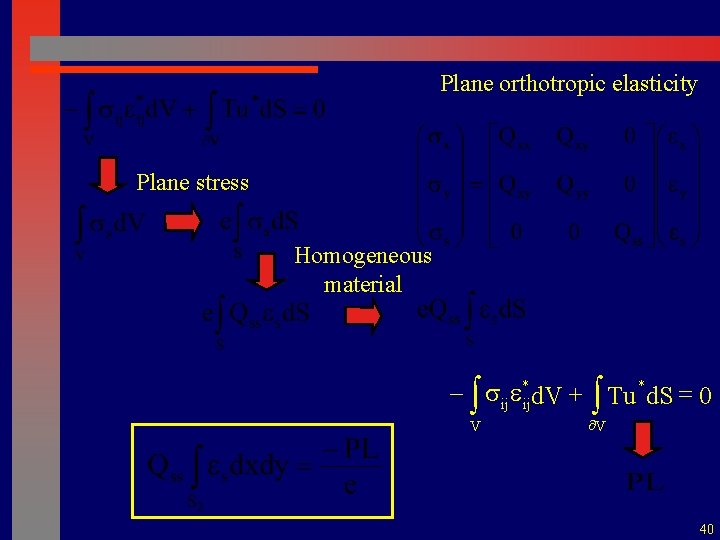
Plane orthotropic elasticity Plane stress Homogeneous material - ò s ije*ijd. V + ò Tu *d. S = 0 V ¶V 40

Field n° 2: Bernoulli bending Sur S 2 y x Sur S 3 k = -L 3 41

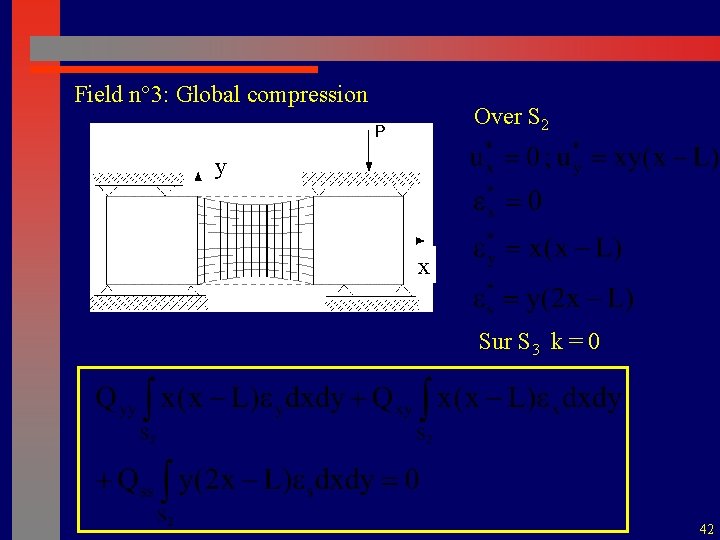
Field n° 3: Global compression Over S 2 y x Sur S 3 k = 0 42

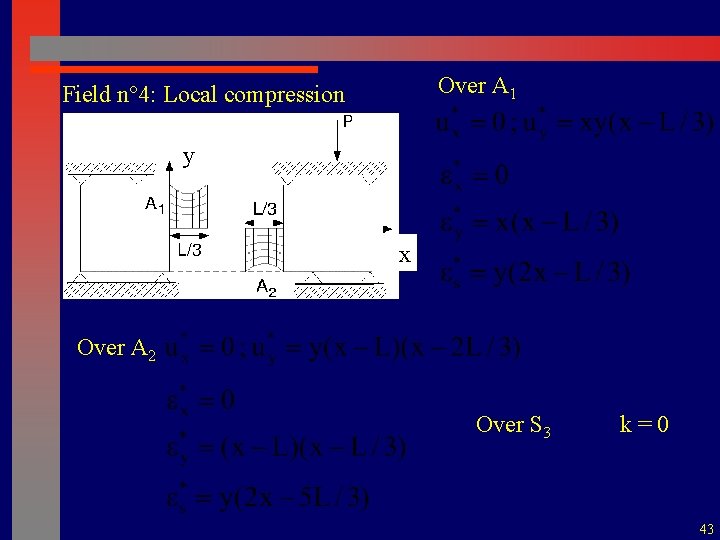
Over A 1 Field n° 4: Local compression y x Over A 2 Over S 3 k = 0 43

Field n° 4: Local compression 44

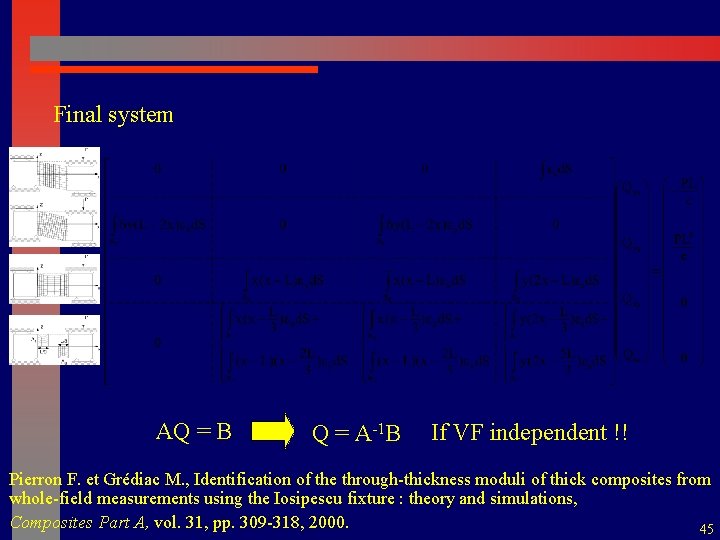
Final system AQ = B Q = A-1 B If VF independent !! Pierron F. et Grédiac M. , Identification of the through-thickness moduli of thick composites from whole-field measurements using the Iosipescu fixture : theory and simulations, Composites Part A, vol. 31, pp. 309 -318, 2000. 45

Experimental examples in linear elasticity 46

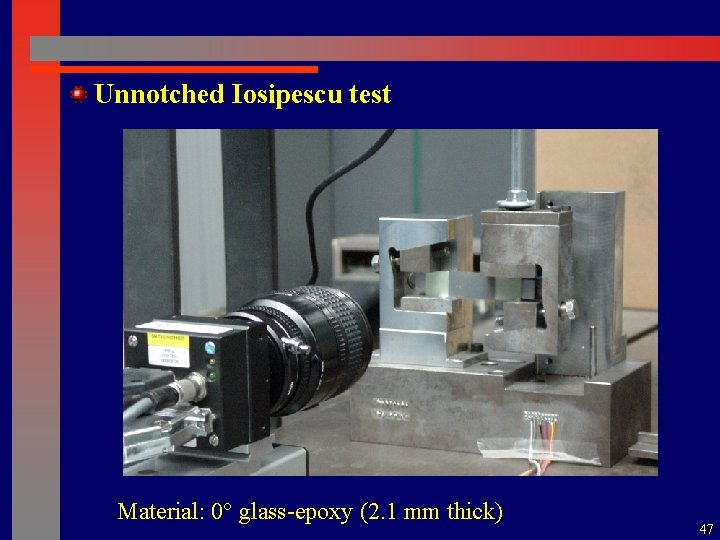
Unnotched Iosipescu test Material: 0° glass-epoxy (2. 1 mm thick) 47

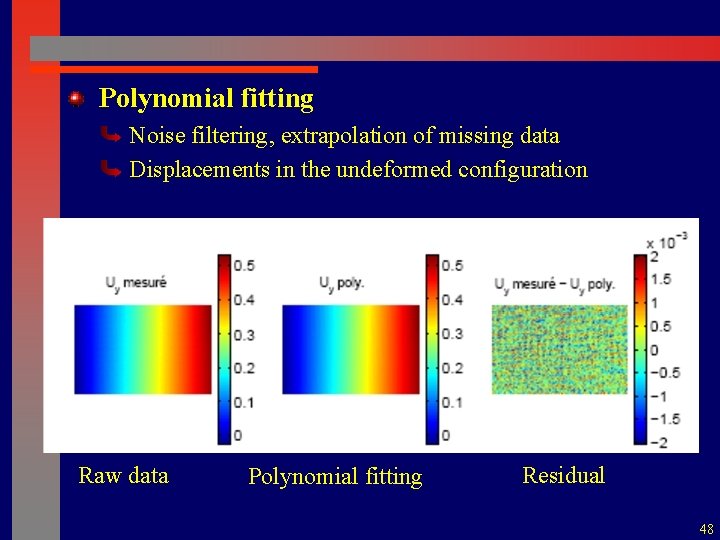
Polynomial fitting Noise filtering, extrapolation of missing data Displacements in the undeformed configuration Raw data Polynomial fitting Residual 48

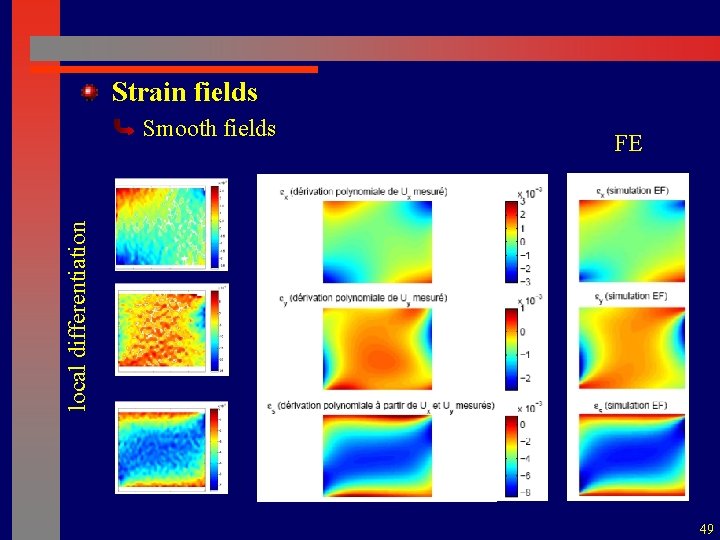
Strain fields FE local differentiation Smooth fields 49

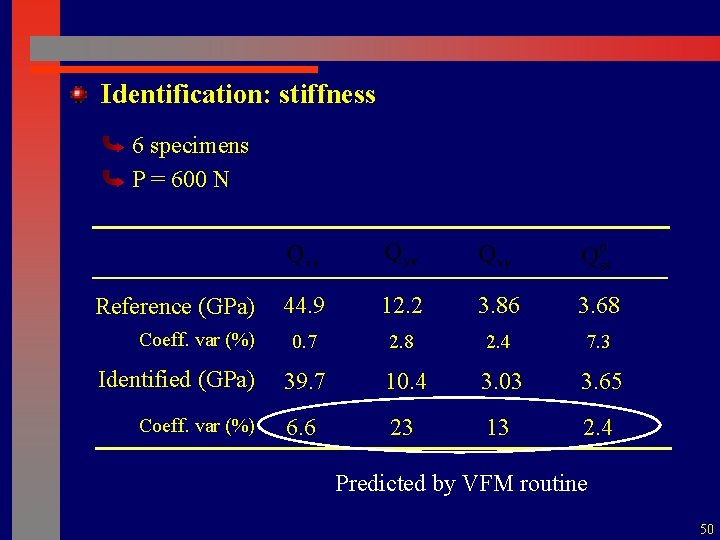
Identification: stiffness 6 specimens P = 600 N Reference (GPa) Coeff. var (%) Identified (GPa) Coeff. var (%) 44. 9 12. 2 3. 86 3. 68 0. 7 2. 8 2. 4 7. 3 39. 7 10. 4 3. 03 3. 65 6. 6 23 13 2. 4 Predicted by VFM routine 50

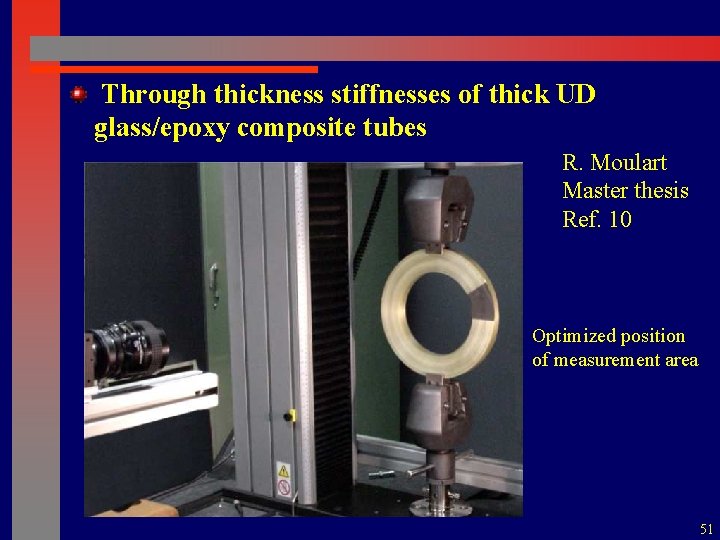
Through thickness stiffnesses of thick UD glass/epoxy composite tubes R. Moulart Master thesis Ref. 10 Optimized position of measurement area 51

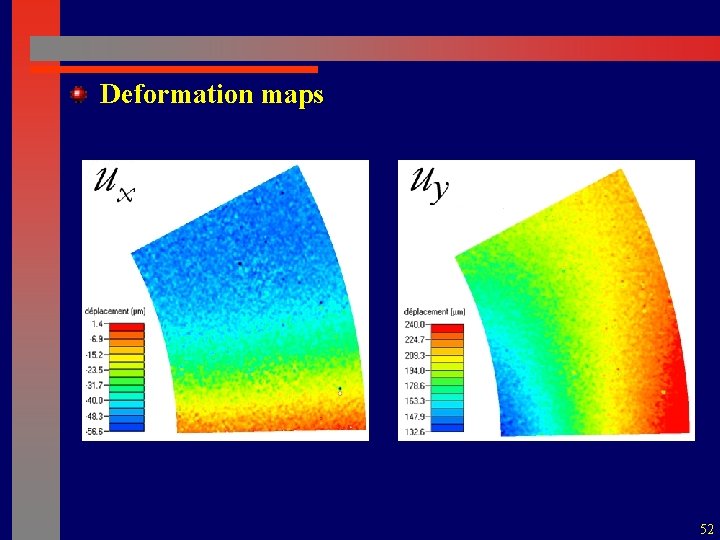
Deformation maps 52

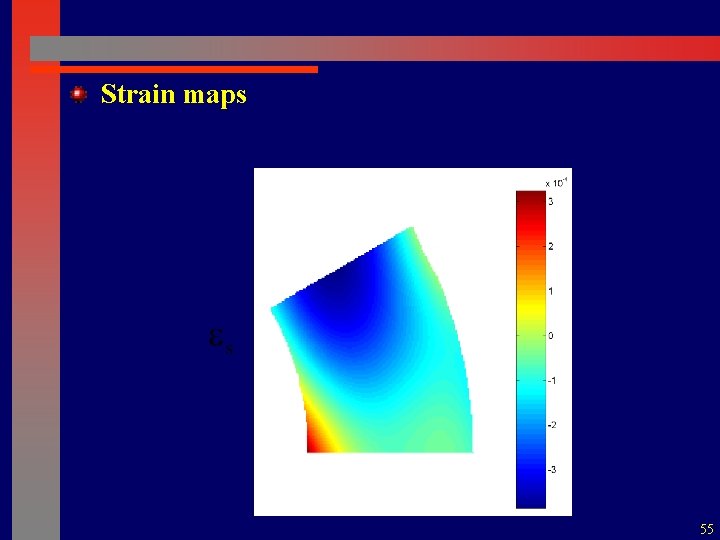
Strain maps Polynomial fit, degree 3, transform to cylindrical and analytical differentiation 53

Strain maps 54

Strain maps 55

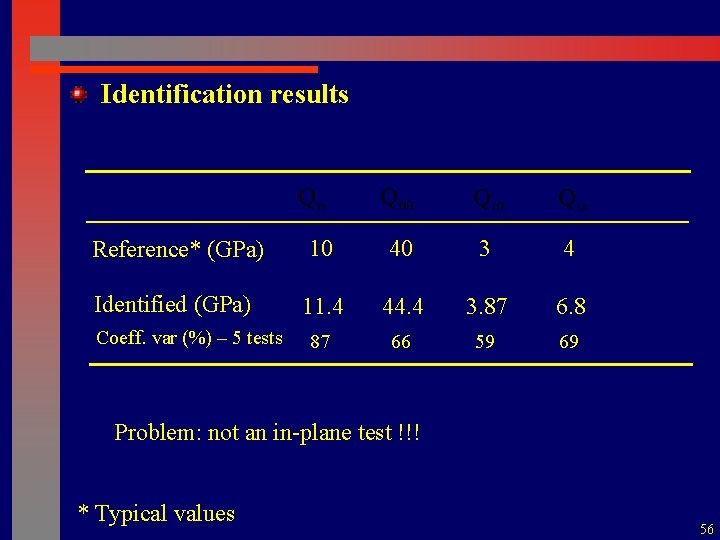
Identification results Reference* (GPa) 10 40 3 4 Identified (GPa) 11. 4 44. 4 3. 87 6. 8 87 66 59 69 Coeff. var (%) – 5 tests Problem: not an in-plane test !!! * Typical values 56

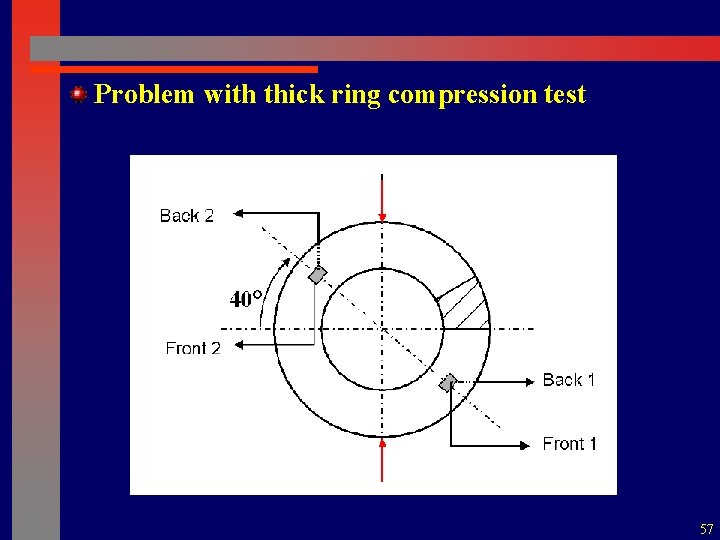
Problem with thick ring compression test 57

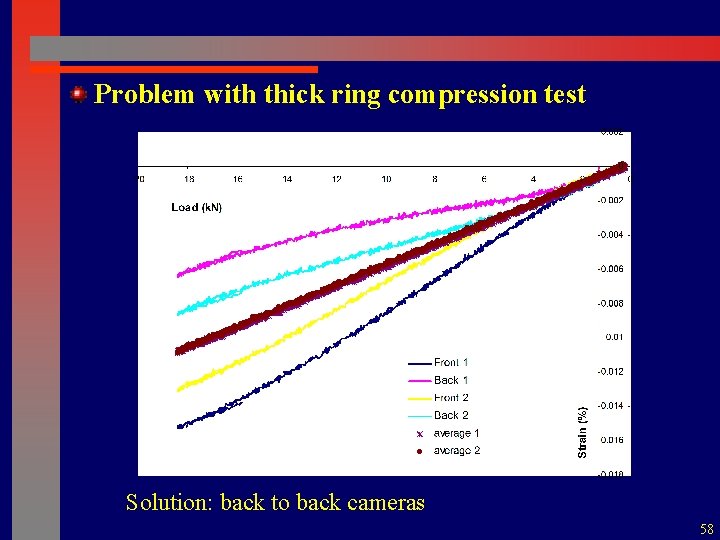
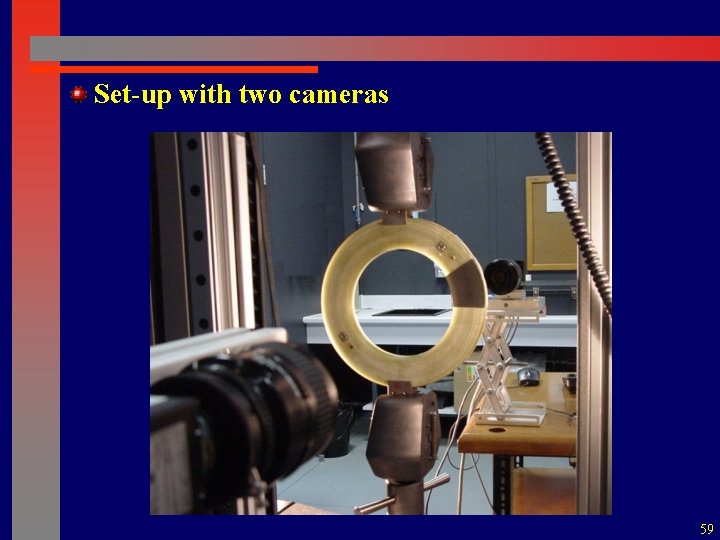
Problem with thick ring compression test Solution: back to back cameras 58

Set-up with two cameras 59

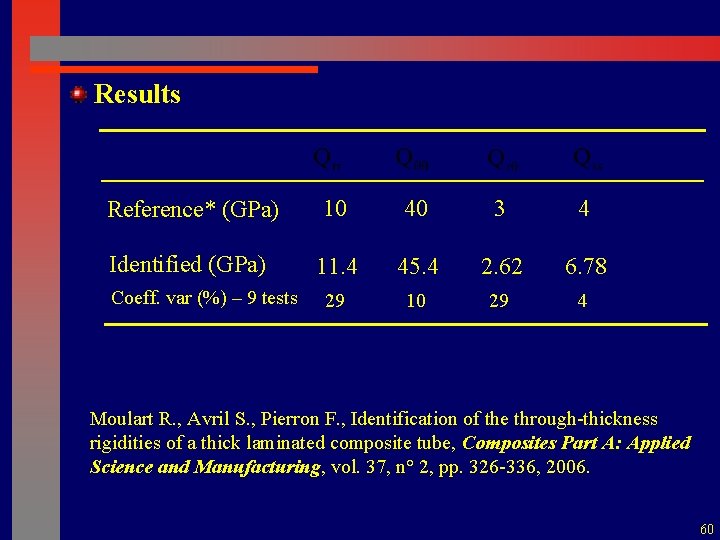
Results Reference* (GPa) 10 40 3 4 Identified (GPa) 11. 4 45. 4 2. 62 6. 78 29 10 29 4 Coeff. var (%) – 9 tests Moulart R. , Avril S. , Pierron F. , Identification of the through-thickness rigidities of a thick laminated composite tube, Composites Part A: Applied Science and Manufacturing, vol. 37, n° 2, pp. 326 -336, 2006. 60

ACKNOWLEDGEMENTS Professor Michel GREDIAC Blaise Pascal University, France Colleagues and students from my research group: Dr Stéphane Avril, Dr Alain Giraudeau, Dr René Rotinat Dr Hocine Chalal, Mr Baoqiao Guo, Dr Yannick Pannier, Mr Raphaël Moulart French CNRS network (GDR): « full-field measurements and identification in solid mechanics » 61

ACKNOWLEDGEMENTS Funding French Ministry for Research French National Research Agency (ANR) Champagne Ardenne Regional Council Engineering and Physical Sciences Research Council (UK) Airbus UK 62
 Virtual fields method
Virtual fields method Virtual fields method
Virtual fields method Red fields
Red fields Promotion from assistant to associate professor
Promotion from assistant to associate professor Multicullar
Multicullar Has virtual functions and accessible non-virtual destructor
Has virtual functions and accessible non-virtual destructor Papercut job tickerting print management
Papercut job tickerting print management Introduction product overview
Introduction product overview Introduction product overview
Introduction product overview Introduction product overview
Introduction product overview Symposium method of teaching pdf
Symposium method of teaching pdf Electric forces and fields concept review
Electric forces and fields concept review We plow the fields and scatter
We plow the fields and scatter New consultant training
New consultant training Learning: module 26: magnetic forces and fields
Learning: module 26: magnetic forces and fields Magnetism lesson outline answer key
Magnetism lesson outline answer key Chapter 16: electric forces and fields answers
Chapter 16: electric forces and fields answers Solve the following riddles
Solve the following riddles Displacement current formula
Displacement current formula Psychology branches and fields
Psychology branches and fields Chapter 33 conceptual physics
Chapter 33 conceptual physics Finite fields in cryptography and network security
Finite fields in cryptography and network security Electric currents and magnetic fields
Electric currents and magnetic fields Visualizing magnetic field
Visualizing magnetic field Hình ảnh bộ gõ cơ thể búng tay
Hình ảnh bộ gõ cơ thể búng tay Lp html
Lp html Bổ thể
Bổ thể Tỉ lệ cơ thể trẻ em
Tỉ lệ cơ thể trẻ em Chó sói
Chó sói Chụp tư thế worms-breton
Chụp tư thế worms-breton Chúa sống lại
Chúa sống lại Các môn thể thao bắt đầu bằng tiếng chạy
Các môn thể thao bắt đầu bằng tiếng chạy Thế nào là hệ số cao nhất
Thế nào là hệ số cao nhất Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Công thức tính thế năng
Công thức tính thế năng Trời xanh đây là của chúng ta thể thơ
Trời xanh đây là của chúng ta thể thơ Mật thư anh em như thể tay chân
Mật thư anh em như thể tay chân Làm thế nào để 102-1=99
Làm thế nào để 102-1=99 Phản ứng thế ankan
Phản ứng thế ankan Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Thơ thất ngôn tứ tuyệt đường luật
Thơ thất ngôn tứ tuyệt đường luật Quá trình desamine hóa có thể tạo ra
Quá trình desamine hóa có thể tạo ra Một số thể thơ truyền thống
Một số thể thơ truyền thống Bàn tay mà dây bẩn
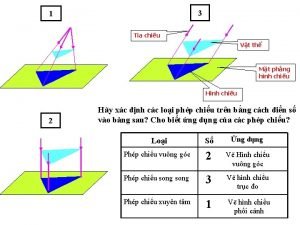
Bàn tay mà dây bẩn Vẽ hình chiếu vuông góc của vật thể sau
Vẽ hình chiếu vuông góc của vật thể sau Biện pháp chống mỏi cơ
Biện pháp chống mỏi cơ đặc điểm cơ thể của người tối cổ
đặc điểm cơ thể của người tối cổ V cc
V cc Vẽ hình chiếu đứng bằng cạnh của vật thể
Vẽ hình chiếu đứng bằng cạnh của vật thể Vẽ hình chiếu vuông góc của vật thể sau
Vẽ hình chiếu vuông góc của vật thể sau Thẻ vin
Thẻ vin đại từ thay thế
đại từ thay thế điện thế nghỉ
điện thế nghỉ Tư thế ngồi viết
Tư thế ngồi viết Diễn thế sinh thái là
Diễn thế sinh thái là Các loại đột biến cấu trúc nhiễm sắc thể
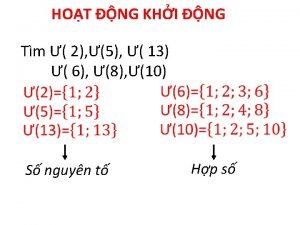
Các loại đột biến cấu trúc nhiễm sắc thể Thế nào là số nguyên tố
Thế nào là số nguyên tố Tư thế ngồi viết
Tư thế ngồi viết Lời thề hippocrates
Lời thề hippocrates Thiếu nhi thế giới liên hoan
Thiếu nhi thế giới liên hoan ưu thế lai là gì
ưu thế lai là gì Khi nào hổ con có thể sống độc lập
Khi nào hổ con có thể sống độc lập Khi nào hổ con có thể sống độc lập
Khi nào hổ con có thể sống độc lập