Springs and Hookes Law Physics 11 Springs A









- Slides: 12

Springs and Hooke’s Law Physics 11

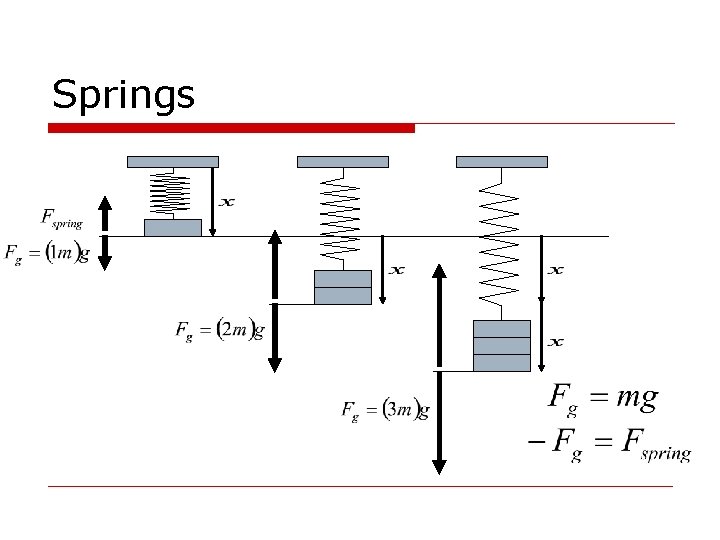
Springs A mass-spring system is given below. As mass is added to the end of the spring, how would you expect the spring to stretch?

Springs

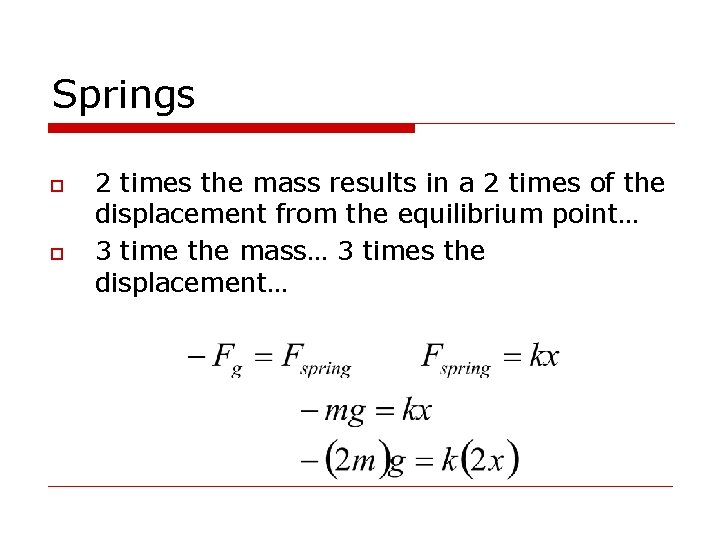
Springs o o 2 times the mass results in a 2 times of the displacement from the equilibrium point… 3 time the mass… 3 times the displacement…

What kind of energy is this? o Potential Energy n Elastic Potential Energy to be exact!

What else besides springs has elastic potential energy? o o o Diving boards Bows (bow and arrows) Bungee cord

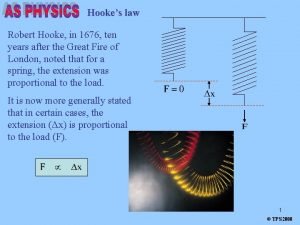
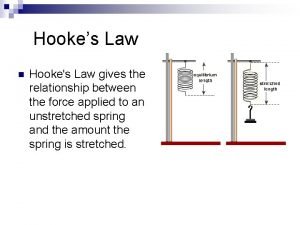
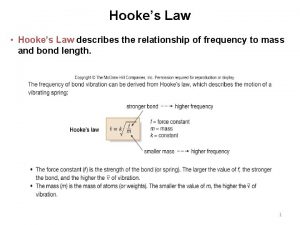
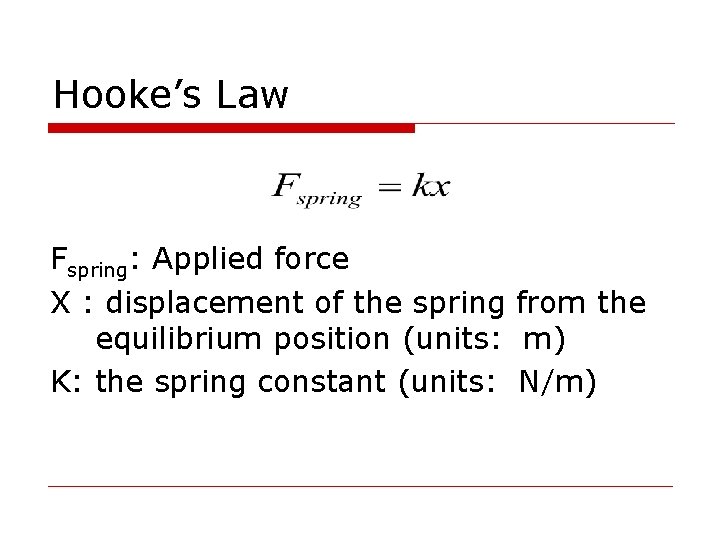
Hooke’s Law Fspring: Applied force X : displacement of the spring from the equilibrium position (units: m) K: the spring constant (units: N/m)

Hooke’s Law o the restoring force is opposite the applied force. (negative sign) n Gravity applied in the negative direction, the restoring force is in the positive direction

Example o An archery bow requires a force of 133 N to hold an arrow at “full draw” (pulled back 71 cm). Assuming that the bow obeys Hooke’s Law, what is its spring constant?

o o F = kx 133 = k(0. 71) k = 133/0. 71 k = 187. 32 N/m 190 N/m

Restoring Force o o The restoring force is the force that is needed to put the spring back to equilibrium. Example: If you stretch a spring by 0. 5 m and you had to use 150 N of force, the restoring force is -150 N.

Example 2: o A 70. kg person bungee jumps off a 50. m bridge with his ankles attached to a 15 m long bungee cord. Assume the person stops at the edge of the water and he is 2. 0 m tall, what is the force constant of the bungee cord?
 Hooke's law stress strain
Hooke's law stress strain Hooke's law formula
Hooke's law formula A graph of force against extension
A graph of force against extension Hooke's law example
Hooke's law example Hookes law
Hookes law State hooke's law in physics
State hooke's law in physics Newton's first law and second law and third law
Newton's first law and second law and third law Newton's first law
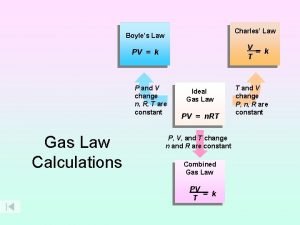
Newton's first law Boyle's law charles law avogadro's law
Boyle's law charles law avogadro's law Charles law constant
Charles law constant Why does it happen
Why does it happen University physics with modern physics fifteenth edition
University physics with modern physics fifteenth edition Physics ia topic
Physics ia topic