Special Right Triangles Theorem 4590 Triangle Theorem In


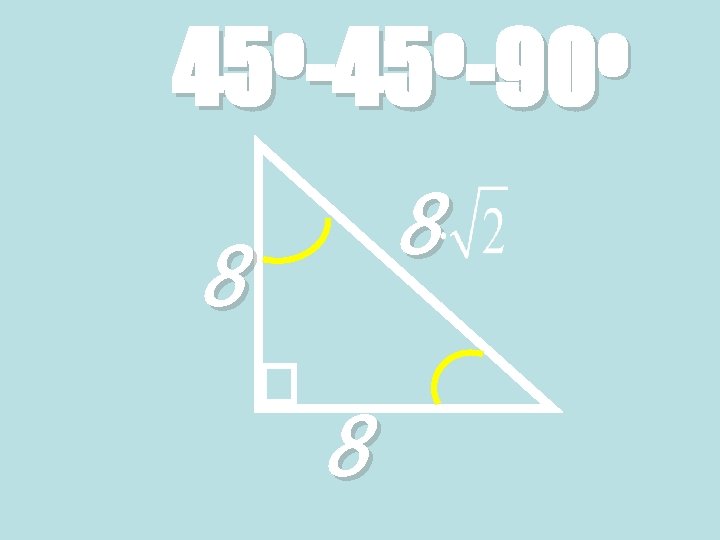
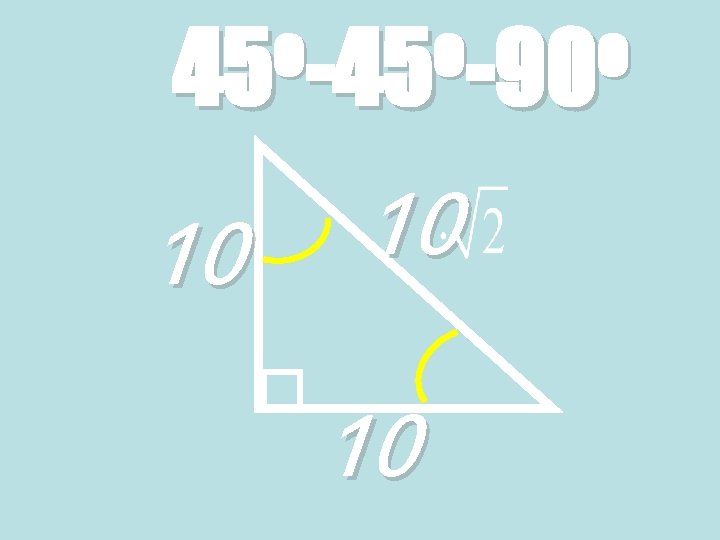


- Slides: 33

Special Right Triangles

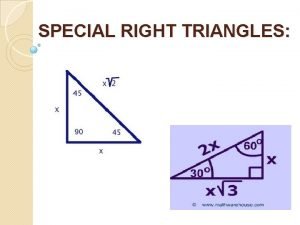
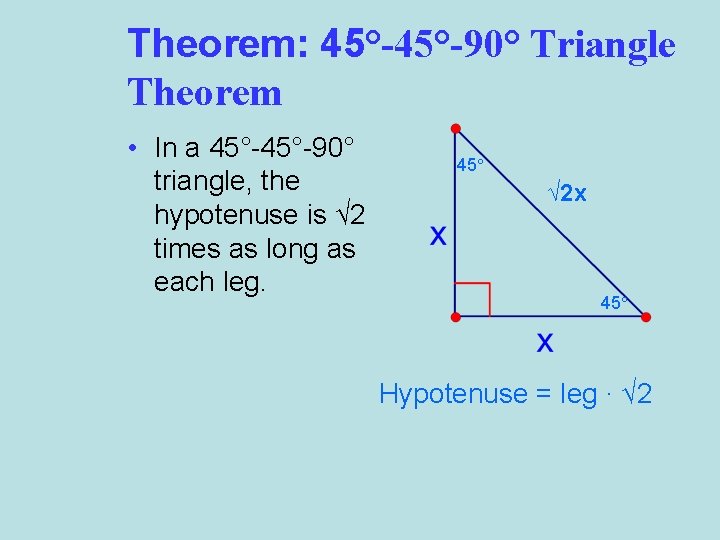
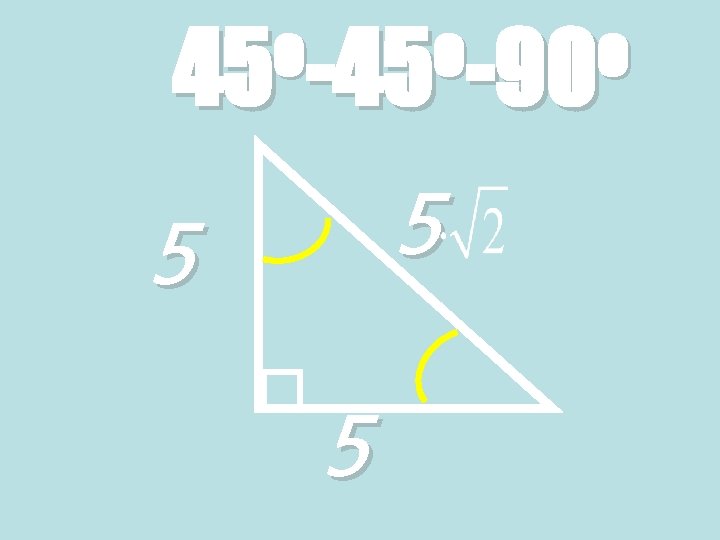
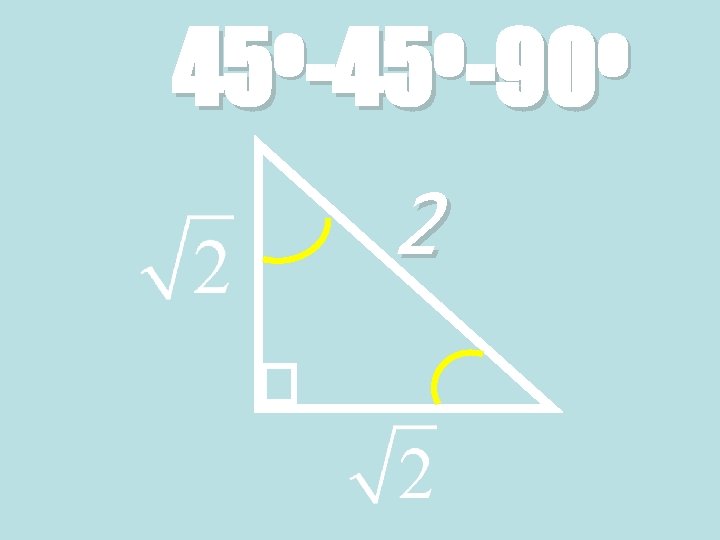
Theorem: 45°-90° Triangle Theorem • In a 45°-90° triangle, the hypotenuse is √ 2 times as long as each leg. 45° √ 2 x 45° Hypotenuse = leg ∙ √ 2

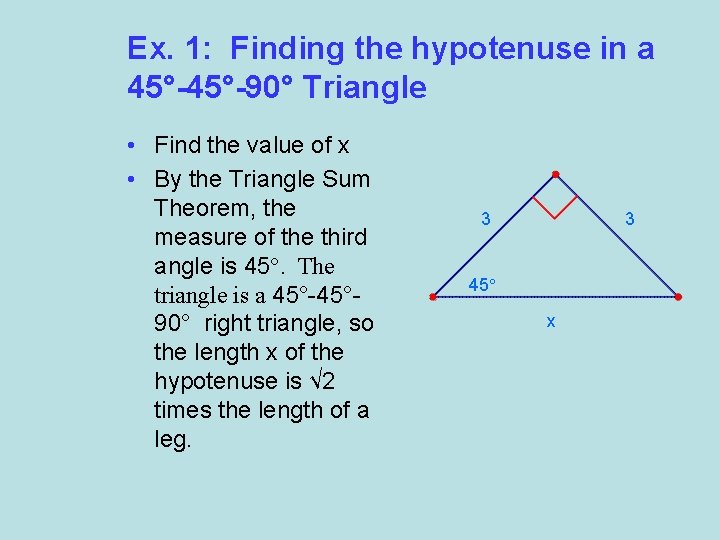
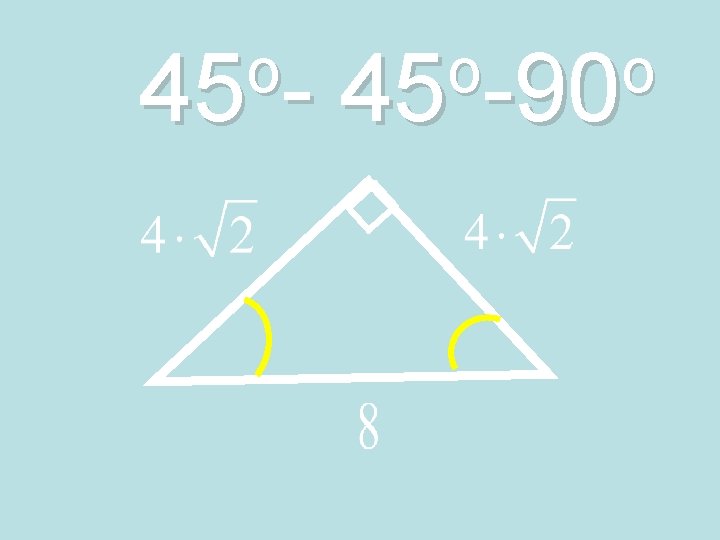
Ex. 1: Finding the hypotenuse in a 45°-90° Triangle • Find the value of x • By the Triangle Sum Theorem, the measure of the third angle is 45°. The triangle is a 45°-45° 90° right triangle, so the length x of the hypotenuse is √ 2 times the length of a leg. 3 3 45° x

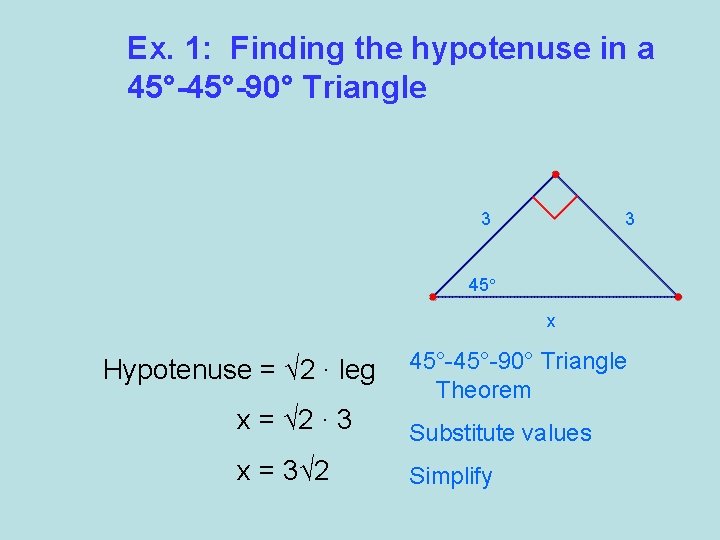
Ex. 1: Finding the hypotenuse in a 45°-90° Triangle 3 3 45° x Hypotenuse = √ 2 ∙ leg 45°-90° Triangle Theorem x = √ 2 ∙ 3 Substitute values x = 3√ 2 Simplify

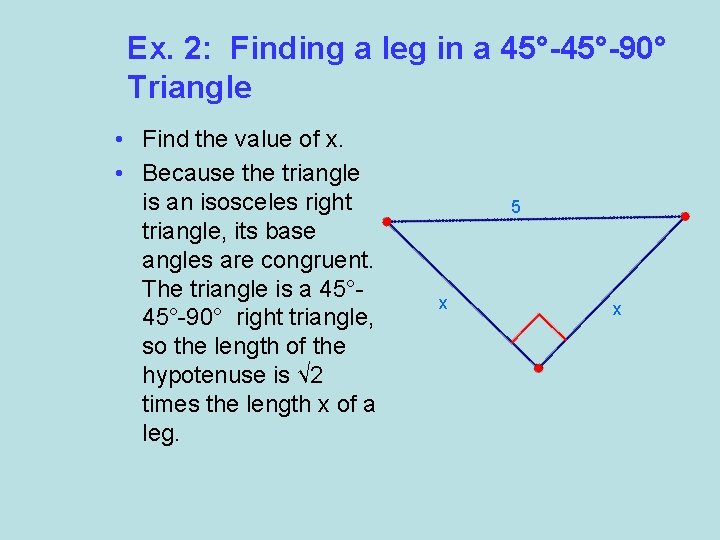
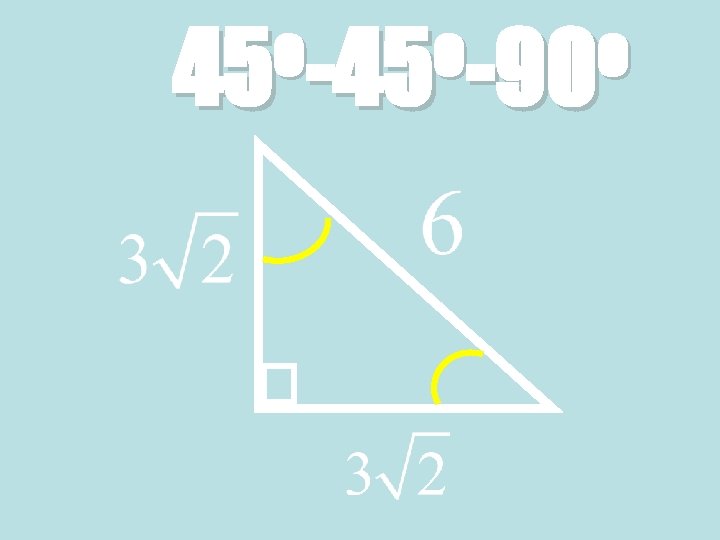
Ex. 2: Finding a leg in a 45°-90° Triangle • Find the value of x. • Because the triangle is an isosceles right triangle, its base angles are congruent. The triangle is a 45°-90° right triangle, so the length of the hypotenuse is √ 2 times the length x of a leg. 5 x x

Ex. 2: Finding a leg in a 45°-90° 5 Triangle x Statement: Reasons: Hypotenuse = √ 2 ∙ leg 5 = √ 2 ∙ x 5 √ 2 √ 2 x 5 √ 2 5√ 2 2 = √ 2 x √ 2 = x 45°-90° Triangle Theorem Substitute values Divide each side by √ 2 Simplify = x Multiply numerator and denominator by √ 2 = x Simplify
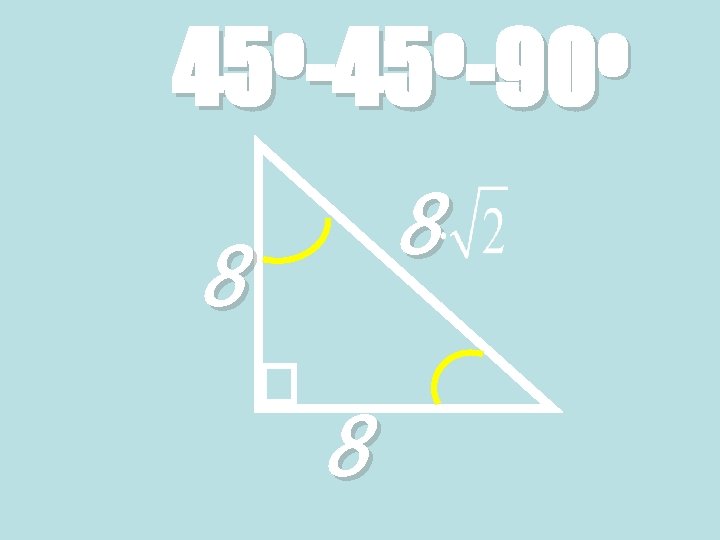
o o o 45 -90 8 8 8

o o o 45 -90 5 5 5
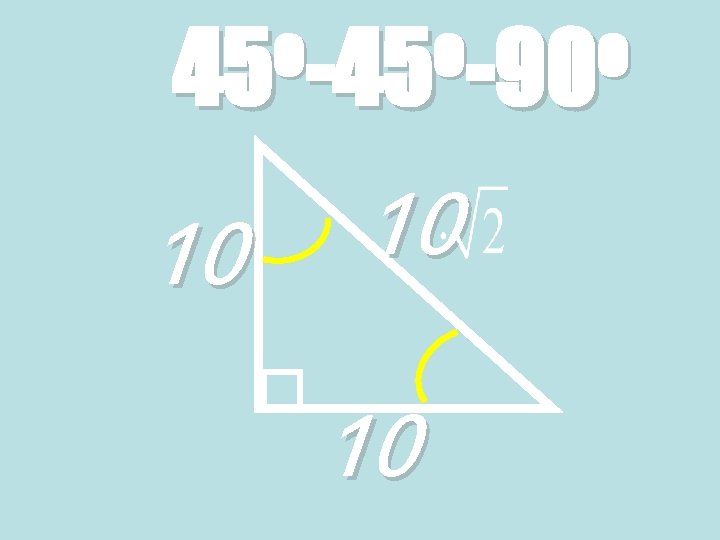
o o o 45 -90 10 10 10

o o o 45 -90 2

o o o 45 -90

o 45 - o o 45 -90

o o o 45 -90

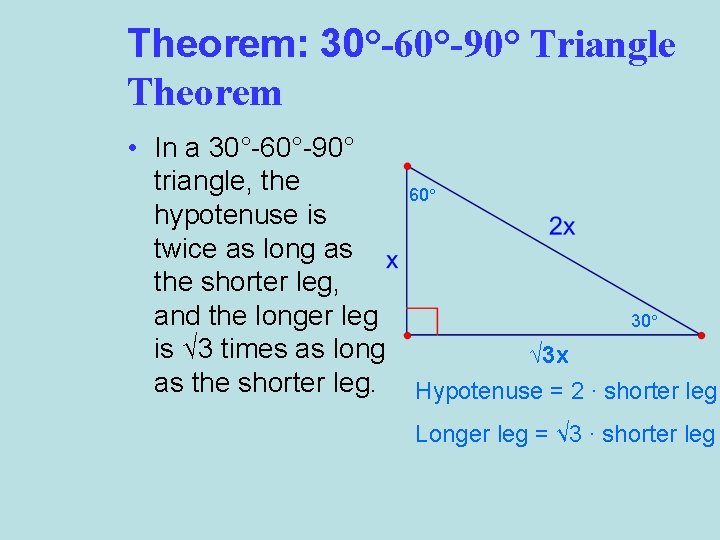
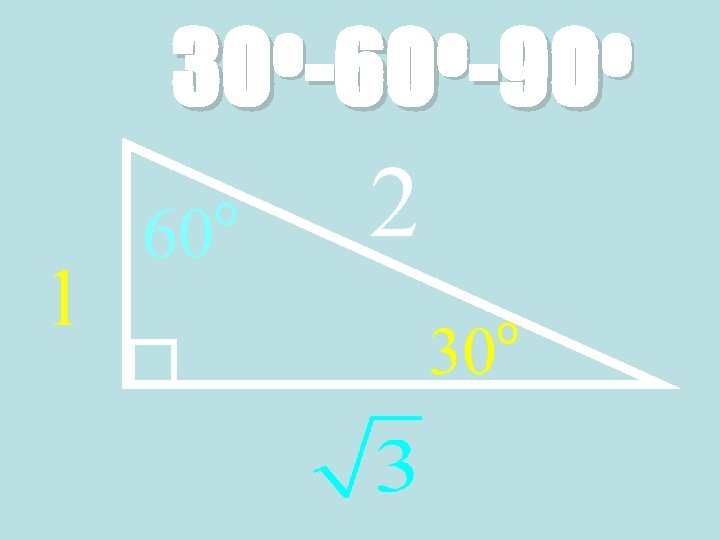
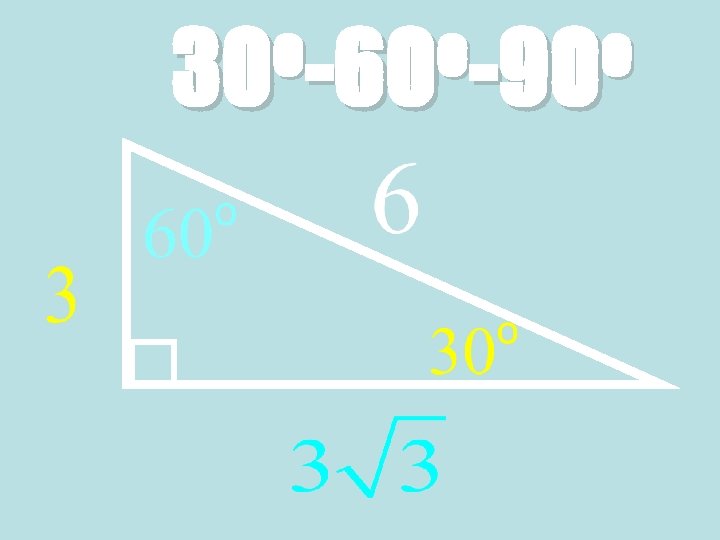
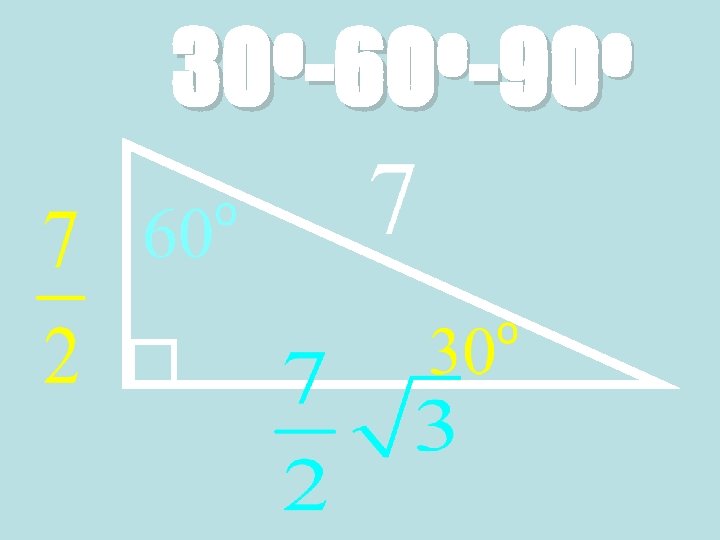
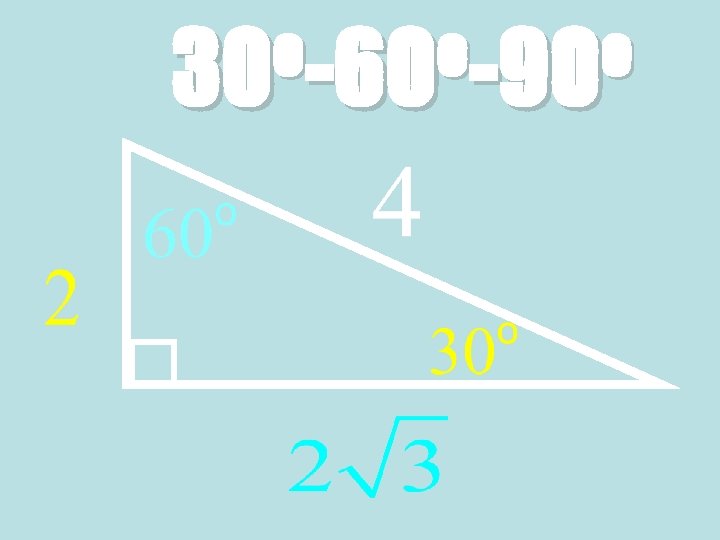
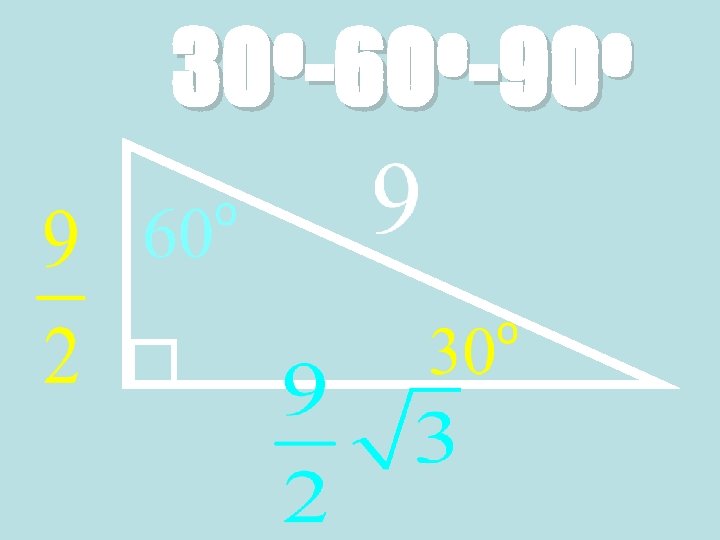
Theorem: 30°-60°-90° Triangle Theorem • In a 30°-60°-90° triangle, the hypotenuse is twice as long as the shorter leg, and the longer leg is √ 3 times as long as the shorter leg. 60° 30° √ 3 x Hypotenuse = 2 ∙ shorter leg Longer leg = √ 3 ∙ shorter leg

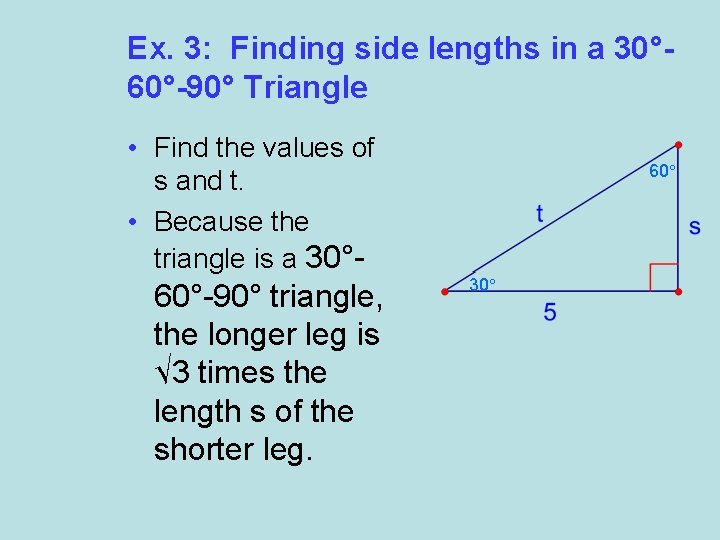
Ex. 3: Finding side lengths in a 30° 60°-90° Triangle • Find the values of s and t. • Because the triangle is a 30°- 60°-90° triangle, the longer leg is √ 3 times the length s of the shorter leg. 60° 30°

Ex. 3: Side lengths in a 30°-60°-90° Triangle 60° 30° Statement: Reasons: Longer leg = √ 3 ∙ shorter leg 5 = √ 3 ∙ s 5 √ 3 √ 3 5√ 3 3 = √ 3 s √ 3 = s 30°-60°-90° Triangle Theorem Substitute values Divide each side by √ 3 Simplify = s Multiply numerator and denominator by √ 3 = s Simplify

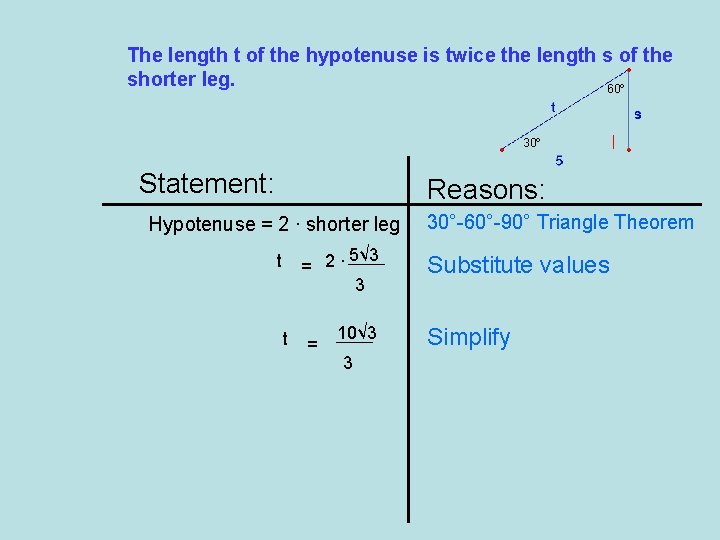
The length t of the hypotenuse is twice the length s of the shorter leg. 60° 30° Statement: Reasons: Hypotenuse = 2 ∙ shorter leg 5√ 3 = 2∙ 3 t t = 10√ 3 3 30°-60°-90° Triangle Theorem Substitute values Simplify

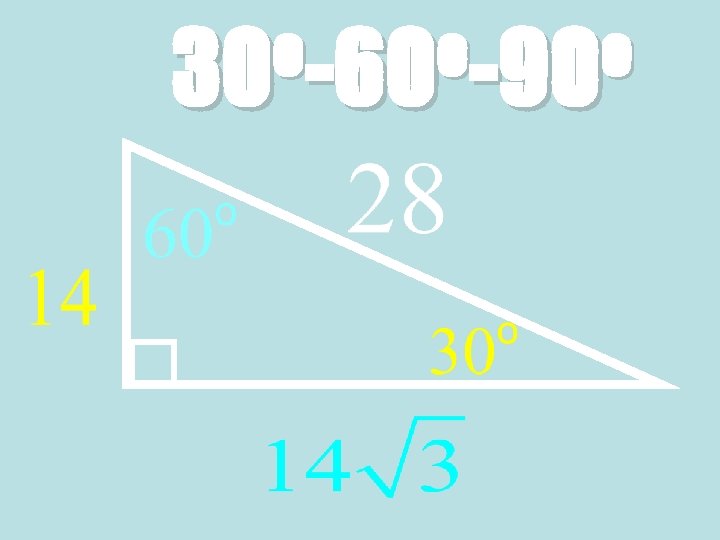
o o o 30 -60 -90

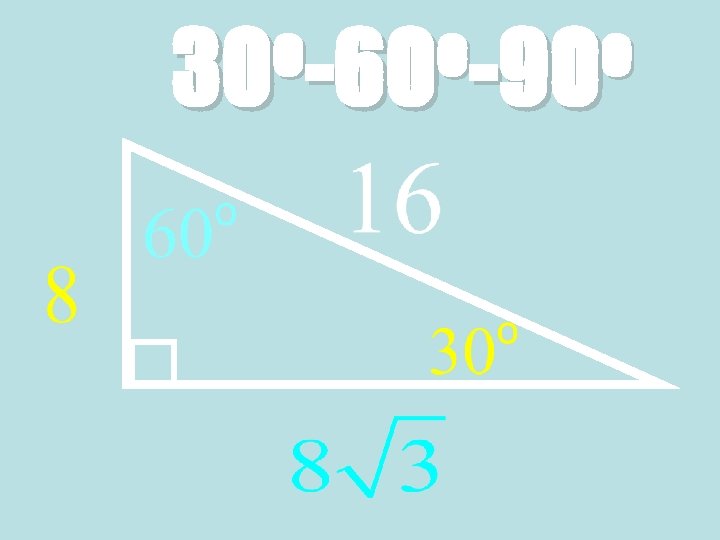
o o o 30 -60 -90

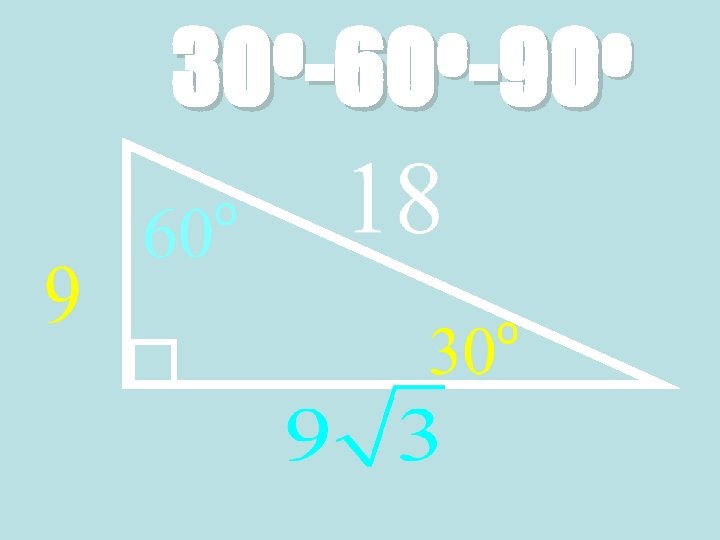
o o o 30 -60 -90

o o o 30 -60 -90

o o o 30 -60 -90

o o o 30 -60 -90

o o o 30 -60 -90

o o o 30 -60 -90

o o o 30 -60 -90

o o o 30 -60 -90

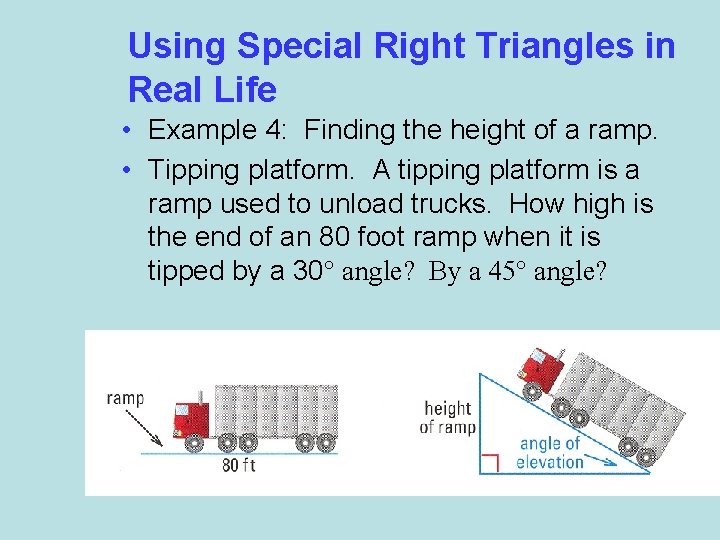
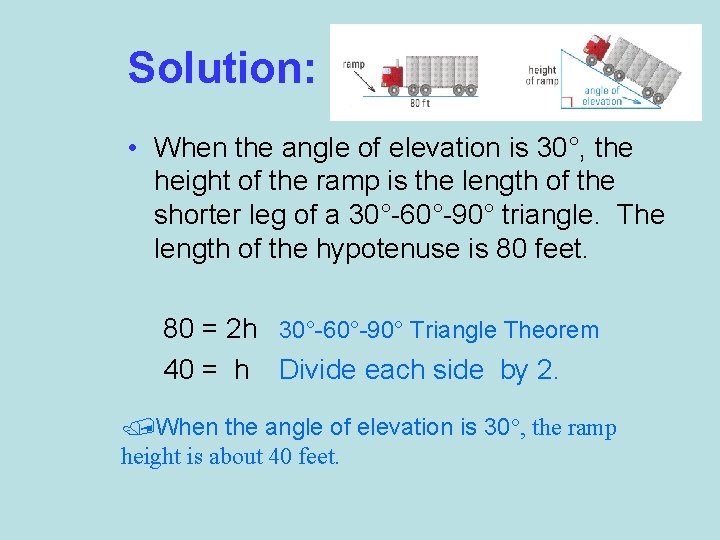
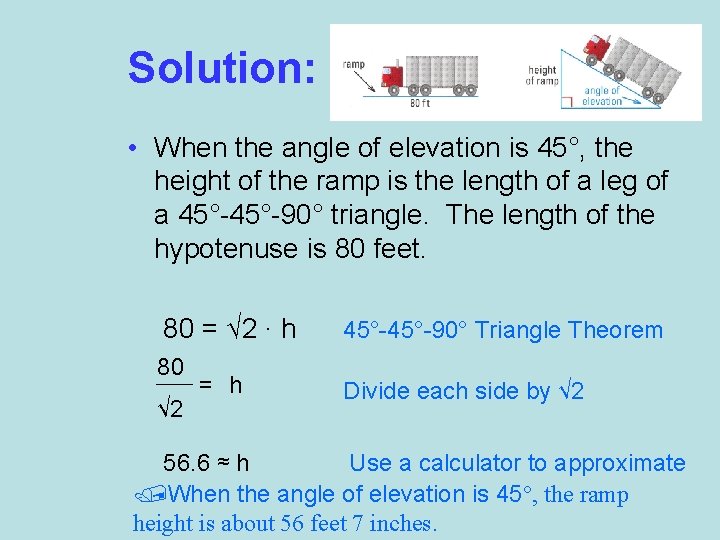
Using Special Right Triangles in Real Life • Example 4: Finding the height of a ramp. • Tipping platform. A tipping platform is a ramp used to unload trucks. How high is the end of an 80 foot ramp when it is tipped by a 30° angle? By a 45° angle?

Solution: • When the angle of elevation is 30°, the height of the ramp is the length of the shorter leg of a 30°-60°-90° triangle. The length of the hypotenuse is 80 feet. 80 = 2 h 30°-60°-90° Triangle Theorem 40 = h Divide each side by 2. When the angle of elevation is 30°, the ramp height is about 40 feet.

Solution: • When the angle of elevation is 45°, the height of the ramp is the length of a leg of a 45°-90° triangle. The length of the hypotenuse is 80 feet. 80 = √ 2 ∙ h 80 √ 2 = h 45°-90° Triangle Theorem Divide each side by √ 2 56. 6 ≈ h Use a calculator to approximate When the angle of elevation is 45°, the ramp height is about 56 feet 7 inches.

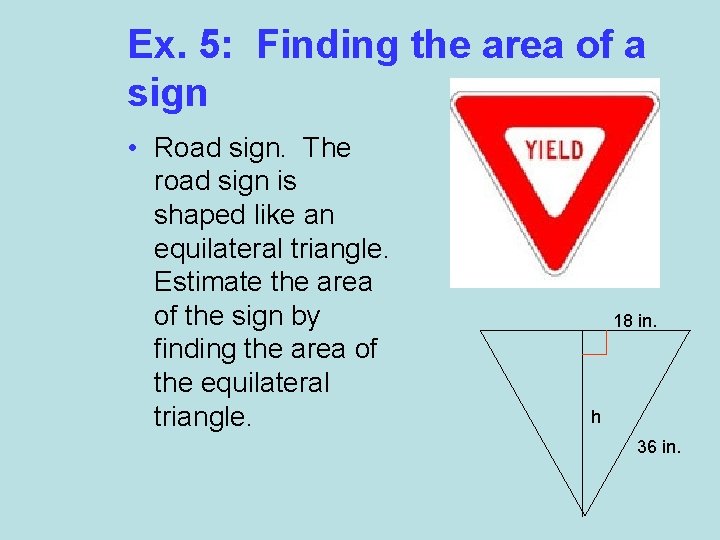
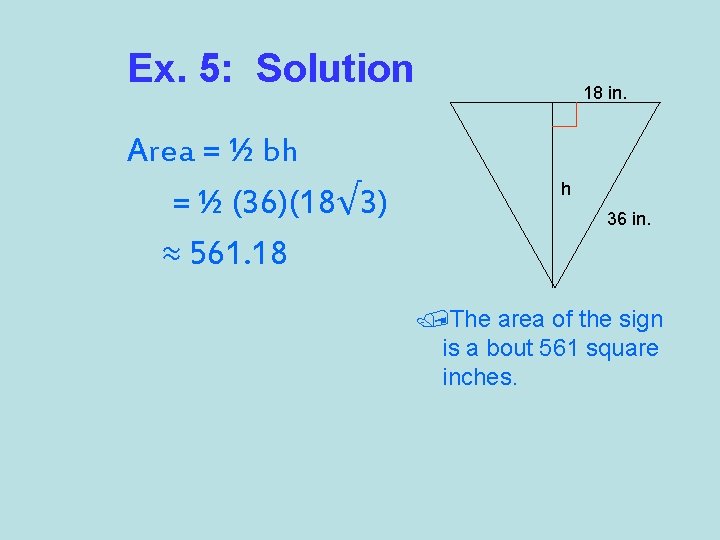
Ex. 5: Finding the area of a sign • Road sign. The road sign is shaped like an equilateral triangle. Estimate the area of the sign by finding the area of the equilateral triangle. 18 in. h 36 in.

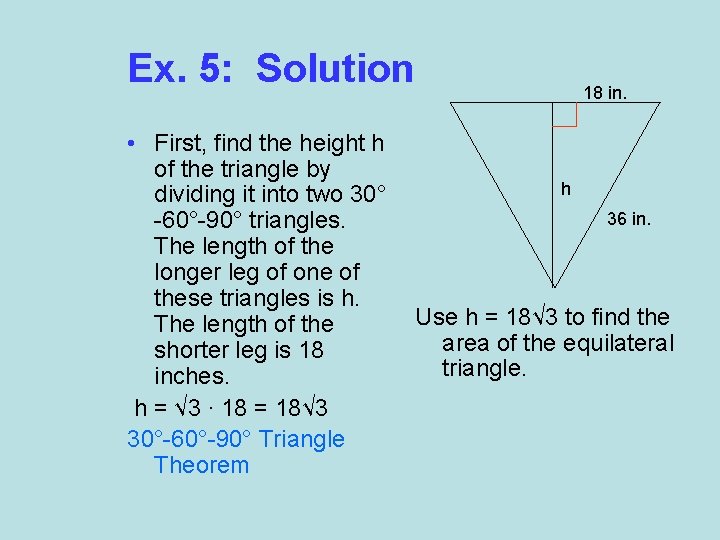
Ex. 5: Solution • First, find the height h of the triangle by dividing it into two 30° -60°-90° triangles. The length of the longer leg of one of these triangles is h. The length of the shorter leg is 18 inches. h = √ 3 ∙ 18 = 18√ 3 30°-60°-90° Triangle Theorem 18 in. h 36 in. Use h = 18√ 3 to find the area of the equilateral triangle.

Ex. 5: Solution Area = ½ bh = ½ (36)(18√ 3) ≈ 561. 18 18 in. h 36 in. The area of the sign is a bout 561 square inches.
 30 60 90 theorem statement
30 60 90 theorem statement Right product right place right time right price
Right product right place right time right price Right time right place right quantity right quality
Right time right place right quantity right quality Special right triangles
Special right triangles Special right triangles radians
Special right triangles radians Lesson 13-3 special right triangles
Lesson 13-3 special right triangles δqrs is a right triangle.
δqrs is a right triangle. Lesson 7-3 triangles (homework practice)
Lesson 7-3 triangles (homework practice) Special right triangles
Special right triangles Special properties of right triangles
Special properties of right triangles 8-4 special right triangles
8-4 special right triangles Common triangles
Common triangles Section 7 topic 4 special right triangles
Section 7 topic 4 special right triangles Special right triangles in real life
Special right triangles in real life Special right triangles calculator
Special right triangles calculator Lesson 5-8 applying special right triangles answer key
Lesson 5-8 applying special right triangles answer key 5-8 applying special right triangles
5-8 applying special right triangles Simplest radical form of 45
Simplest radical form of 45 8-2 special right triangles
8-2 special right triangles Unit 3 lesson 2 special right triangles
Unit 3 lesson 2 special right triangles Special triangles practice
Special triangles practice Special right triangle investigation
Special right triangle investigation 5-8 practice b applying special right triangles
5-8 practice b applying special right triangles Find the variable
Find the variable 5-8 applying special right triangles
5-8 applying special right triangles 5-8 applying special right triangles
5-8 applying special right triangles Special right triangle investigation
Special right triangle investigation A2+b2=c2
A2+b2=c2 Applying special right triangles
Applying special right triangles Trigonometric ratios
Trigonometric ratios The right man on the right place at the right time
The right man on the right place at the right time Cpctc theorem
Cpctc theorem Geometric mean in right triangles
Geometric mean in right triangles 8-1 similarity in right triangles
8-1 similarity in right triangles