SOLVING SYSTEMS USING SUBSTITUTION 6 2 Solve the

SOLVING SYSTEMS USING SUBSTITUTION 6 -2

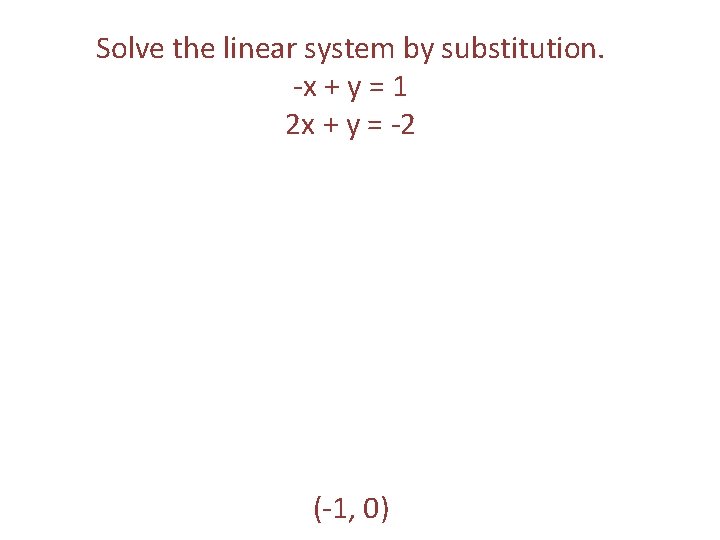
Solve the linear system by substitution. -x + y = 1 2 x + y = -2 (-1, 0)

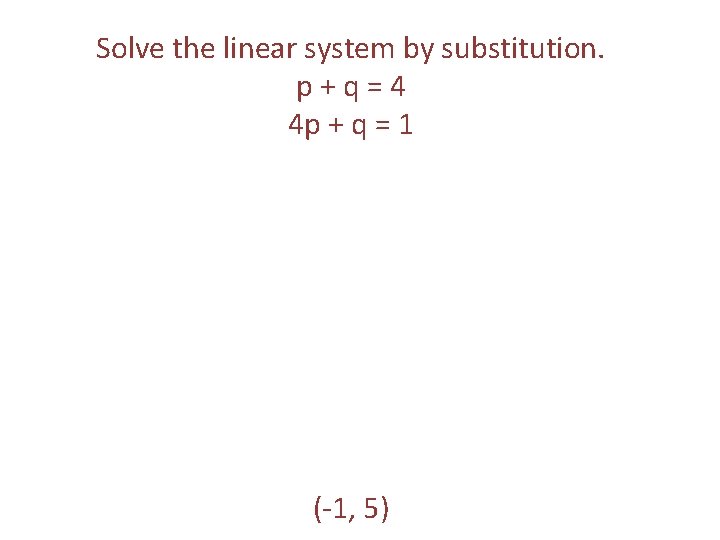
Solve the linear system by substitution. p+q=4 4 p + q = 1 (-1, 5)

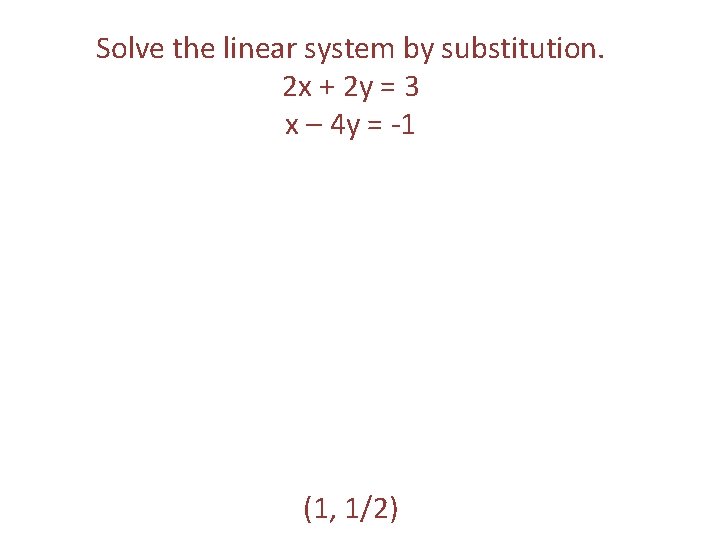
Solve the linear system by substitution. 2 x + 2 y = 3 x – 4 y = -1 (1, 1/2)

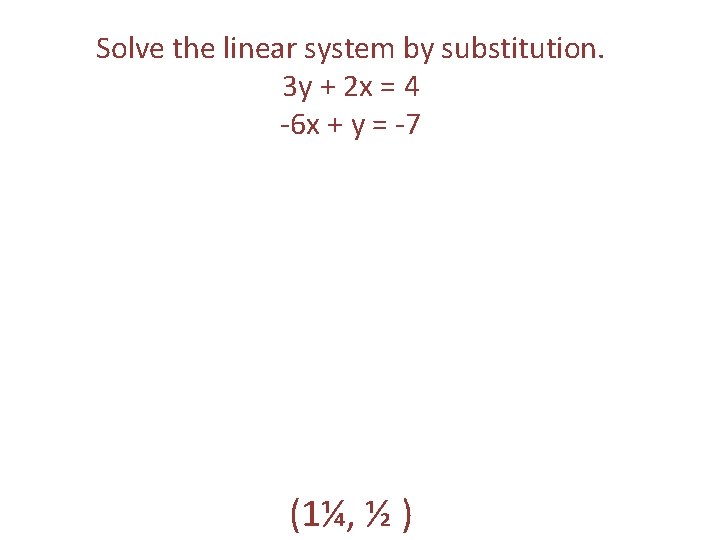
Solve the linear system by substitution. 3 y + 2 x = 4 -6 x + y = -7 (1¼, ½ )

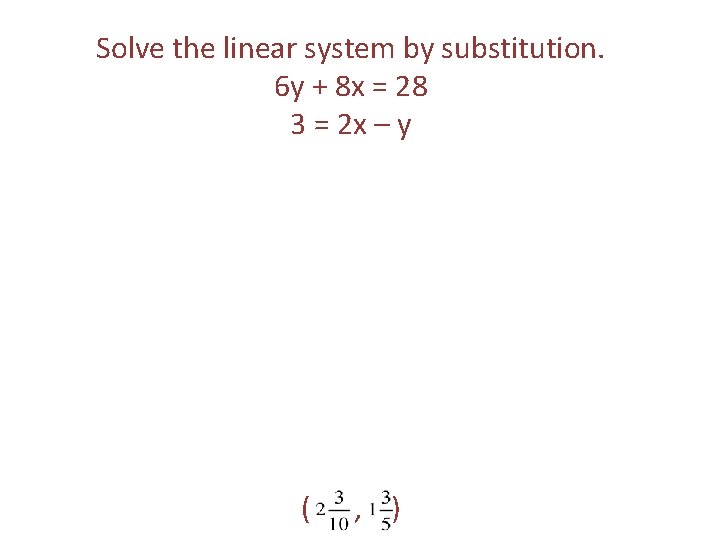
Solve the linear system by substitution. 6 y + 8 x = 28 3 = 2 x – y ( , )

Solve the linear system by substitution. 3 x + y = 5 2 x – y = 10 (3, -4)

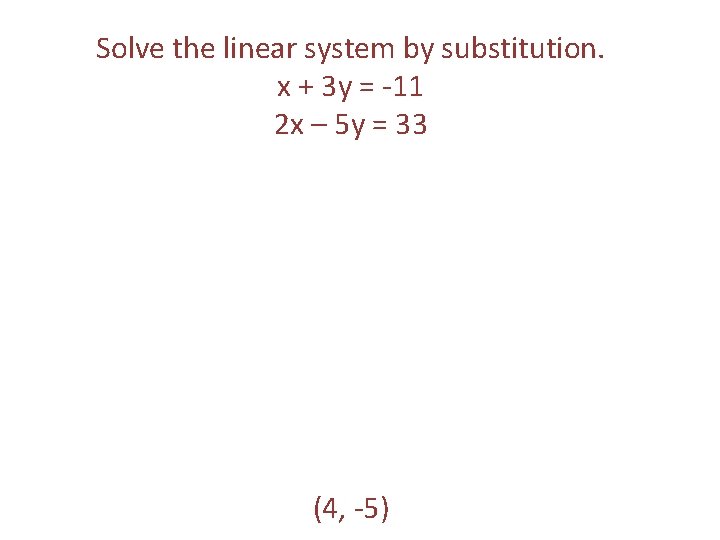
Solve the linear system by substitution. x + 3 y = -11 2 x – 5 y = 33 (4, -5)

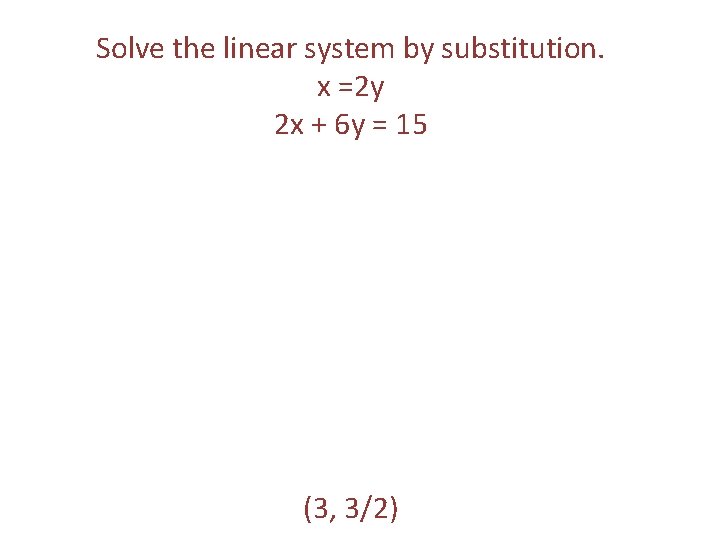
Solve the linear system by substitution. x =2 y 2 x + 6 y = 15 (3, 3/2)

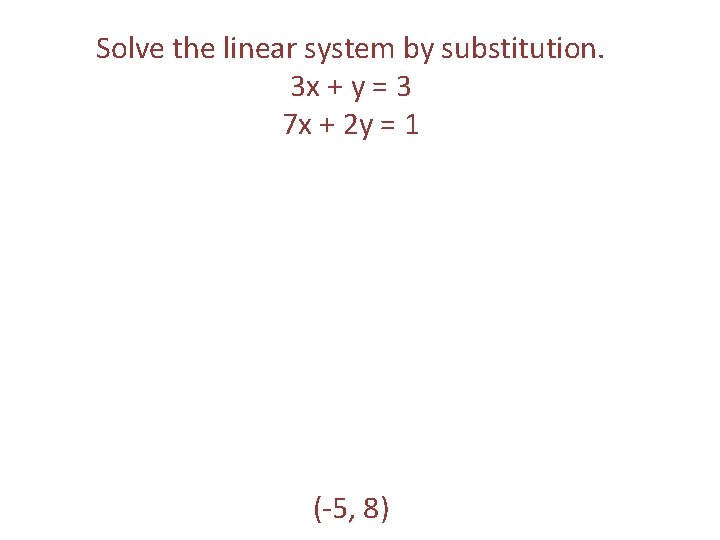
Solve the linear system by substitution. 3 x + y = 3 7 x + 2 y = 1 (-5, 8)

Solve the linear system by substitution. 2 x + y = 4 -x + y = 1 (1, 2)

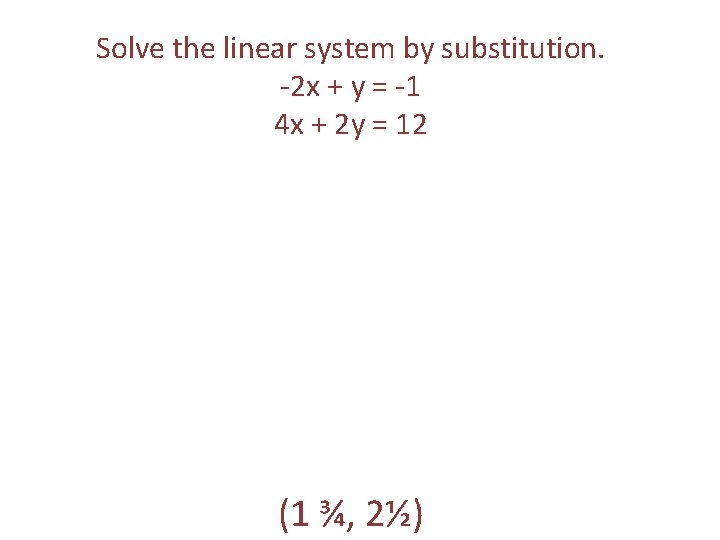
Solve the linear system by substitution. -2 x + y = -1 4 x + 2 y = 12 (1 ¾, 2½)

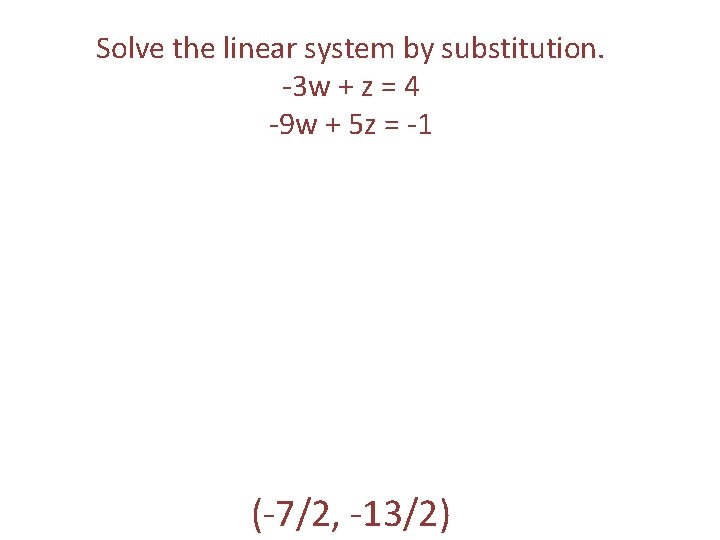
Solve the linear system by substitution. -3 w + z = 4 -9 w + 5 z = -1 (-7/2, -13/2)

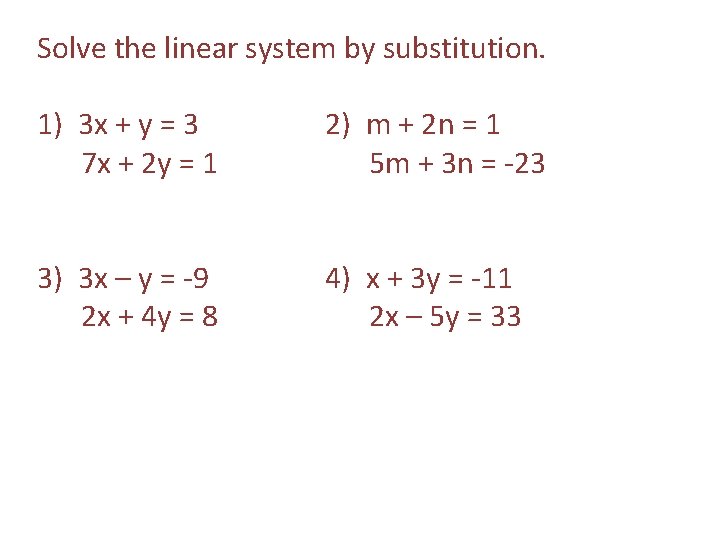
Solve the linear system by substitution. 1) 3 x + y = 3 7 x + 2 y = 1 2) m + 2 n = 1 5 m + 3 n = -23 3) 3 x – y = -9 2 x + 4 y = 8 4) x + 3 y = -11 2 x – 5 y = 33

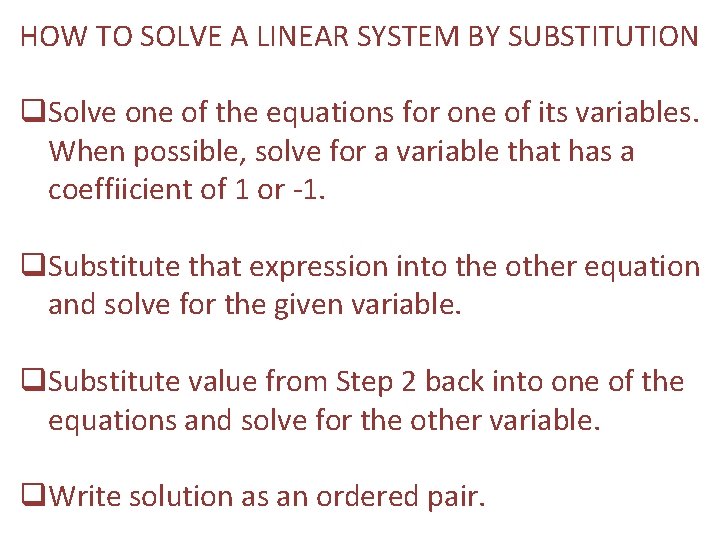
HOW TO SOLVE A LINEAR SYSTEM BY SUBSTITUTION q. Solve one of the equations for one of its variables. When possible, solve for a variable that has a coeffiicient of 1 or -1. q. Substitute that expression into the other equation and solve for the given variable. q. Substitute value from Step 2 back into one of the equations and solve for the other variable. q. Write solution as an ordered pair.