Solving Inequalities Problem Solving with Inequalities Review Inequalities

Solving Inequalities & Problem Solving with Inequalities

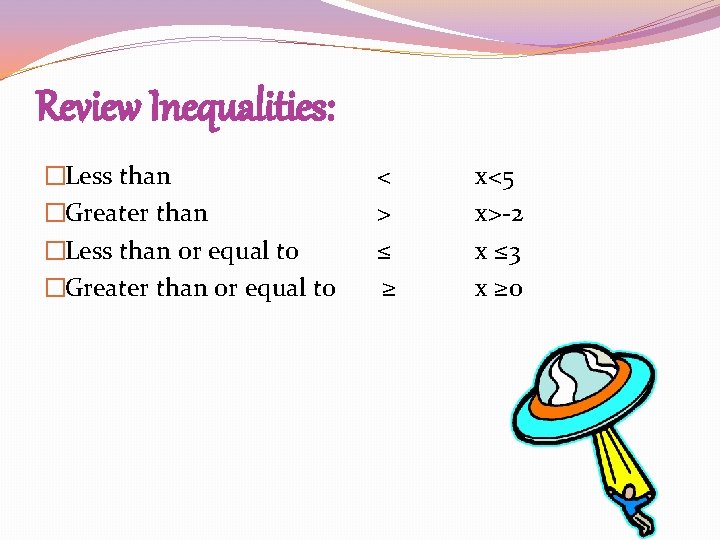
Review Inequalities: �Less than �Greater than �Less than or equal to �Greater than or equal to < > ≤ ≥ x<5 x>-2 x ≤ 3 x ≥ 0

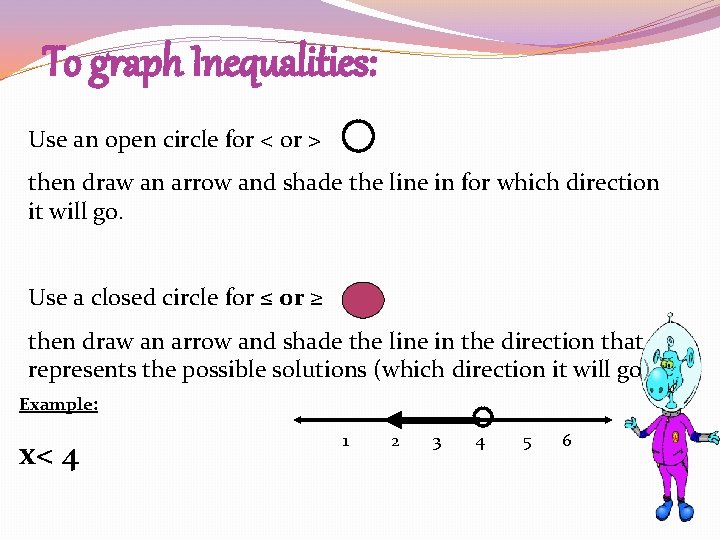
To graph Inequalities: Use an open circle for < or > then draw an arrow and shade the line in for which direction it will go. Use a closed circle for ≤ or ≥ then draw an arrow and shade the line in the direction that represents the possible solutions (which direction it will go). Example: x< 4 1 2 3 4 5 6

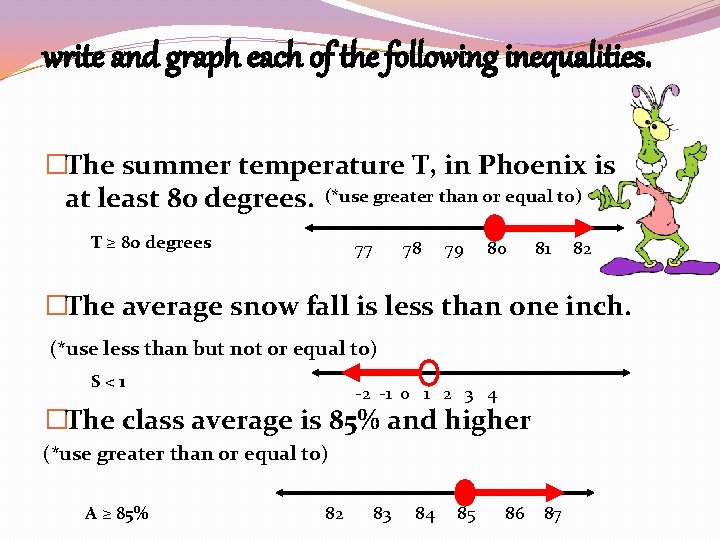
write and graph each of the following inequalities. �The summer temperature T, in Phoenix is at least 80 degrees. (*use greater than or equal to) T ≥ 80 degrees 77 78 79 80 81 82 �The average snow fall is less than one inch. (*use less than but not or equal to) S<1 -2 -1 0 1 2 3 4 �The class average is 85% and higher (*use greater than or equal to) A ≥ 85% 82 83 84 85 86 87

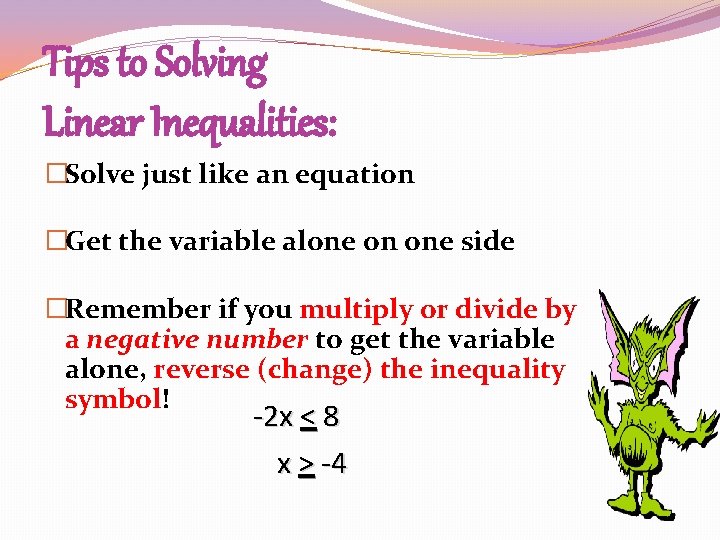
Tips to Solving Linear Inequalities: �Solve just like an equation �Get the variable alone on one side �Remember if you multiply or divide by a negative number to get the variable alone, reverse (change) the inequality symbol! -2 x < 8 x > -4

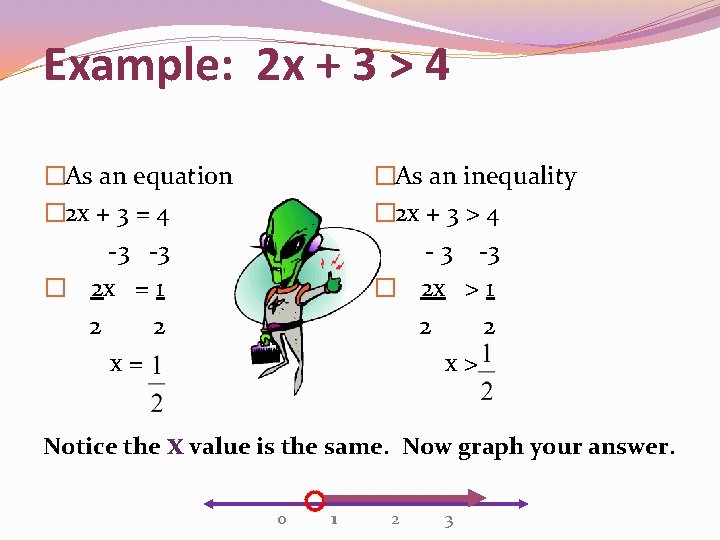
Example: 2 x + 3 > 4 �As an equation � 2 x + 3 = 4 -3 -3 � 2 x = 1 2 2 x= �As an inequality � 2 x + 3 > 4 - 3 -3 � 2 x > 1 2 2 x> Notice the x value is the same. Now graph your answer. 0 1 2 3

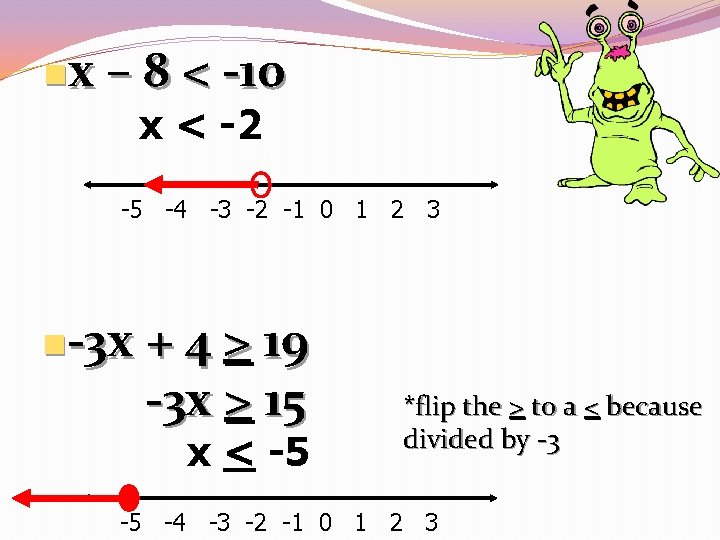
nx – 8 < -10 x < -2 -5 -4 -3 -2 -1 0 1 2 3 n-3 x + 4 > 19 -3 x > 15 x < -5 *flip the > to a < because divided by -3 -5 -4 -3 -2 -1 0 1 2 3

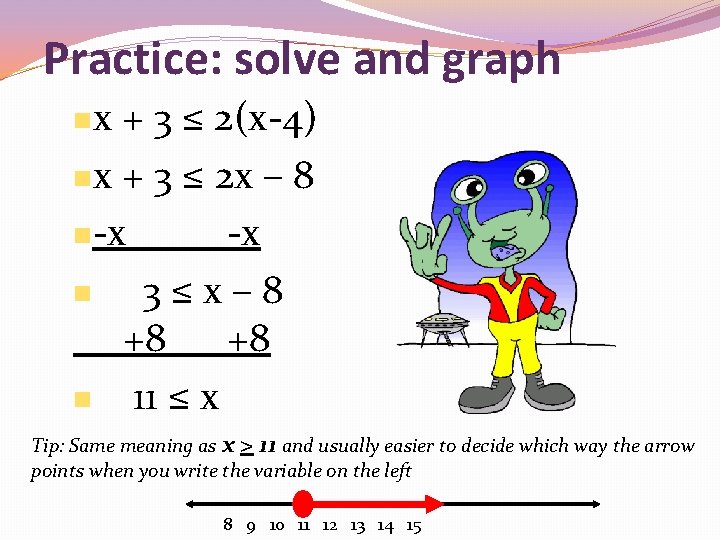
Practice: solve and graph nx + 3 ≤ 2(x-4) nx + 3 ≤ 2 x – 8 n-x -x n 3≤x– 8 +8 +8 n 11 ≤ x Tip: Same meaning as x > 11 and usually easier to decide which way the arrow points when you write the variable on the left 8 9 10 11 12 13 14 15

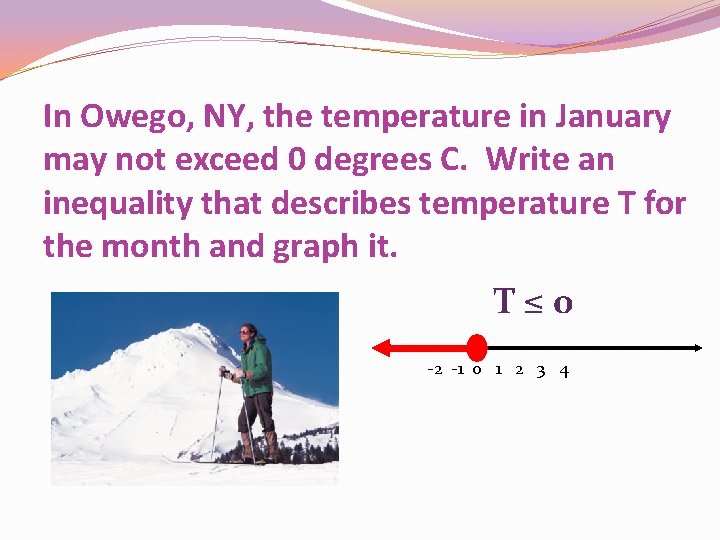
In Owego, NY, the temperature in January may not exceed 0 degrees C. Write an inequality that describes temperature T for the month and graph it. T≤ 0 -2 -1 0 1 2 3 4

Problem Solving With Inequalities You have $32. 14 in your pocket that you want to spend on a new pair of shoes. If sales tax is 6%, what’s the most your shoes can cost before tax? • Label what you know: • You have = $32. 14 • Sales tax =. 06 • “x” cost of shoes • Write an inequality. • x + x(. 06) ≤ 32. 14 • Solve • 1. 06 x ≤ 32. 14 • x ≤ 30. 32 • Answer the question: • The shoes can cost up to $30. 32 and you would have enough money to pay for them.

The last problem to solve together: You need some new CD’s to copy your photos on to send to your grandma in St. Louis. You found a great deal where you can get CD’s for $3. 00 each plus a flat shipping fee of $2. 00. If you have $25. 00 to spend, how many CD’s can you order? • Label what you know: • CDs= $3 • Shipping = $2 • You have = $25 • “x” number of CDs • Write an inequality. • 3 x + 2 ≤ 25 • Solve • 3 x ≤ 23 • x ≤ 7 2/ 3 • Answer the question: • You can order up to 7 CDs and have enough money to pay for them.