Solving Equations with 10 2 Variables on Both

Solving Equations with 10 -2 Variables on Both Sides Additional Example 1: Using Inverse Operations to Group Terms with Variables Group the terms with variables on one side of the equal sign, and simplify. A. 60 – 4 y = 8 y 60 – 4 y + 4 y = 8 y + 4 y 60 = 12 y Add 4 y to both sides. Simplify. B. – 5 b + 72 = – 2 b + 5 b 72 = 3 b Add 5 b to both sides. Simplify.

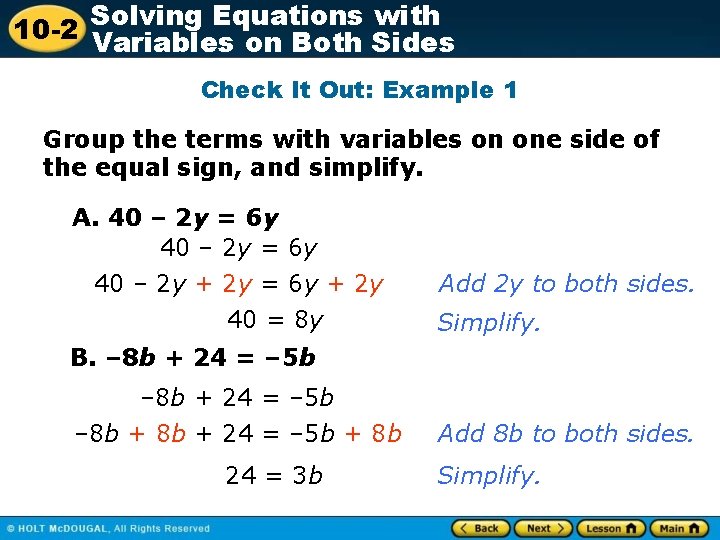
Solving Equations with 10 -2 Variables on Both Sides Check It Out: Example 1 Group the terms with variables on one side of the equal sign, and simplify. A. 40 – 2 y = 6 y 40 – 2 y + 2 y = 6 y + 2 y 40 = 8 y Add 2 y to both sides. Simplify. B. – 8 b + 24 = – 5 b + 8 b 24 = 3 b Add 8 b to both sides. Simplify.

Solving Equations with 10 -2 Variables on Both Sides Additional Example 2 A: Solving Equations with Variables on Both Sides Solve. 7 c = 2 c + 55 7 c – 2 c = 2 c – 2 c + 55 Subtract 2 c from both sides. 5 c = 55 Simplify. 5 c = 55 5 5 c = 11 Divide both sides by 5.

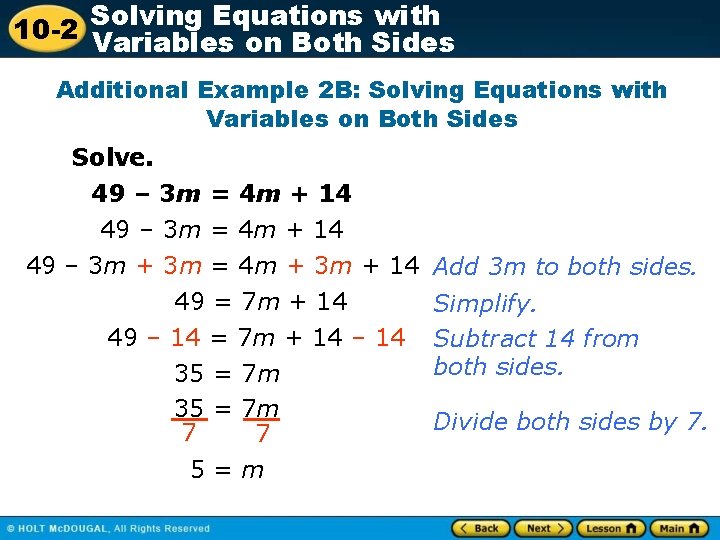
Solving Equations with 10 -2 Variables on Both Sides Additional Example 2 B: Solving Equations with Variables on Both Sides Solve. 49 – 3 m = 4 m + 14 49 – 3 m + 3 m = 4 m + 3 m + 14 49 = 7 m + 14 49 – 14 = 7 m + 14 – 14 35 = 7 m 7 7 5=m Add 3 m to both sides. Simplify. Subtract 14 from both sides. Divide both sides by 7.

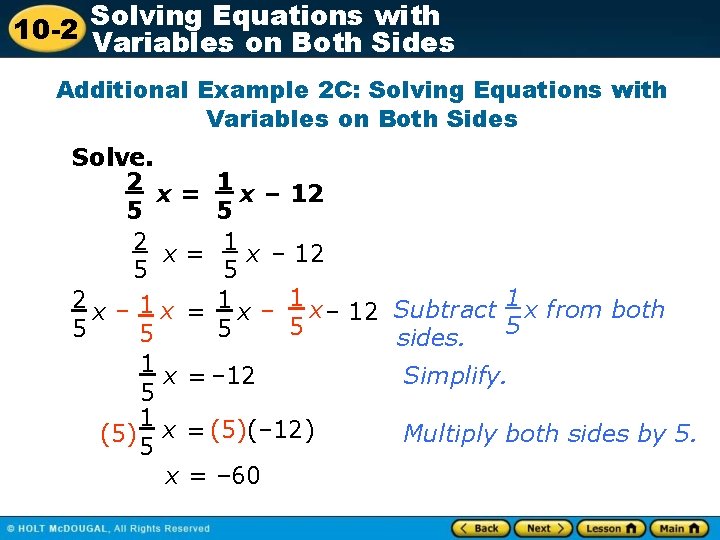
Solving Equations with 10 -2 Variables on Both Sides Additional Example 2 C: Solving Equations with Variables on Both Sides Solve. 2 x = 1 x – 12 5 5 1 2 – 1 x = 1 – 1 x Subtract x from both x x – 12 5 5 5 sides. 1 x – 12 Simplify. = 5 1 x (5)(– 12) = (5) Multiply both sides by 5. 5 x = – 60

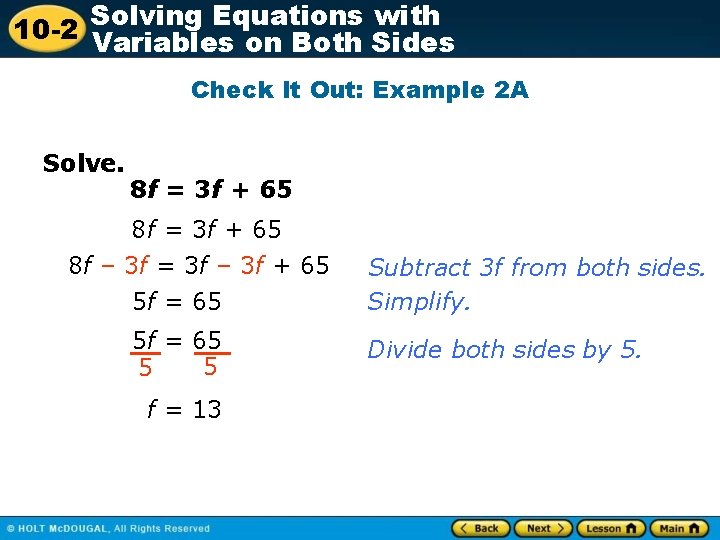
Solving Equations with 10 -2 Variables on Both Sides Check It Out: Example 2 A Solve. 8 f = 3 f + 65 8 f – 3 f = 3 f – 3 f + 65 5 f = 65 5 5 f = 13 Subtract 3 f from both sides. Simplify. Divide both sides by 5.

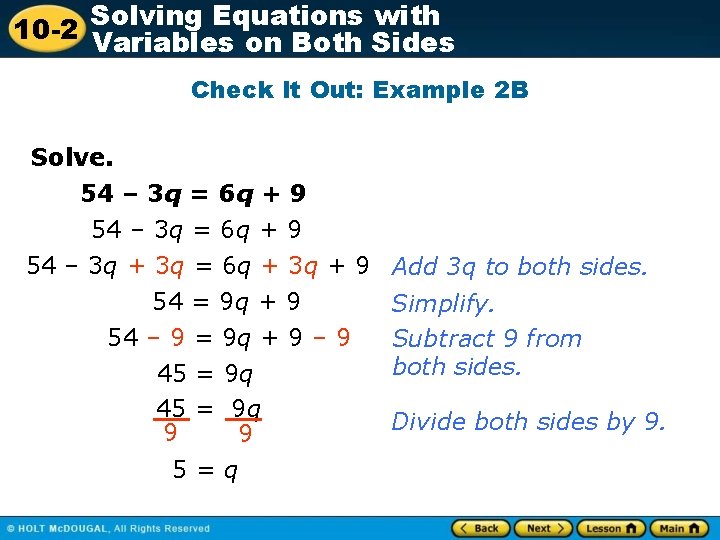
Solving Equations with 10 -2 Variables on Both Sides Check It Out: Example 2 B Solve. 54 – 3 q = 6 q + 9 54 – 3 q + 3 q = 6 q + 3 q + 9 54 = 9 q + 9 54 – 9 = 9 q + 9 – 9 45 = 9 q 9 9 5=q Add 3 q to both sides. Simplify. Subtract 9 from both sides. Divide both sides by 9.

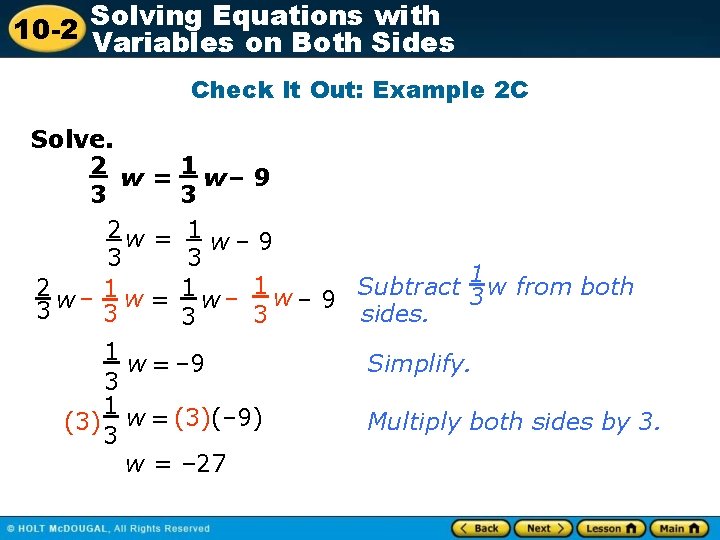
Solving Equations with 10 -2 Variables on Both Sides Check It Out: Example 2 C Solve. 2 w= 3 2 w = 3 2 – 1 w= 3 w 3 1 w– 9 3 1 1 Subtract w from both 1 – w 3 w – 9 sides. 3 3 1 w = – 9 3 1 (3) w = (3)(– 9) 3 w = – 27 Simplify. Multiply both sides by 3.

Solving Equations with 10 -2 Variables on Both Sides Additional Example 3: Consumer Math Application Christine can buy a new snowboard for $136. 50. She will still need to rent boots for $8. 50 a day. She can rent a snowboard and boots for $18. 25 a day. How many days would Christine need to rent both the snowboard and the boots to pay as much as she would if she buys the snowboard and rents only the boots for the season?

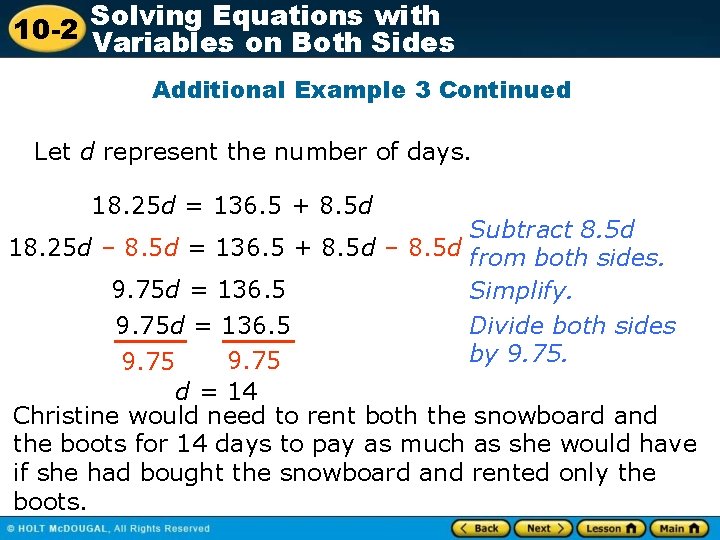
Solving Equations with 10 -2 Variables on Both Sides Additional Example 3 Continued Let d represent the number of days. 18. 25 d = 136. 5 + 8. 5 d Subtract 8. 5 d 18. 25 d – 8. 5 d = 136. 5 + 8. 5 d – 8. 5 d from both sides. 9. 75 d = 136. 5 Simplify. 9. 75 d = 136. 5 Divide both sides by 9. 75 d = 14 Christine would need to rent both the snowboard and the boots for 14 days to pay as much as she would have if she had bought the snowboard and rented only the boots.

Solving Equations with 10 -2 Variables on Both Sides Check It Out: Example 3 A local telephone company charges $40 per month for services plus a fee of $0. 10 a minute for long distance calls. Another company charges $75. 00 a month for unlimited service. How many minutes does it take for a person who subscribes to the first plan to pay as much as a person who subscribes to the unlimited plan?

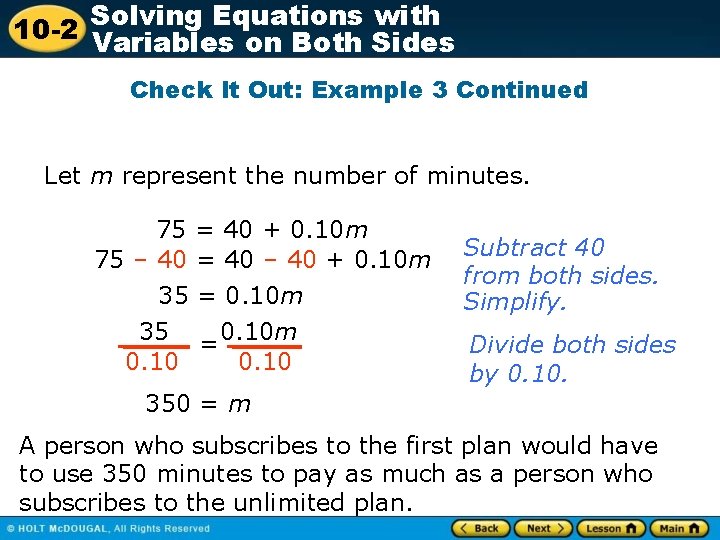
Solving Equations with 10 -2 Variables on Both Sides Check It Out: Example 3 Continued Let m represent the number of minutes. 75 = 40 + 0. 10 m 75 – 40 = 40 – 40 + 0. 10 m 35 = 0. 10 m 0. 10 Subtract 40 from both sides. Simplify. Divide both sides by 0. 10. 350 = m A person who subscribes to the first plan would have to use 350 minutes to pay as much as a person who subscribes to the unlimited plan.