Solve the following system using the substitution method

Solve the following system using the substitution method. x = 4 y + 1 3 x – 2 y = 13 Notice that the first equation is solve for x. Replace the x with (4 y + 1) in the 2 nd equation. 3(4 y + 1) – 2 y = 13 12 y + 3 – 2 y = 13 Solve the equation for y. → 10 y + 3 = 13 → 10 y = 10 → y = 1 Replace the y with 1 in either equation from the system. x = 4 y + 1 → x = 4(1) + 1 → x = 5 The solution to the system is ( 5 , 1 ). x = 5 and y = 1

10. 03 Solving Systems of Equations by Addition

Another method that can be used to solve a system of equations is called the addition method. To use the addition method, both equations must be in standard form with either the x or y terms being opposites. Remember that opposite terms have the same numbers and variables, but different signs. 5 x and – 5 x are opposites. – 3 y and 3 y are opposites.

To solve a system where both equations are in standard form and at least one set of the variable terms are opposites, add the two equations together. The two opposite variable terms will cancel leaving an equation with only one variable. Solve that equation. Use the value of that variable ( x or y ) to find the value of the other variable. To do this, replace the variable with the solved value in either equation of the system and solve for the remaining variable.

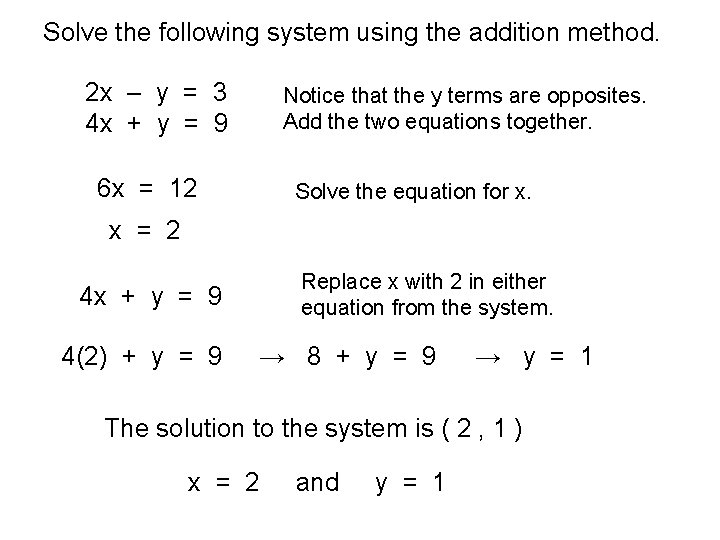
Solve the following system using the addition method. 2 x – y = 3 4 x + y = 9 Notice that the y terms are opposites. Add the two equations together. 6 x = 12 Solve the equation for x. x = 2 Replace x with 2 in either equation from the system. 4 x + y = 9 4(2) + y = 9 → 8 + y = 9 → y = 1 The solution to the system is ( 2 , 1 ) x = 2 and y = 1

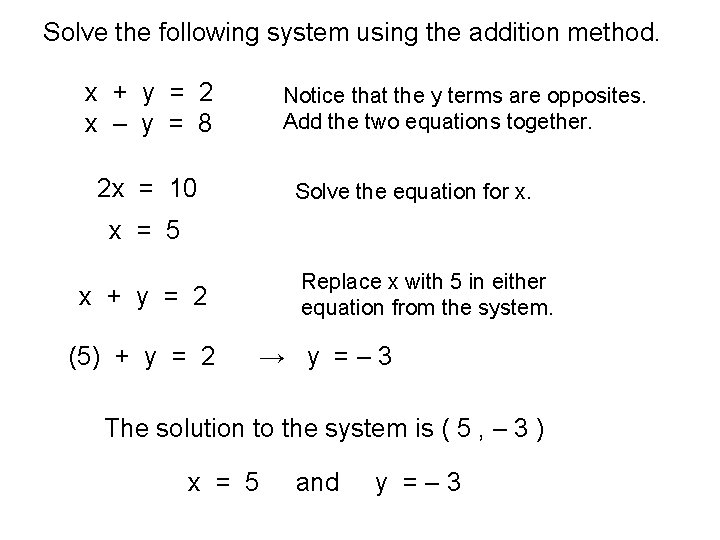
Solve the following system using the addition method. x + y = 2 x – y = 8 Notice that the y terms are opposites. Add the two equations together. 2 x = 10 Solve the equation for x. x = 5 Replace x with 5 in either equation from the system. x + y = 2 (5) + y = 2 → y =– 3 The solution to the system is ( 5 , – 3 ) x = 5 and y =– 3

Solve the following system using the addition method. – 4 x + y = 7 4 x + 3 y = 5 Notice that the x terms are opposites. Add the two equations together. 4 y = 12 y = 3 Solve the equation for y. Replace y with 3 in either equation from the system. 4 x + 3 y = 5 4 x + 3(3) = 5 → 4 x + 9 = 5 → 4 x = – 4 → x = – 1 The solution to the system is ( – 1 , 3 ) x =– 1 and y = 3

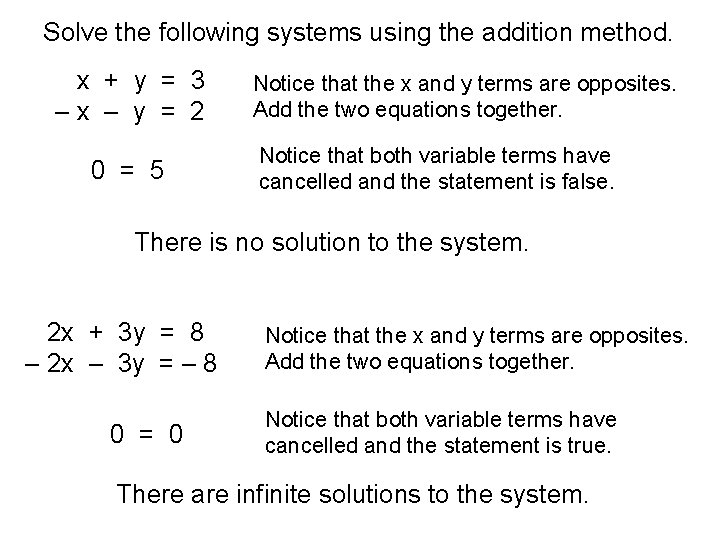
Solve the following systems using the addition method. x + y = 3 –x – y = 2 0 = 5 Notice that the x and y terms are opposites. Add the two equations together. Notice that both variable terms have cancelled and the statement is false. There is no solution to the system. 2 x + 3 y = 8 – 2 x – 3 y = – 8 0 = 0 Notice that the x and y terms are opposites. Add the two equations together. Notice that both variable terms have cancelled and the statement is true. There are infinite solutions to the system.

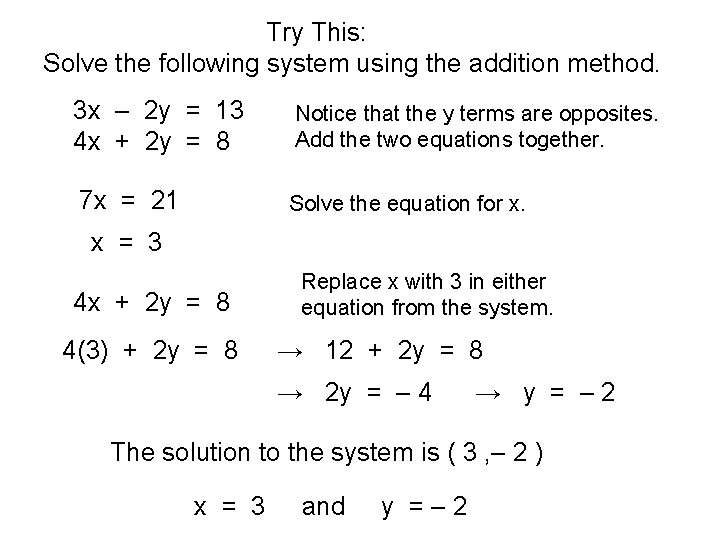
Try This: Solve the following system using the addition method. 3 x – 2 y = 13 4 x + 2 y = 8 Notice that the y terms are opposites. Add the two equations together. 7 x = 21 Solve the equation for x. x = 3 4 x + 2 y = 8 4(3) + 2 y = 8 Replace x with 3 in either equation from the system. → 12 + 2 y = 8 → 2 y = – 4 → y = – 2 The solution to the system is ( 3 , – 2 ) x = 3 and y =– 2