Solve Quadratics by Completing the Square Quadratic Formula

Solve Quadratics by Completing the Square & Quadratic Formula Skill 58

Objective HSA-REI. 4. a: Solve quadratic equations by completing the square and by using the quadratic formula.

Many quadratic equations contain expressions that cannot be easily factored. For equations containing these types of expressions, you can use square roots to find roots.

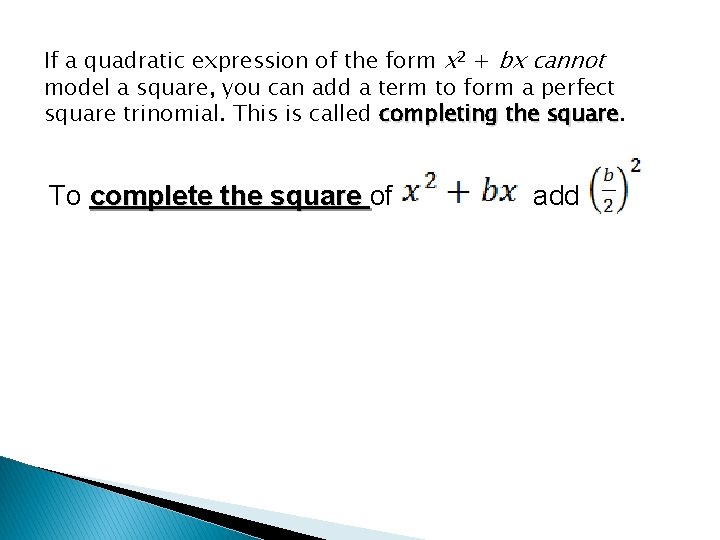
If a quadratic expression of the form x 2 + bx cannot model a square, you can add a term to form a perfect square trinomial. This is called completing the square To complete the square of add

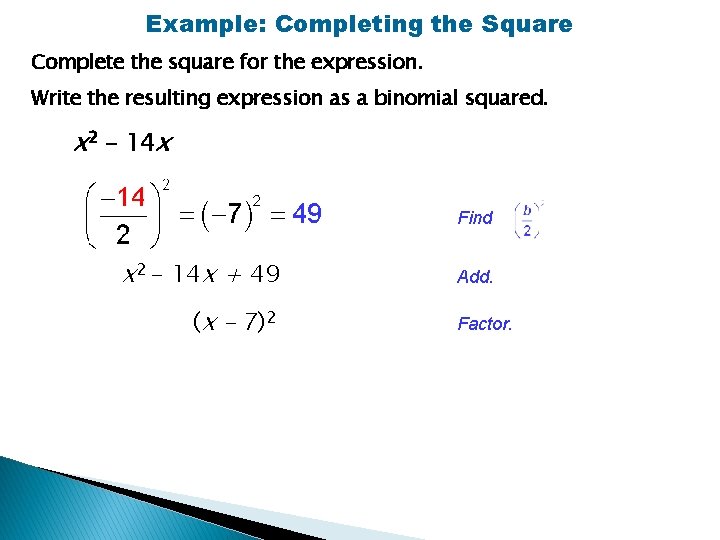
Example: Completing the Square Complete the square for the expression. Write the resulting expression as a binomial squared. x 2 – 14 x Find x 2 – 14 x + 49 (x – 7)2 Add. Factor. .

Do It Yourself: Example Complete the square for the expression. Write the resulting expression as a binomial squared. x 2 + 4 x Find x 2 + 4 x + 4 (x + 2)2 Add. Factor. .

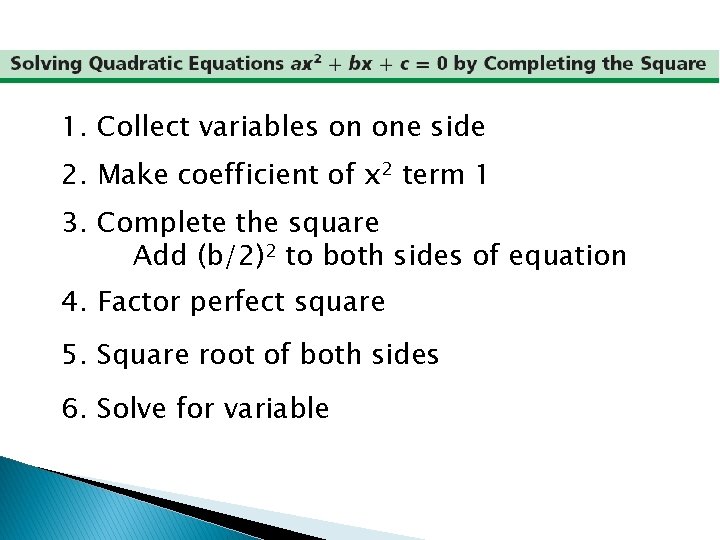
1. Collect variables on one side 2. Make coefficient of x 2 term 1 3. Complete the square Add (b/2)2 to both sides of equation 4. Factor perfect square 5. Square root of both sides 6. Solve for variable

Example: Solving a Quadratic Equation by Completing the Square Solve the equation by completing the square. x 2 = 12 x – 20 x 2 – 12 x = – 20 Collect variable terms on one side. x 2 – 12 x + Set up to complete the square. = – 20 + Add x 2 – 12 x + 36 = – 20 + 36 to both sides. Simplify.

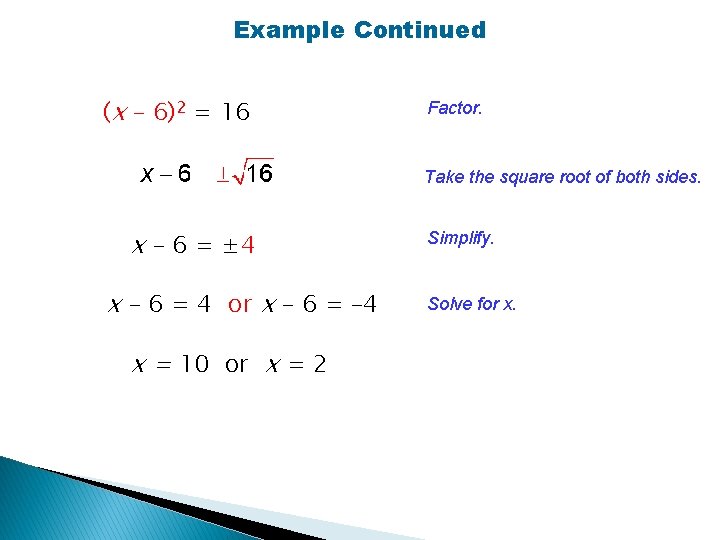
Example Continued (x – 6)2 = 16 Factor. Take the square root of both sides. x – 6 = ± 4 x – 6 = 4 or x – 6 = – 4 x = 10 or x = 2 Simplify. Solve for x.

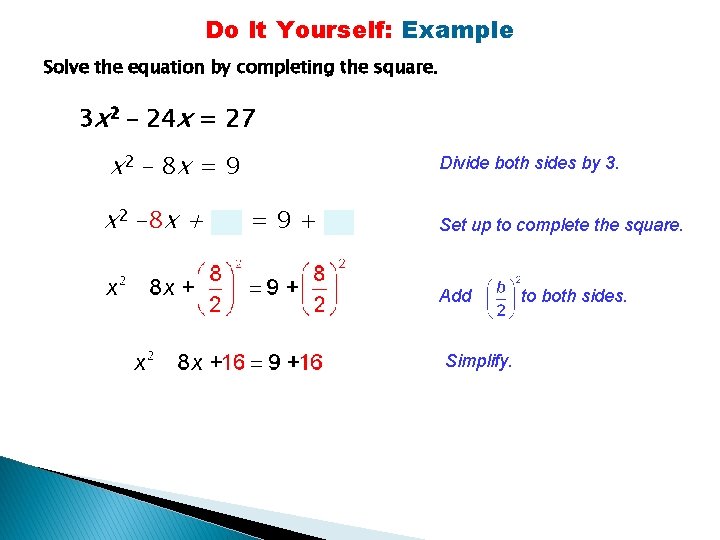
Do It Yourself: Example Solve the equation by completing the square. 3 x 2 – 24 x = 27 x 2 – 8 x = 9 x 2 – 8 x + Divide both sides by 3. =9+ Set up to complete the square. Add Simplify. to both sides.

Do It Yourself: Example Continued Solve the equation by completing the square. Factor. Take the square root of both sides. Simplify. x – 4 =– 5 or x – 4 = 5 x =– 1 or x = 9 Solve for x.

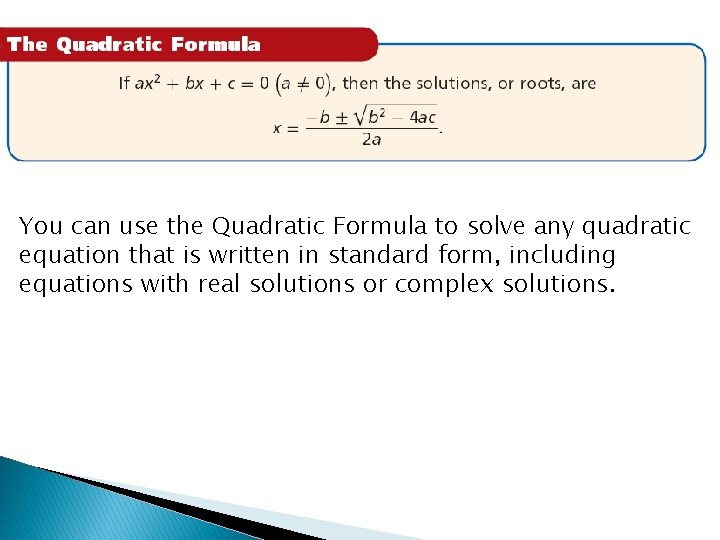
You can use the Quadratic Formula to solve any quadratic equation that is written in standard form, including equations with real solutions or complex solutions.

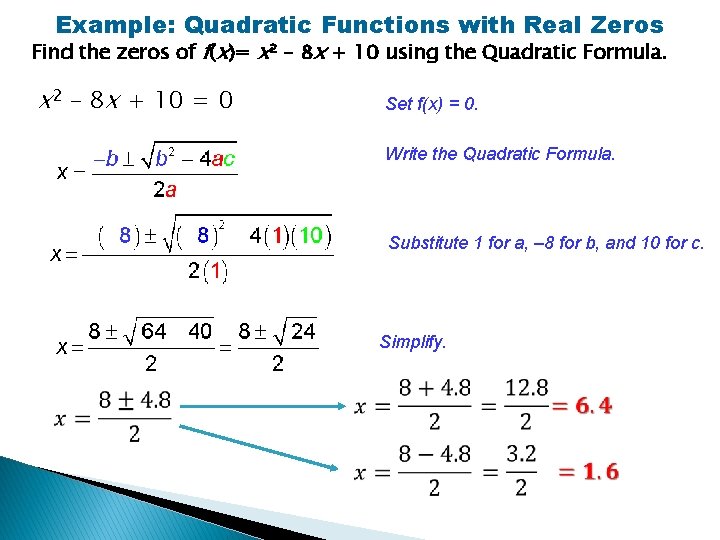
Example: Quadratic Functions with Real Zeros Find the zeros of f(x)= x 2 – 8 x + 10 using the Quadratic Formula. x 2 – 8 x + 10 = 0 Set f(x) = 0. Write the Quadratic Formula. Substitute 1 for a, – 8 for b, and 10 for c. Simplify.

Do It Yourself: Example Find the zeros of f(x)= 5 x 2 – 10 x + 5 using the Quadratic Formula. 5 x 2 – 15 x + 5 = 0 Set f(x) = 0. Write the Quadratic Formula.

58: Solving Quadratics by Completing the Square & Quadratic Formula Ø Questions Ø Summarize your notes Ø Homework Ø Google Classroom Ø Quiz