Simultaneous Equations Solving simultaneous equations by SUBSTITUTION ELIMINATION

Simultaneous Equations

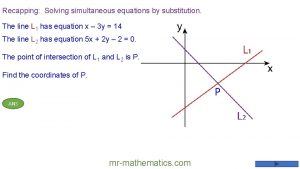
Solving simultaneous equations by SUBSTITUTION ELIMINATION There is another way of Substitution is ONLY used ELIMINATION is the solving simultaneous for solving Simultaneous main method for equations solving which does NOTthey involve Equations when are simultaneous equations drawing graphs or easily using given in or can be that you will use, but you Substitution!! rearranged into the form MUST be aware of and The second method is called y =? ? x =to? ? use But what knoworhow the about other equations not in. ELIMINATION other methods as well. this form?

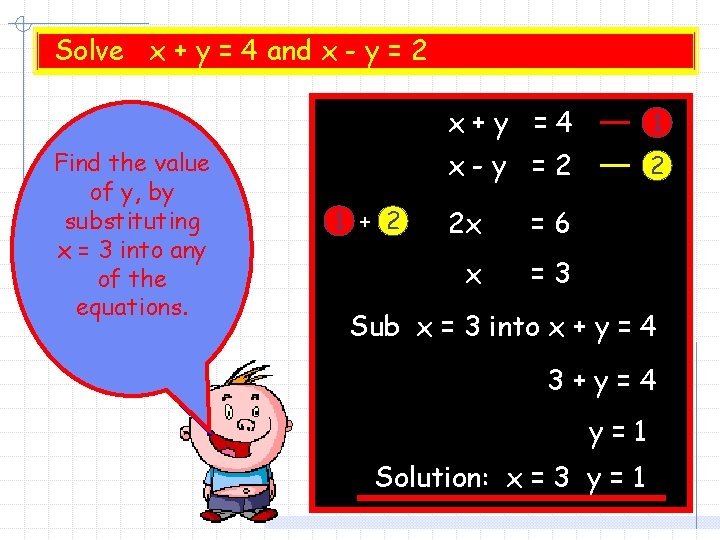
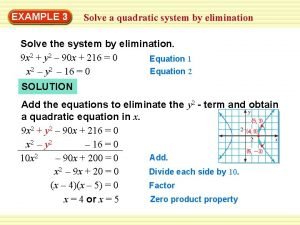
Solve x + y = 4 and x - y = 2 Find Label When the each the value Now solve equation signs ofthe in y, by front using substituting ofa the number term equation inside x = 3 tointo abe circle any using the eliminated like of the this: normal are equations. different werules!! ADD the equations!! 1 + 2 x+y =4 1 x-y =2 2 2 x =6 x =3 Sub x = 3 into x + y = 4 3+y=4 y=1 Solution: x = 3 y = 1

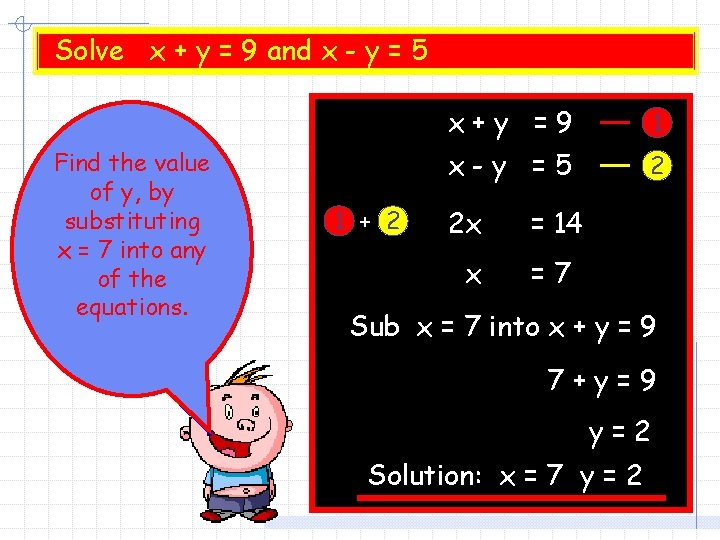
Solve x + y = 9 and x - y = 5 Find Label When the each the value Now solve equation signs ofthe in y, by front using substituting ofa the number term equation inside x = 7 tointo abe circle any using the eliminated like of the this: normal are equations. different werules!! ADD the equations!! 1 + 2 x+y =9 1 x-y =5 2 2 x x = 14 =7 Sub x = 7 into x + y = 9 7+y=9 y=2 Solution: x = 7 y = 2

Solve x + y = 7 and 3 x - y = 3 Find Label When the each the value Now solve equation signs ofthe in y, by front using substituting ofa the number term equation inside x = 3 tointo abe circle any using the eliminated like of the this: normal are equations. different werules!! ADD the equations!! 1 + 2 x+y =7 1 3 x - y = 5 2 4 x x = 12 =3 Sub x = 3 into x + y = 7 3+y=7 y=4 Solution: x = 3 y = 4

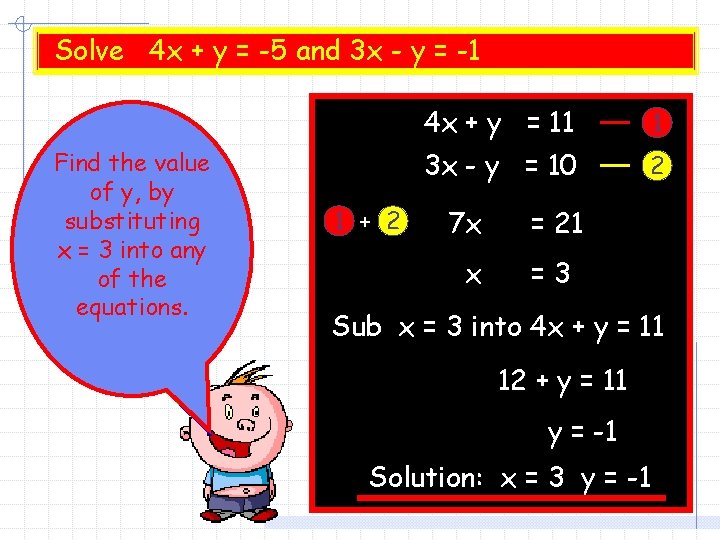
Solve 4 x + y = -5 and 3 x - y = -1 Find Label When the each the value Now solve equation signs ofthe in y, by front using substituting ofa the number term equation inside x = 3 tointo abe circle any using the eliminated like of the this: normal are equations. different werules!! ADD the equations!! 1 + 2 4 x + y = 11 1 3 x - y = 10 2 7 x x = 21 =3 Sub x = 3 into 4 x + y = 11 12 + y = 11 y = -1 Solution: x = 3 y = -1

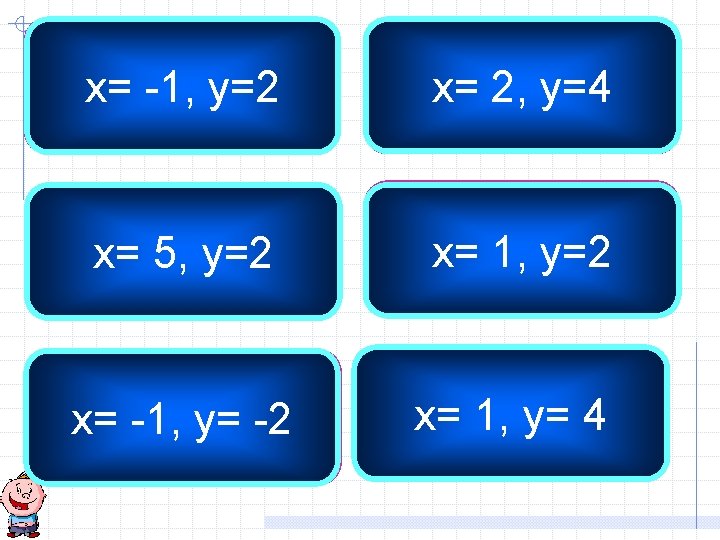
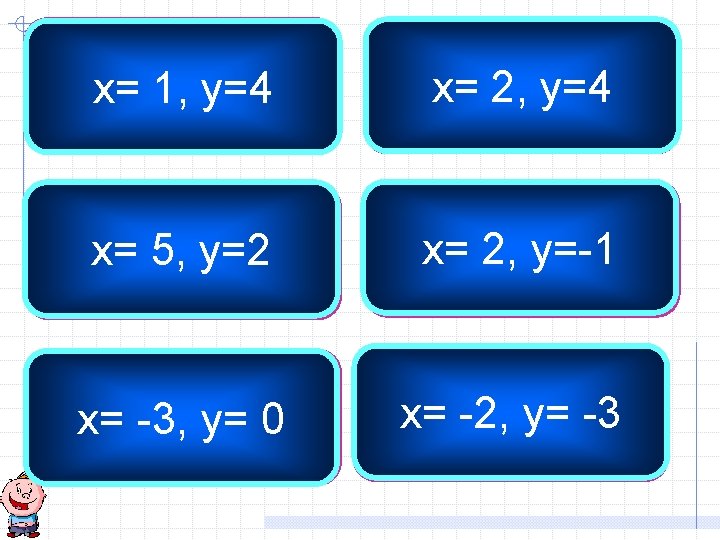
10 x + y = -8 x= -1, y=2 2 x - y = -4 -x + y = 2 x= 2, y=4 2 x - y = 0 2 x + 2 y = 14 x= 5, y=2 3 x - 2 y = 11 x + 5 y = 11 x= 1, y=2 2 x - 5 y = -8 2 x + y = 6 3 x + 2 y = -7 x= 1, y= 4 x= -1, y= -2 -3 x + 8 y =-13 -2 x - 5 y = -22

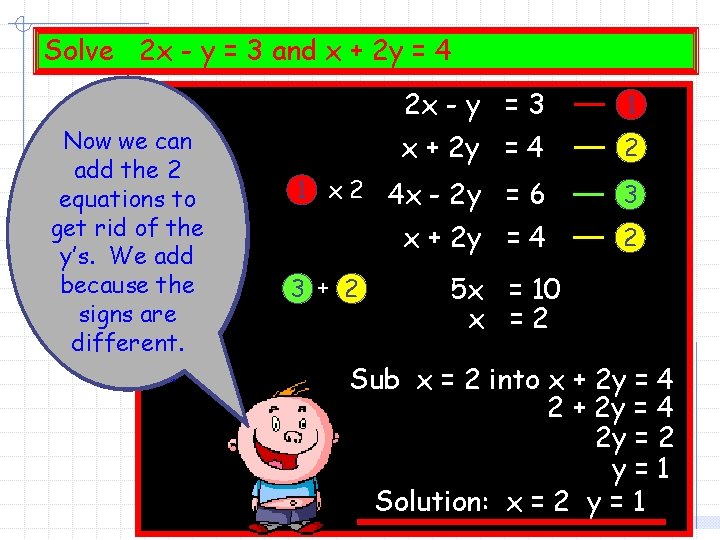
Solve 2 x - y = 3 and x + 2 y = 4 Now we can We need to add theone 2 multiply equations or both to get rid of the equations so y’s. that. We theadd y because the values are signs arein the same different. each equation! 2 x - y = 3 1 x + 2 y = 4 2 11 +-x 22 4 x 3 x y ==-1 76 x --+3 y 2 y 3 x + 2 y = 4 2 3 + 2 5 x = 10 x =2 Sub x = 2 into x + 2 y = 4 2 y = 2 y=1 Solution: x = 2 y = 1

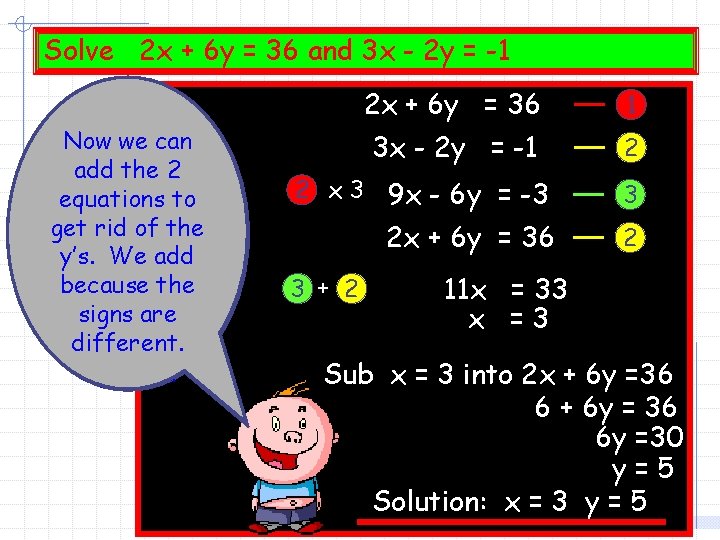
Solve 2 x + 6 y = 36 and 3 x - 2 y = -1 Now we can We need to add theone 2 multiply equations or both to get rid of the equations so y’s. that. We theadd y because the values are signs arein the same different. each equation! 2 x + 6 y = 36 1 3 x - 2 y = -1 2 12 +-x 23 9 x 3 x y == -1 7 -3 x --+3 y 6 y 3 2 x + 6 y = 36 2 3 + 2 11 x = 33 x =3 Sub x = 3 into 2 x + 6 y =36 6 + 6 y = 36 6 y =30 y=5 Solution: x = 3 y = 5

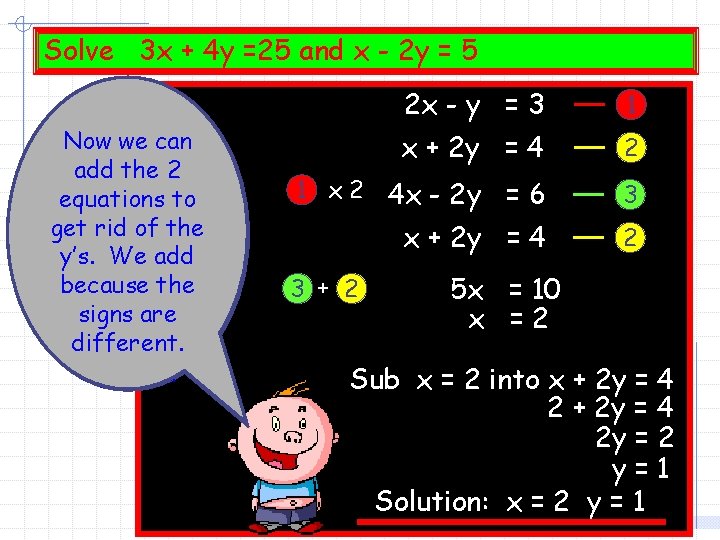
Solve 3 x + 4 y =25 and x - 2 y = 5 Now we can We need to add theone 2 multiply equations or both to get rid of the equations so y’s. that. We theadd y because the values are signs arein the same different. each equation! 2 x - y = 3 1 x + 2 y = 4 2 11 +-x 22 4 x 3 x y ==-1 76 x --+3 y 2 y 3 x + 2 y = 4 2 3 + 2 5 x = 10 x =2 Sub x = 2 into x + 2 y = 4 2 y = 2 y=1 Solution: x = 2 y = 1

3 x + 2 y = 11 x= 1, y=4 2 x - y = -2 3 x - 2 y = 11 x= 5, y=2 7 x -8 y = 51 3 x + 4 y = 22 x= 2, y=4 8 x - 2 y = 8 x - y=3 x= 2, y=-1 3 x + 5 y = 1 + 2 y = -12 9 x + 4 y = -27 3 x x= -2, y= -3 x= -3, y= 0 -x + y = -1 -3 x + 8 y = 9

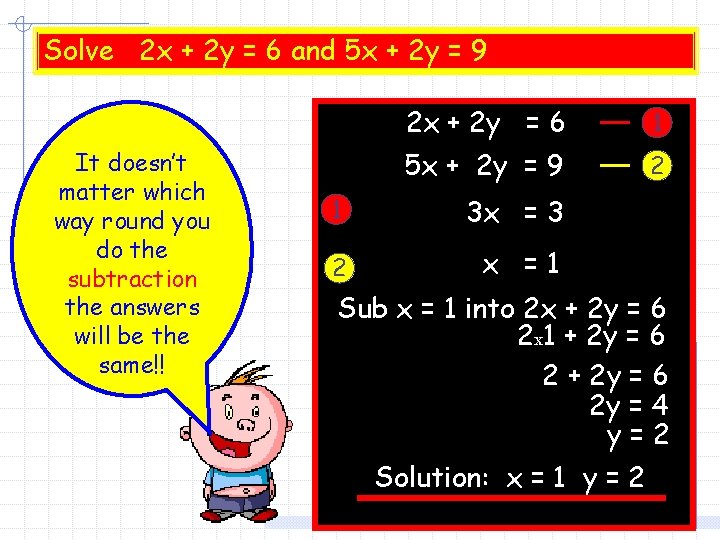
Solve 2 x + 2 y = 6 and 5 x + 2 y = 9 It doesn’t matter which way round you do the subtraction the answers will be the same!! 2 x + 2 y = 6 1 5 x + 2 y = 9 2 1 3 x = 3 2 x =1 Sub x = 1 into 2 x + 2 y = 6 2 x 1 + 2 y = 6 2 y = 4 y=2 Solution: x = 1 y = 2

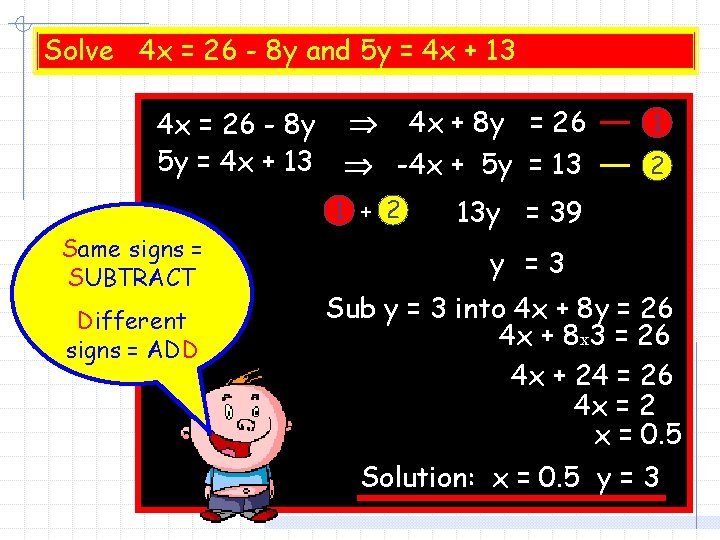
Solve 4 x = 26 - 8 y and 5 y = 4 x + 13 4 x = 26 - 8 y 5 y = 4 x + 13 4 x + 8 y = 26 1 -4 x + 5 y = 13 2 1 + 2 Same signs = SUBTRACT Different signs = ADD 13 y = 39 y =3 Sub y = 3 into 4 x + 8 y = 26 4 x + 8 x 3 = 26 4 x + 24 = 26 4 x = 2 x = 0. 5 Solution: x = 0. 5 y = 3

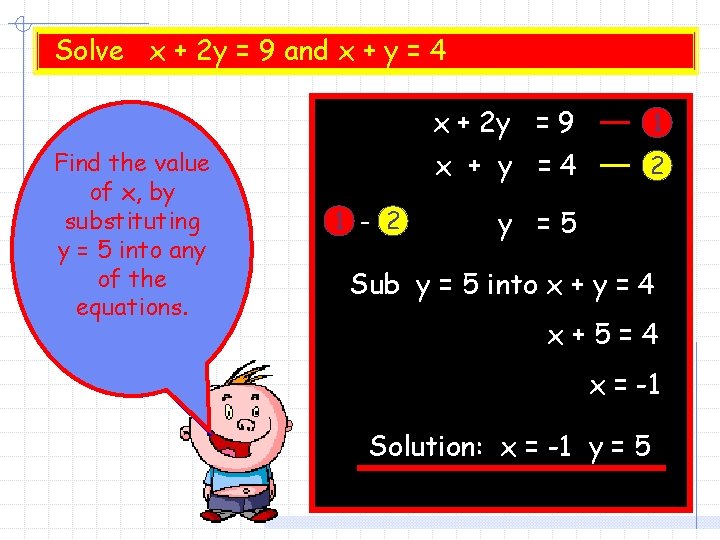
Solve x + 2 y = 9 and x + y = 4 Find Label When theeach the value equation signs of in x, front by using substituting ofa the number term inside y = 5 tointo abe circle any eliminated like of the this: are equations. the same we SUBTRACT 1 - 2 x + 2 y = 9 1 x + y =4 2 y =5 Sub y = 5 into x + y = 4 x+5=4 x = -1 Solution: x = -1 y = 5

Solving HARDER simultaneous equations by by. ELIMINATION We. However have now seen three in allallthe methods forproblems solving Elimination Simultaneous Equations: seen so far it has been DRAWING fairly easy to eliminate one term. What about SUBSTITUTION equations were a term is ELIMINATION not readily eliminated? .

Solve 2 x + 3 y = 8 and -x + 5 y = 22 You don’t Now we always need to can ADD eliminate y. In the two it this example is easier to equations eliminate x! to eliminate x. 2 x 2 2 x + 3 y = 8 -x + 5 y = 22 2 2 x + 3 y = 8 1 -2 x + 10 y = 44 3 1 13 y = 52 y =4 Sub y = 4 into 2 x + 3 y = 8 2 x + 3 x 4 = 8 2 x + 12 = 8 2 x = -4 x = -2 1 + 3 Solution: x = -2 y = 4

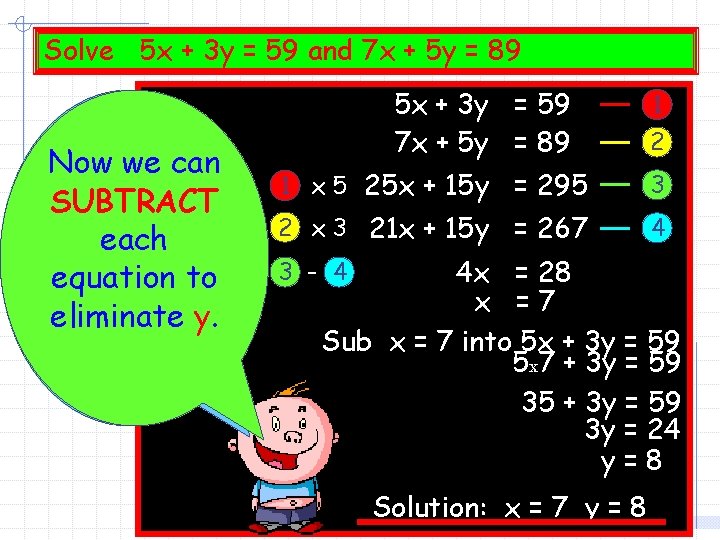
Solve 5 x + 3 y = 59 and 7 x + 5 y = 89 In the Now welast can example we SUBTRACT only had to each multiply one of the equations. equation to Here we need eliminate to do both! y. 5 x + 3 y = 59 7 x + 5 y = 89 1 2 1 x 5 25 x + 15 y = 295 3 2 x 3 21 x + 15 y = 267 4 4 x = 28 x =7 Sub x = 7 into 5 x + 3 y = 59 5 x 7 + 3 y = 59 35 + 3 y = 59 3 y = 24 y=8 3 - 4 Solution: x = 7 y = 8

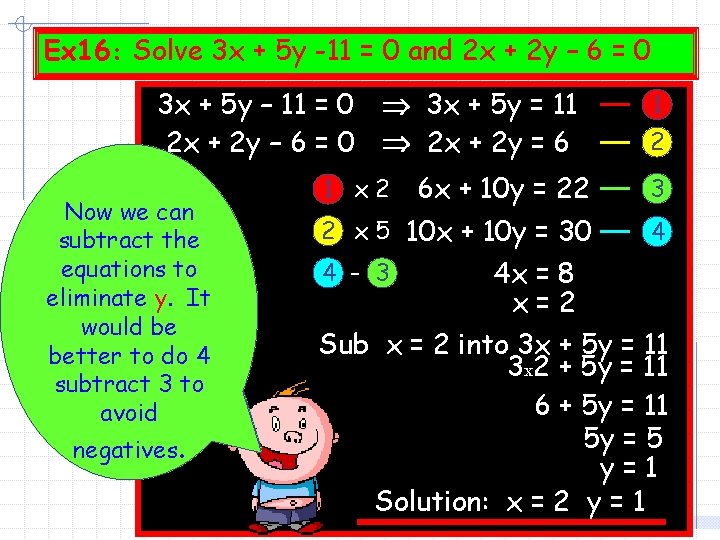
Ex 16: Solve 3 x + 5 y -11 = 0 and 2 x + 2 y – 6 = 0 3 x + 5 y – 11 = 0 3 x + 5 y = 11 2 x + 2 y – 6 = 0 2 x + 2 y = 6 Now weneed can We will subtract the to rearrange equations to the 2 eliminate y. It equations would be before better to do 4 trying 3 toto subtract avoid solve them!! negatives. 1 2 6 x + 10 y = 22 3 2 x 5 10 x + 10 y = 30 4 1 x 2 4 x = 8 x=2 Sub x = 2 into 3 x + 5 y = 11 3 x 2 + 5 y = 11 6 + 5 y = 11 5 y = 5 y=1 Solution: x = 2 y = 1 4 - 3

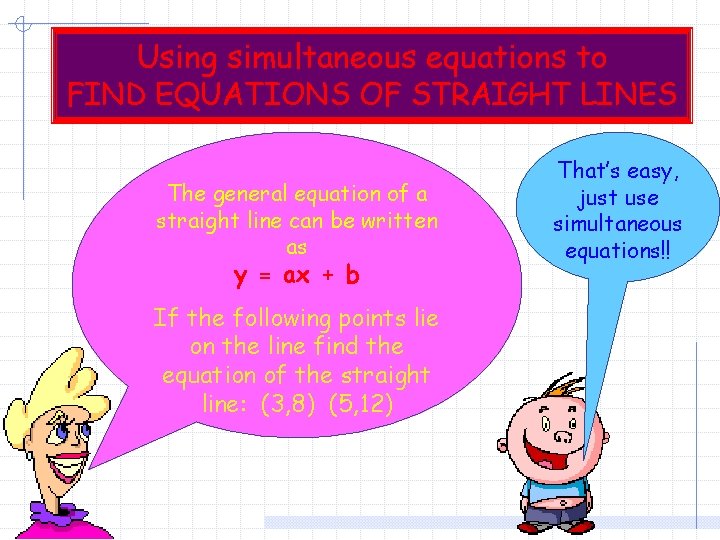
Using simultaneous equations to FIND EQUATIONS OF STRAIGHT LINES The general equation of a straight line can be written as y = ax + b If the following points lie on the line find the equation of the straight line: (3, 8) (5, 12) That’s easy, just use simultaneous equations!!

Told you it was easy!! Use each coordinate Now we to simply writesolve an them equation like any by other subing pair in xof for equations. x and y for y!!! (3, 8) : (5, 12): 2 - 1 ax + b = y 3 a + b = 8 5 a + b = 12 2 a = 4 a =2 Sub a = 2 into 3 a + b = 8 3 x 2+ b = 8 6+b=8 b=2 Equation: y = 2 x + 2 1 2

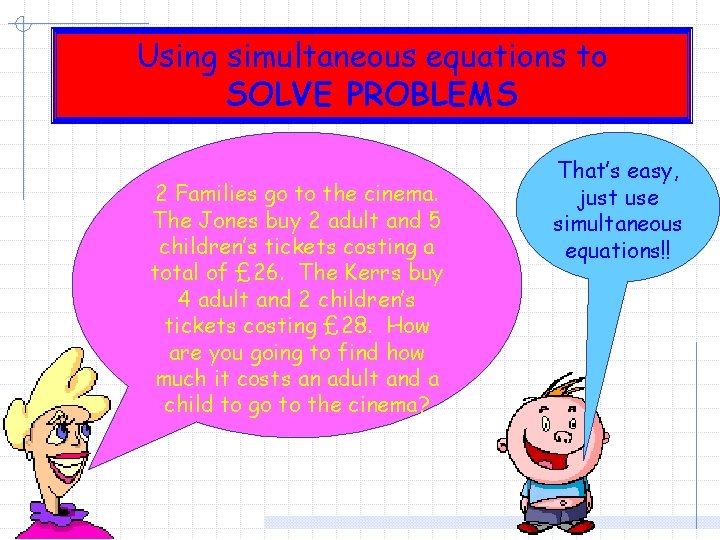
Using simultaneous equations to SOLVE PROBLEMS 2 Families go to the cinema. The Jones buy 2 adult and 5 children’s tickets costing a total of £ 26. The Kerrs buy 4 adult and 2 children’s tickets costing £ 28. How are you going to find how much it costs an adult and a child to go to the cinema? That’s easy, just use simultaneous equations!!

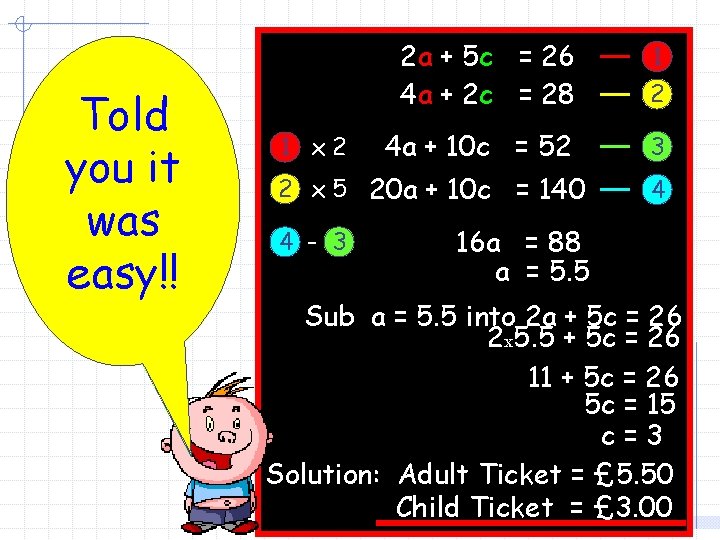
Told you it was easy!! Firstly introduce Now we LETTERS simply solve to them represent like any other each type pair of equations. ticket, i. e. a for Adult and c for Child 1 x 2 2 a + 5 c = 26 4 a + 2 c = 28 2 4 a + 10 c = 52 3 2 x 5 20 a + 10 c 4 - 3 = 140 1 4 16 a = 88 a = 5. 5 Sub a = 5. 5 into 2 a + 5 c = 26 2 x 5. 5 + 5 c = 26 11 + 5 c = 26 5 c = 15 c=3 Solution: Adult Ticket = £ 5. 50 Child Ticket = £ 3. 00

I can’t take any more equation solving. I think my head will explode if I see another x or y!!!!!!!

Okay smarty pants! What about this one. 2 alloys can be made by mixing Lead and Iron. The first alloy is made by mixing 3 cm 3 of Iron and 4 cm 3 of Lead. This alloy weighs 65 g. The second alloy is made from 5 cm 3 of Iron and 7 cm 3 of Lead and it weighs 112 g. Find how much 1 cm 3 of Lead and 1 cm 3 of Iron weigh. Again that’s easy, just use simultaneous equations!!

Now So Easy do Peasy!! Pg 132 Ex 10 Firstly introduce Now we LETTERS simply solve to them represent like any other each type pair of equations. metal, i. e. I for iron and L for lead 3 I + 4 L = 65 5 I + 7 L = 112 1 x 5 15 I + 20 L = 325 3 2 x 3 15 I + 21 L = 336 4 4 - 3 L = 11 Sub L = 11 into 3 I + 4 L = 65 3 I + 4 x 11 = 65 3 I + 44 = 65 3 I = 21 I=7 Solution: 1 cm 3 of Iron = 7 g 1 cm 3 of Lead = 11 g

Okay try this one. Tickets for a sports club disco cost £ 2 for members and £ 3 for non -members. The total money collected was £ 580. A total of 250 people attended the disco. Find the number of members and nonmembers that went to the disco. Wait Thata exercise minute was I didn’t easy! Got say impossible!! anything else?

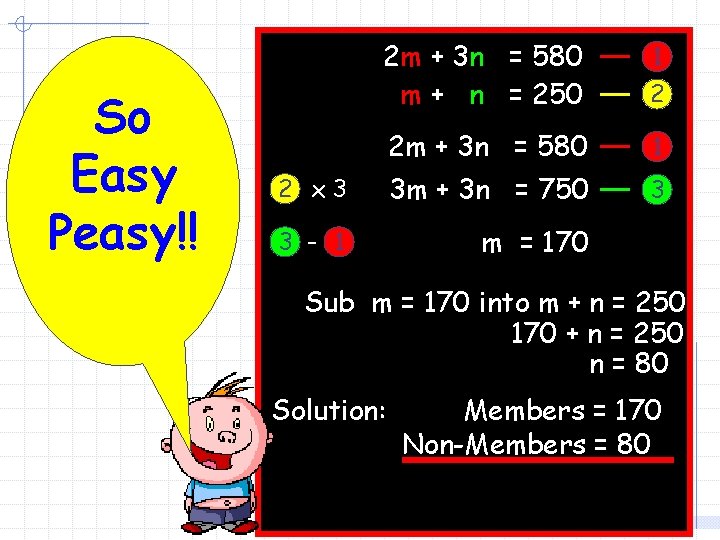
So Easy Peasy!! 2 m + 3 n = 580 m + n = 250 2 2 m + 3 n = 580 1 2 x 3 3 m + 3 n = 750 3 3 - 1 m = 170 1 Sub m = 170 into m + n = 250 170 + n = 250 n = 80 Solution: Members = 170 Non-Members = 80