Simplifying Square Root Expressions Numbers with a Root
Simplifying Square Root Expressions

Numbers with a Root Radical numbers are typically irrational numbers (unless they simplify to a rational number). Our calculator gives: But the decimal will go on forever and not repeat because it is an irrational number. For the exact answer just use: Some radicals can be simplified similar to simplifying a fraction.

Radical Product Property ONLY when a≥ 0 and b≥ 0 For Example: Equal
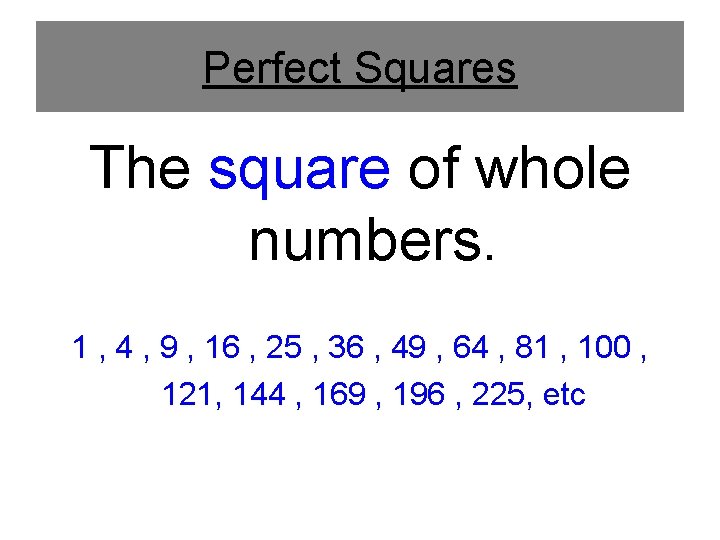
Perfect Squares The square of whole numbers. 1 , 4 , 9 , 16 , 25 , 36 , 49 , 64 , 81 , 100 , 121, 144 , 169 , 196 , 225, etc
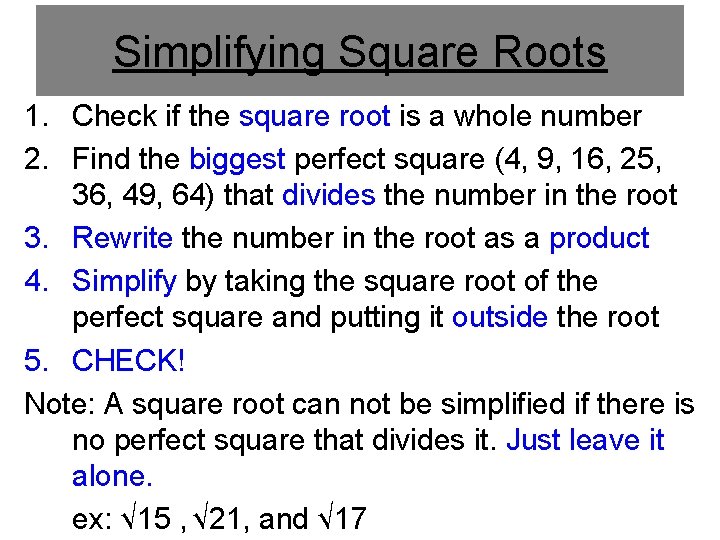
Simplifying Square Roots 1. Check if the square root is a whole number 2. Find the biggest perfect square (4, 9, 16, 25, 36, 49, 64) that divides the number in the root 3. Rewrite the number in the root as a product 4. Simplify by taking the square root of the perfect square and putting it outside the root 5. CHECK! Note: A square root can not be simplified if there is no perfect square that divides it. Just leave it alone. ex: √ 15 , √ 21, and √ 17
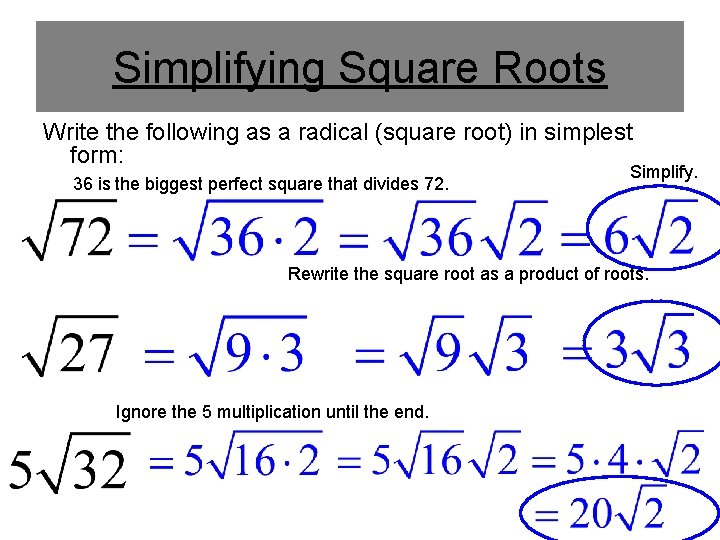
Simplifying Square Roots Write the following as a radical (square root) in simplest form: 36 is the biggest perfect square that divides 72. Simplify. Rewrite the square root as a product of roots. Ignore the 5 multiplication until the end.
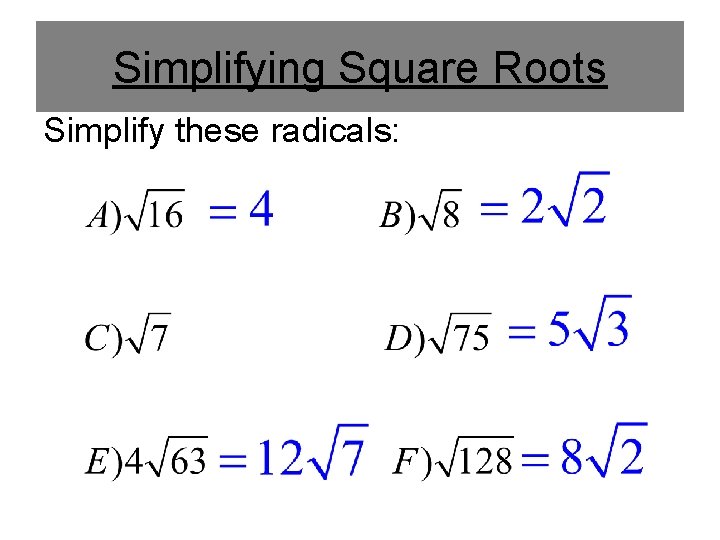
Simplifying Square Roots Simplify these radicals:
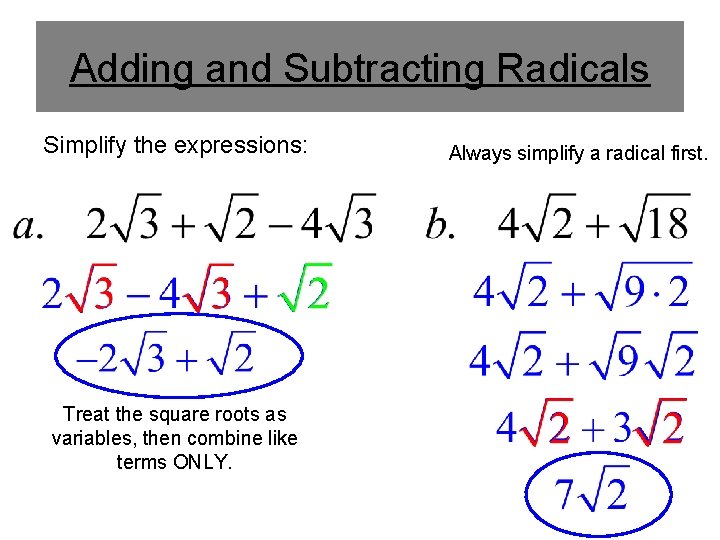
Adding and Subtracting Radicals Simplify the expressions: Treat the square roots as variables, then combine like terms ONLY. Always simplify a radical first.
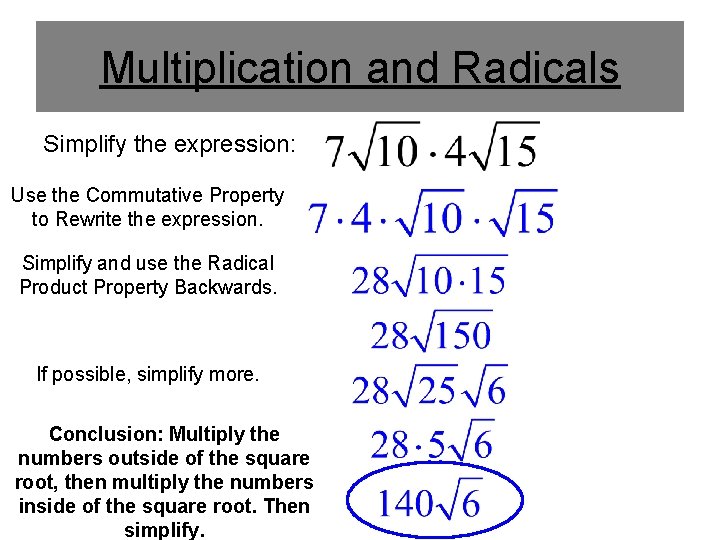
Multiplication and Radicals Simplify the expression: Use the Commutative Property to Rewrite the expression. Simplify and use the Radical Product Property Backwards. If possible, simplify more. Conclusion: Multiply the numbers outside of the square root, then multiply the numbers inside of the square root. Then simplify.
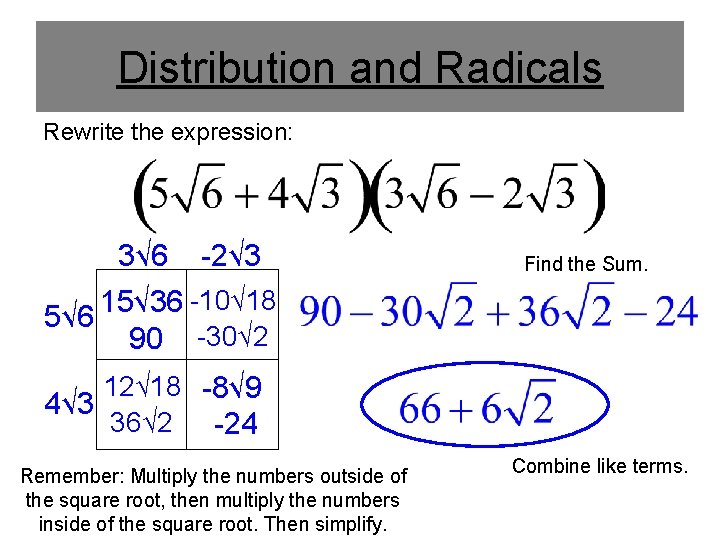
Distribution and Radicals Rewrite the expression: 3√ 6 -2√ 3 Find the Sum. -10√ 18 15√ 36 5√ 6 90 -30√ 2 12√ 18 -8√ 9 4√ 3 36√ 2 -24 Remember: Multiply the numbers outside of the square root, then multiply the numbers inside of the square root. Then simplify. Combine like terms.
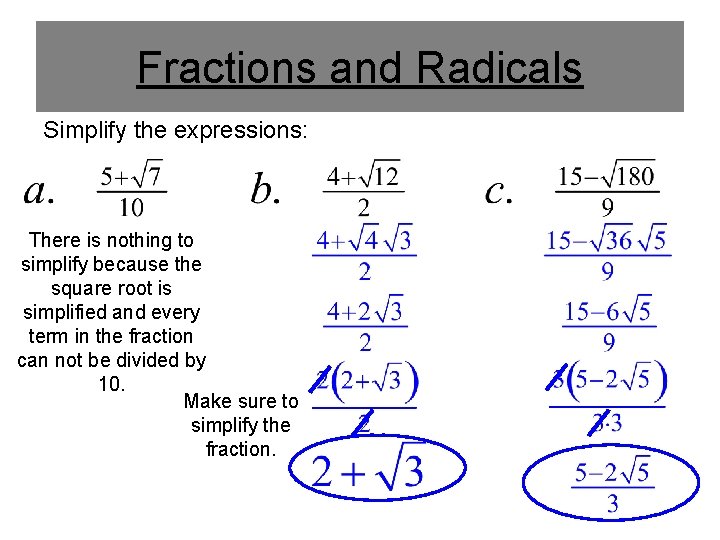
Fractions and Radicals Simplify the expressions: There is nothing to simplify because the square root is simplified and every term in the fraction can not be divided by 10. Make sure to simplify the fraction.
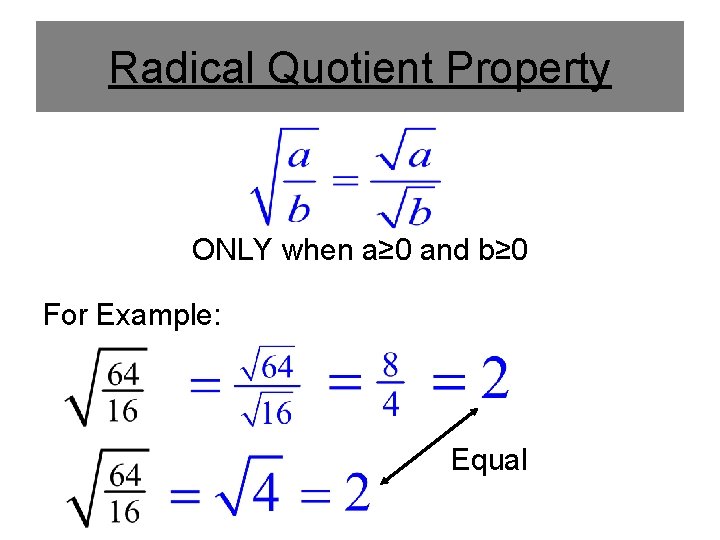
Radical Quotient Property ONLY when a≥ 0 and b≥ 0 For Example: Equal

The Square Root of a Fraction Write the following as a radical (square root) in simplest form: Take the square root of the numerator and the denominator Simplify.
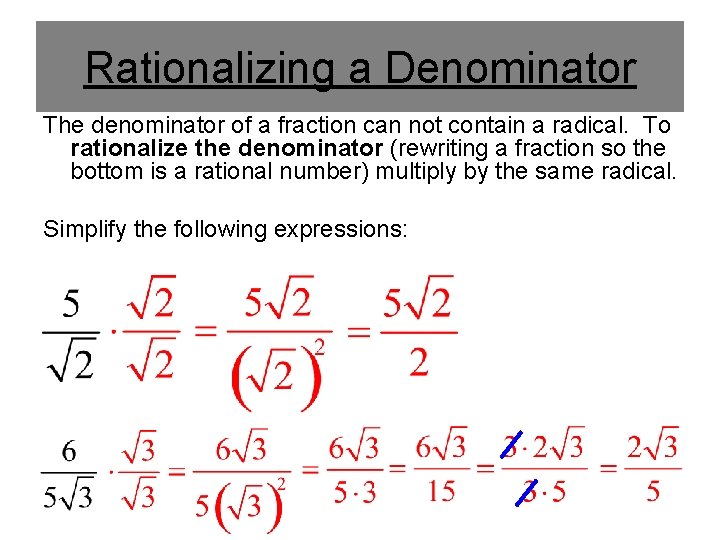
Rationalizing a Denominator The denominator of a fraction can not contain a radical. To rationalize the denominator (rewriting a fraction so the bottom is a rational number) multiply by the same radical. Simplify the following expressions:
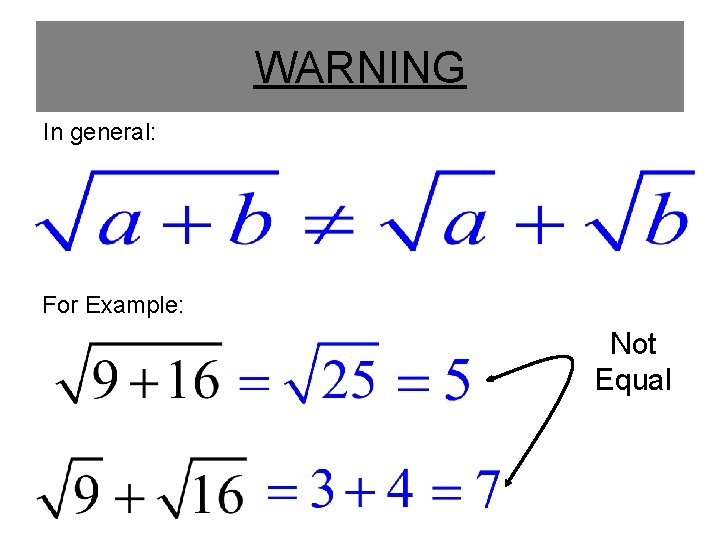
WARNING In general: For Example: Not Equal
- Slides: 15