SIMPLE HARMONIC MOTION AND SPRINGS SIMPLE HARMONIC MOTION

SIMPLE HARMONIC MOTION Starts from a stable point or a rest point When an

SPRING MOTION When a spring is stretched and let go, it undergoes a longitudinal

FOR THE PURPOSES OF SHM… We consider an ideal spring… An ideal spring has

IF WE WERE TO EXAMINE THE MOTION WRT TIME What does this look like?

LET’S SEE HOW A UNIT CIRCLE RELATES TO SHM https: //www. geogebratube. org/student/m 87292

HOW CAN WE RELATE UCM TO SHM? What do we know about things moving

SHM ISN’T REAL LIFE SO LET’S LOOK AT DHM Damped Harmonic Motion Periodic or

DAMPING OF CAR SHOCKS Damping for a car’s shocks: - 0. 7 is ideal

EXAMPLE A pendulum is disturbed from rest and is released from an amplitude of

EXAMPLE A spring (k=20 N/m) is compressed 30 cm by a ball (m= 100

26. A 0. 20 kg mass is hung from a vertical spring of force

EXAMPLE A 35 kg child is bouncing on a pogo stick, if the spring

REVIEW VIDEO https: //www. youtube. com/watch? v=Vn. Gko. Mo. Ukg. I

4. 5 Pg 217 Q 23 -26 Pg 219 Q 12 -14 P 9

Slides: 19

Download presentation

SIMPLE HARMONIC MOTION AND SPRINGS

SIMPLE HARMONIC MOTION Starts from a stable point or a rest point When an object is disturbed, it has a restorative force which tries to restore the object to its rest position Generally, the force (and therefore) acceleration is proportional to displacement This results in a back and forth motion that continues indefinitely

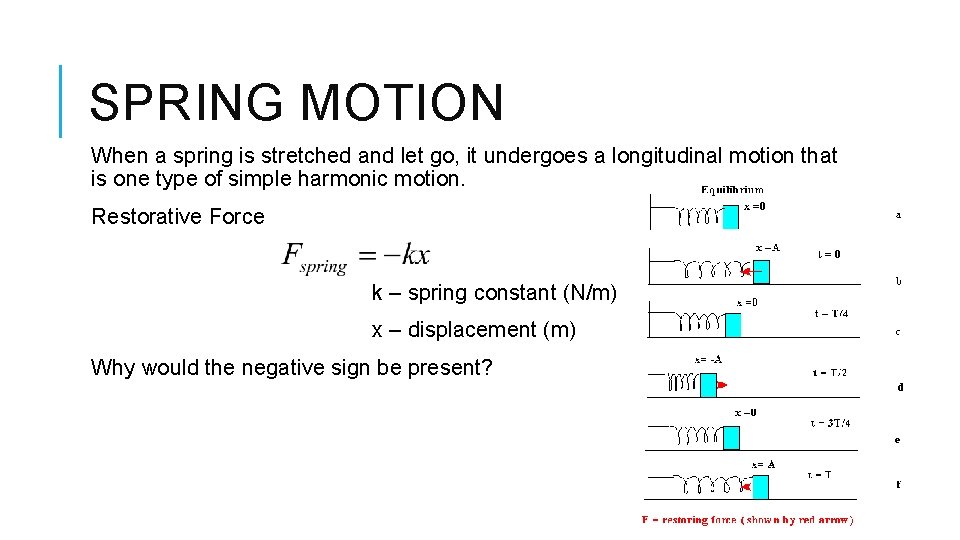
SPRING MOTION When a spring is stretched and let go, it undergoes a longitudinal motion that is one type of simple harmonic motion. Restorative Force k – spring constant (N/m) x – displacement (m) Why would the negative sign be present?

FOR THE PURPOSES OF SHM… We consider an ideal spring… An ideal spring has no internal or external friction acting upon it In a practical (unrealistic) sense, this means that spring keeps oscillating forever

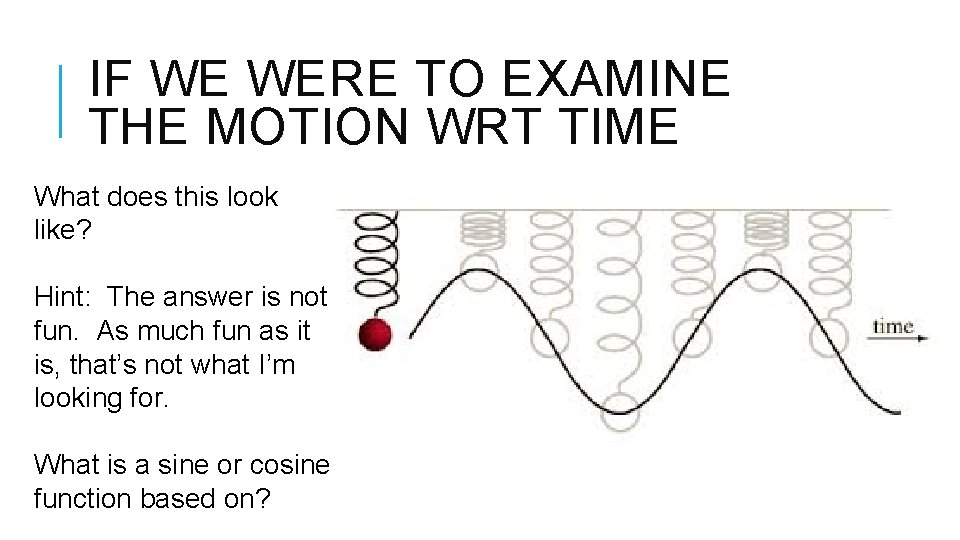
IF WE WERE TO EXAMINE THE MOTION WRT TIME What does this look like? Hint: The answer is not fun. As much fun as it is, that’s not what I’m looking for. What is a sine or cosine function based on?

LET’S SEE HOW A UNIT CIRCLE RELATES TO SHM https: //www. geogebratube. org/student/m 87292 How does SHM relate to the unit circle? Observe! Maximum speed through the rest point Stopped at the amplitude f of motion on a point of the circle = f of vibration in SHM They two motions are in phase Radius of the circle = amplitude

HOW CAN WE RELATE UCM TO SHM? What do we know about things moving in a circle? Derive

SHM ISN’T REAL LIFE SO LET’S LOOK AT DHM Damped Harmonic Motion Periodic or repeated motion where amplitude decreases with time There are no perfect springs!!!

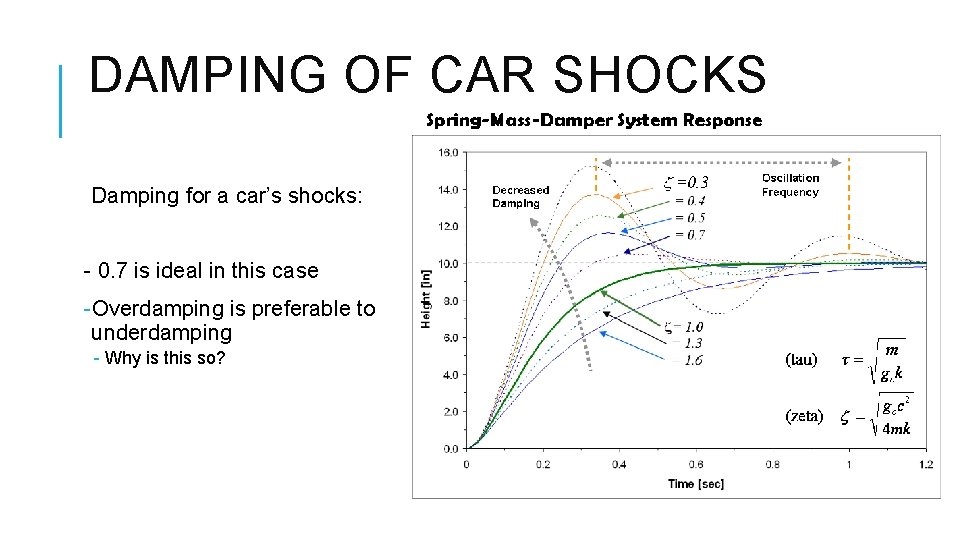
DAMPING OF CAR SHOCKS Damping for a car’s shocks: - 0. 7 is ideal in this case -Overdamping is preferable to underdamping - Why is this so?

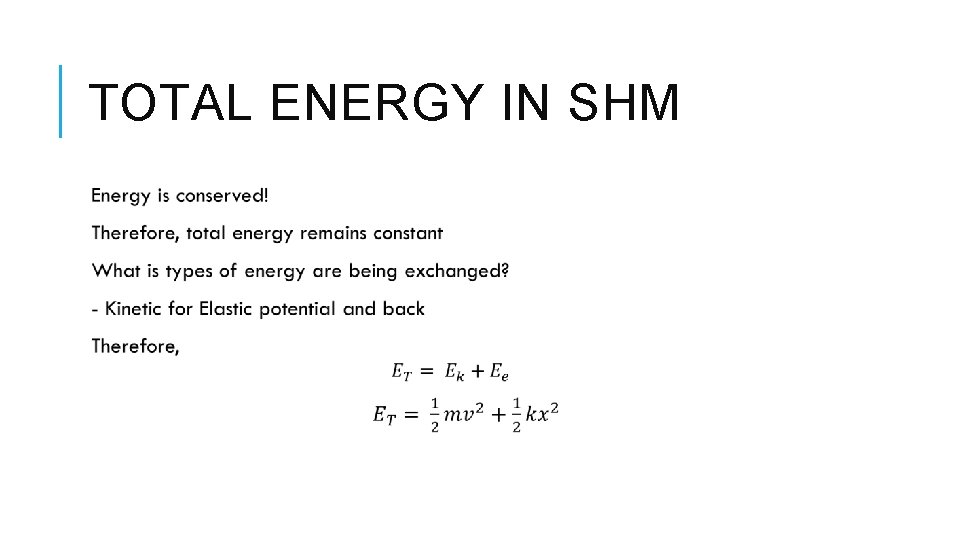
TOTAL ENERGY IN SHM

EXAMPLE A pendulum is disturbed from rest and is released from an amplitude of 15 cm. If the pendulum has a mass of 45 g and a spring constant of 26 N/m, what will the period of the oscillation be?

EXAMPLE A spring (k=20 N/m) is compressed 30 cm by a ball (m= 100 g) and fired upwards. How fast will the object be moving after it has a vertical displacement of 20 cm after it leaves the spring?

26. A 0. 20 kg mass is hung from a vertical spring of force constant 55 N/m. When the spring is released from its unstretched equilibrium position, the mass is allowed to fall. Use the conservation of energy to determine: a) the speed of the mass after it falls 1. 5 cm. b) the distance the mass will fall before reversing direction

EXAMPLE A 35 kg child is bouncing on a pogo stick, if the spring constant is 4945 N/m and it is compressed by 25 cm, how high will the child bounce?

QUESTION # 16

#18 -20