Simple Groups of Lie Type David Renardy Simple

Simple Groups of Lie Type David Renardy

Simple Groups Simple Group- A nontrivial group whose only normal subgroups are itself and the trivial subgroup. Simple groups are thought to be classified as either: ▪ ▪ Cyclic groups of prime order (Ex. G=<p>) Alternating groups of degree at least 5. (Ex. A 5 ) Groups of Lie Type (Ex. E 8 ) One of the 26 Sporadic groups (Ex. The Monster) First complete proof in the early 90’s, 2 nd Generation proof running around 5, 000 pages.

Lie Groups Named after Sophus Lie (1842 -1899) Definition: A group which is a differentiable manifold and whose operations are differentiable. Manifold- A mathematical space where every point has a neighborhood representing Euclidean space. These neighborhoods can be considered “maps” and the representation of the entire manifold, an “atlas” (Ex. Using maps when the earth is a sphere) Differentiable Manifolds-Manifolds where transformations between maps are all differentiable.

Lie Groups (cont’d) Examples: Points on the Real line under addition A circle with arbitrary identity point and multiplication by Θ mod 2π representing the rotation of the circle by Θ radians. The Orthogonal group (set of all orthogonal nxn matrices. ) Standard Model in particle physics U(1)×SU(2)×SU(3)

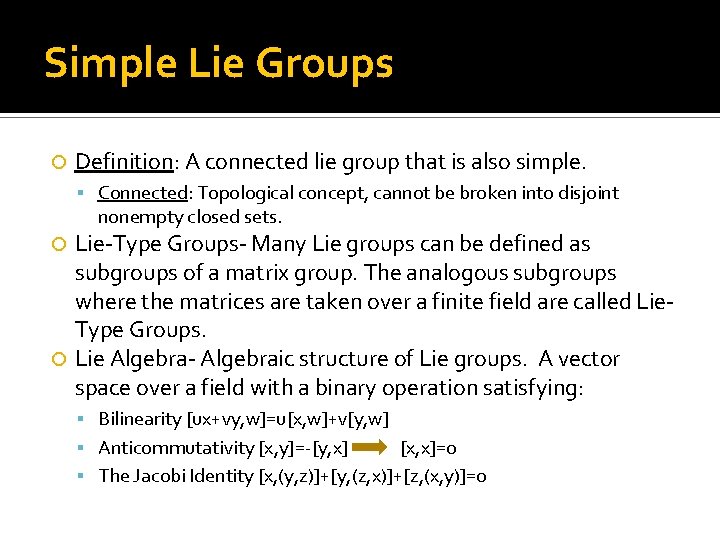
Simple Lie Groups Definition: A connected lie group that is also simple. Connected: Topological concept, cannot be broken into disjoint nonempty closed sets. Lie-Type Groups- Many Lie groups can be defined as subgroups of a matrix group. The analogous subgroups where the matrices are taken over a finite field are called Lie. Type Groups. Lie Algebra- Algebraic structure of Lie groups. A vector space over a field with a binary operation satisfying: Bilinearity [ux+vy, w]=u[x, w]+v[y, w] Anticommutativity [x, y]=-[y, x] [x, x]=0 The Jacobi Identity [x, (y, z)]+[y, (z, x)]+[z, (x, y)]=0

Classification of Simple Lie Groups Infinite families An series corresponds to the Special Unital Groups SU(n+1) (nxn unitary matrices with unit determinant) Bn series corresponds to the Special Orthogonal Group SO(2 n+1) (nxn orthogonal matrices with unit determinatnt) Cn series corresponds to the Symplectic (quaternionic unitary) group Sp(2 n) Dn series corresponds to the Special Orthogonal Group SO(2 n)

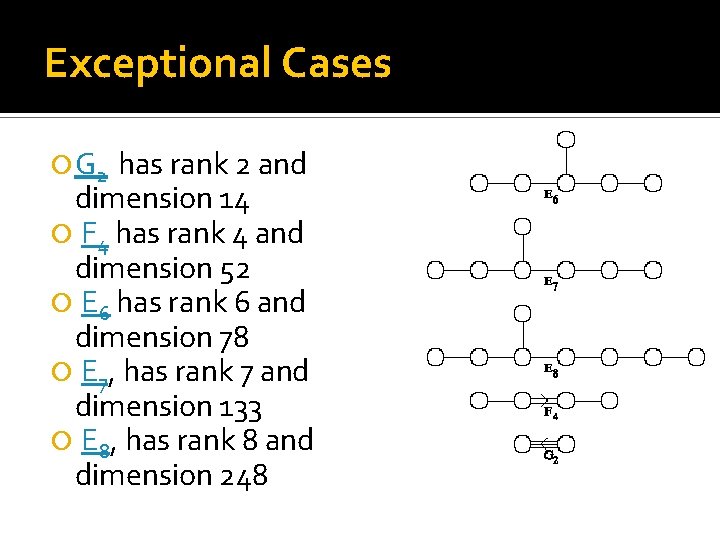
Exceptional Cases G 2 has rank 2 and dimension 14 F 4 has rank 4 and dimension 52 E 6 has rank 6 and dimension 78 E 7, has rank 7 and dimension 133 E 8, has rank 8 and dimension 248

Simple Groups of Lie Type Classical Groups Special Linear, orthogonal, symplectic, or unitary group. Chevalley Groups Defined Simple Groups of Lie Type over the integers by constructing a Chevalley basis. Steinberg Groups Completed the classical groups with unitary groups and split orthogonal groups ▪ ▪ the unitary groups 2 An, from the order 2 automorphism of An; further orthogonal groups 2 Dn, from the order 2 automorphism of Dn; the new series 2 E 6, from the order 2 automorphism of E 6; the new series 3 D 4, from the order 3 automorphism of D 4.

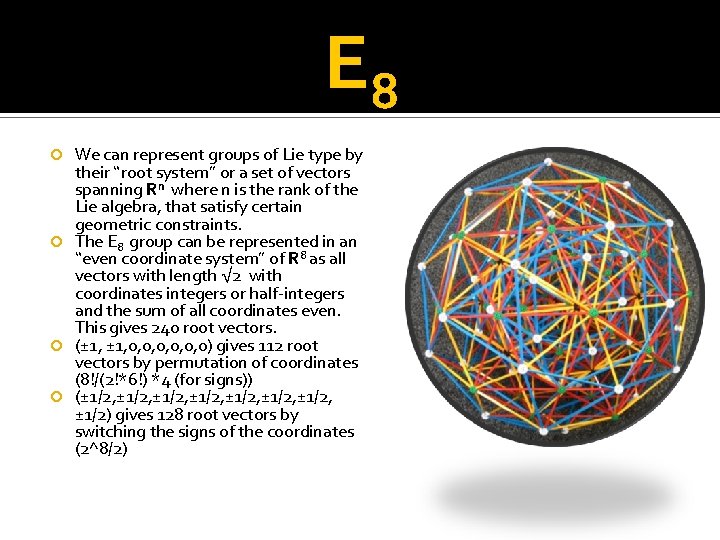
E 8 We can represent groups of Lie type by their “root system” or a set of vectors spanning Rn where n is the rank of the Lie algebra, that satisfy certain geometric constraints. The E 8 group can be represented in an “even coordinate system” of R 8 as all vectors with length √ 2 with coordinates integers or half-integers and the sum of all coordinates even. This gives 240 root vectors. (± 1, 0, 0, 0, 0) gives 112 root vectors by permutation of coordinates (8!/(2!*6!) *4 (for signs)) (± 1/2, ± 1/2, ± 1/2) gives 128 root vectors by switching the signs of the coordinates (2^8/2)

Science and E 8 Applications in Theoretical Physics relate to String Theory and “supergravity” “The group E 8×E 8 (the Cartesian product of two copies of E 8) serves as the gauge group of one of the two types of heterotic string and is one of two anomaly-free gauge groups that can be coupled to the N = 1 supergravity in 10 dimensions. ”

Sources http: //cache. eb. com/eb/image? id=2106&rend. Type. Id= 4 http: //aimath. org/E 8/images/e 8 plane 2 a. jpg http: //www. mpagarching. mpg. de/galform/press/seq. D_063 a_small. jpg http: //superstruny. aspweb. cz/images/fyzika/aether/h oneycomb. gif Wikipedia. org Mathworld. com Aschbacher, Michael. The Finite Simple Groups and Their Classification. United States: Yale University, 1980.