Sections 11 1 11 2 11 3 11

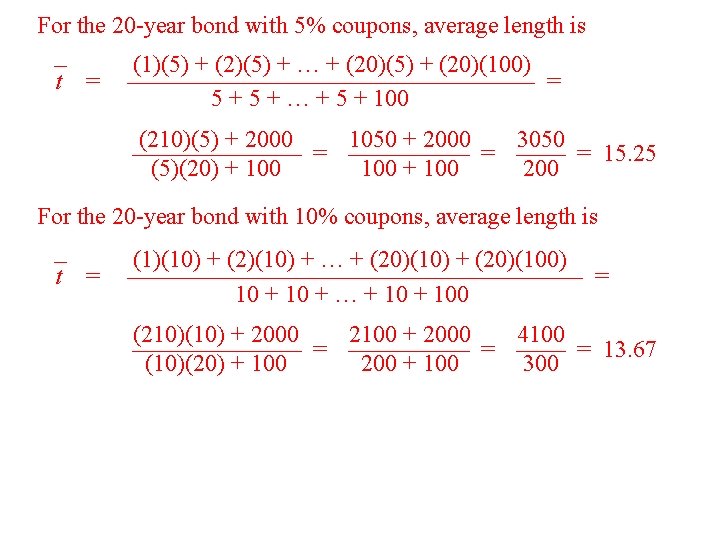
























- Slides: 47

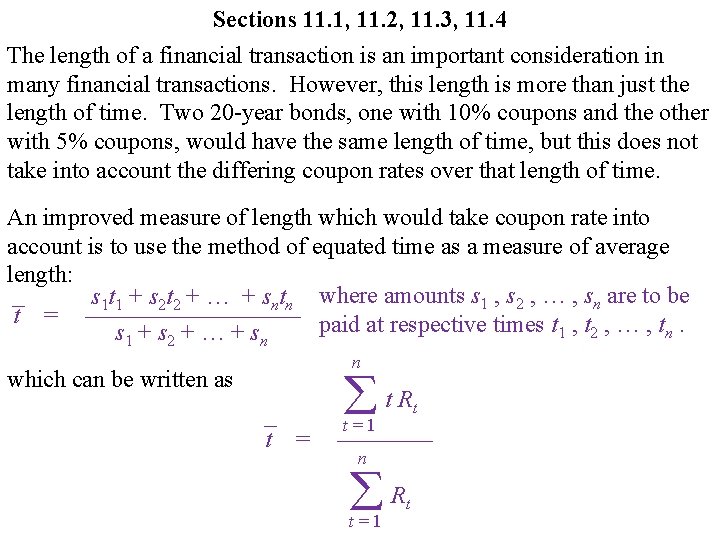
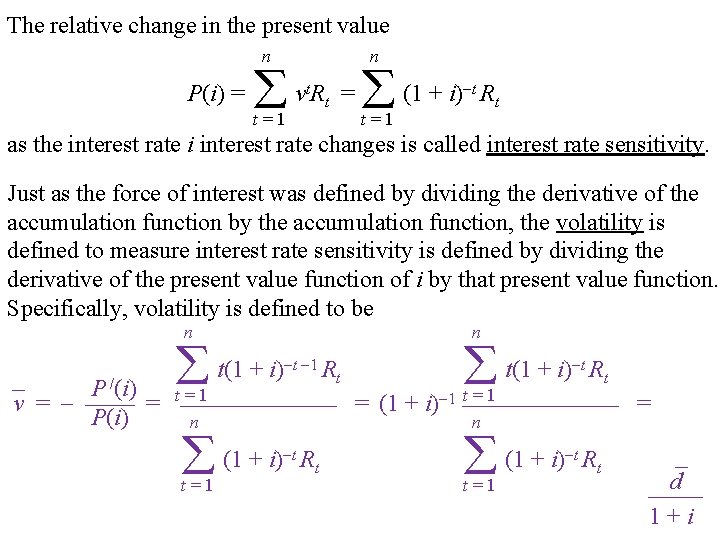
Sections 11. 1, 11. 2, 11. 3, 11. 4 The length of a financial transaction is an important consideration in many financial transactions. However, this length is more than just the length of time. Two 20 -year bonds, one with 10% coupons and the other with 5% coupons, would have the same length of time, but this does not take into account the differing coupon rates over that length of time. An improved measure of length which would take coupon rate into account is to use the method of equated time as a measure of average length: s 1 t 1 + s 2 t 2 + … + sntn where amounts s 1 , s 2 , … , sn are to be t = ————— paid at respective times t , … , t. 1 2 n s +s +…+s 1 2 n n t. R which can be written as t = t=1 t ———— n R t=1 t

Chapter 11 Exercises 1. Consider two 20 -year bonds, one with 5% coupons and the other with 10% coupons. (a) Use the method of equated time to find the average length for each of the two bonds. Note that in doing the calculation, we can use any face value, say $100, since this value can be changed by multiplying the numerator and denominator by any value. For the 20 -year bond with 5% coupons, average length is t = (1)(5) + (2)(5) + … + (20)(5) + (20)(100) ————————— = 5 + … + 5 + 100 (210)(5) + 2000 1050 + 2000 3050 = = = 15. 25 (5)(20) + 100 200 For the 20 -year bond with 10% coupons, average length is
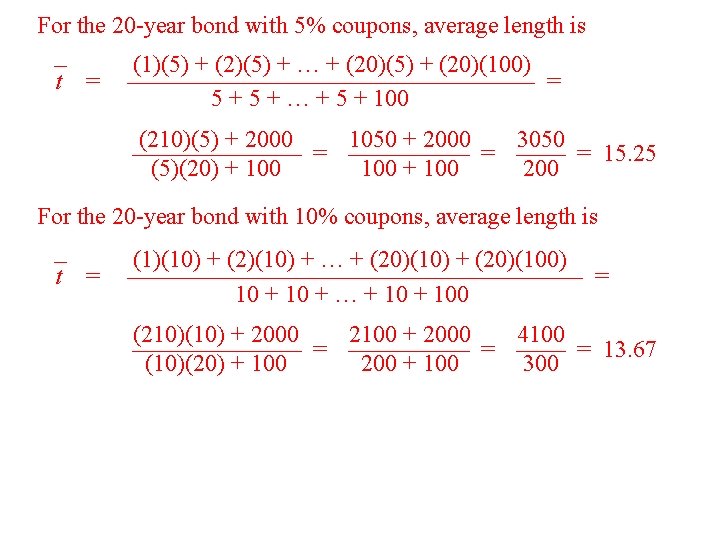
For the 20 -year bond with 5% coupons, average length is t = (1)(5) + (2)(5) + … + (20)(5) + (20)(100) ————————— = 5 + … + 5 + 100 (210)(5) + 2000 1050 + 2000 3050 = = = 15. 25 (5)(20) + 100 200 For the 20 -year bond with 10% coupons, average length is t = (1)(10) + (2)(10) + … + (20)(10) + (20)(100) —————————— = 10 + … + 100 (210)(10) + 2000 2100 + 2000 4100 = = = 13. 67 (10)(20) + 100 200 + 100 300

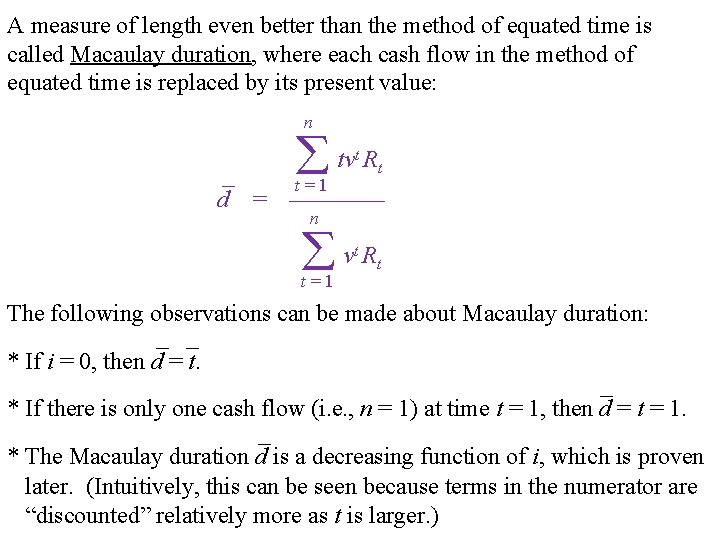
A measure of length even better than the method of equated time is called Macaulay duration, where each cash flow in the method of equated time is replaced by its present value: n tv R t t=1 t d = ———— n v. R t t=1 t The following observations can be made about Macaulay duration: * If i = 0, then d = t. * If there is only one cash flow (i. e. , n = 1) at time t = 1, then d = t = 1. * The Macaulay duration d is a decreasing function of i, which is proven later. (Intuitively, this can be seen because terms in the numerator are “discounted” relatively more as t is larger. )

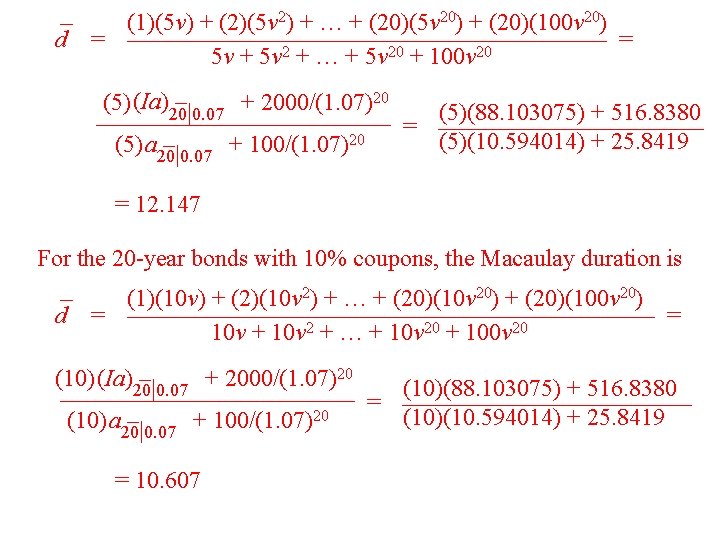
Chapter 11 Exercises 1. Consider two 20 -year bonds, one with a 5% annual coupon rate and the other with a 10% annual coupon rate. (b) Find the Macaulay duration for each of the two bonds with a 7% effective rate of interest. For the 20 -year bond with 5% coupons, the Macaulay duration is (1)(5 v) + (2)(5 v 2) + … + (20)(5 v 20) + (20)(100 v 20) d = —————————— = 2 20 20 5 v + … + 5 v + 100 v –|0. 07 + 2000/(1. 07)20 (5) (Ia)20 –|0. 07 + 100/(1. 07)20 (5) a 20 (5)(88. 103075) + 516. 8380 = (5)(10. 594014) + 25. 8419 = 12. 147 For the 20 -year bond with 10% coupons, the Macaulay duration is

(1)(5 v) + (2)(5 v 2) + … + (20)(5 v 20) + (20)(100 v 20) d = —————————— = 2 20 20 5 v + … + 5 v + 100 v –|0. 07 + 2000/(1. 07)20 (5) (Ia)20 –|0. 07 + 100/(1. 07)20 (5) a 20 (5)(88. 103075) + 516. 8380 = (5)(10. 594014) + 25. 8419 = 12. 147 For the 20 -year bonds with 10% coupons, the Macaulay duration is (1)(10 v) + (2)(10 v 2) + … + (20)(10 v 20) + (20)(100 v 20) d = ——————————— = 2 20 20 10 v + … + 10 v + 100 v –|0. 07 + 2000/(1. 07)20 (10) (Ia)20 –|0. 07 + 100/(1. 07)20 (10) a 20 = 10. 607 (10)(88. 103075) + 516. 8380 = (10)(10. 594014) + 25. 8419

The relative change in the present value n P(i) = n v R = (1 + i) t t t=1 t R t=1 t as the interest rate i interest rate changes is called interest rate sensitivity. Just as the force of interest was defined by dividing the derivative of the accumulation function by the accumulation function, the volatility is defined to measure interest rate sensitivity is defined by dividing the derivative of the present value function of i by that present value function. Specifically, volatility is defined to be n P /(i) v = = P(i) t(1 + i) n t 1 R t=1 n (1 + i) t=1 t t(1 + i) t R = (1 + i) 1 t = 1 = n t R t (1 + i) t=1 t t R t d 1+i

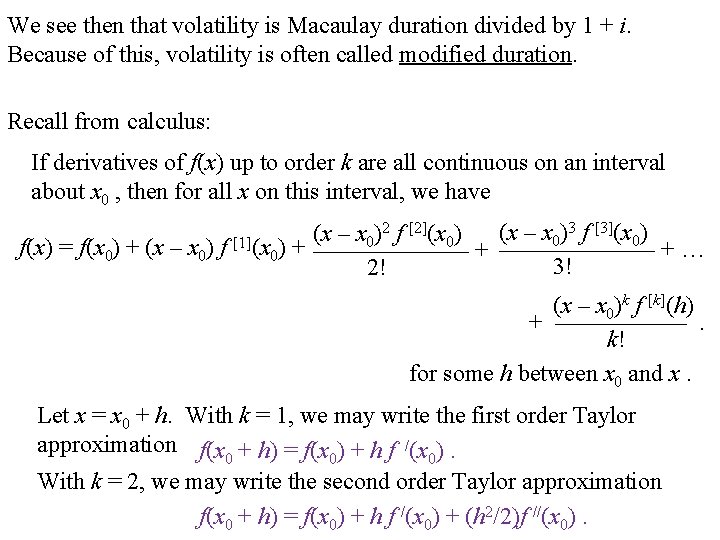
We see then that volatility is Macaulay duration divided by 1 + i. Because of this, volatility is often called modified duration. Recall from calculus: If derivatives of f(x) up to order k are all continuous on an interval about x 0 , then for all x on this interval, we have f(x) = f(x 0) + (x – x 0) f [1](x (x – x 0)3 f [3](x 0) (x – x 0)2 f [2](x 0) 0) + —————–— + … 3! 2! (x – x 0)k f [k](h) + —————–. k! for some h between x 0 and x. Let x = x 0 + h. With k = 1, we may write the first order Taylor approximation f(x + h) = f(x ) + h f /(x ). 0 0 0 With k = 2, we may write the second order Taylor approximation f(x 0 + h) = f(x 0) + h f /(x 0) + (h 2/2)f //(x 0).

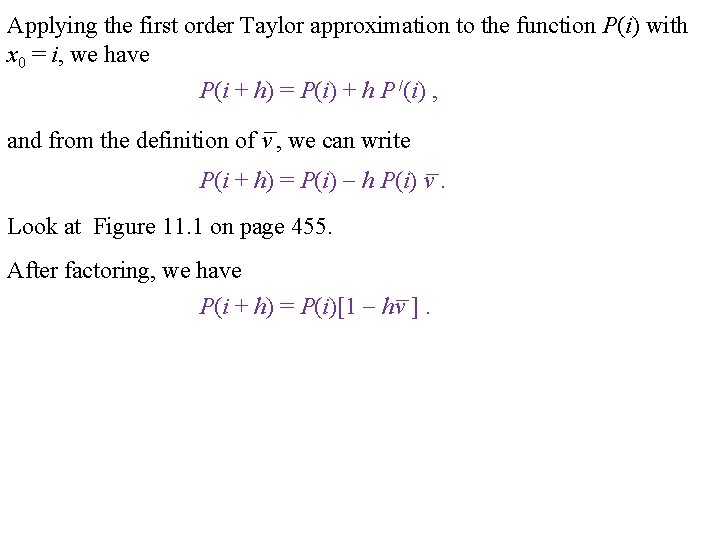
Applying the first order Taylor approximation to the function P(i) with x 0 = i, we have P(i + h) = P(i) + h P /(i) , and from the definition of v , we can write P(i + h) = P(i) h P(i) v. Look at Figure 11. 1 on page 455. After factoring, we have P(i + h) = P(i)[1 hv ].

Chapter 11 Exercises 2. Consider a 20 -year bond with a par value of $1000 and an annual coupon rate of 7%. Suppose 7% is the effective rate of interest. (a) Find the price of the bond. 1 = $1000 as should be expected! –| 0. 07 + 1000 70 a 20 1. 0720 (b) Find the actual price of the bond if the effective interest rate were to change to 8%. 1 = $901. 82 –| 0. 08 + 1000 P(0. 08) = 70 a 20 1. 0820 (c) Find the Macaulay duration and the modified duration (using the 7% effective rate).

Chapter 11 Exercises 2. Consider a 20 -year bond with a par value of $1000 and an annual coupon rate of 7%. Suppose 7% is the effective rate of interest. (c) Find the Macaulay duration and the modified duration (using the 7% effective rate). (1)(7 v) + (2)(7 v 2) + … + (20)(7 v 20) + (20)(100 v 20) d = —————————— = 2 20 20 7 v + … + 7 v + 100 v –|0. 07 + 2000/(1. 07)20 (7) (Ia)20 –|0. 07 + 100/(1. 07)20 (7) a 20 = 11. 3356 v d = —— = 10. 5940 1. 07 (7)(88. 103075) + 516. 8380 = (7)(10. 594014) + 25. 8419

Chapter 11 Exercises 2. Consider a 20 -year bond with a par value of $1000 and an annual coupon rate of 7%. Suppose 7% is the effective rate of interest. (d) Use the first order Taylor approximation to find the estimated price of the bond if the effective interest rate were to change to 8%. P(i + h) = P(i)[1 hv ] P(0. 07 + 0. 01) = P(0. 08) = P(0. 07)[1 0. 01(10. 5940)] = (1000)[0. 89406] = $894. 06 (e) Find the difference between the actual price and the estimated price based on the first order Taylor approximation. $901. 82 $894. 06 = $7. 76

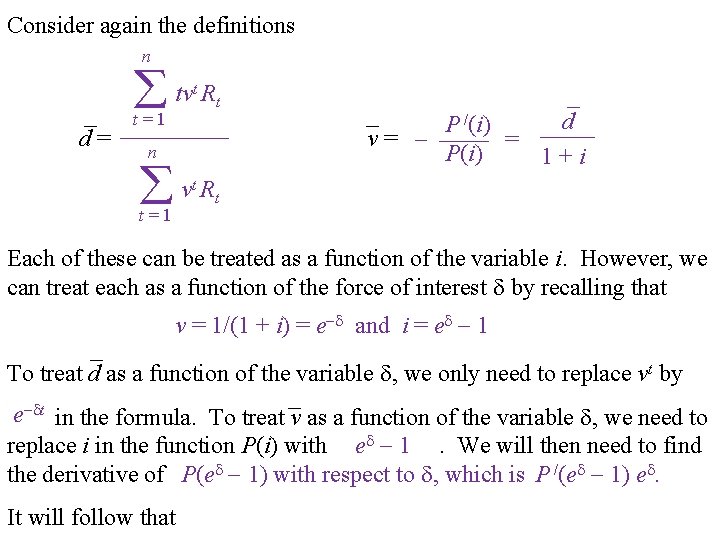
Consider again the definitions n tv R t d= t=1 t n v. R t t=1 d P /(i) v = = P(i) 1+i t Each of these can be treated as a function of the variable i. However, we can treat each as a function of the force of interest by recalling that v = 1/(1 + i) = e and i = e 1 To treat d as a function of the variable , we only need to replace vt by e t in the formula. To treat v as a function of the variable , we need to replace i in the function P(i) with e 1. We will then need to find the derivative of P(e 1) with respect to , which is P /(e 1) e. It will follow that

n te d= t R t=1 n e t=1 t R t t P /(i) P /(e 1) e (1 + i) = v = P(i) P(e 1) d (1 + i) = d 1+i In other words, the Macaulay duration and modified duration are the same when a force of interest is used rather than a discrete interest rate.

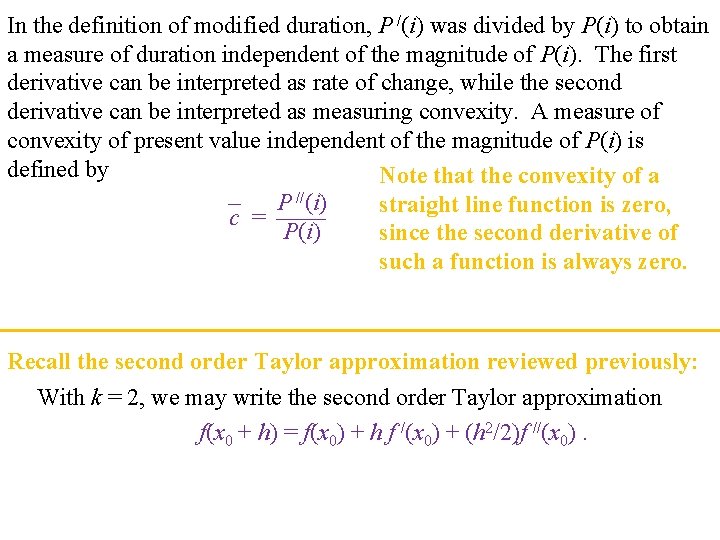
In the definition of modified duration, P /(i) was divided by P(i) to obtain a measure of duration independent of the magnitude of P(i). The first derivative can be interpreted as rate of change, while the second derivative can be interpreted as measuring convexity. A measure of convexity of present value independent of the magnitude of P(i) is defined by Note that the convexity of a P //(i) straight line function is zero, c = P(i) since the second derivative of such a function is always zero. Recall the second order Taylor approximation reviewed previously: With k = 2, we may write the second order Taylor approximation f(x 0 + h) = f(x 0) + h f /(x 0) + (h 2/2)f //(x 0).

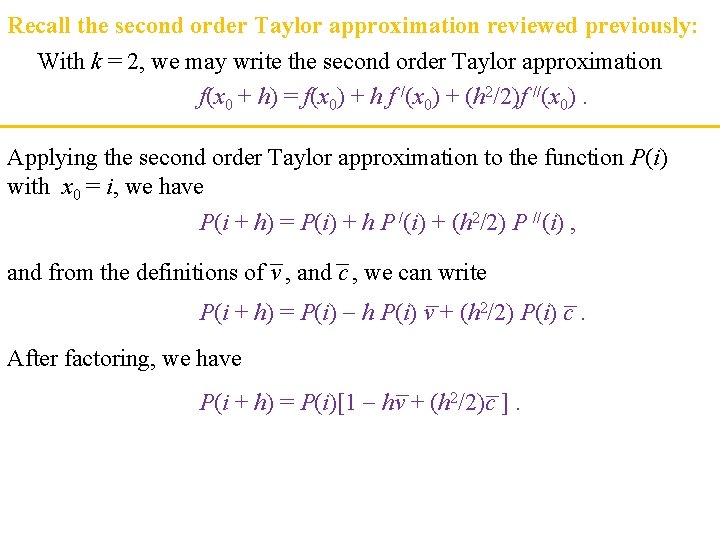
Recall the second order Taylor approximation reviewed previously: With k = 2, we may write the second order Taylor approximation f(x 0 + h) = f(x 0) + h f /(x 0) + (h 2/2)f //(x 0). Applying the second order Taylor approximation to the function P(i) with x 0 = i, we have P(i + h) = P(i) + h P /(i) + (h 2/2) P //(i) , and from the definitions of v , and c , we can write P(i + h) = P(i) h P(i) v + (h 2/2) P(i) c. After factoring, we have P(i + h) = P(i)[1 hv + (h 2/2)c ].

Modified duration is used as a measure of interest sensitivity (i. e. , rate of change) in present value, and is based on the derivative of present value with respect to interest rate i. A measure of interest sensitivity (i. e. , rate of change) in modified duration can be based on the derivative of present value with respect to interest rate i (and should be no surprise that convexity will be involved, since a second derivative is in general a rate of change of rate of change). 2 dv d P /(i) P //(i) [P /(i)]2 P /(i) P //(i) = = = 2 di di P(i) [P(i)] P(i) v 2 c

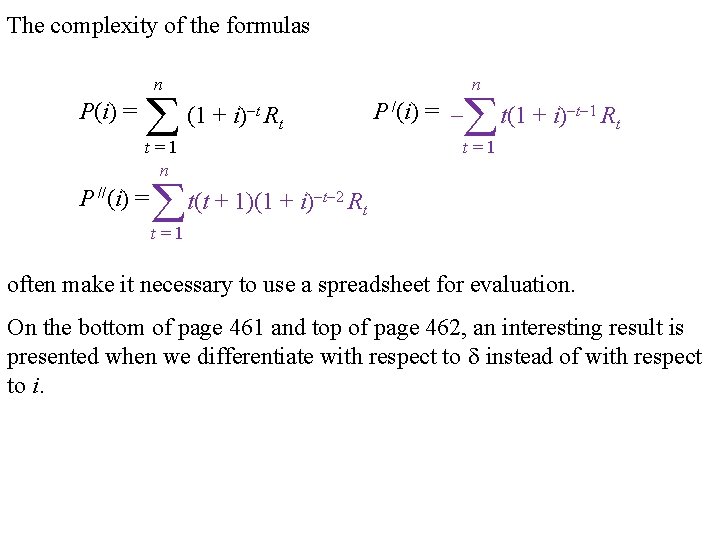
The complexity of the formulas n P(i) = n (1 + i) t Rt t(t + 1)(1 + i) t 2 Rt t=1 n P //(i) = P /(i) = t(1 + i) t 1 Rt t=1 often make it necessary to use a spreadsheet for evaluation. On the bottom of page 461 and top of page 462, an interesting result is presented when we differentiate with respect to instead of with respect to i.

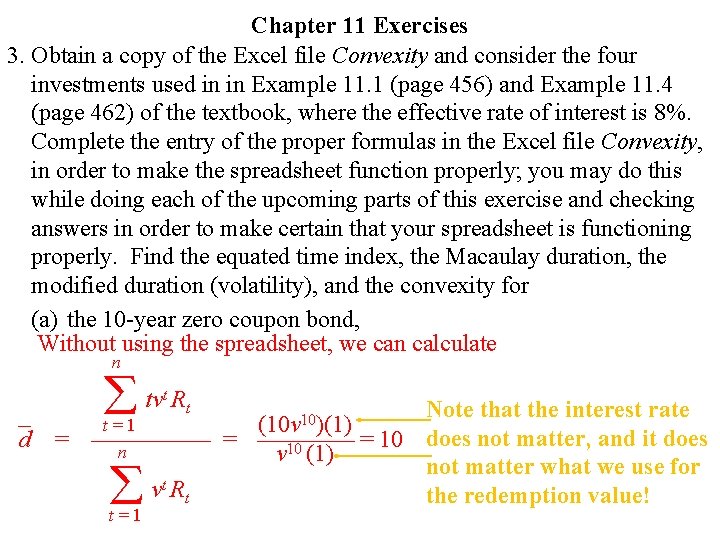
Chapter 11 Exercises 3. Obtain a copy of the Excel file Convexity and consider the four investments used in in Example 11. 1 (page 456) and Example 11. 4 (page 462) of the textbook, where the effective rate of interest is 8%. Complete the entry of the proper formulas in the Excel file Convexity, in order to make the spreadsheet function properly; you may do this while doing each of the upcoming parts of this exercise and checking answers in order to make certain that your spreadsheet is functioning properly. Find the equated time index, the Macaulay duration, the modified duration (volatility), and the convexity for (a) the 10 -year zero coupon bond, Without using the spreadsheet, we can calculate n t. R t = t (10)(1) ———— = = 10 (1) n t=1 R t=1 t Note that the interest rate does not matter, and it does not matter what we use for the redemption value!

Chapter 11 Exercises 3. Obtain a copy of the Excel file Convexity and consider the four investments used in in Example 11. 1 (page 456) and Example 11. 4 (page 462) of the textbook, where the effective rate of interest is 8%. Complete the entry of the proper formulas in the Excel file Convexity, in order to make the spreadsheet function properly; you may do this while doing each of the upcoming parts of this exercise and checking answers in order to make certain that your spreadsheet is functioning properly. Find the equated time index, the Macaulay duration, the modified duration (volatility), and the convexity for (a) the 10 -year zero coupon bond, Without using the spreadsheet, we can calculate n tv R t d = t t=1 ————— n v. R t t=1 t Note that the interest rate = 10 does not matter, and it does = 10 v (1) not matter what we use for the redemption value! (10 v 10)(1)

Chapter 11 Exercises 3. Obtain a copy of the Excel file Convexity and consider the four investments used in in Example 11. 1 (page 456) and Example 11. 4 (page 462) of the textbook, where the effective rate of interest is 8%. Complete the entry of the proper formulas in the Excel file Convexity, in order to make the spreadsheet function properly; you may do this while doing each of the upcoming parts of this exercise and checking answers in order to make certain that your spreadsheet is functioning properly. Find the equated time index, the Macaulay duration, the modified duration (volatility), and the convexity for (a) the 10 -year zero coupon bond, Without using the spreadsheet, we can define 1 10 / P(i) = 10 P (i) = 11 (1 + i) P /(0. 08) 10 v = = = 9. 259259 P(0. 08) 1. 08 P //(i) 110 = 12 (1 + i) Note that it does not matter what we use for the redemption value!

Chapter 11 Exercises 3. Obtain a copy of the Excel file Convexity and consider the four investments used in in Example 11. 1 (page 456) and Example 11. 4 (page 462) of the textbook, where the effective rate of interest is 8%. Complete the entry of the proper formulas in the Excel file Convexity, in order to make the spreadsheet function properly; you may do this while doing each of the upcoming parts of this exercise and checking answers in order to make certain that your spreadsheet is functioning properly. Find the equated time index, the Macaulay duration, the modified duration (volatility), and the convexity for (a) the 10 -year zero coupon bond, Without using the spreadsheet, we can define 1 10 / P(i) = 10 P (i) = 11 (1 + i) P //(0. 08) 110 c = = = 94. 30727 P(0. 08) 1. 082 P //(i) 110 = 12 (1 + i) Note that it does not matter what we use for the redemption value!

Chapter 11 Exercises 3. Obtain a copy of the Excel file Convexity and consider the four investments used in in Example 11. 1 (page 456) and Example 11. 4 (page 462) of the textbook, where the effective rate of interest is 8%. Complete the entry of the proper formulas in the Excel file Convexity, in order to make the spreadsheet function properly; you may do this while doing each of the upcoming parts of this exercise and checking answers in order to make certain that your spreadsheet is functioning properly. Find the equated time index, the Macaulay duration, the modified duration (volatility), and the convexity for (a) the 10 -year zero coupon bond, From the spreadsheet: t = 10 d = 10 v = 9. 259259 c = 94. 30727 Note that changing the redemption value of the bond has no effect on any of these values; also note that changing the 8% effective interest rate affects only the modified duration and the convexity.

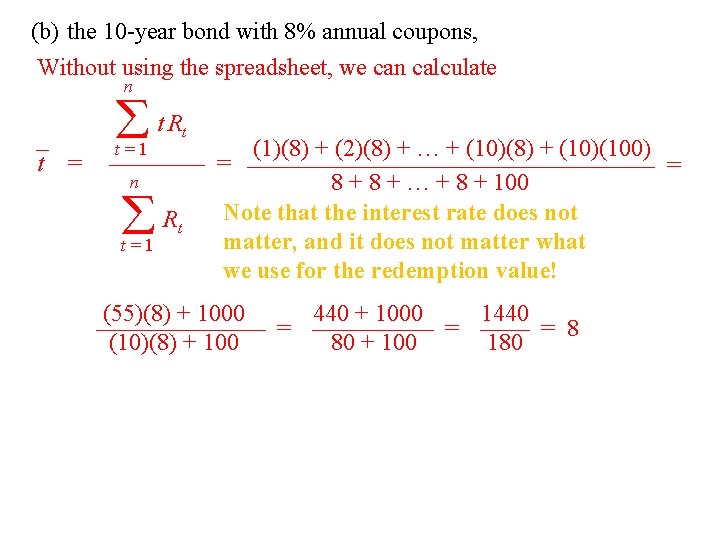
(b) the 10 -year bond with 8% annual coupons, Without using the spreadsheet, we can calculate n t. R t = t (1)(8) + (2)(8) + … + (10)(8) + (10)(100) ———— = ————————— = n 8 + … + 8 + 100 Note that the interest rate does not Rt matter, and it does not matter what t=1 we use for the redemption value! t=1 (55)(8) + 1000 440 + 1000 1440 = = = 8 (10)(8) + 100 80 + 100 180

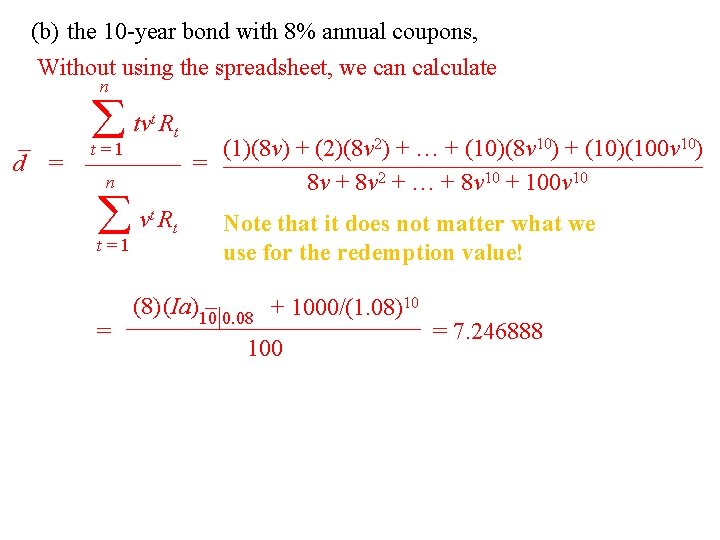
(b) the 10 -year bond with 8% annual coupons, Without using the spreadsheet, we can calculate n tv R t t (1)(8 v) + (2)(8 v 2) + … + (10)(8 v 10) + (10)(100 v 10) d = ———————————— n 8 v + 8 v 2 + … + 8 v 10 + 100 v 10 t=1 v. R t t=1 = t Note that it does not matter what we use for the redemption value! –|0. 08 + 1000/(1. 08)10 (8) (Ia)10 100 = 7. 246888

(b) the 10 -year bond with 8% annual coupons, Without using the spreadsheet, we can define 1 –| i + 1000 (1 + i)10 P(i) = 8 a 10 but finding P /(i) and P //(i) will not be easy. From the spreadsheet: t = 8 v = 6. 710081 d = 7. 246888 c = 60. 53132 Note that changing the amount of the mortgage has no effect on any of these values; also note that changing the 8% effective interest rate affects only the modified duration and the convexity.

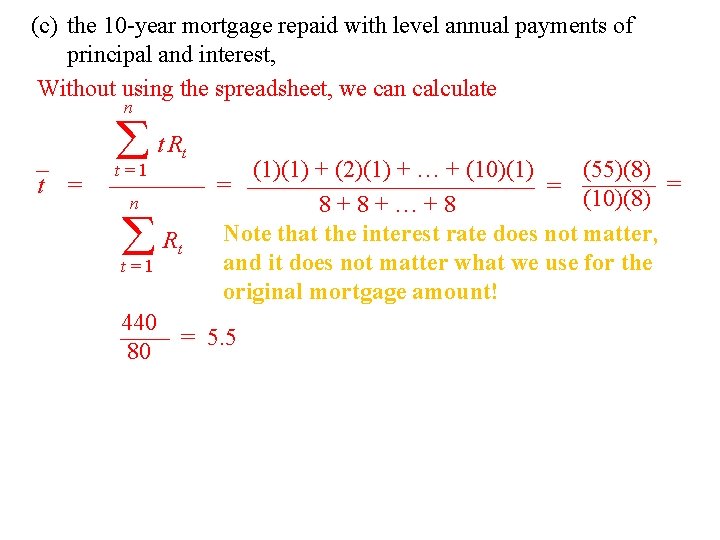
(c) the 10 -year mortgage repaid with level annual payments of principal and interest, Without using the spreadsheet, we can calculate n t. R t = t (1)(1) + (2)(1) + … + (10)(1) (55)(8) ———— = —————— = = (10)(8) n 8+8+…+8 Note that the interest rate does not matter, Rt and it does not matter what we use for the t=1 original mortgage amount! 440 = 5. 5 80 t=1

(c) the 10 -year mortgage repaid with level annual payments of principal and interest, Without using the spreadsheet, we can calculate n tv R t t (Ia)10 –|0. 08 v + 2 v 2 + … + 10 v 10 = 4. 871314 d = ———————— = a– 2 10 n v+v +…+v 10|0. 08 vt Rt Note that it does not matter what we t=1 use for the original mortgage amount! t=1

(c) the 10 -year mortgage repaid with level annual payments of principal and interest, Without using the spreadsheet, we can define –| i P(i) = a 10 but finding P /(i) and P //(i) will not be easy. From the spreadsheet: t = 5. 5 v = 4. 510476 d = 4. 871314 c = 31. 38711 Note that changing the amount of the mortgage has no effect on any of these values; also note that changing the 8% effective interest rate affects only the modified duration and the convexity.

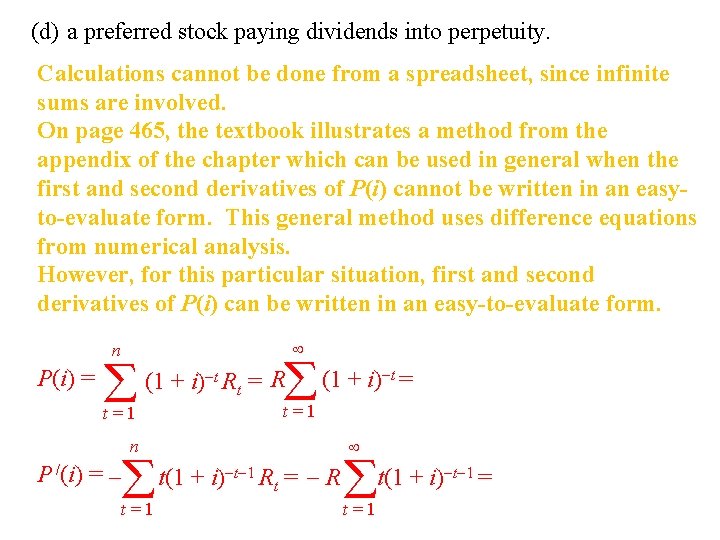
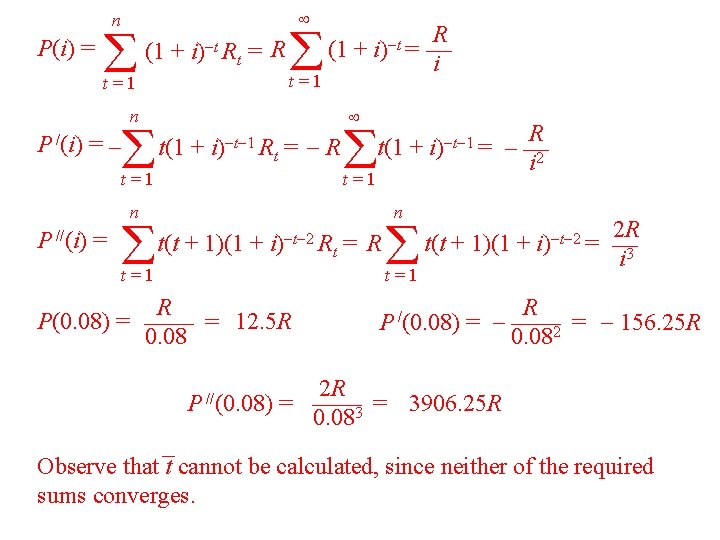
(d) a preferred stock paying dividends into perpetuity. Calculations cannot be done from a spreadsheet, since infinite sums are involved. On page 465, the textbook illustrates a method from the appendix of the chapter which can be used in general when the first and second derivatives of P(i) cannot be written in an easyto-evaluate form. This general method uses difference equations from numerical analysis. However, for this particular situation, first and second derivatives of P(i) can be written in an easy-to-evaluate form. n P(i) = (1 + i) t Rt = R t=1 (1 + i) t = t=1 n P /(i) = t=1 t(1 + i) t 1 Rt = R t(1 + i) t 1 = t=1

n P(i) = (1 + t=1 i) t R t = R (1 + t=1 n P /(i) = i) t = t(1 + i) t 1 Rt = R t=1 R t(1 + i) t 1 = i 2 n P //(i) = n t(t + 1)(1 + i) t 2 Rt = R t=1 R P(0. 08) = = 12. 5 R 0. 08 R i 2 R t(t + 1)(1 + i) t 2 = 3 i P /(0. 08) R = 2 = 156. 25 R 0. 08 2 R P //(0. 08) = 3 = 3906. 25 R 0. 08 Observe that t cannot be calculated, since neither of the required sums converges.

R P(0. 08) = = 12. 5 R 0. 08 P /(0. 08) R = 2 = 156. 25 R 0. 08 2 R P //(0. 08) = 3 = 3906. 25 R 0. 08 Observe that t cannot be calculated, since neither of the required sums converges. d = 12. 5(1. 08) = 13. 5 156. 25 R 12. 5 P /(0. 08) = v = = 12. 5 R P(0. 08) P //(0. 08) 3906. 25 R c = = = 312. 5 P(0. 08) 12. 5 R

Chapter 11 Exercises 4. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (a) Use the Excel file Convexity to find the equated time index, the Macaulay duration, the modified duration (volatility), and the convexity. Compare the values of the Macaulay duration and the modified duration (volatility) found from the spreadsheet with the values found in Exercise #2(c). From the spreadsheet: t = 14. 45833 v = 10. 59401 d = 11. 3356 c = 164. 6786 As expected, the values of the Macaulay duration and the modified duration (volatility) from the spreadsheet are the same values found in Exercise #2(c).

Chapter 11 Exercises 4. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (b) Use the second order Taylor approximation to find the estimated price of the bond if the effective interest rate were to change to 8%. P(0. 07) = 1000 from Exercise #2(a) P(i + h) = P(i)[1 hv + (h 2/2)c ] P(0. 07 + 0. 01) = P(0. 08) = P(0. 07)[1 0. 01(10. 5940) + (0. 012/2)(164. 6786)] = (1000)[0. 89406] = $902. 29

Chapter 11 Exercises 4. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (c) Assuming the effective interest rate were to change to 8%, find the difference between the actual price found in Exercise #2(b) and the estimated price based on the second order Taylor approximation found in part (b) of this exercise. $901. 82 $902. 29 = $0. 47 (d) Comment on how the accuracy of the estimated price based on the first order Taylor approximation found in Exercise #2(b) compares with the estimated price based on the second order Taylor approximation found in part (b) of this exercise. The first order Taylor approximation is an underestimation of $7. 76, while the second order Taylor approximation is an overestimation of $0. 47.

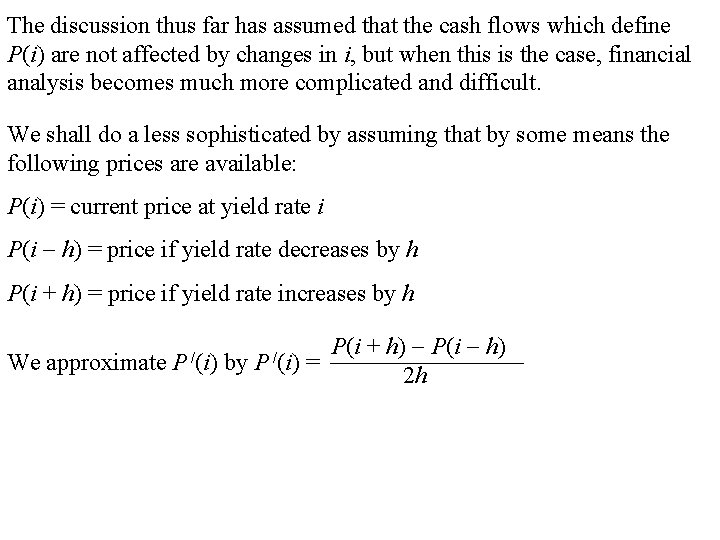
The discussion thus far has assumed that the cash flows which define P(i) are not affected by changes in i, but when this is the case, financial analysis becomes much more complicated and difficult. We shall do a less sophisticated by assuming that by some means the following prices are available: P(i) = current price at yield rate i P(i h) = price if yield rate decreases by h P(i + h) = price if yield rate increases by h We approximate P /(i) by P /(i) P(i + h) P(i h) = 2 h

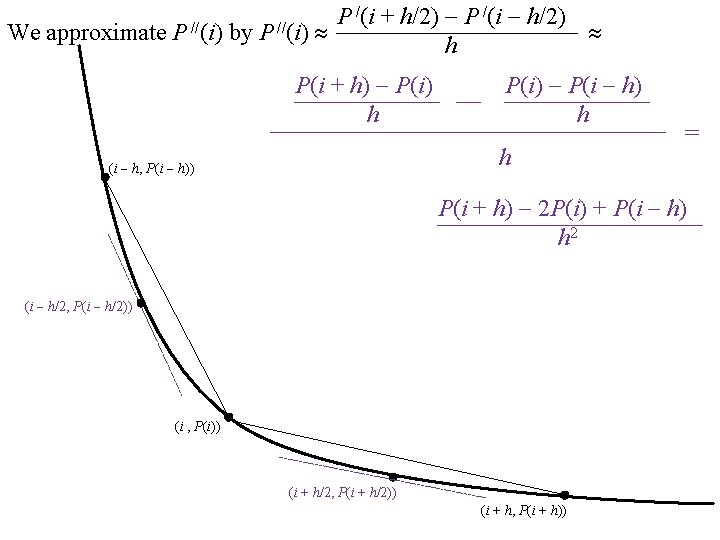
We approximate P /(i) by P /(i) P(i + h) P(i h) 2 h (i h, P(i h)) The slope of the tangent line at (i , P(i)) is approximated by the slope of a secant line (i , P(i)) (i + h, P(i + h))

/(i + h/2) P /(i h/2) P We approximate P //(i) by P //(i) h P(i + h) P(i) h P(i) P(i h) h h (i h, P(i h)) = P(i + h) 2 P(i) + P(i h) h 2 (i h/2, P(i h/2)) (i , P(i)) (i + h/2, P(i + h/2)) (i + h, P(i + h))

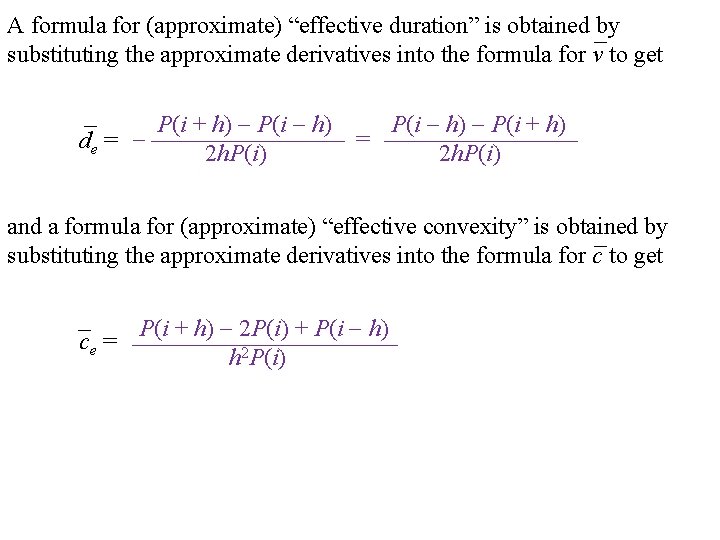
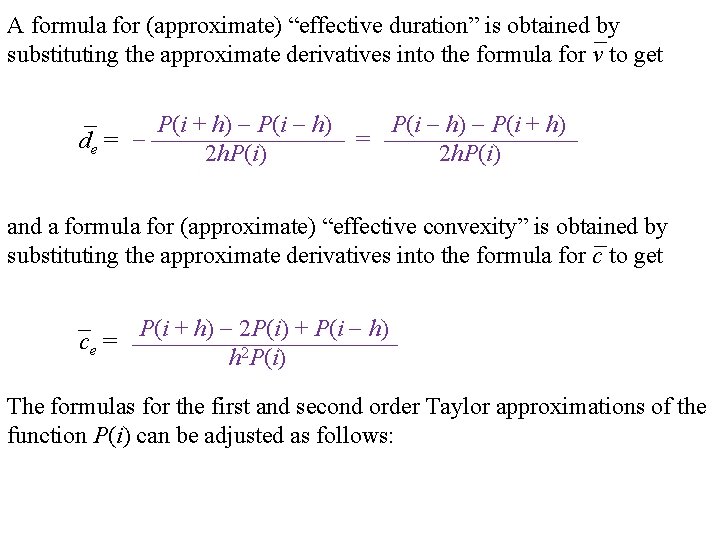
A formula for (approximate) “effective duration” is obtained by substituting the approximate derivatives into the formula for v to get P(i + h) P(i + h) de = = 2 h. P(i) and a formula for (approximate) “effective convexity” is obtained by substituting the approximate derivatives into the formula for c to get P(i + h) 2 P(i) + P(i h) ce = h 2 P(i)

Chapter 11 Exercises 5. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (a) Calculate the effective duration and effective convexity at i = 7% using h = 1%, and compare these values with the modified duration (volatility) and the convexity calculated in Exercise #4(a). We first need the obtain the following prices: 1 P(0. 07) = 70 a – + 1000 1. 0720 = $1000 as should be expected! 20| 0. 07 (and done in Exercise #2(a)) 1 = $1114. 70 –| 0. 06 + 1000 P(0. 06) = 70 a 20 1. 0620 –| 0. 08 P(0. 08) = 70 a 20 1 + 1000 1. 0820 = $901. 82 (as done in Exercise #2(b))

Chapter 11 Exercises 5. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (a) Calculate the effective duration and effective convexity at i = 7% using h = 1%, and compare these values with the modified duration (volatility) and the convexity calculated in Exercise #4(a). P(i h) P(i + h) P(0. 06) P(0. 08) de = = 2 h. P(i) 2(0. 01)P(0. 07) 1114. 70 901. 82 = 10. 644 2(0. 01)(1000) The effective duration is very close in value to the modified duration v = 10. 59401 calculated in Exercise #4(a).

Chapter 11 Exercises 5. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (a) Calculate the effective duration and effective convexity at i = 7% using h = 1%, and compare these values with the modified duration (volatility) and the convexity calculated in Exercise #4(a). + h) 2 P(i) + P(i h) ce = P(i = h 2 P(i) P(0. 08) 2 P(0. 07) + P(0. 06) = (0. 01)2 P(0. 07) 901. 82 2(1000) + 1114. 70 = (0. 01) 2(1000) 165. 2 The effective convexity is very close in value to the convexity c = 164. 6786 calculated in Exercise #4(a).

Chapter 11 Exercises 5. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (b) Suppose that the bond is callable at the end of 10 years at par, and that the bond will be called if the rate of interest falls but not if it rises. Calculate the effective duration and effective convexity at i = 7% using h = 1%, and compare these values with the modified duration (volatility) and the convexity calculated in Exercise part (a) of this exercise. Since the bond will be called only if the rate of interest falls, we need to adjust the prices accordingly: 1 = $1073. 60 –| 0. 06 + 1000 P(0. 06) = 70 a 10 1. 0610 P(0. 07) = $1000, since no adjustment is necessary P(0. 08) = $901. 82, since no adjustment is necessary

Chapter 11 Exercises 5. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (b) Suppose that the bond is callable at the end of 10 years at par, and that the bond will be called if the rate of interest falls but not if it rises. Calculate the effective duration and effective convexity at i = 7% using h = 1%, and compare these values with the modified duration (volatility) and the convexity calculated in Exercise part (a) of this exercise. P(0. 06) P(0. 08) de = = 2(0. 01)P(0. 07) 1073. 60 901. 82 = 8. 589 2(0. 01)(1000) The effective duration is very different from (smaller than) the value for duration calculated in part (a); the smaller value indicates the shortened duration resulting from the possibility that the bond is called early.

Chapter 11 Exercises 5. Consider again the 20 -year bond with a par value of $1000 and an annual coupon rate of 7% in Exercise #2, where the effective rate of interest is 7%. (b) Suppose that the bond is callable at the end of 10 years at par, and that the bond will be called if the rate of interest falls but not if it rises. Calculate the effective duration and effective convexity at i = 7% using h = 1%, and compare these values with the modified duration (volatility) and the convexity calculated in Exercise part (a) of this exercise. P(0. 08) 2 P(0. 07) + P(0. 06) ce = = (0. 01)2 P(0. 07) 901. 82 2(1000) + 1073. 60 = 245. 8 (0. 01) 2(1000) The effective convexity is very different from the value for the convexity calculated in part (a); effective convexity can be negative whereas convexity must be positive with non-interest sensitive cash flows.

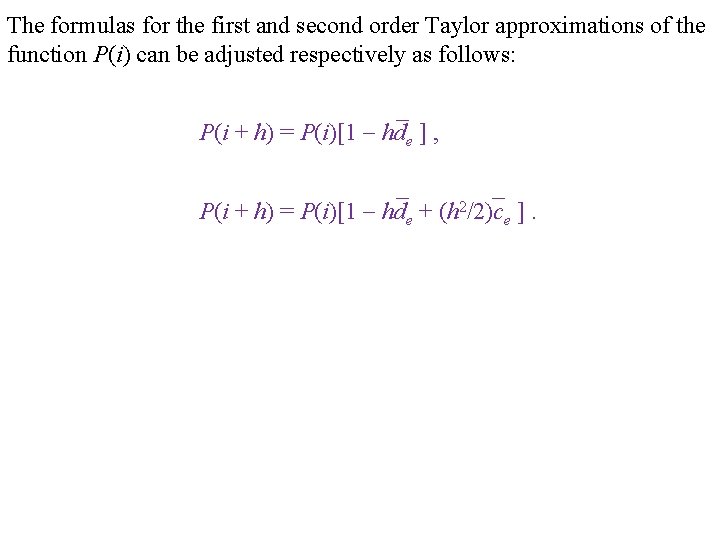
A formula for (approximate) “effective duration” is obtained by substituting the approximate derivatives into the formula for v to get P(i + h) P(i + h) de = = 2 h. P(i) and a formula for (approximate) “effective convexity” is obtained by substituting the approximate derivatives into the formula for c to get P(i + h) 2 P(i) + P(i h) ce = h 2 P(i) The formulas for the first and second order Taylor approximations of the function P(i) can be adjusted as follows:

The formulas for the first and second order Taylor approximations of the function P(i) can be adjusted respectively as follows: P(i + h) = P(i)[1 hde ] , P(i + h) = P(i)[1 hde + (h 2/2)ce ].