Section 8 8 Improper Integrals IMPROPER INTEGRALS OF

![DEFINITION (CONTINUED) (b) If f is continuous on (a, b] and is discontinuous at DEFINITION (CONTINUED) (b) If f is continuous on (a, b] and is discontinuous at](https://slidetodoc.com/presentation_image_h2/5b3acb2f257a05715d8999270ebb8271/image-9.jpg)
- Slides: 11

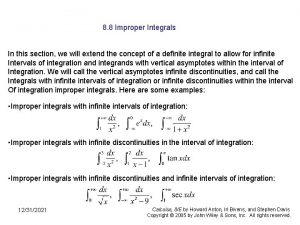
Section 8. 8 Improper Integrals

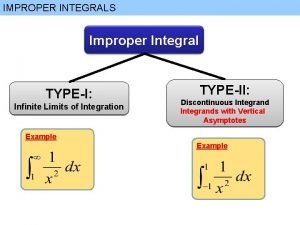
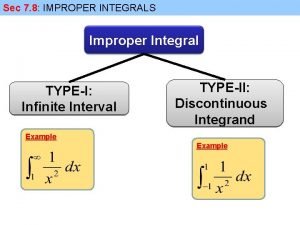
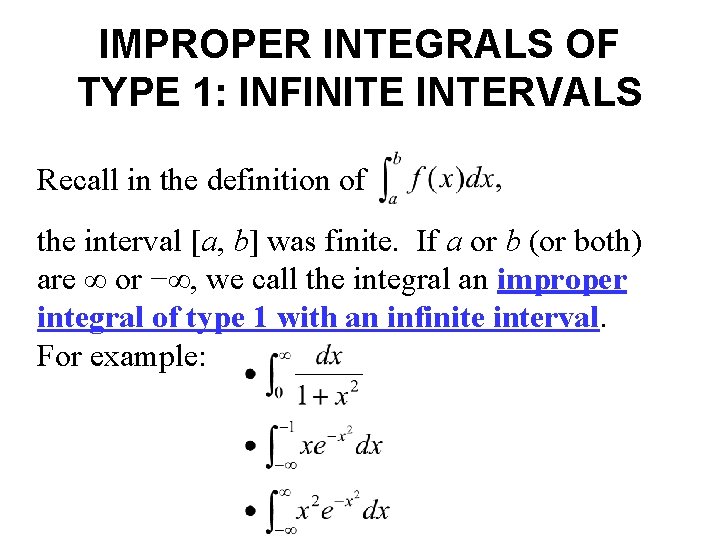
IMPROPER INTEGRALS OF TYPE 1: INFINITE INTERVALS Recall in the definition of the interval [a, b] was finite. If a or b (or both) are ∞ or −∞, we call the integral an improper integral of type 1 with an infinite interval. For example:

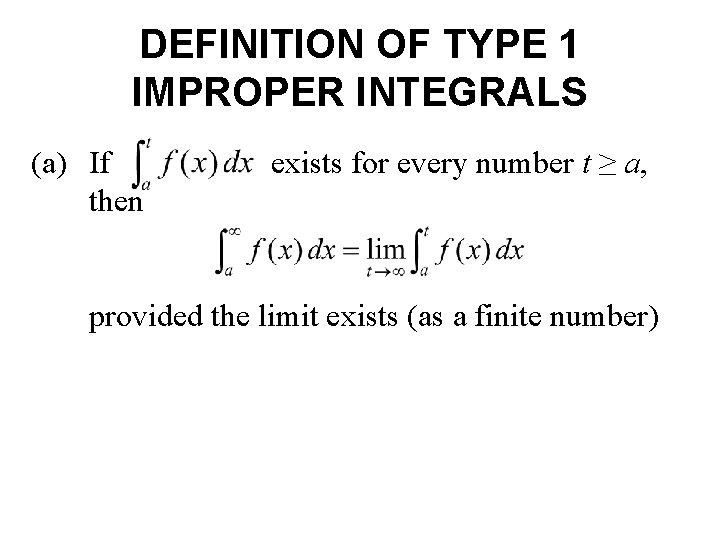
DEFINITION OF TYPE 1 IMPROPER INTEGRALS (a) If then exists for every number t ≥ a, provided the limit exists (as a finite number)

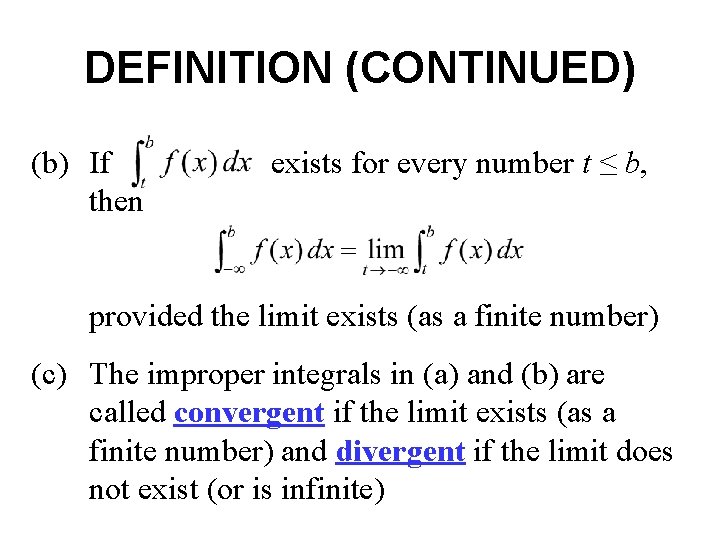
DEFINITION (CONTINUED) (b) If then exists for every number t ≤ b, provided the limit exists (as a finite number) (c) The improper integrals in (a) and (b) are called convergent if the limit exists (as a finite number) and divergent if the limit does not exist (or is infinite)

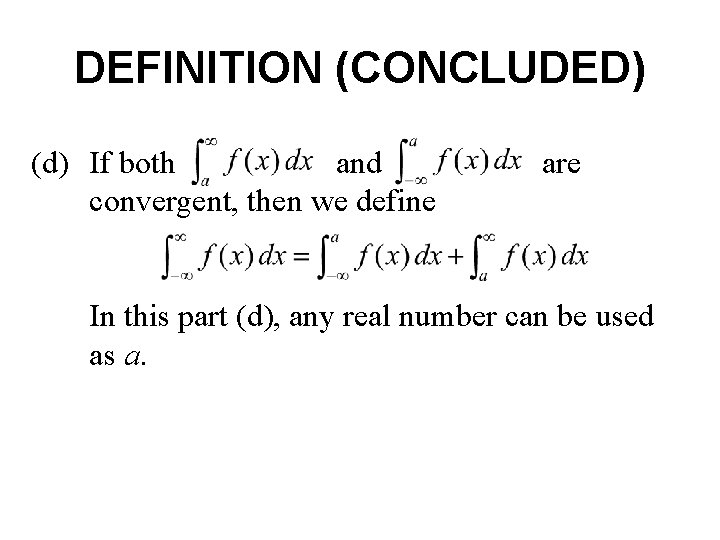
DEFINITION (CONCLUDED) (d) If both and convergent, then we define are In this part (d), any real number can be used as a.

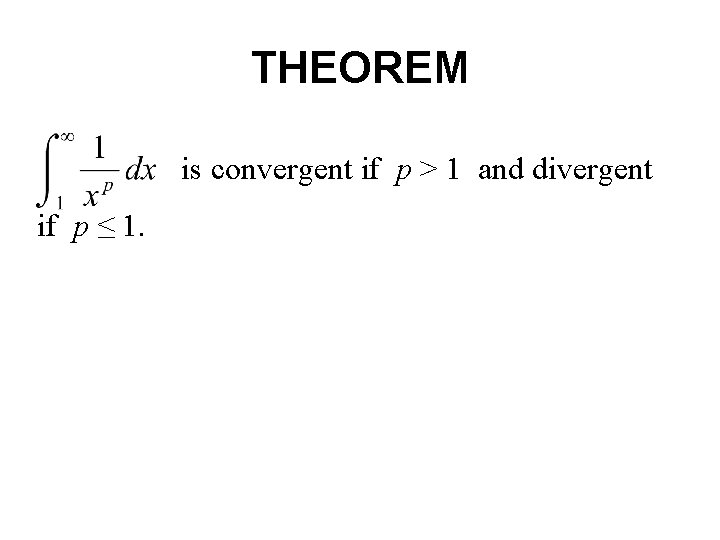
THEOREM is convergent if p > 1 and divergent if p ≤ 1.

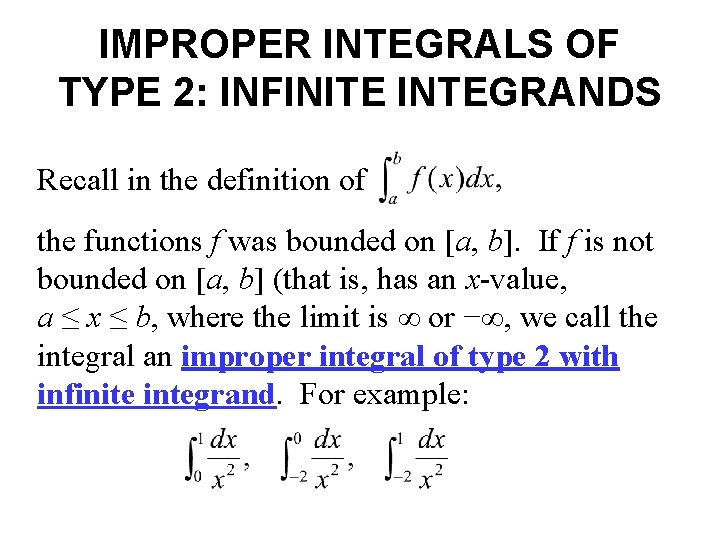
IMPROPER INTEGRALS OF TYPE 2: INFINITE INTEGRANDS Recall in the definition of the functions f was bounded on [a, b]. If f is not bounded on [a, b] (that is, has an x-value, a ≤ x ≤ b, where the limit is ∞ or −∞, we call the integral an improper integral of type 2 with infinite integrand. For example:

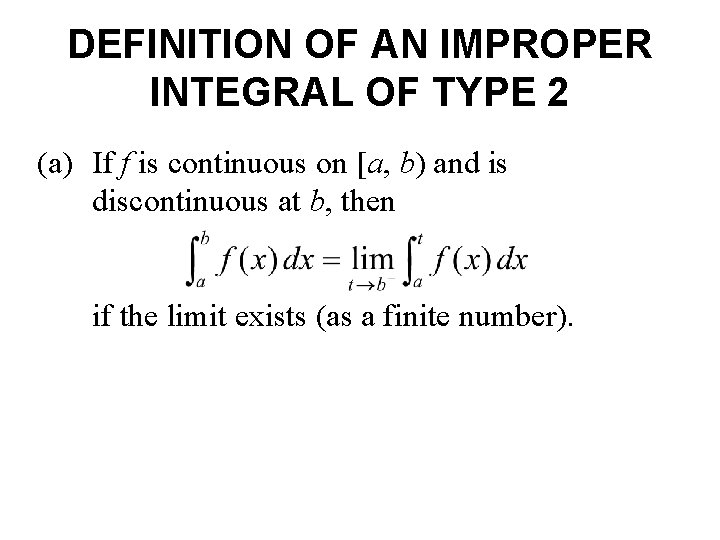
DEFINITION OF AN IMPROPER INTEGRAL OF TYPE 2 (a) If f is continuous on [a, b) and is discontinuous at b, then if the limit exists (as a finite number).
![DEFINITION CONTINUED b If f is continuous on a b and is discontinuous at DEFINITION (CONTINUED) (b) If f is continuous on (a, b] and is discontinuous at](https://slidetodoc.com/presentation_image_h2/5b3acb2f257a05715d8999270ebb8271/image-9.jpg)
DEFINITION (CONTINUED) (b) If f is continuous on (a, b] and is discontinuous at a, then if the limit exists (as a finite number). (c) The improper integrals in parts (a) and (b) are called convergent if the limits exits (as a finite number) and divergent if the limit does not exist (or is infinite).

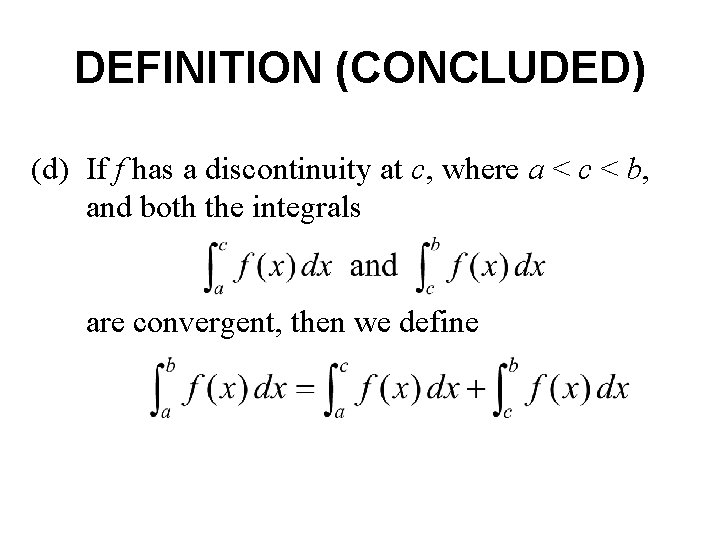
DEFINITION (CONCLUDED) (d) If f has a discontinuity at c, where a < c < b, and both the integrals are convergent, then we define

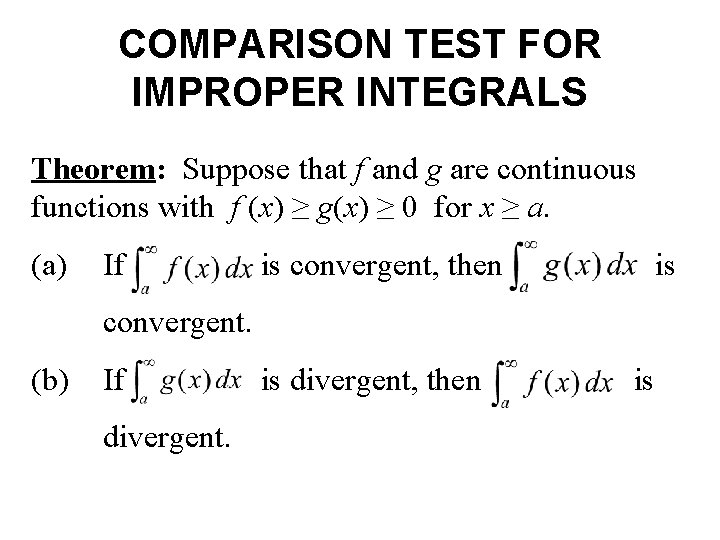
COMPARISON TEST FOR IMPROPER INTEGRALS Theorem: Suppose that f and g are continuous functions with f (x) ≥ g(x) ≥ 0 for x ≥ a. (a) If is convergent, then is convergent. (b) If divergent. is divergent, then is
 Improper integrals
Improper integrals Notasi integral
Notasi integral Introduction to double integrals
Introduction to double integrals Contoh soal integral permukaan
Contoh soal integral permukaan Integrate e
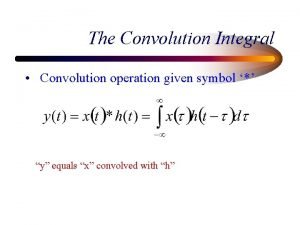
Integrate e Convolution integral example
Convolution integral example Polar integrals
Polar integrals Application of residue theorem to evaluate real integrals
Application of residue theorem to evaluate real integrals How to find the area of a curve
How to find the area of a curve Properties of double integrals
Properties of double integrals Convolution integrals
Convolution integrals Double integrals over rectangles
Double integrals over rectangles