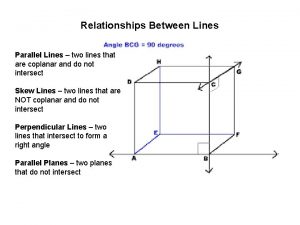
Section 3 2 Properties of Parallel Lines Calculations

Section 3 -2 Properties of Parallel Lines, Calculations. Michael Schuetz

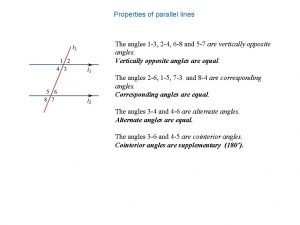
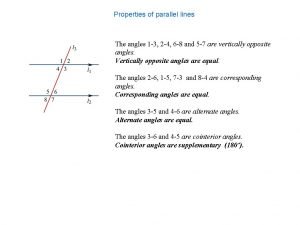
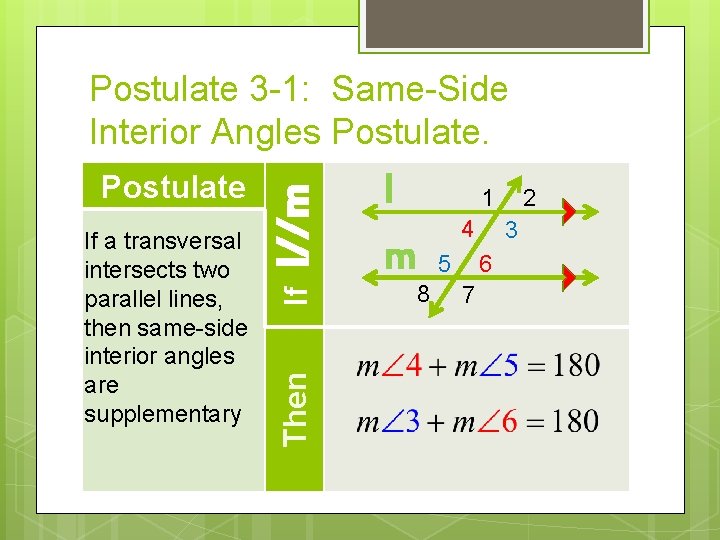
If If a transversal intersects two parallel lines, then same-side interior angles are supplementary Then Postulate l//m Postulate 3 -1: Same-Side Interior Angles Postulate. l m 8 1 4 5 3 6 7 2

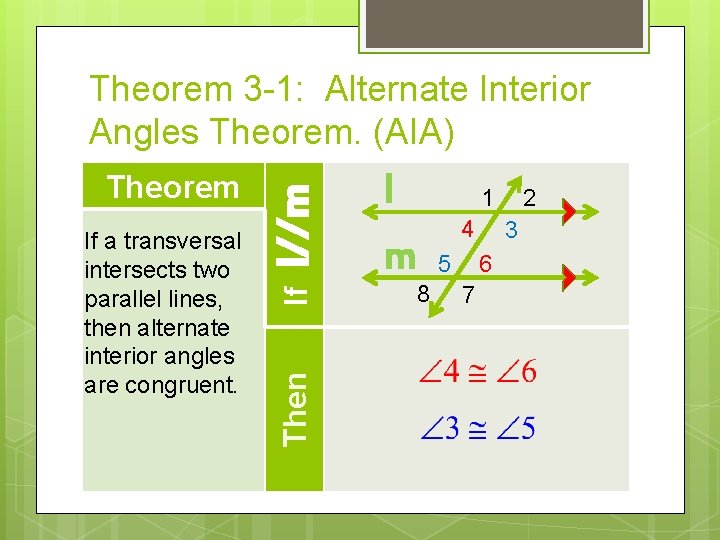
If If a transversal intersects two parallel lines, then alternate interior angles are congruent. Then Theorem l//m Theorem 3 -1: Alternate Interior Angles Theorem. (AIA) l m 8 1 4 5 3 6 7 2

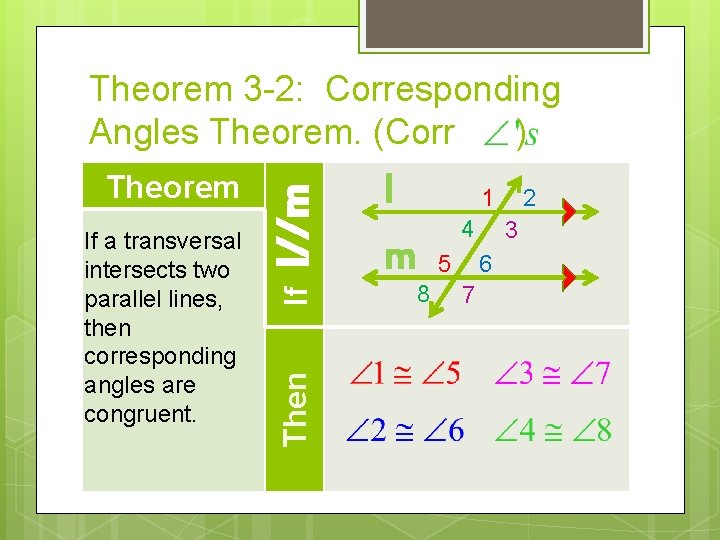
If If a transversal intersects two parallel lines, then corresponding angles are congruent. Then Theorem l//m Theorem 3 -2: Corresponding Angles Theorem. (Corr ) l m 8 1 4 5 3 6 7 2

If If a transversal intersects two parallel lines, then Alternate exterior angles are congruent. Then Theorem l//m Theorem 3 -3: Alternate Exterior Angles Theorem. (AEA) l m 8 1 4 5 3 6 7 2

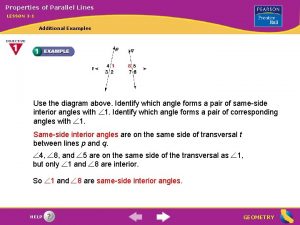
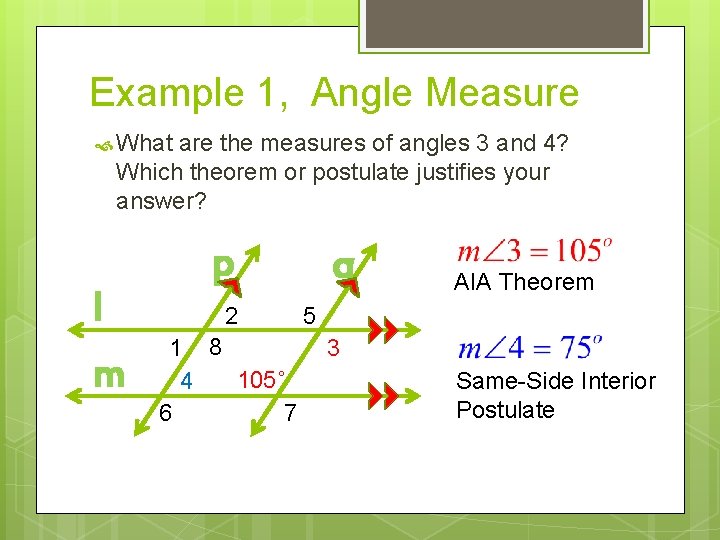
Example 1, Angle Measure What are the measures of angles 3 and 4? Which theorem or postulate justifies your answer? l m p 2 1 8 105˚ 4 6 7 q AIA Theorem 5 3 Same-Side Interior Postulate

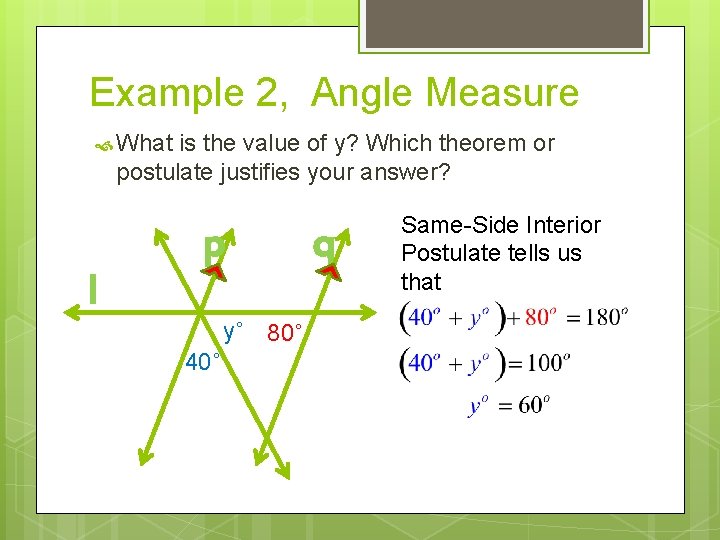
Example 2, Angle Measure What is the value of y? Which theorem or postulate justifies your answer? l p y˚ 40˚ q 80˚ Same-Side Interior Postulate tells us that

Classwork: P. 153, #’s 7, 12, 15, 16, 19, 20

Section 3 -2 Properties of Parallel Lines, Proofs.

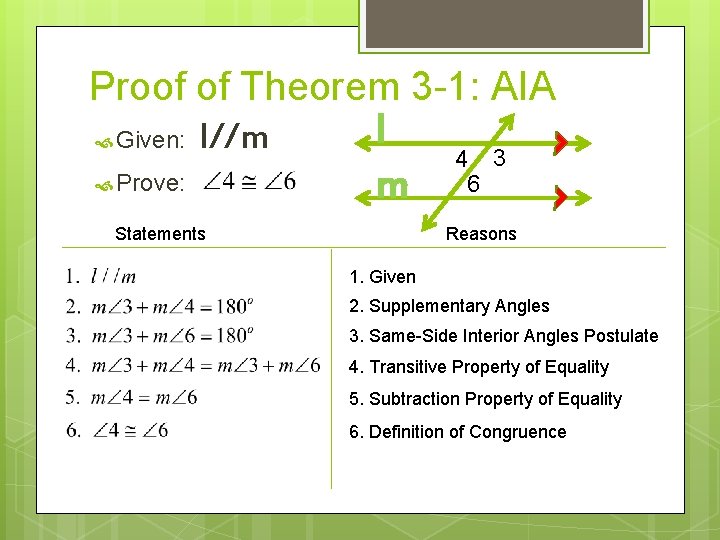
Proof of Theorem 3 -1: AIA l Given: l//m 4 3 Prove: m Statements 6 Reasons 1. Given 2. Supplementary Angles 3. Same-Side Interior Angles Postulate 4. Transitive Property of Equality 5. Subtraction Property of Equality 6. Definition of Congruence

Proving an angle relationship 1 Given: l//m l m Prove: are supplementary 8 Statements 5 7 Reasons 1. Given 2. If lines are //, then Corr 3. 4. have equal measure. that form a linear pair are suppl. 5. Def. of suppl. 6. Substitution Property 7. Def. of suppl.

Homework: P. 153, #’s 10, 11, 25, 26.