Section 08 Joint Marginal and Conditional Distributions Joint

Section 08 Joint, Marginal, and Conditional Distributions

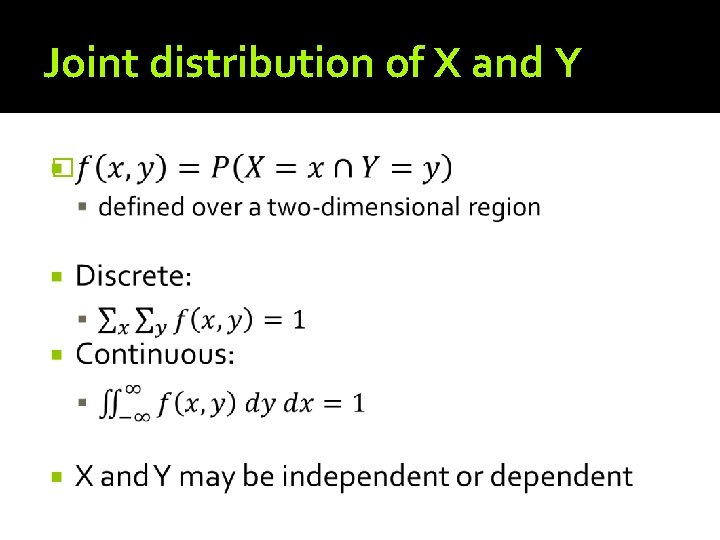
Joint distribution of X and Y �

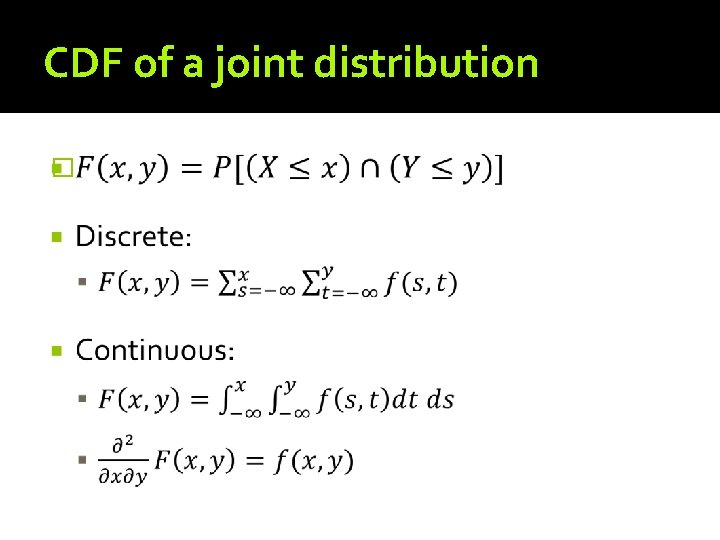
CDF of a joint distribution �

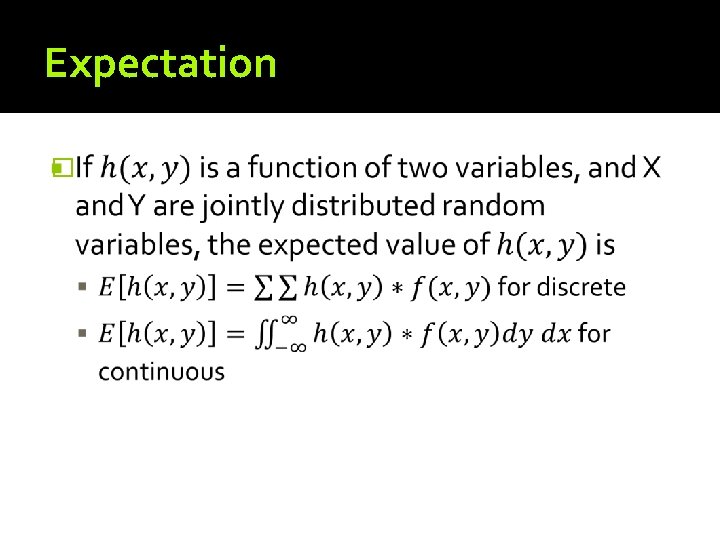
Expectation �

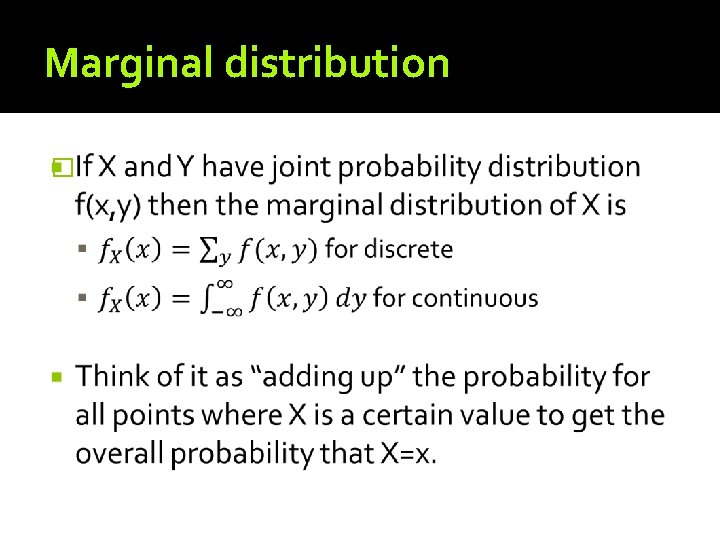
Marginal distribution �

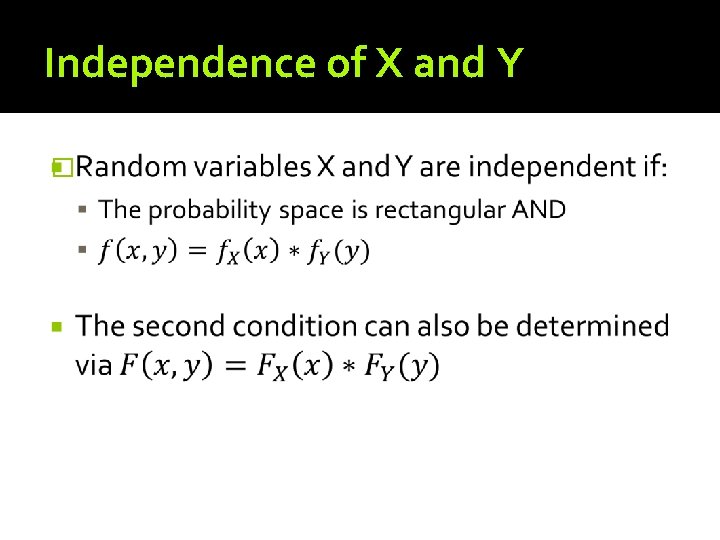
Independence of X and Y �

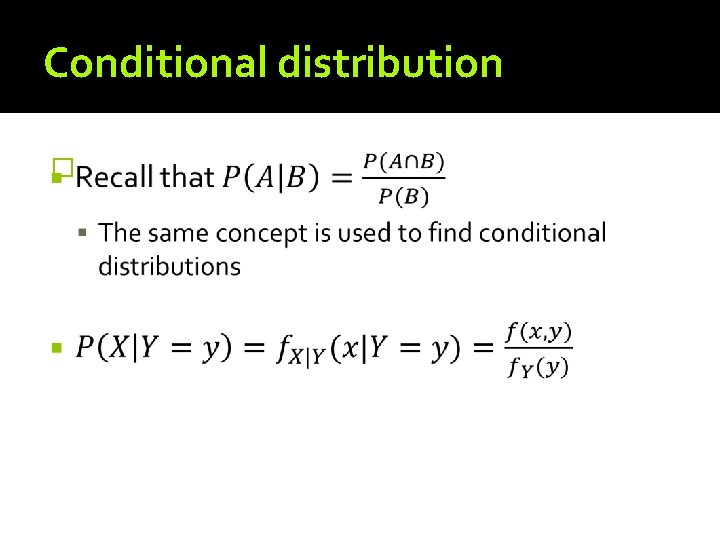
Conditional distribution �

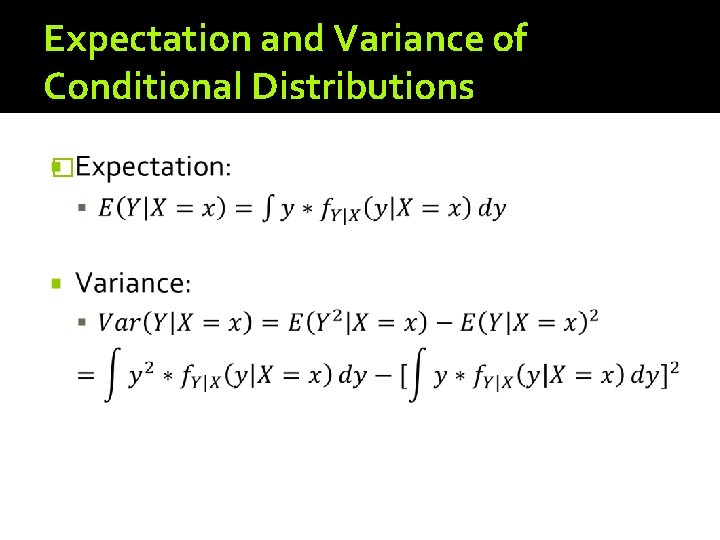
Expectation and Variance of Conditional Distributions �

Covariance �

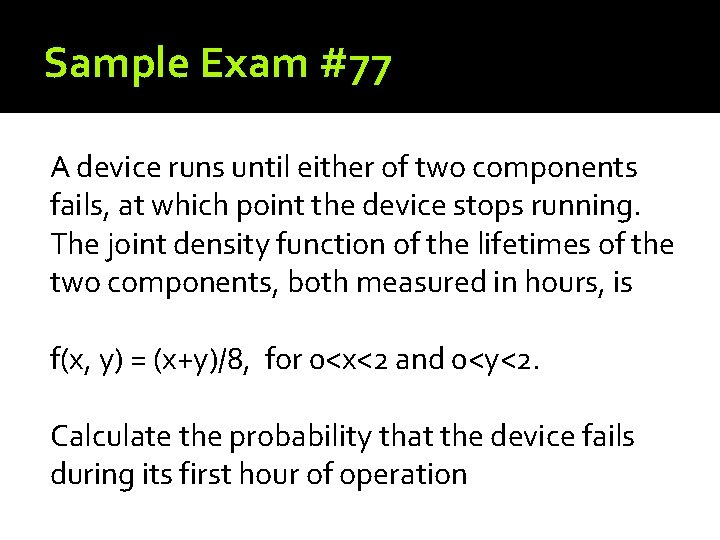
Sample Exam #77 A device runs until either of two components fails, at which point the device stops running. The joint density function of the lifetimes of the two components, both measured in hours, is f(x, y) = (x+y)/8, for 0<x<2 and 0<y<2. Calculate the probability that the device fails during its first hour of operation

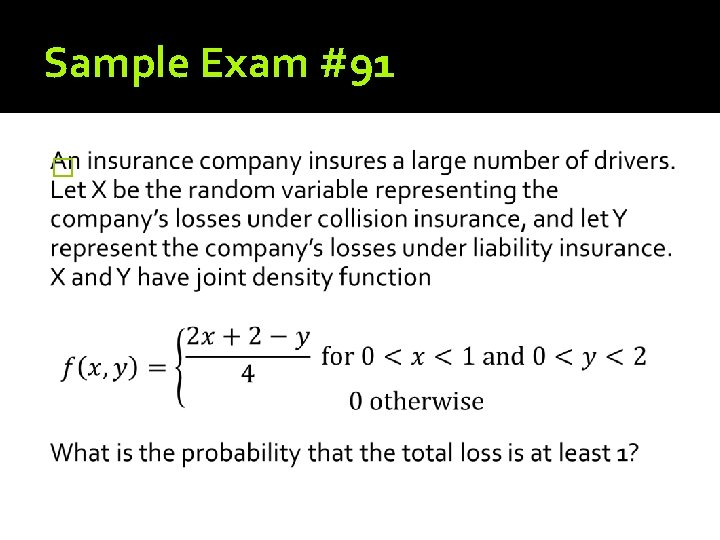
Sample Exam #91 �

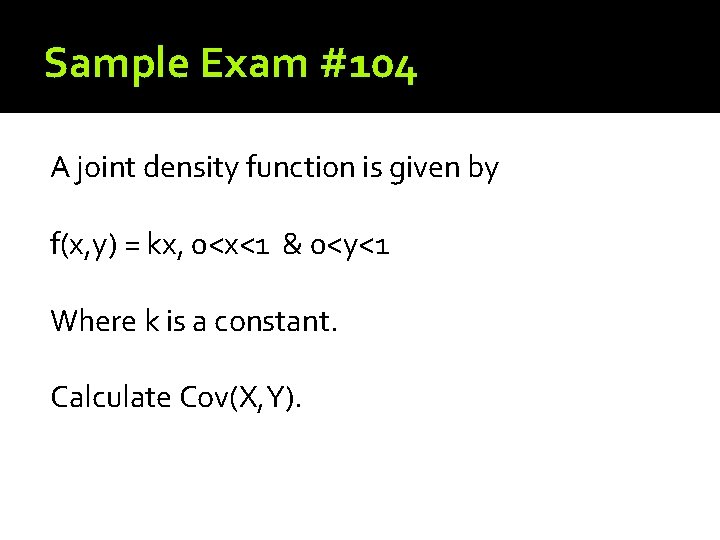
Sample Exam #104 A joint density function is given by f(x, y) = kx, 0<x<1 & 0<y<1 Where k is a constant. Calculate Cov(X, Y).

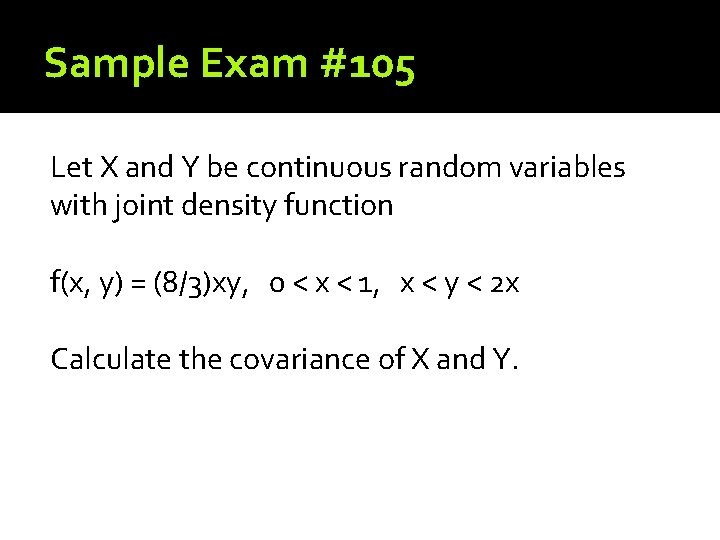
Sample Exam #105 Let X and Y be continuous random variables with joint density function f(x, y) = (8/3)xy, 0 < x < 1, x < y < 2 x Calculate the covariance of X and Y.

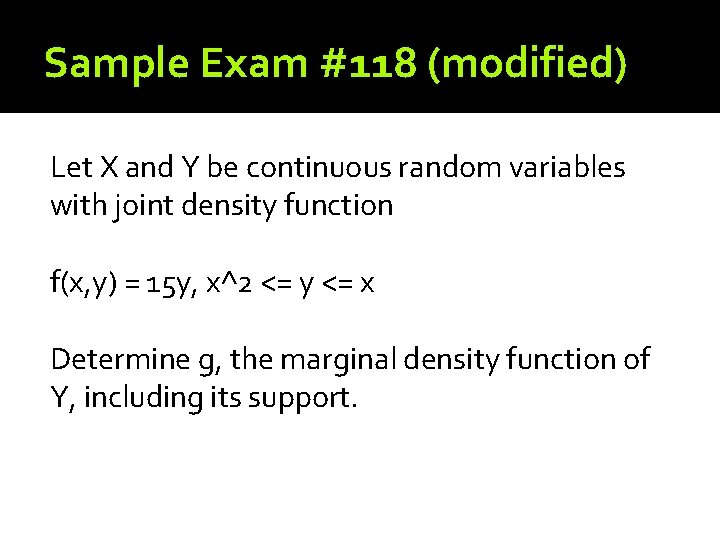
Sample Exam #118 (modified) Let X and Y be continuous random variables with joint density function f(x, y) = 15 y, x^2 <= y <= x Determine g, the marginal density function of Y, including its support.

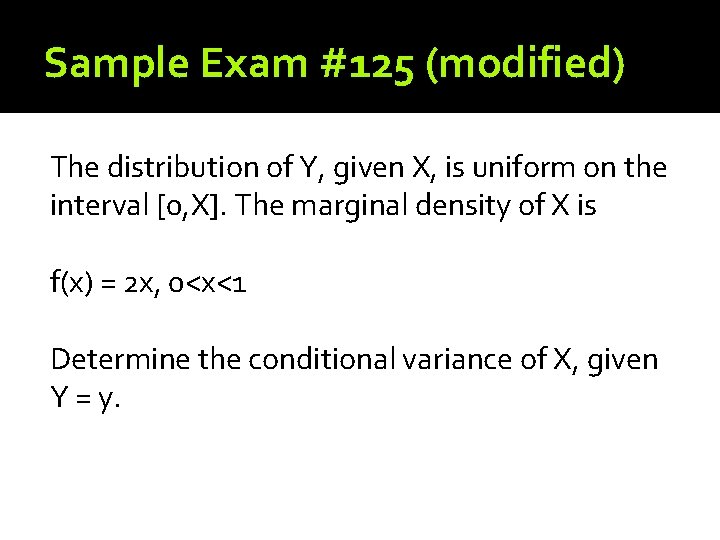
Sample Exam #125 (modified) The distribution of Y, given X, is uniform on the interval [0, X]. The marginal density of X is f(x) = 2 x, 0<x<1 Determine the conditional variance of X, given Y = y.

Sample Exam #160 An actuary analyzes a company’s annual personal auto claims, M, and annual commercial auto claims, N. The analysis reveals that Var(M) = 1600, Var(N) = 900, and the correlation between M and N is 0. 64. Calculate Var(M+N).

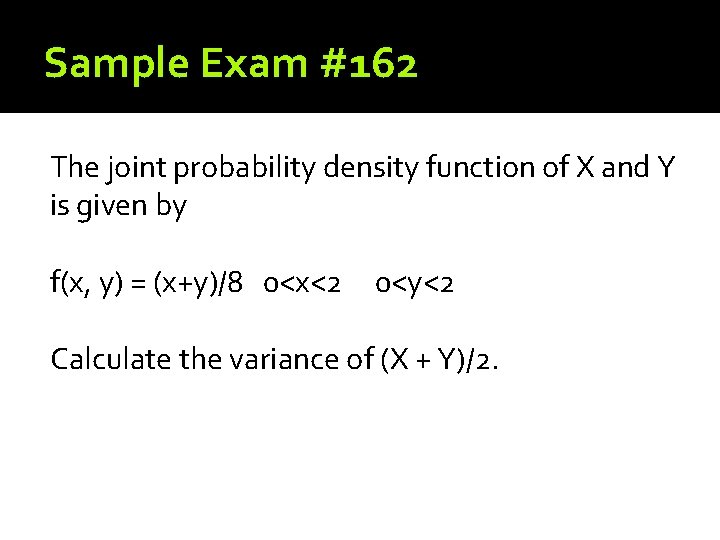
Sample Exam #162 The joint probability density function of X and Y is given by f(x, y) = (x+y)/8 0<x<2 0<y<2 Calculate the variance of (X + Y)/2.

Sample Exam # 172 A policyholder has probability 0. 7 of having no claims, 0. 2 of having exactly one claim, and 0. 1 of having exactly two claims. Claim amounts are uniformly distributed on the interval [0, 60] and are independent. The insurer covers 100% of each claim. Calculate the probability that the total benefit paid to the policyholder is 48 or less.

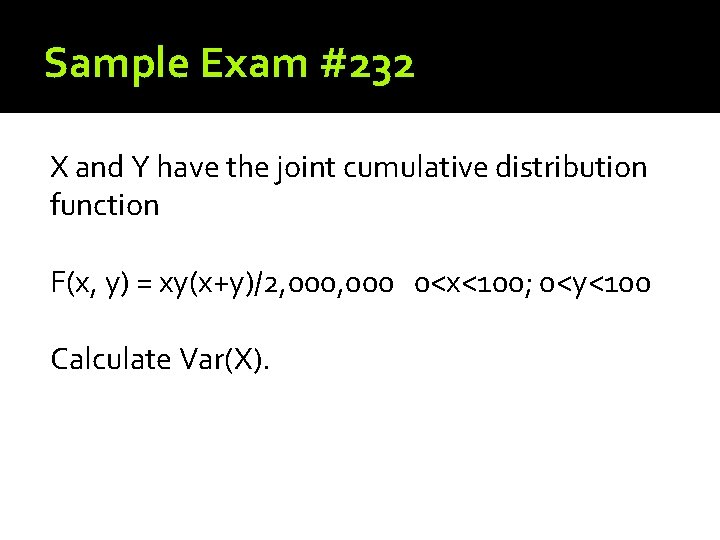
Sample Exam #232 X and Y have the joint cumulative distribution function F(x, y) = xy(x+y)/2, 000 0<x<100; 0<y<100 Calculate Var(X).

Sample Exam # 309 A city with borders forming a square with sides of length 1 has its city hall located at the origin when a rectangular coordinate system is imposed on the city so that two sides of the square on the positive axes. The density function of the population is f(x, y) = 1. 5(x^2 + y^2), 0<x, y<1 A resident of the city can travel to the city hall only along a route whose segments are parallel to the city borders. Calculate the expected value of the travel distance to the city hall of a randomly chosen resident of the city.