Regents Physics Unit VI Work Power Energy PART














- Slides: 22

Regents Physics Unit VI: Work, Power & Energy

PART I: WORK

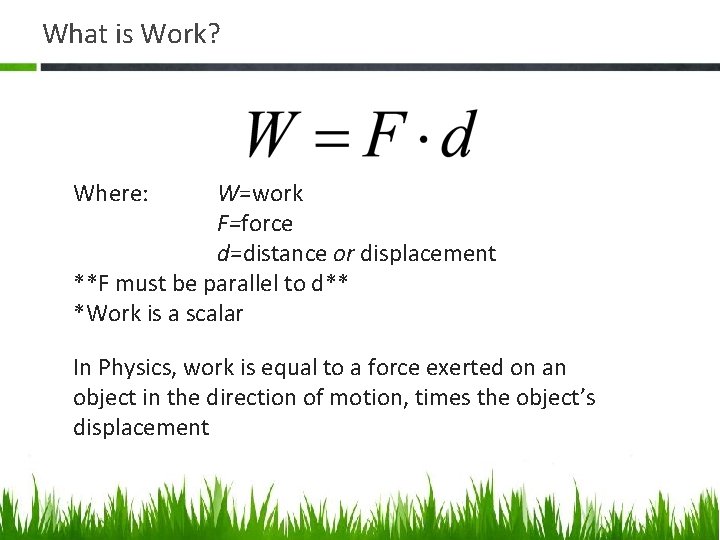
What is Work? Where: W=work F=force d=distance or displacement **F must be parallel to d** *Work is a scalar In Physics, work is equal to a force exerted on an object in the direction of motion, times the object’s displacement

Work F d

“Spinning Your Wheels…” • In other words, if the object doesn’t move, you do no work.


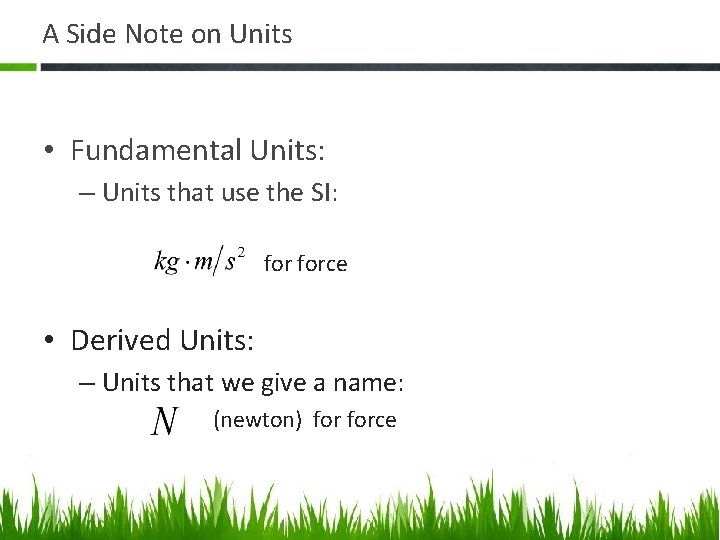
A Side Note on Units • Fundamental Units: – Units that use the SI: • F force • Derived Units: – Units that we give a name: (newton) force

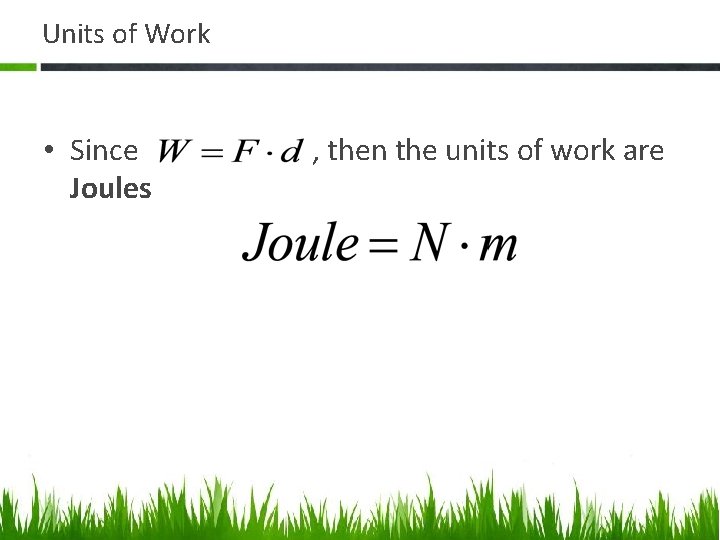
Units of Work • Since Joules , then the units of work are

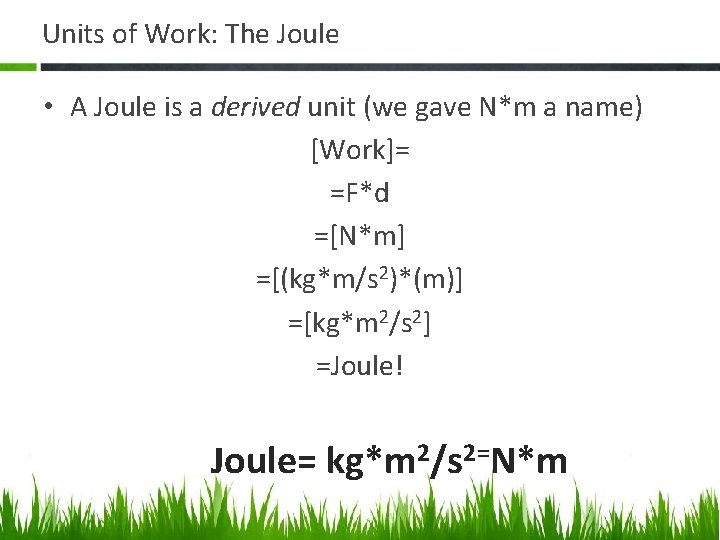
Units of Work: The Joule • A Joule is a derived unit (we gave N*m a name) [Work]= =F*d =[N*m] =[(kg*m/s 2)*(m)] =[kg*m 2/s 2] =Joule! Joule= kg*m 2/s 2=N*m

What is a Joule? • The Joule (SI units) is equivalent to the Calorie (pre-SI units). – *There are kilocalories (capital C) and calories (lower case c). • You intake “Calories” every day. Without eating you would be weak and you wouldn’t have much energy… • It turns out that the Joule(Calorie/calorie) is a unit of Energy.

What is a Joule? (Cont’d) • One “food” Calorie = 4, 180 Joules - A Snickers bar has about 280 Calories, or 1, 170, 400 Joules. - So…Doing “Work” on something changes its total energy. - We’ll come back to this later.

Work: An example problem A 105 kg hockey puck is sliding across the ice. A player exerts a constant 4. 5 N force over a distance of 0. 150 m. How much work does the player do on the puck?

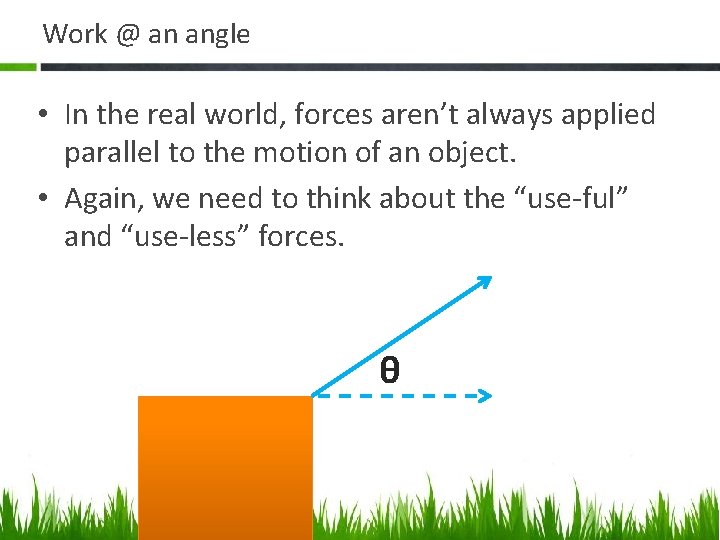
Work @ an angle • In the real world, forces aren’t always applied parallel to the motion of an object. • Again, we need to think about the “use-ful” and “use-less” forces. θ

Work @ an angle (cont’d) • Formulas: – You can derive formulas each time using SOH-CAHTOA – Or you can memorize these: • For “Horizontal Work”, • For “Vertical Work”, – These formulas work if the Θ is the angle between the force and the horizontal. – *Hint: You might want to write these down here:

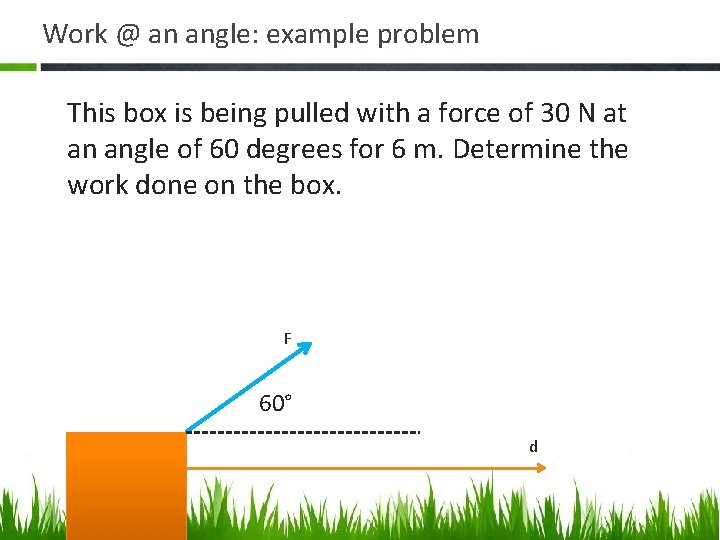
Work @ an angle: example problem This box is being pulled with a force of 30 N at an angle of 60 degrees for 6 m. Determine the work done on the box. F 60° d

PART II: POWER

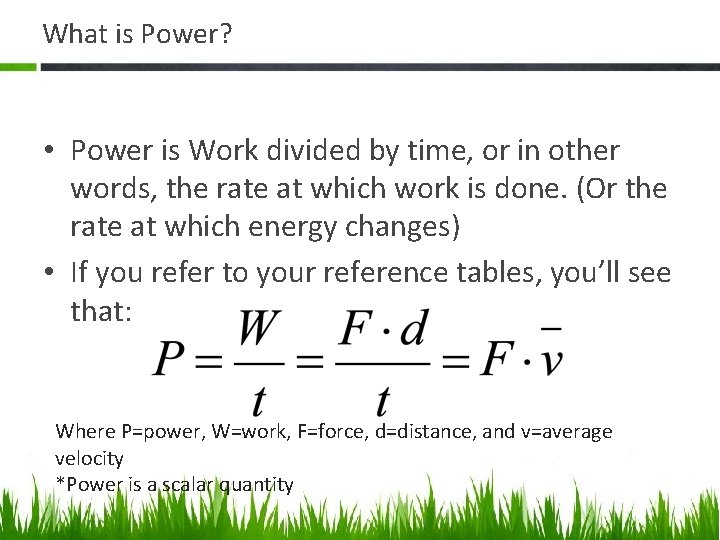
What is Power? • Power is Work divided by time, or in other words, the rate at which work is done. (Or the rate at which energy changes) • If you refer to your reference tables, you’ll see that: Where P=power, W=work, F=force, d=distance, and v=average velocity *Power is a scalar quantity


Units of Power • The unit of Power is the Watt. – We use the letter W to represent the unit Watt (don’t confuse it with W for work on the reference tables) Watt=Joules/seconds Watt? Don’t worry, Joule get it in a second!

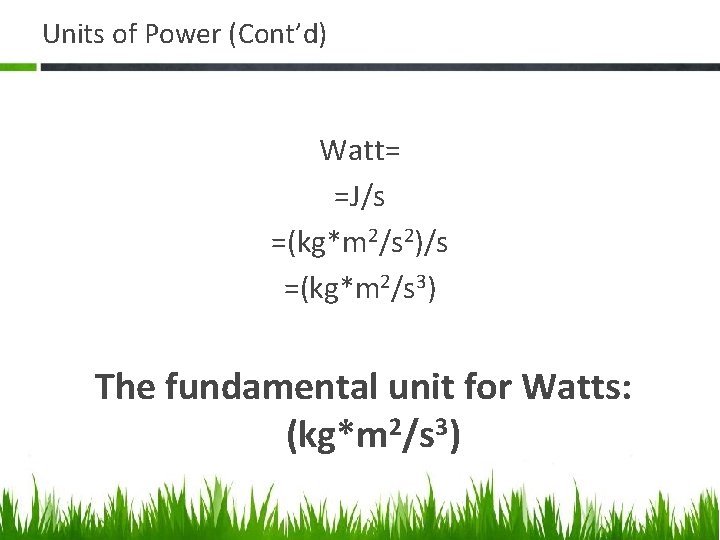
Units of Power (Cont’d) Watt= =J/s =(kg*m 2/s 2)/s =(kg*m 2/s 3) The fundamental unit for Watts: (kg*m 2/s 3)

Power Example Problem: • When Justin Beiber lifts his love child, 1400 J of work is done in 2. 5 s. Calculate how much power Justin develops.

Another Power Example Problem… • Ndamukong Suh stomps on a player with a force of 750 N at an angle of 45 degrees. He pushes the player a distance of 1. 5 m in. 5 seconds. How much power did he deliver to the player?