Proportionality Mc GrawHill Proportionality Ratio and proportion Direct

Proportionality © Mc. Graw-Hill

Proportionality Ratio and proportion Direct proportionality Percentages Increases and decreases Directly proportional divisions Inverse proportionality Inversely proportional divisions

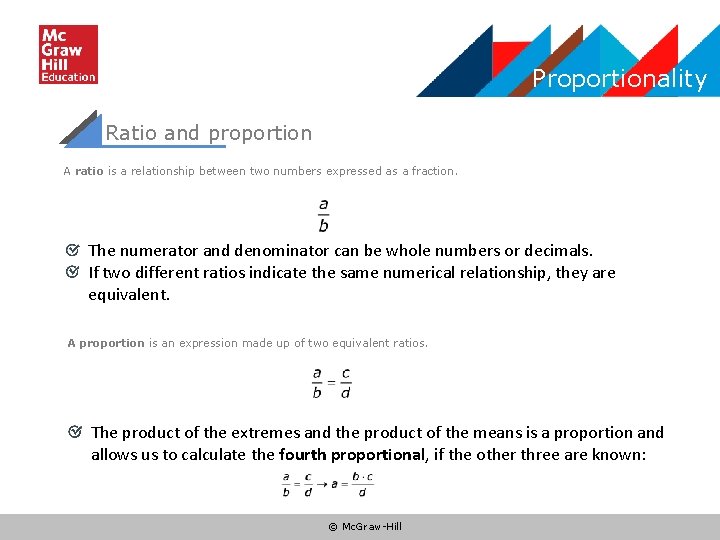
Proportionality Ratio and proportion A ratio is a relationship between two numbers expressed as a fraction. The numerator and denominator can be whole numbers or decimals. If two different ratios indicate the same numerical relationship, they are equivalent. A proportion is an expression made up of two equivalent ratios. The product of the extremes and the product of the means is a proportion and allows us to calculate the fourth proportional, if the other three are known: © Mc. Graw-Hill

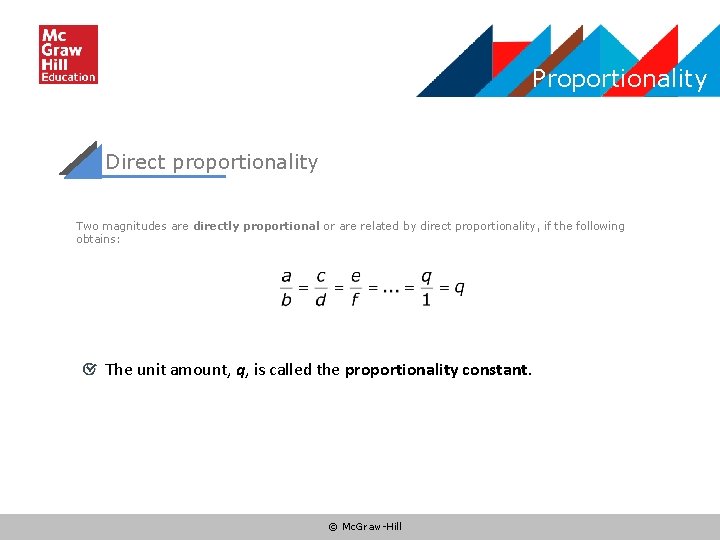
Proportionality Direct proportionality Two magnitudes are directly proportional or are related by direct proportionality, if the following obtains: The unit amount, q, is called the proportionality constant. © Mc. Graw-Hill

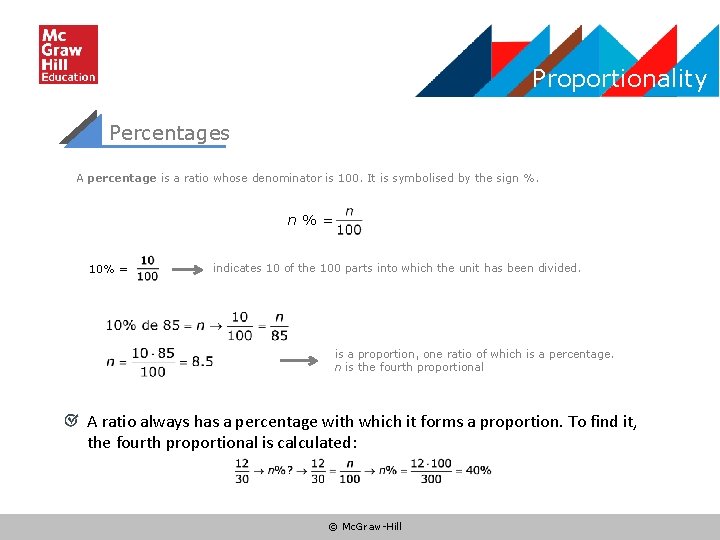
Proportionality Percentages A percentage is a ratio whose denominator is 100. It is symbolised by the sign %. n%= 10% = indicates 10 of the 100 parts into which the unit has been divided. is a proportion, one ratio of which is a percentage. n is the fourth proportional A ratio always has a percentage with which it forms a proportion. To find it, the fourth proportional is calculated: © Mc. Graw-Hill

Proportionality Increases and decreases Decrease Increase This is the decrease of a numerical value. An amount is subtracted from the initial value which is calculated by applying the percentage decrease of this value. This is the increase in a numerical value. An amount is added to the initial value which is calculated by applying the percentage increase of this value. 2% increase of € 90: 90 + 2% of 90 = 90 – = 90 + 1. 8 = € 91. 8 10% decrease of € 6. 50: 6. 5 – 10% of 6. 5 = 6. 5 – 0. 65 = € 5. 85 The information to explain what increase or decrease is applied is usually expressed by a percentage: - The CPI from June 2015 in Catalonia indicates that prices rose an average of 1. 1% since the start of the year. - Discounts of 50% are offered during the sales season. © Mc. Graw-Hill

Proportionality Directly proportional divisions This consists of dividing an amount (a) into directly proportion parts in specific values (x, y, z) to get the amounts b, c and d, by the expression: To calculate the amounts b, c and d resulting from a directly proportional division, the corresponding fourth proportional is calculated in each case: © Mc. Graw-Hill

Proportionality Inverse proportionality Two magnitudes a and b are inversely proportional if when one increases the other decreases in the same proportion, and therefore it occurs when: a·b=k For example: The distance between two objects is 63 m. A robot can travel this distance at the constant speed at which it is programmed. The quicker it goes, the less time it will need, and the slower it goes, the most time it will need. The constant speed of the robot and the time it needs to travel 63 m are inversely proportional magnitudes because when one of the two magnitudes is multiplied by a given value the other is divided by the same value. The product of two pairs of values of two inversely proportional magnitudes is constant (k). © Mc. Graw-Hill

Proportionality Inversely proportional divisions This consists of dividing an amount a into two parts b and c, which are inversely proportional to the values x and y. Calculating the amounts b and c resulting from an inversely proportional division is the same as calculating the directly proportional division of © Mc. Graw-Hill and