Population Dynamics Application of Eigenvalues Eigenvectors Consider the

Population Dynamics Application of Eigenvalues & Eigenvectors

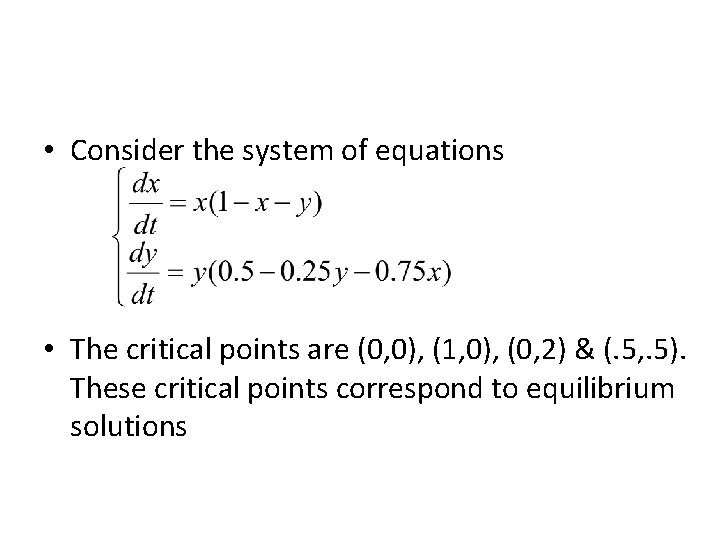
• Consider the system of equations • The critical points are (0, 0), (1, 0), (0, 2) & (. 5, . 5). These critical points correspond to equilibrium solutions

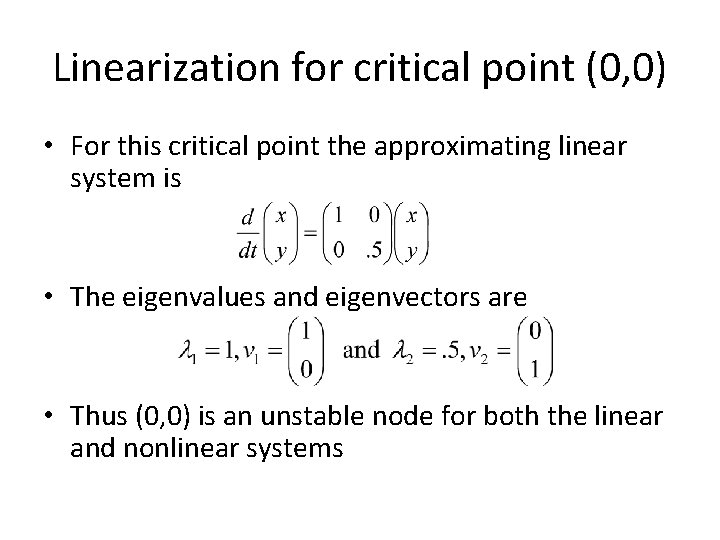
Linearization for critical point (0, 0) • For this critical point the approximating linear system is • The eigenvalues and eigenvectors are • Thus (0, 0) is an unstable node for both the linear and nonlinear systems

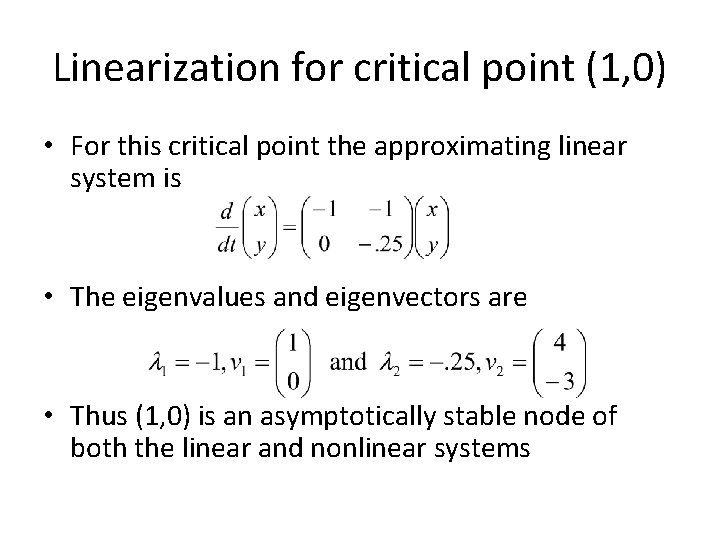
Linearization for critical point (1, 0) • For this critical point the approximating linear system is • The eigenvalues and eigenvectors are • Thus (1, 0) is an asymptotically stable node of both the linear and nonlinear systems

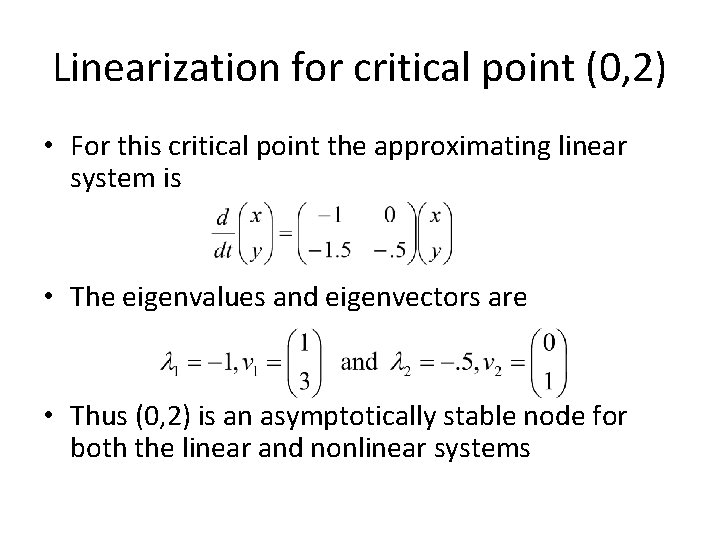
Linearization for critical point (0, 2) • For this critical point the approximating linear system is • The eigenvalues and eigenvectors are • Thus (0, 2) is an asymptotically stable node for both the linear and nonlinear systems

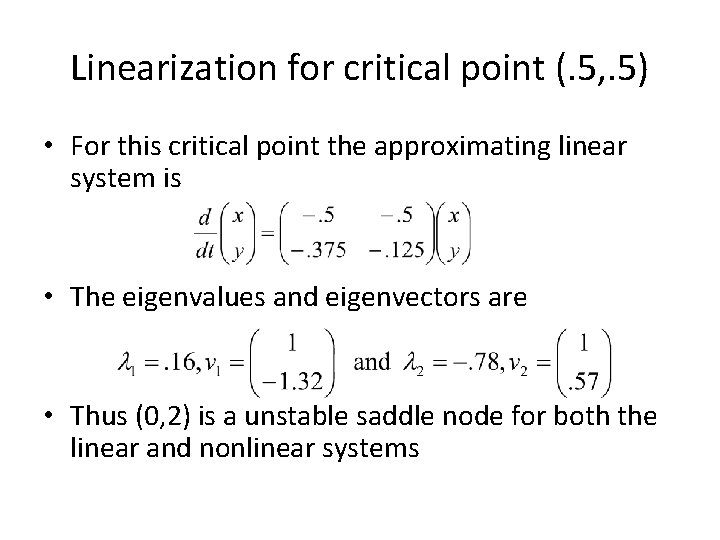
Linearization for critical point (. 5, . 5) • For this critical point the approximating linear system is • The eigenvalues and eigenvectors are • Thus (0, 2) is a unstable saddle node for both the linear and nonlinear systems

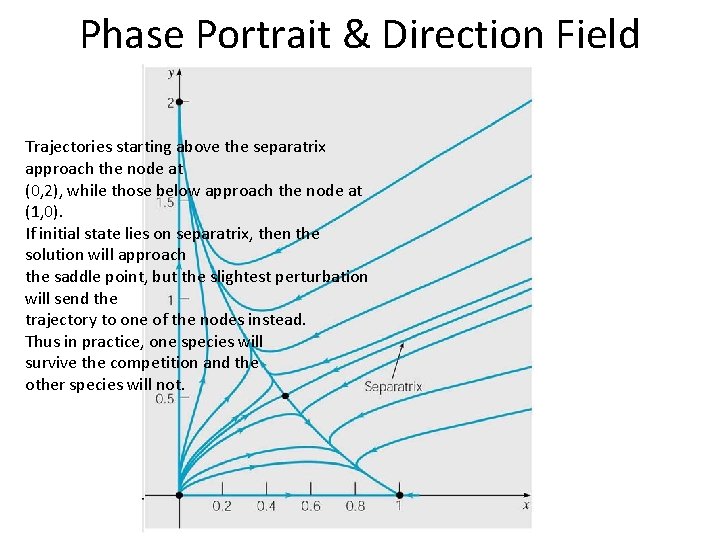
Phase Portrait & Direction Field Trajectories starting above the separatrix approach the node at (0, 2), while those below approach the node at (1, 0). If initial state lies on separatrix, then the solution will approach the saddle point, but the slightest perturbation will send the trajectory to one of the nodes instead. Thus in practice, one species will survive the competition and the other species will not.