Outline of the class 1 Vector calculus Vector
![Partial differential equations • For a homogeneous linear problem L[u(r)] = 0 if u Partial differential equations • For a homogeneous linear problem L[u(r)] = 0 if u](https://slidetodoc.com/presentation_image_h/141520fa639d10d32149095315d24021/image-5.jpg)








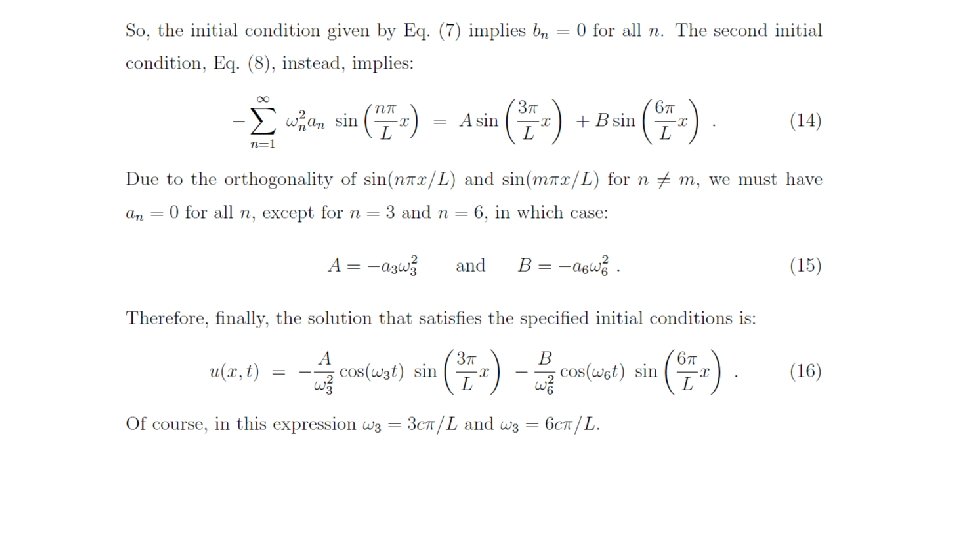



- Slides: 30

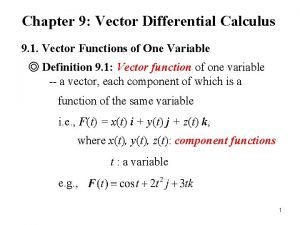
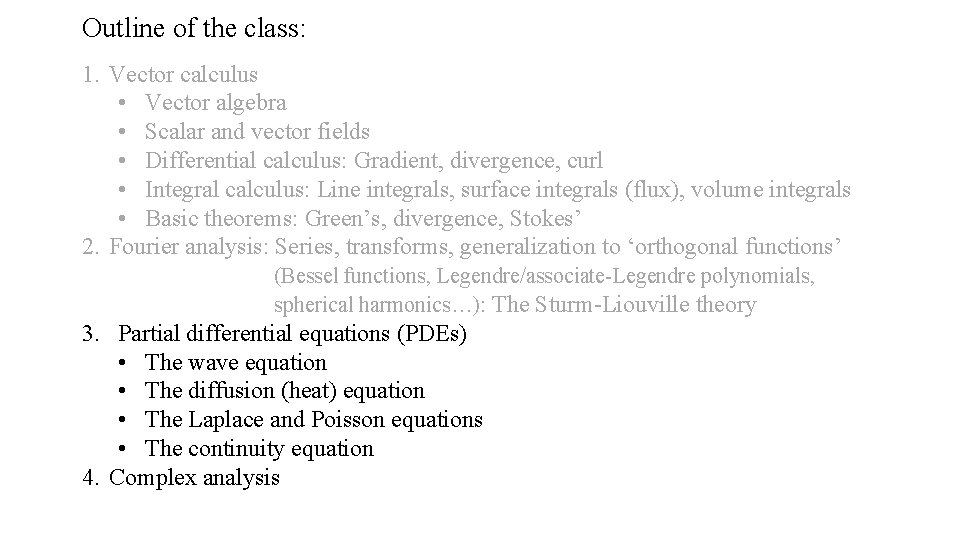
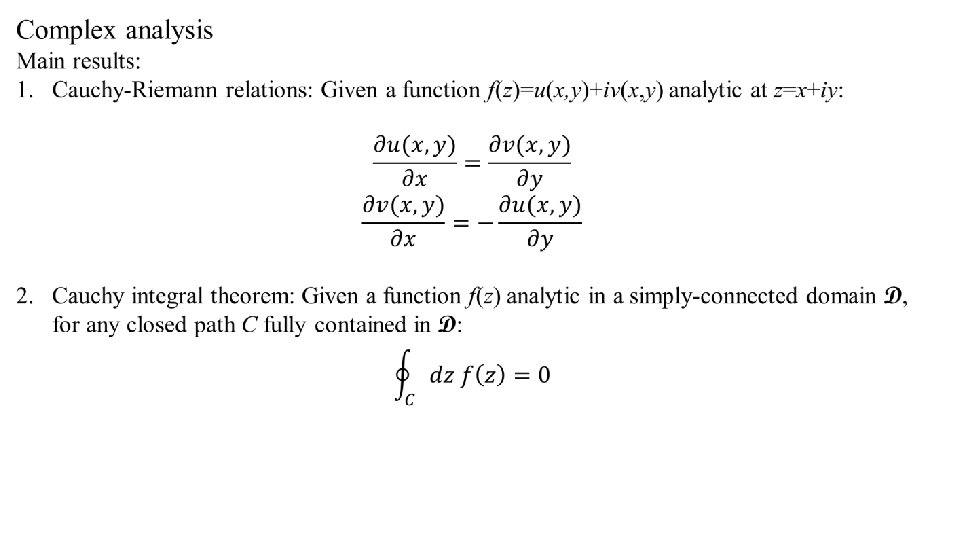
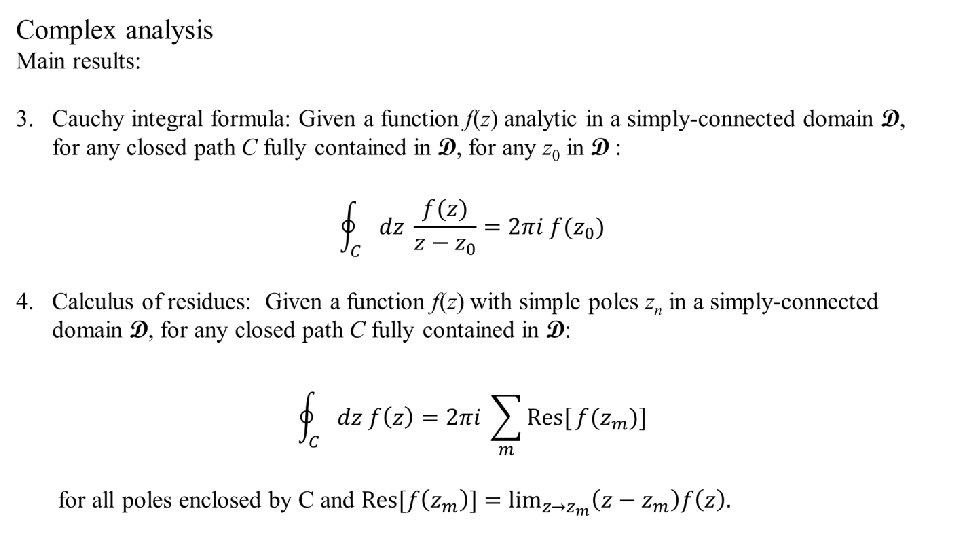
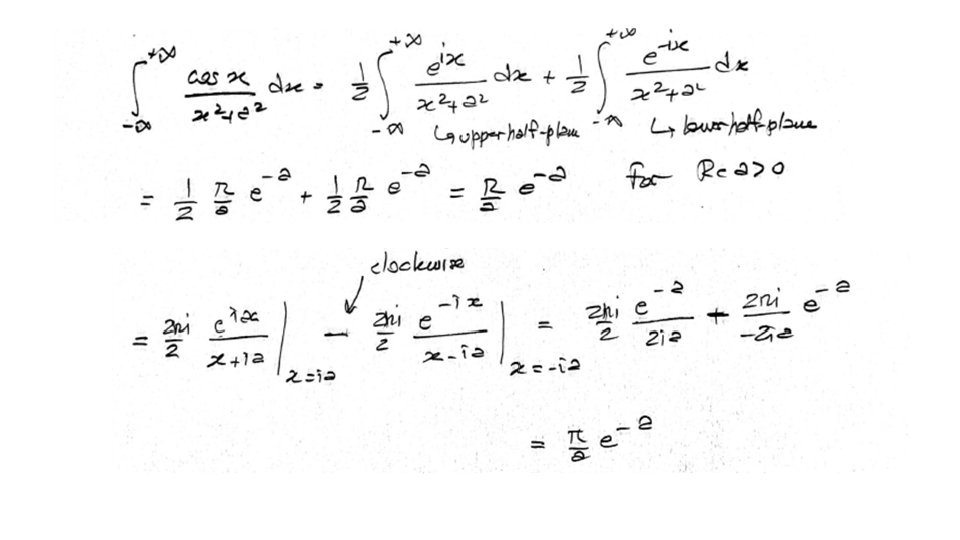
Outline of the class: 1. Vector calculus • Vector algebra • Scalar and vector fields • Differential calculus: Gradient, divergence, curl • Integral calculus: Line integrals, surface integrals (flux), volume integrals • Basic theorems: Green’s, divergence, Stokes’ 2. Fourier analysis: Series, transforms, generalization to ‘orthogonal functions’ (Bessel functions, Legendre/associate-Legendre polynomials, spherical harmonics…): The Sturm-Liouville theory 3. Partial differential equations (PDEs) • The wave equation • The diffusion (heat) equation • The Laplace and Poisson equations • The continuity equation 4. Complex analysis

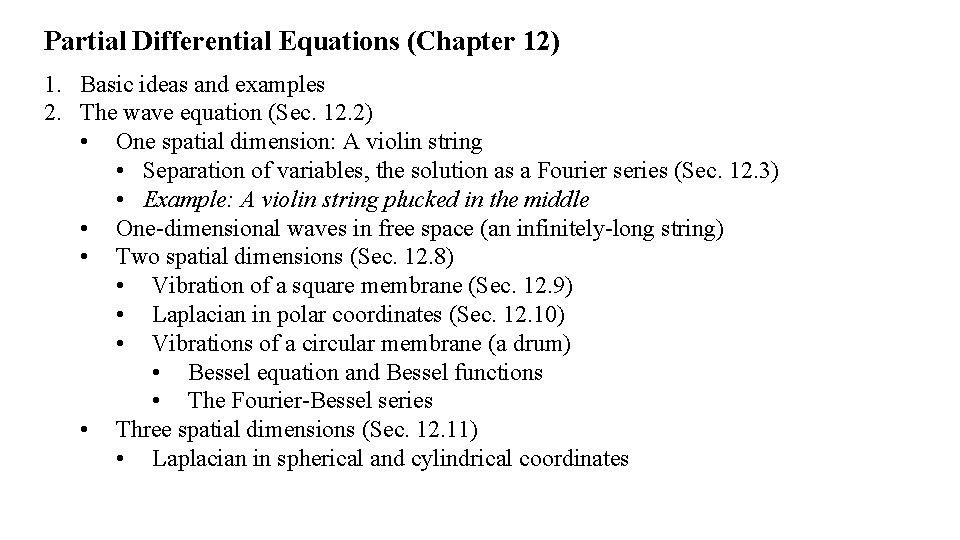
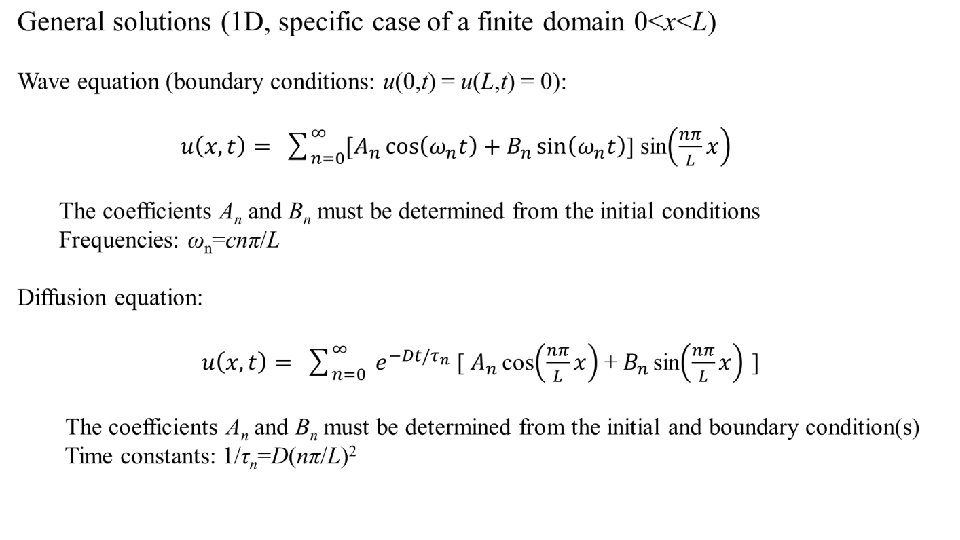
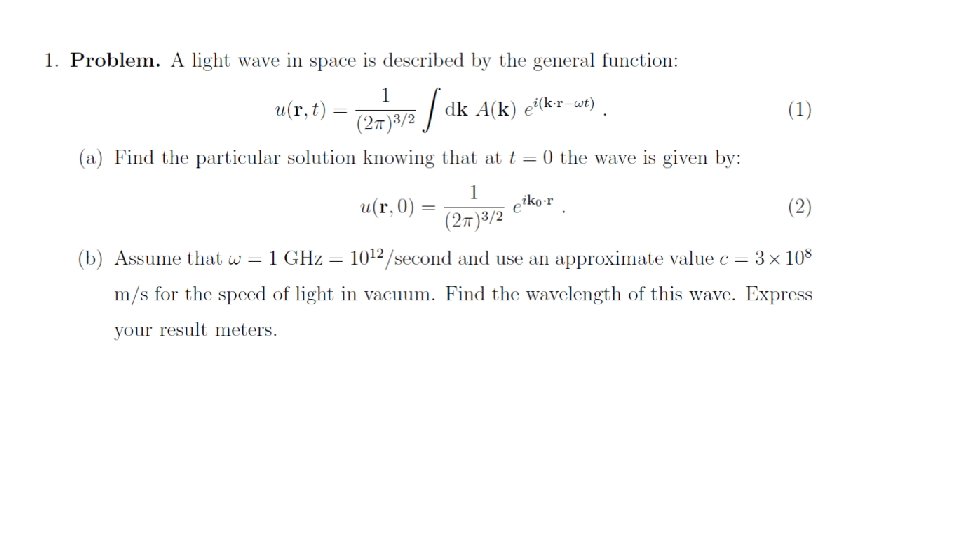
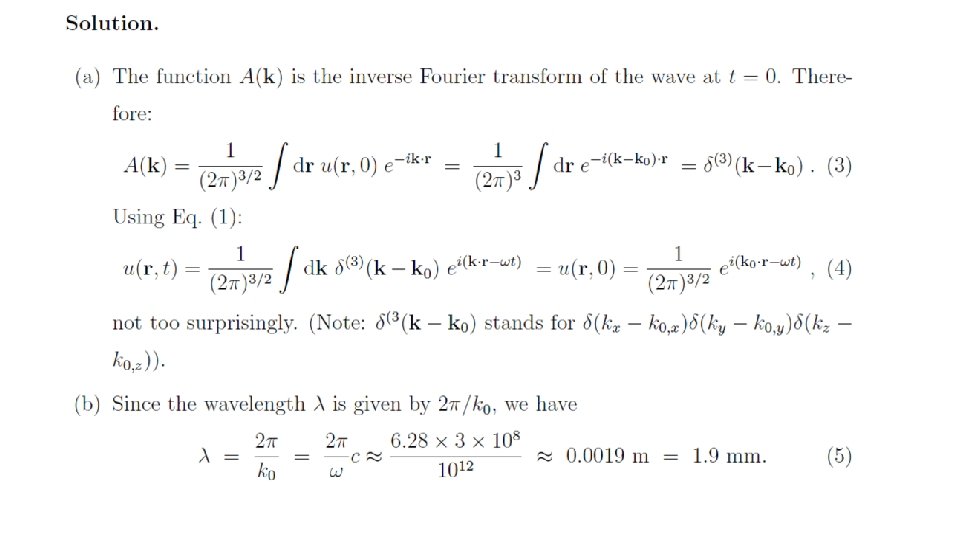
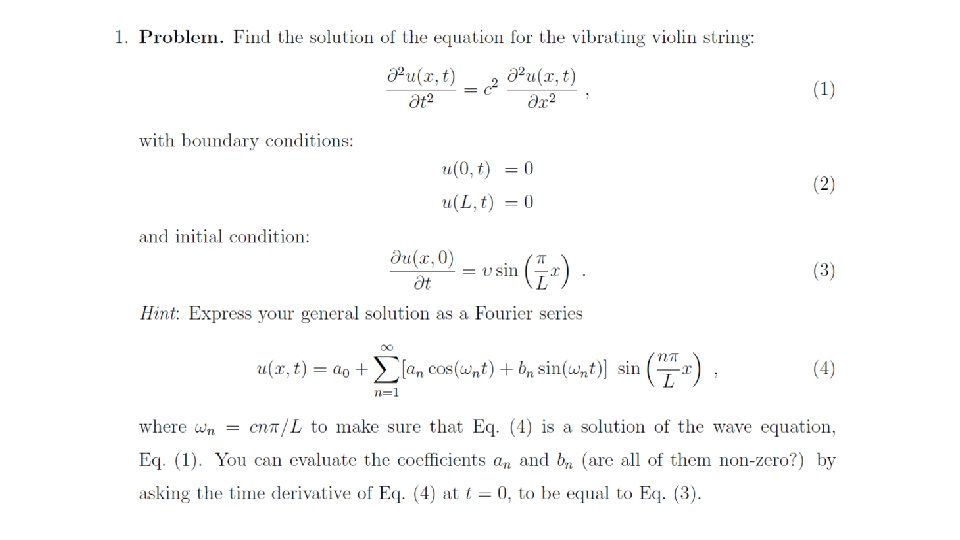
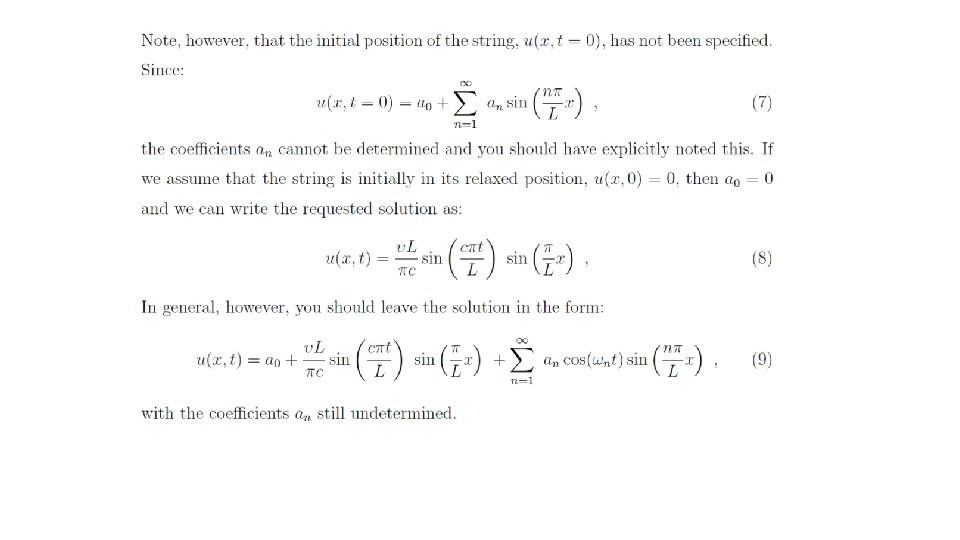
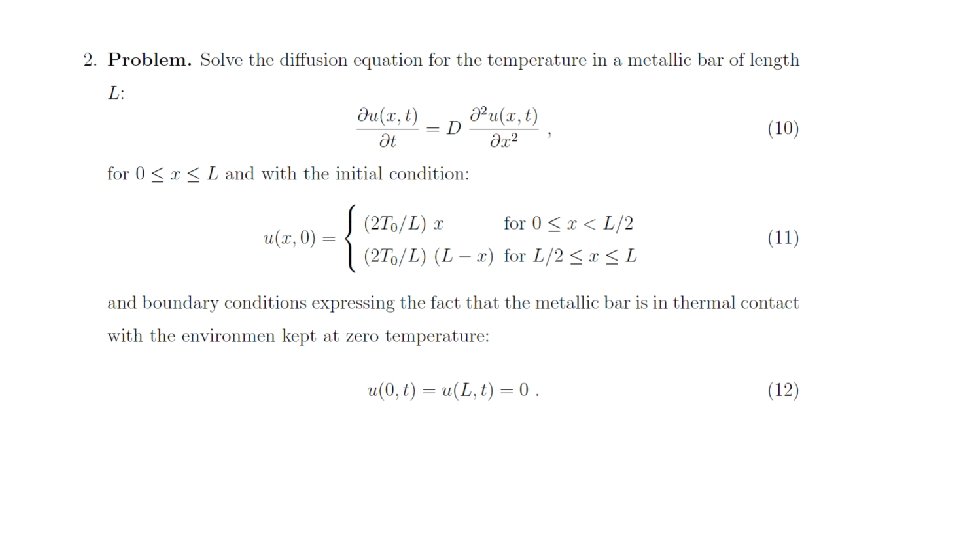
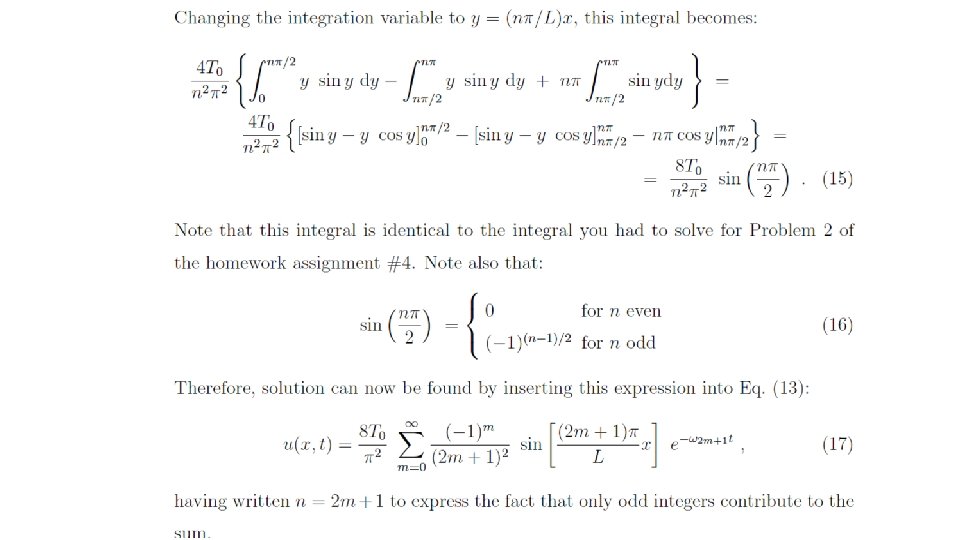
Partial Differential Equations (Chapter 12) 1. Basic ideas and examples 2. The wave equation (Sec. 12. 2) • One spatial dimension: A violin string • Separation of variables, the solution as a Fourier series (Sec. 12. 3) • Example: A violin string plucked in the middle • One-dimensional waves in free space (an infinitely-long string) • Two spatial dimensions (Sec. 12. 8) • Vibration of a square membrane (Sec. 12. 9) • Laplacian in polar coordinates (Sec. 12. 10) • Vibrations of a circular membrane (a drum) • Bessel equation and Bessel functions • The Fourier-Bessel series • Three spatial dimensions (Sec. 12. 11) • Laplacian in spherical and cylindrical coordinates

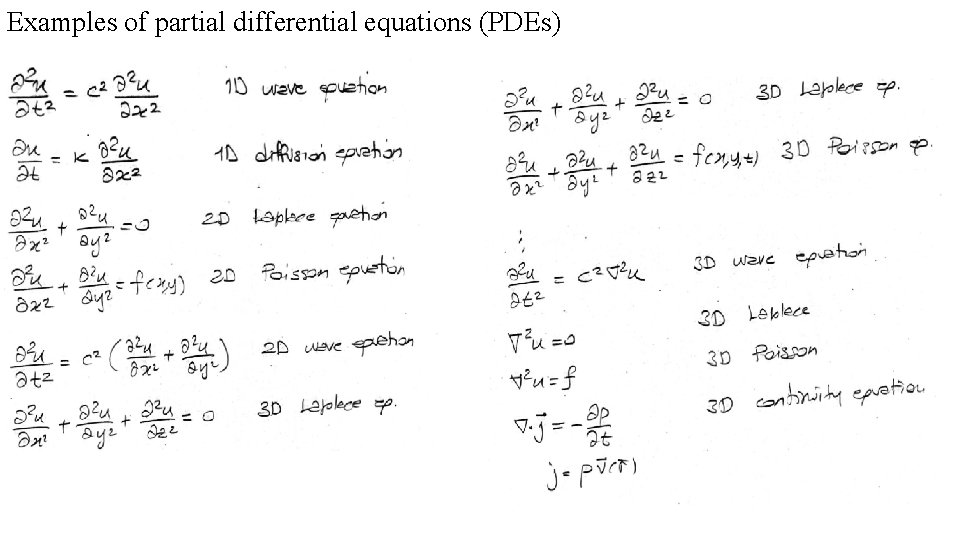
Examples of partial differential equations (PDEs)

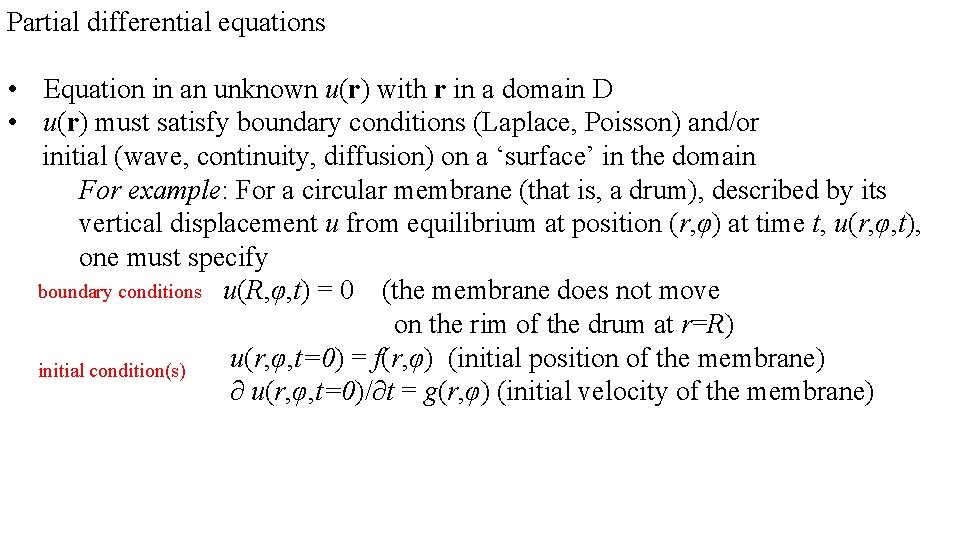
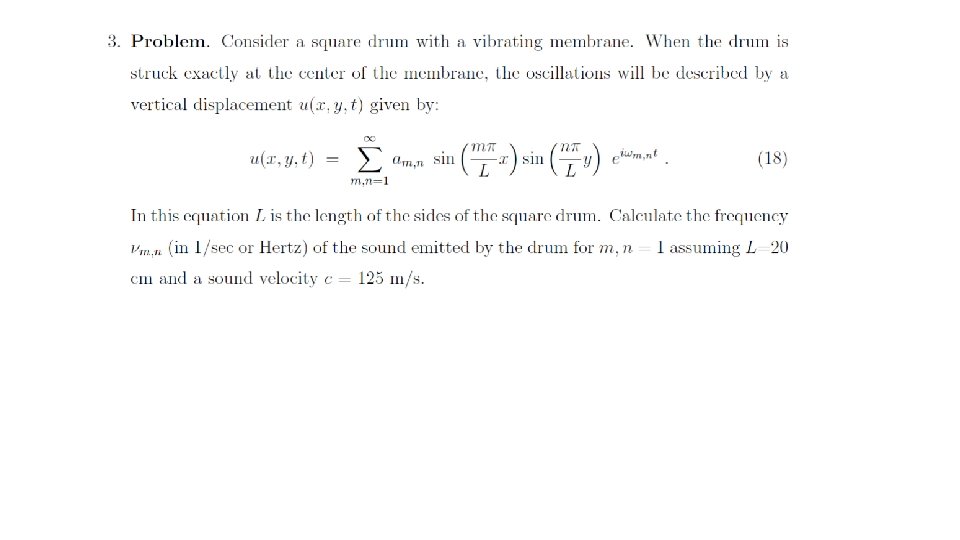
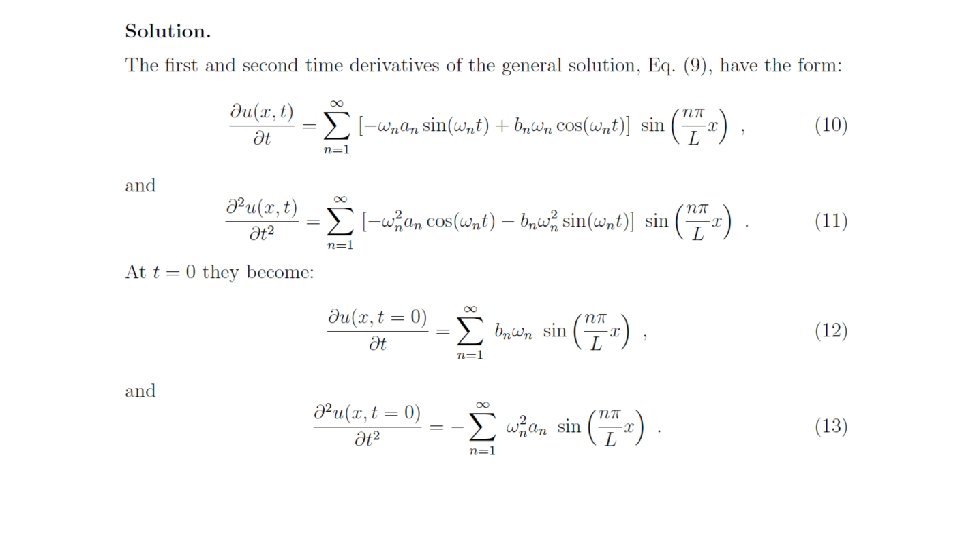
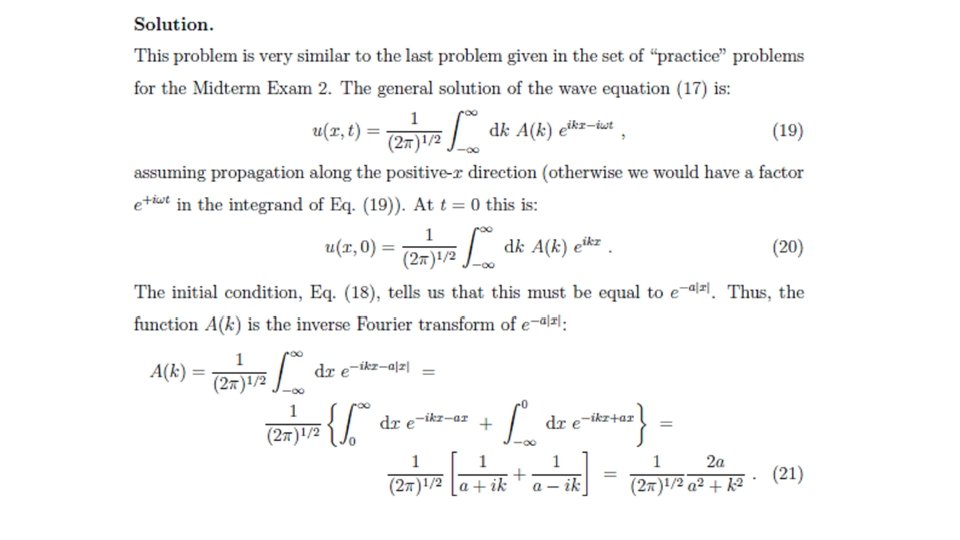
Partial differential equations • Equation in an unknown u(r) with r in a domain D • u(r) must satisfy boundary conditions (Laplace, Poisson) and/or initial (wave, continuity, diffusion) on a ‘surface’ in the domain For example: For a circular membrane (that is, a drum), described by its vertical displacement u from equilibrium at position (r, φ) at time t, u(r, φ, t), one must specify boundary conditions u(R, φ, t) = 0 (the membrane does not move on the rim of the drum at r=R) u(r, φ, t=0) = f(r, φ) (initial position of the membrane) initial condition(s) ∂ u(r, φ, t=0)/∂t = g(r, φ) (initial velocity of the membrane)
![Partial differential equations For a homogeneous linear problem Lur 0 if u Partial differential equations • For a homogeneous linear problem L[u(r)] = 0 if u](https://slidetodoc.com/presentation_image_h/141520fa639d10d32149095315d24021/image-5.jpg)
Partial differential equations • For a homogeneous linear problem L[u(r)] = 0 if u 1(r) and u 1(r) are two solutions, that is: L[u 1(r)] = 0 and L[u 2(r)] = 0, then: au 1(r)+b u 2(r) is also a solution, the same situation we have for ordinary differential equations

















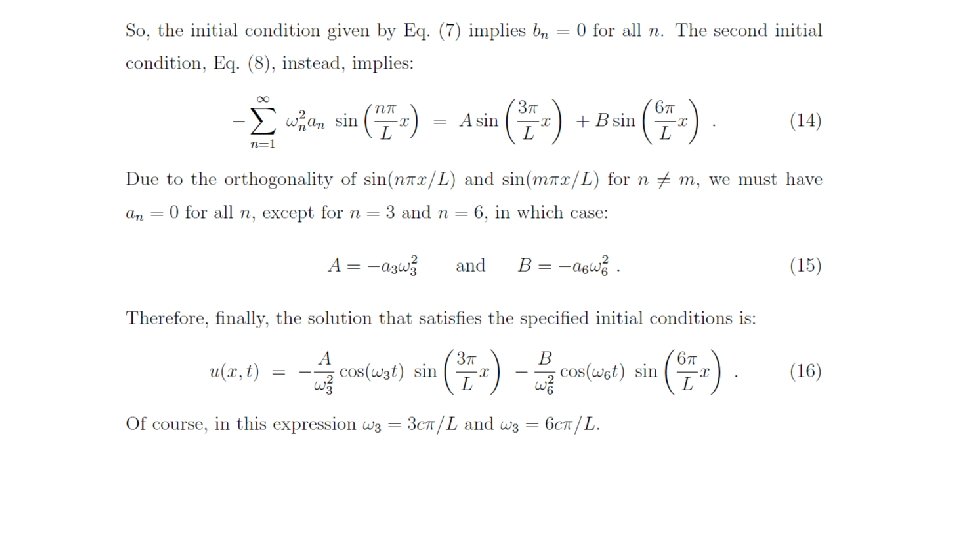







 Ixj
Ixj Nabla operator
Nabla operator Divergence de gradient
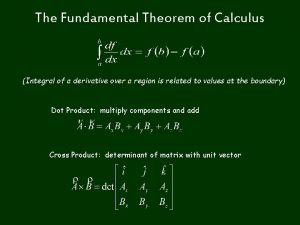
Divergence de gradient Fundamental theorem of vector calculus
Fundamental theorem of vector calculus Project on calculus class 12
Project on calculus class 12 Teorema fundamental kalkulus 2
Teorema fundamental kalkulus 2 It in a sentence
It in a sentence Vector properties
Vector properties Fsica
Fsica Why is vector resolution the opposite of vector addition
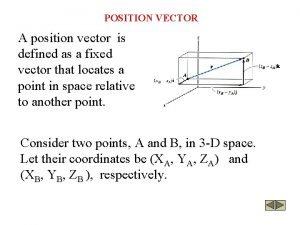
Why is vector resolution the opposite of vector addition A position vector
A position vector Hình ảnh bộ gõ cơ thể búng tay
Hình ảnh bộ gõ cơ thể búng tay Lp html
Lp html Bổ thể
Bổ thể Tỉ lệ cơ thể trẻ em
Tỉ lệ cơ thể trẻ em Voi kéo gỗ như thế nào
Voi kéo gỗ như thế nào Tư thế worms-breton
Tư thế worms-breton Chúa yêu trần thế
Chúa yêu trần thế Môn thể thao bắt đầu bằng từ đua
Môn thể thao bắt đầu bằng từ đua Thế nào là hệ số cao nhất
Thế nào là hệ số cao nhất Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Công thức tính độ biến thiên đông lượng
Công thức tính độ biến thiên đông lượng Trời xanh đây là của chúng ta thể thơ
Trời xanh đây là của chúng ta thể thơ Cách giải mật thư tọa độ
Cách giải mật thư tọa độ 101012 bằng
101012 bằng Phản ứng thế ankan
Phản ứng thế ankan Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Thơ thất ngôn tứ tuyệt đường luật
Thơ thất ngôn tứ tuyệt đường luật Quá trình desamine hóa có thể tạo ra
Quá trình desamine hóa có thể tạo ra Một số thể thơ truyền thống
Một số thể thơ truyền thống Cái miệng nó xinh thế chỉ nói điều hay thôi
Cái miệng nó xinh thế chỉ nói điều hay thôi