Optimisation dans les rseaux Recherche Oprationnelle GCSIE Flot


![Introduction Données : § Graphe (N, A) § Capacités : xij [bij, cij] § Introduction Données : § Graphe (N, A) § Capacités : xij [bij, cij] §](https://slidetodoc.com/presentation_image_h/84f0bcf235d05a8eb69da76e8b9fd93d/image-3.jpg)












- Slides: 45

Optimisation dans les réseaux Recherche Opérationnelle GC-SIE Flot maximal Michel Bierlaire

Le problème du flot maximal Flot maximal Michel Bierlaire
![Introduction Données Graphe N A Capacités xij bij cij Introduction Données : § Graphe (N, A) § Capacités : xij [bij, cij] §](https://slidetodoc.com/presentation_image_h/84f0bcf235d05a8eb69da76e8b9fd93d/image-3.jpg)
Introduction Données : § Graphe (N, A) § Capacités : xij [bij, cij] § Un nœud source s § Un nœud puits t Problème : § Faire passer un maximum de flot de s à t. § Maximiser la divergence de s § Minimiser la divergence de t. Flot maximal Michel Bierlaire 3

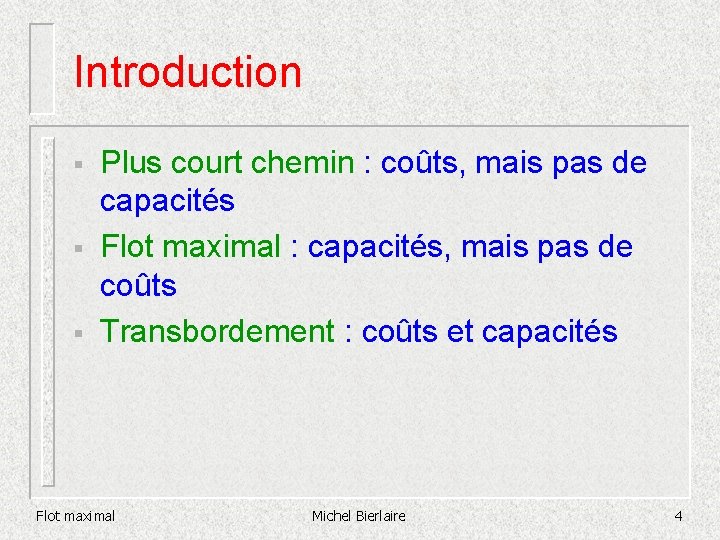
Introduction § § § Plus court chemin : coûts, mais pas de capacités Flot maximal : capacités, mais pas de coûts Transbordement : coûts et capacités Flot maximal Michel Bierlaire 4

Flot maximal et coupe minimale Idée de base : § Un flot x peut être amélioré si l’on trouve un chemin de s à t qui soit non bloqué par rapport à x. § Envoyer un flot le long de ce chemin augmente la divergence de s sans violer les contraintes de capacité. § Question : si on ne trouve pas un tel chemin, sommes-nous à l’optimum ? Flot maximal Michel Bierlaire 5

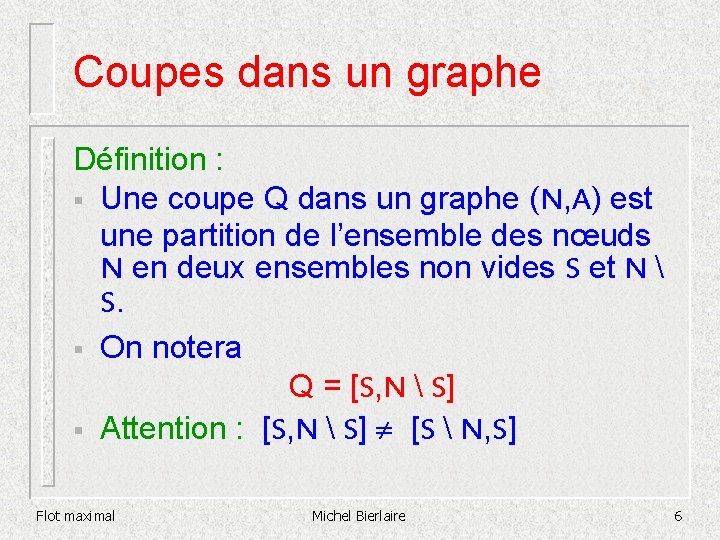
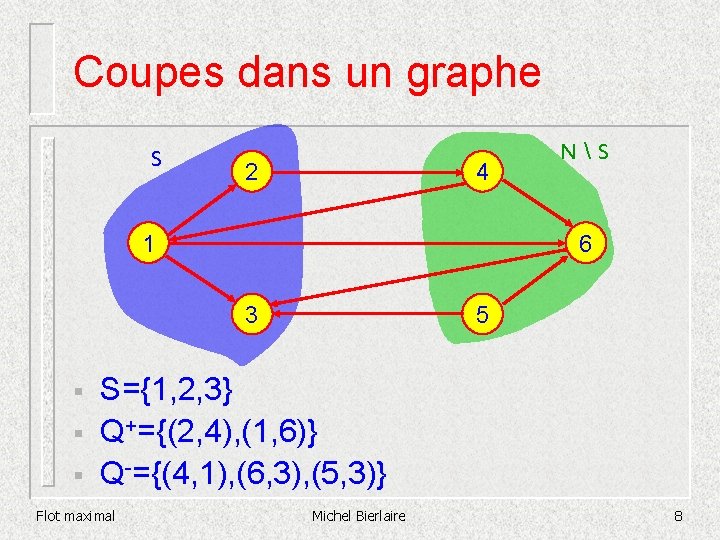
Coupes dans un graphe Définition : § Une coupe Q dans un graphe (N, A) est une partition de l’ensemble des nœuds N en deux ensembles non vides S et N S. § On notera Q = [S, N S] § Attention : [S, N S] [S N, S] Flot maximal Michel Bierlaire 6

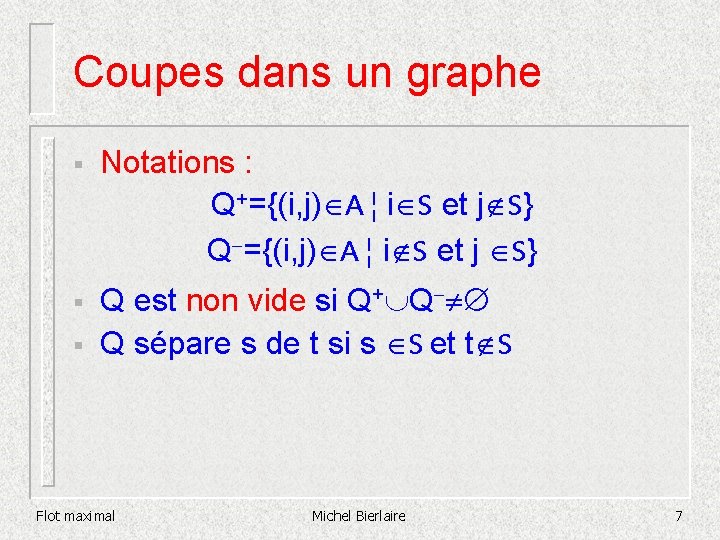
Coupes dans un graphe § Notations : Q+={(i, j) A ¦ i S et j S} Q-={(i, j) A ¦ i S et j S} § Q est non vide si Q+ Q- Q sépare s de t si s S et t S § Flot maximal Michel Bierlaire 7

Coupes dans un graphe S 2 4 1 6 5 3 § § § NS S={1, 2, 3} Q+={(2, 4), (1, 6)} Q-={(4, 1), (6, 3), (5, 3)} Flot maximal Michel Bierlaire 8

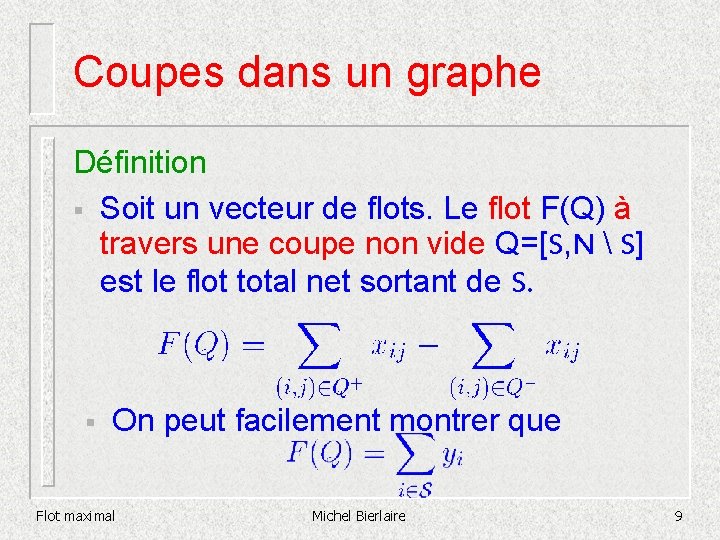
Coupes dans un graphe Définition § Soit un vecteur de flots. Le flot F(Q) à travers une coupe non vide Q=[S, N S] est le flot total net sortant de S. § On peut facilement montrer que Flot maximal Michel Bierlaire 9

Coupes dans un graphe Définition : § Étant données les contraintes de capacité sur les flots, la capacité d’une coupe Q non vide est Flot maximal Michel Bierlaire 10

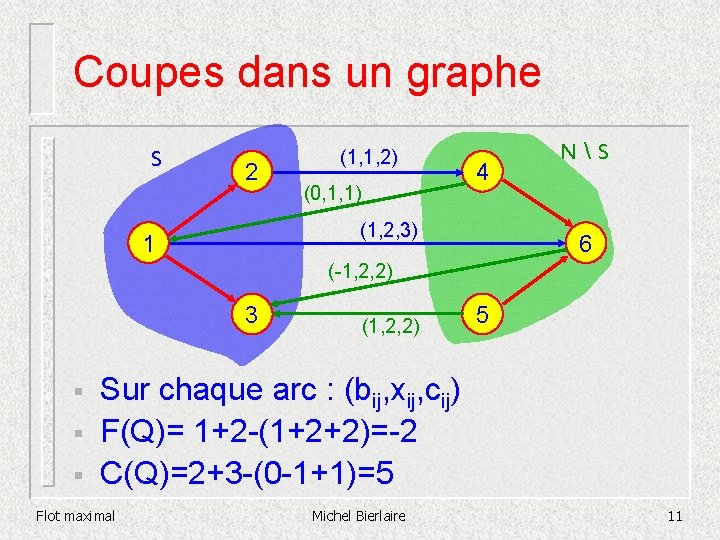
Coupes dans un graphe S 2 (1, 1, 2) (0, 1, 1) 4 (1, 2, 3) 1 NS 6 (-1, 2, 2) 3 § § § (1, 2, 2) 5 Sur chaque arc : (bij, xij, cij) F(Q)= 1+2 -(1+2+2)=-2 C(Q)=2+3 -(0 -1+1)=5 Flot maximal Michel Bierlaire 11

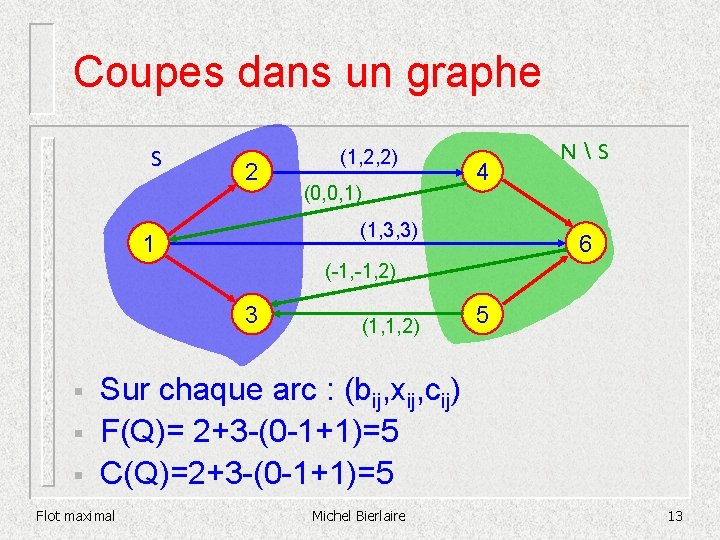
Coupes dans un graphe § § § On a toujours F(Q) £ C(Q) Si F(Q)=C(Q), on dit que la coupe est saturée par rapport au vecteur de flots x. Par convention, une coupe vide est supposée saturée. Flot maximal Michel Bierlaire 12

Coupes dans un graphe S 2 (1, 2, 2) (0, 0, 1) 4 (1, 3, 3) 1 NS 6 (-1, 2) 3 § § § (1, 1, 2) 5 Sur chaque arc : (bij, xij, cij) F(Q)= 2+3 -(0 -1+1)=5 C(Q)=2+3 -(0 -1+1)=5 Flot maximal Michel Bierlaire 13

Coupes dans un graphe Théorème § Soit x un vecteur de flots vérifiant les contraintes de capacité. § Soit s et t deux nœuds. § Exactement une des deux affirmations suivantes est vérifiée : 1. Il existe un chemin simple de s à t non bloqué par rapport à x. 2. Il existe une coupe saturée séparant s de t. Flot maximal Michel Bierlaire 14

Coupes dans un graphe Algorithme du chemin non bloqué Idée : § On génère une suite d’ensembles de nœuds (Tk), avec T 0 = {s} § Tk contient l’ensemble des nœuds qui peuvent être atteints à partir de s par un chemin non bloqué de k arcs. Flot maximal Michel Bierlaire 15

Coupes dans un graphe Algorithme du chemin non bloqué Pour k=0, 1, … § Si Tk = ou t Tk, STOP § Tk+1 = § Pour tout nœud i Tk – Pour tout (i, j) A § – Pour tout (j, i) A § Flot maximal Si xij < cij et j T 0, …Tk alors Tk+1=Tk+1 {j} Si xij > bij et j T 0, …Tk alors Tk+1=Tk+1 {j} Michel Bierlaire 16

Coupes dans un graphe Notes : § Il y a deux manières pour cet algorithme de s’arrêter 1. 2. Flot maximal t Tk. Dans ce cas, il existe un chemin non bloqué de s à t. Tk = . Si on définit S= Ti, alors la coupe [S, N S] est saturée. Michel Bierlaire 17

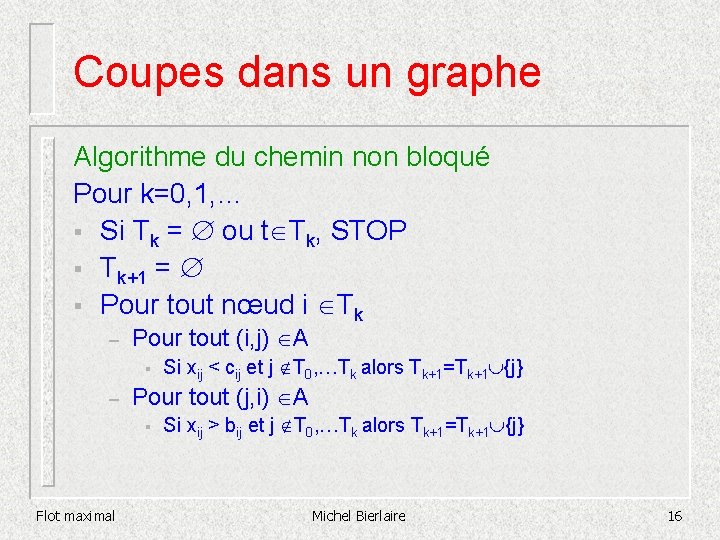
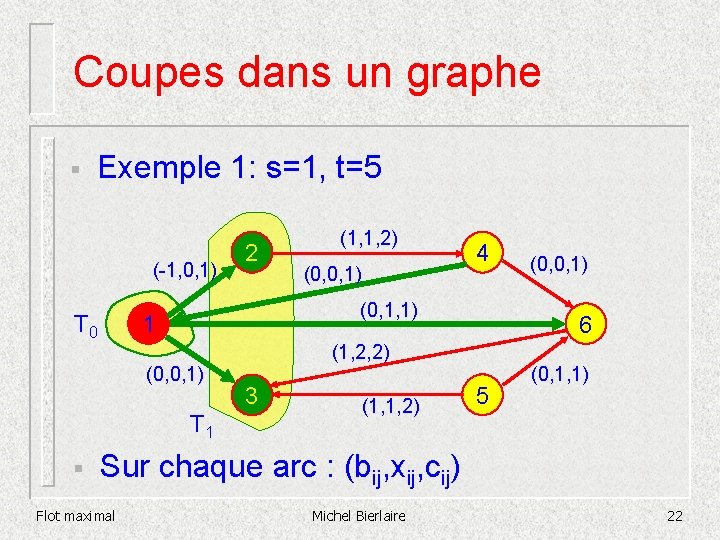
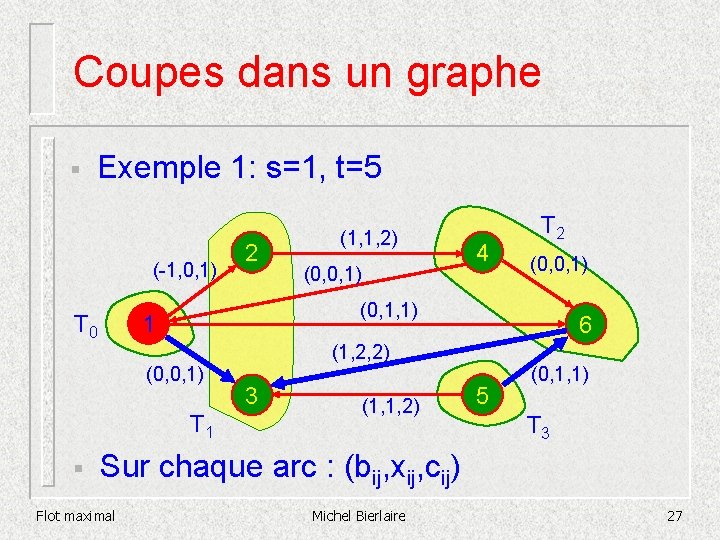
Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) (0, 0, 1) 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 18

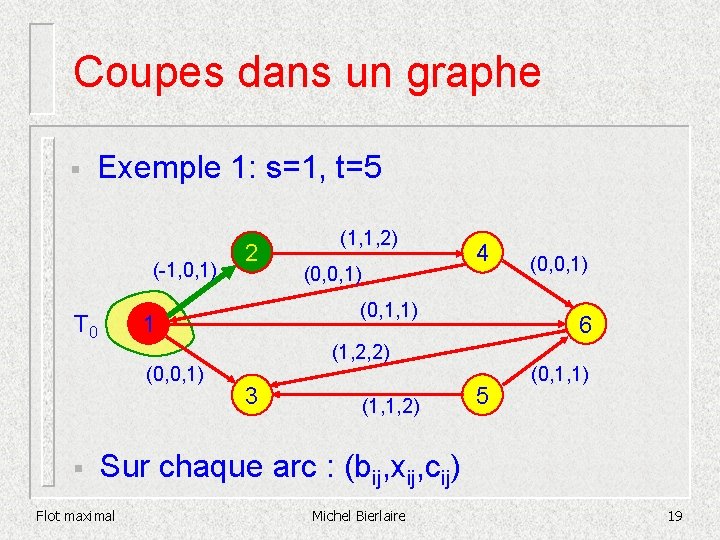
Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) (0, 0, 1) 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 19

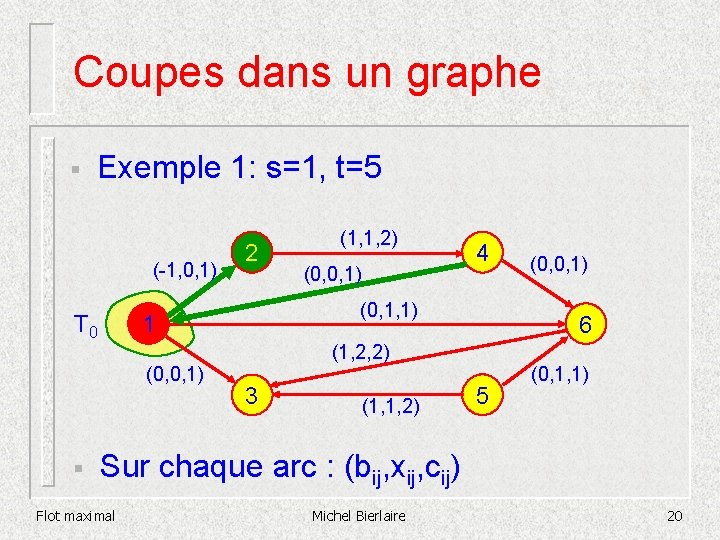
Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) (0, 0, 1) 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 20

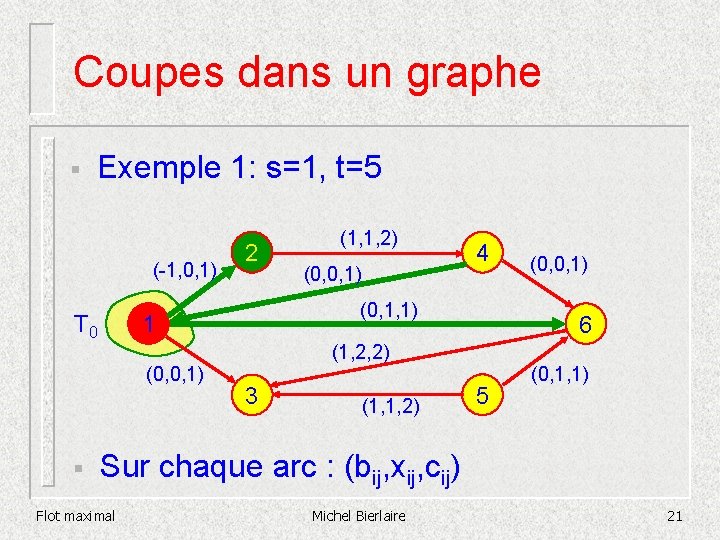
Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) (0, 0, 1) 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 21

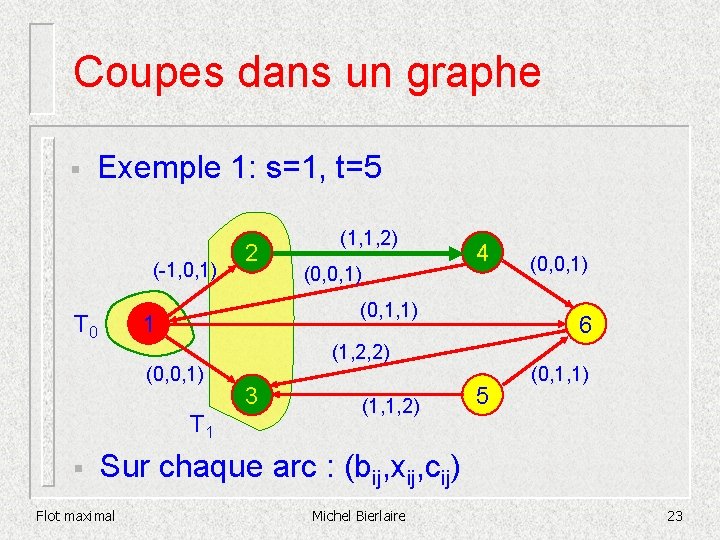
Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) T 1 § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) (0, 0, 1) 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 22

Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) T 1 § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) (0, 0, 1) 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 23

Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) T 1 § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) (0, 0, 1) 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 24

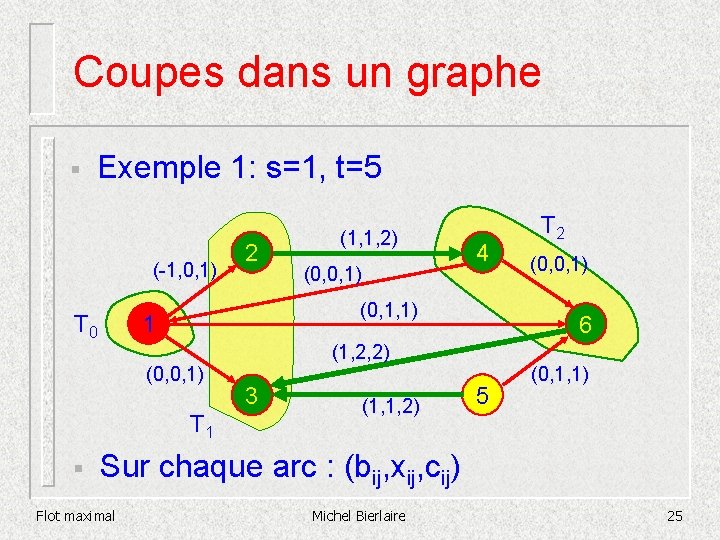
Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) T 1 § 2 (1, 1, 2) (0, 0, 1) 6 (1, 2, 2) 3 T 2 5 (0, 1, 1) Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 25

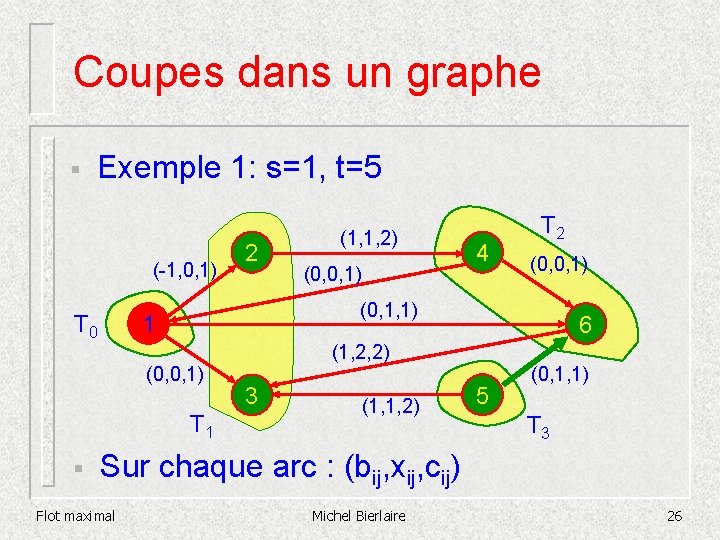
Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 T 2 (0, 0, 1) (0, 1, 1) 1 (0, 0, 1) T 1 § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) 5 (0, 1, 1) T 3 Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 26

Coupes dans un graphe § Exemple 1: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 T 2 (0, 0, 1) (0, 1, 1) 1 (0, 0, 1) T 1 § 2 (1, 1, 2) 6 (1, 2, 2) 3 (1, 1, 2) 5 (0, 1, 1) T 3 Sur chaque arc : (bij, xij, cij) Flot maximal Michel Bierlaire 27

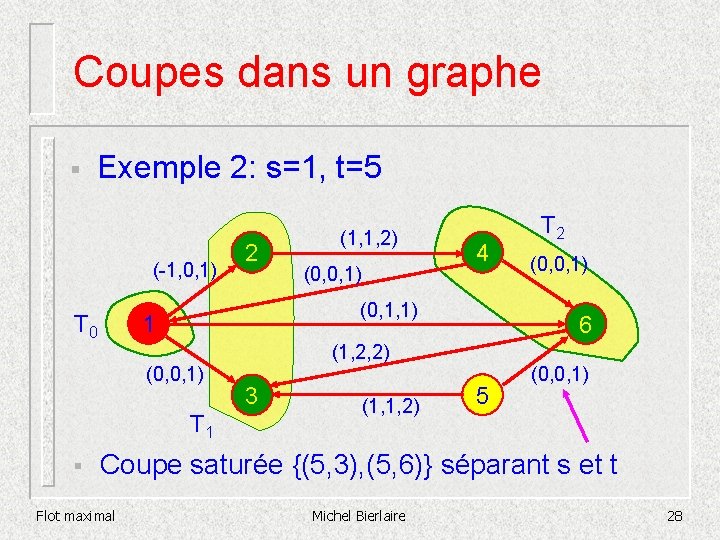
Coupes dans un graphe § Exemple 2: s=1, t=5 (-1, 0, 1) T 0 (0, 0, 1) 4 (0, 1, 1) 1 (0, 0, 1) T 1 § 2 (1, 1, 2) (0, 0, 1) 6 (1, 2, 2) 3 T 2 5 (0, 0, 1) Coupe saturée {(5, 3), (5, 6)} séparant s et t Flot maximal Michel Bierlaire 28

Coupe et flot maximal § § § Dans le problème de flot maximal, on cherche parmi les vecteurs de flot admissibles, ayant une divergence nulle en tout nœud différente de s ou t, celui qui maximise la divergence de s. Soit un de ces vecteurs de flots, et une coupe Q=[S, N S] séparant s de t. La divergence de s est le flot qui traverse Q. En effet, s est le seul nœud de S à divergence non nulle. Flot maximal Michel Bierlaire 29

Coupe et flot maximal § § On a donc Q, divergence s = F(Q) £ C(Q) flot maximal £ capacité de Q Si le problème de flot maximum possède une solution, alors il existe une coupe telle que l’inégalité soit une égalité. Flot maximal Michel Bierlaire 30

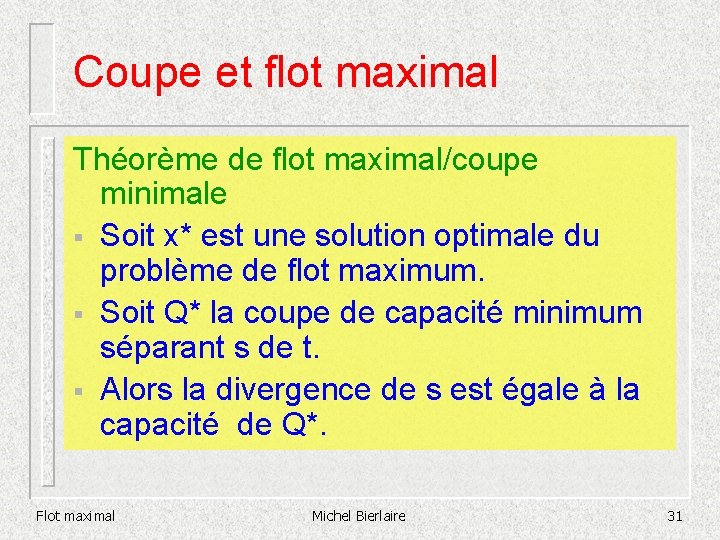
Coupe et flot maximal Théorème de flot maximal/coupe minimale § Soit x* est une solution optimale du problème de flot maximum. § Soit Q* la coupe de capacité minimum séparant s de t. § Alors la divergence de s est égale à la capacité de Q*. Flot maximal Michel Bierlaire 31

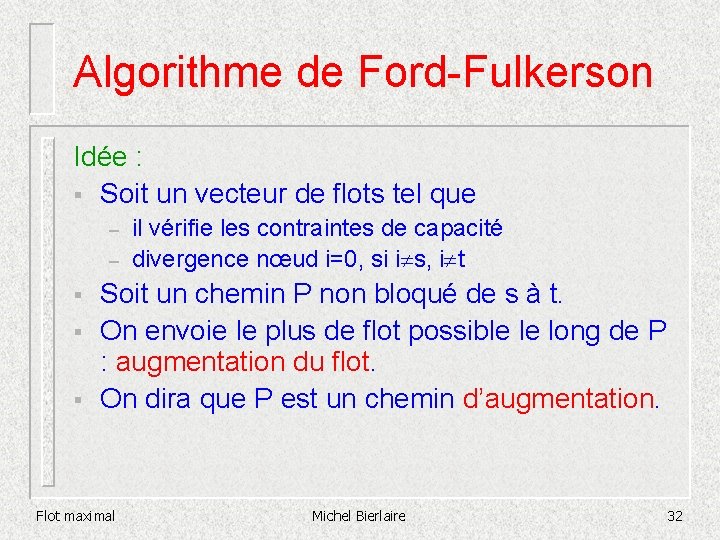
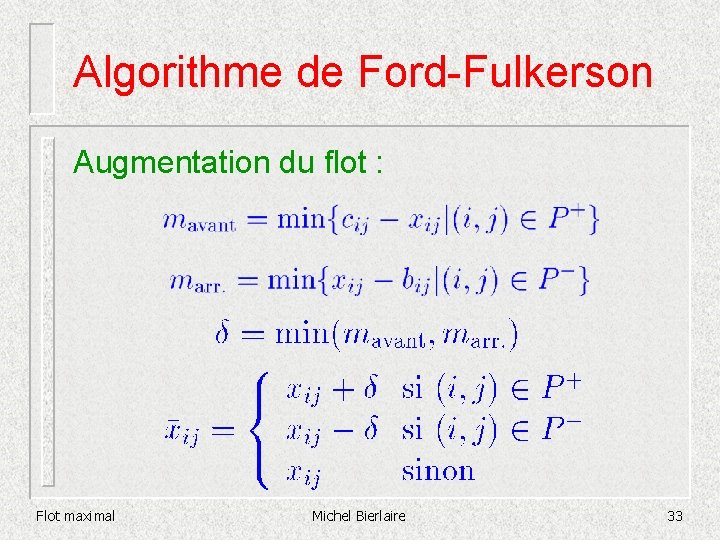
Algorithme de Ford-Fulkerson Idée : § Soit un vecteur de flots tel que – – § § § il vérifie les contraintes de capacité divergence nœud i=0, si i s, i t Soit un chemin P non bloqué de s à t. On envoie le plus de flot possible le long de P : augmentation du flot. On dira que P est un chemin d’augmentation. Flot maximal Michel Bierlaire 32

Algorithme de Ford-Fulkerson Augmentation du flot : Flot maximal Michel Bierlaire 33

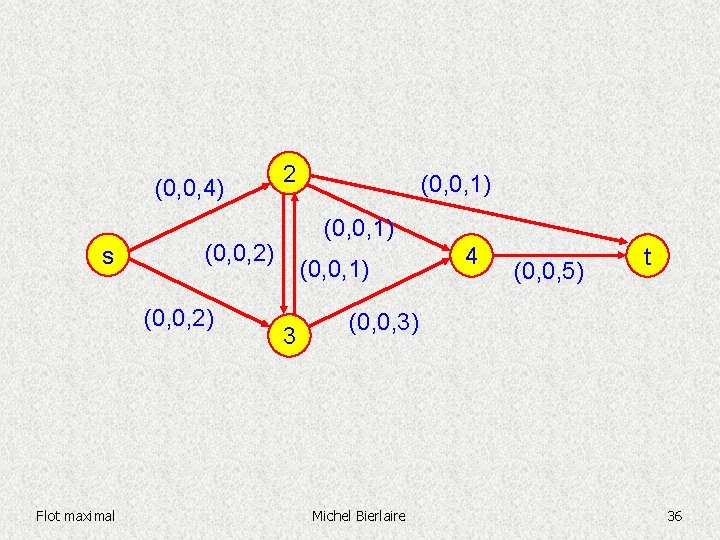
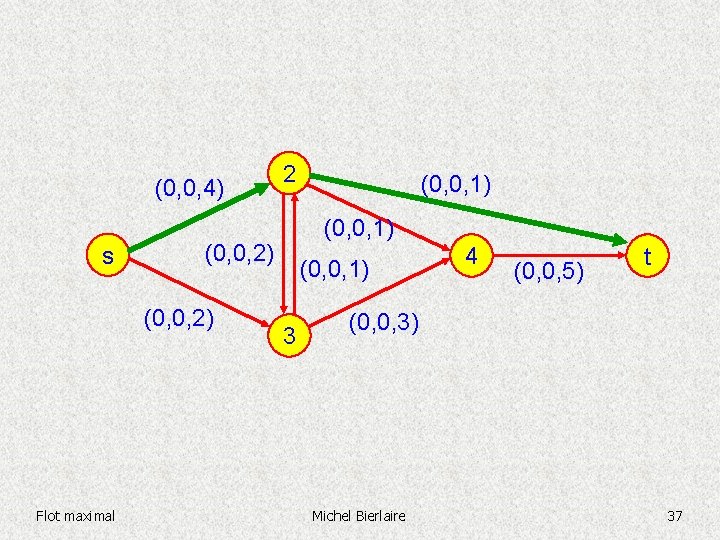
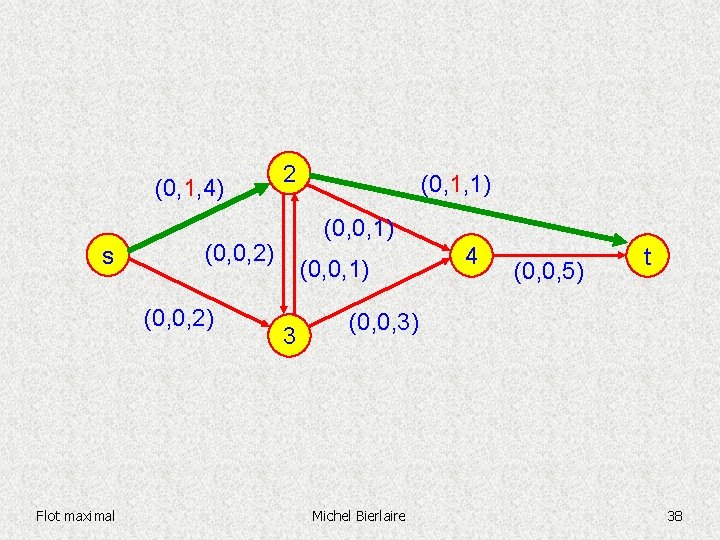
Algorithme de Ford-Fulkerson Initialisation § Trouver un vecteur de flots admissible. § Si toutes les capacités inférieures bij sont nulles, le vecteur nul est admissible. Itérations § Appliquer l’algorithme du chemin non bloqué. § Si coupe saturée : STOP. § Si chemin non bloqué : augmentation de flot. Flot maximal Michel Bierlaire 34

Algorithme de Ford-Fulkerson Notes : § Si le vecteur de flot initial ainsi que les bornes sont entiers ou rationnels, l’algorithme se terminera en un nombre fini d’itérations. § Méthode du plus court chemin d’augmentation : parmi tous les chemins non bloqués, choisir comme chemin d’augmentation celui comportant le moins d’arcs. § Si cette méthode est utilisée, l’algorithme convergera toujours. § De plus, même avec des données entières, cette méthode est plus performante. Flot maximal Michel Bierlaire 35

(0, 0, 4) s (0, 0, 1) (0, 0, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 0, 5) t (0, 0, 3) Michel Bierlaire 36

(0, 0, 4) s (0, 0, 1) (0, 0, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 0, 5) t (0, 0, 3) Michel Bierlaire 37

(0, 1, 4) s (0, 1, 1) (0, 0, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 0, 5) t (0, 0, 3) Michel Bierlaire 38

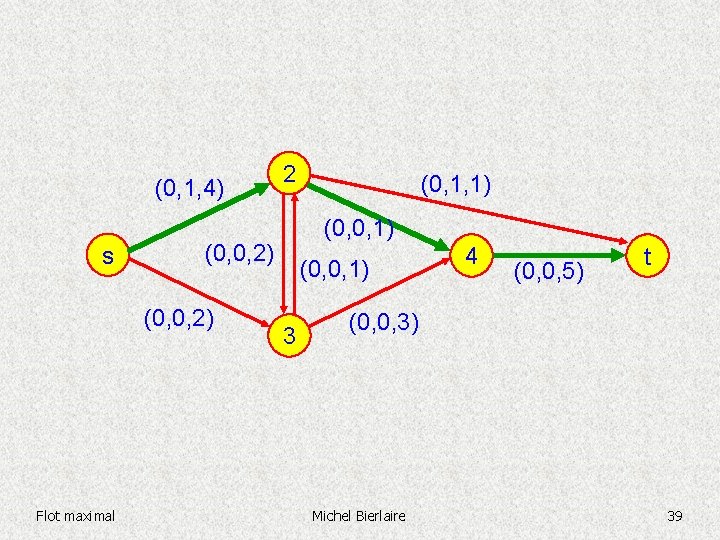
(0, 1, 4) s (0, 1, 1) (0, 0, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 0, 5) t (0, 0, 3) Michel Bierlaire 39

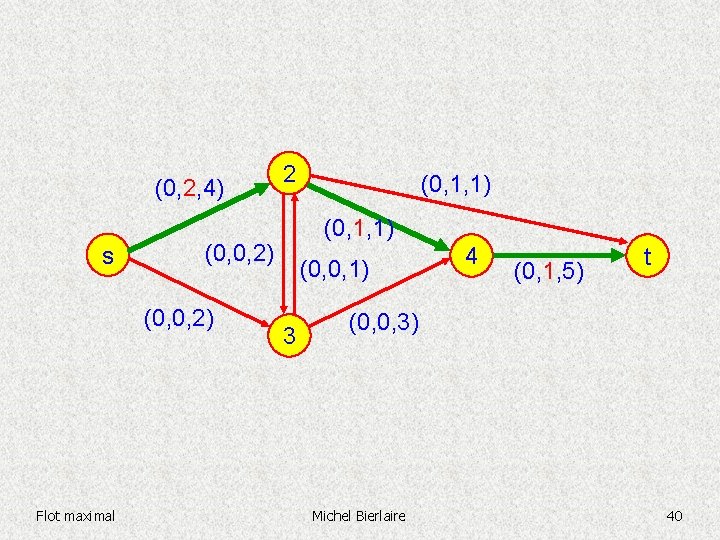
(0, 2, 4) s (0, 1, 1) (0, 0, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 1, 5) t (0, 0, 3) Michel Bierlaire 40

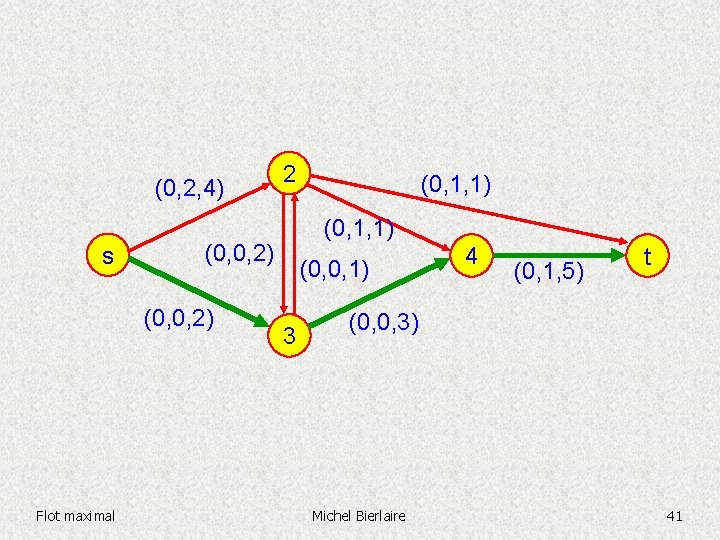
(0, 2, 4) s (0, 1, 1) (0, 0, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 1, 5) t (0, 0, 3) Michel Bierlaire 41

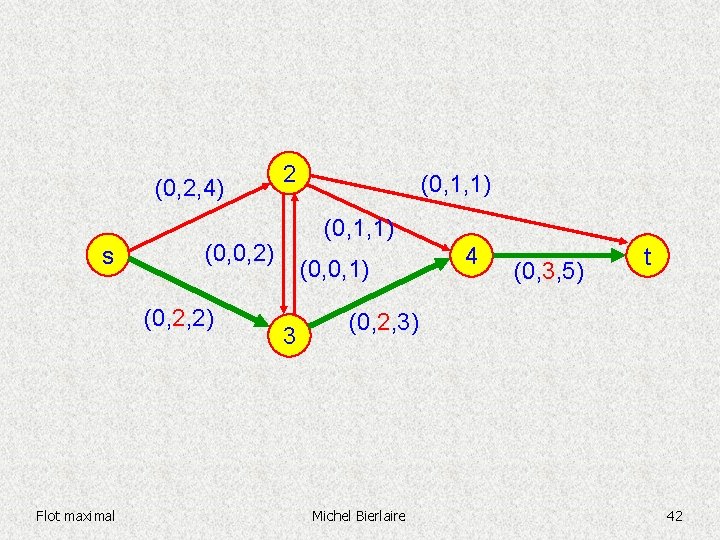
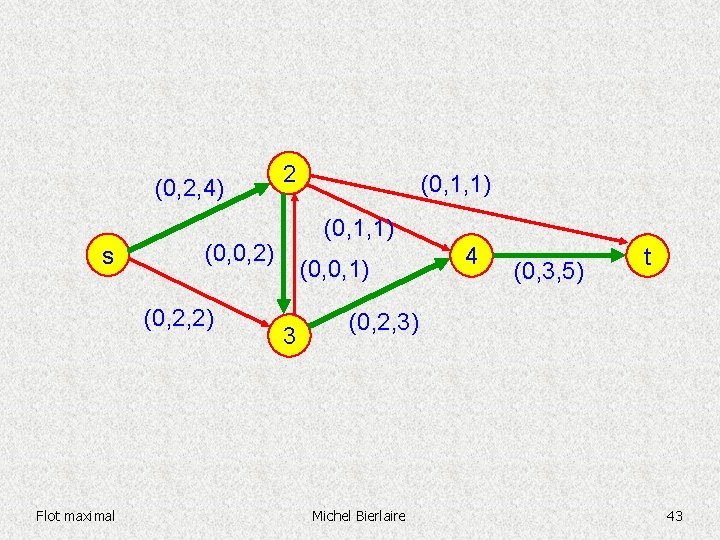
(0, 2, 4) s (0, 1, 1) (0, 0, 2) (0, 2, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 3, 5) t (0, 2, 3) Michel Bierlaire 42

(0, 2, 4) s (0, 1, 1) (0, 0, 2) (0, 2, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 3, 5) t (0, 2, 3) Michel Bierlaire 43

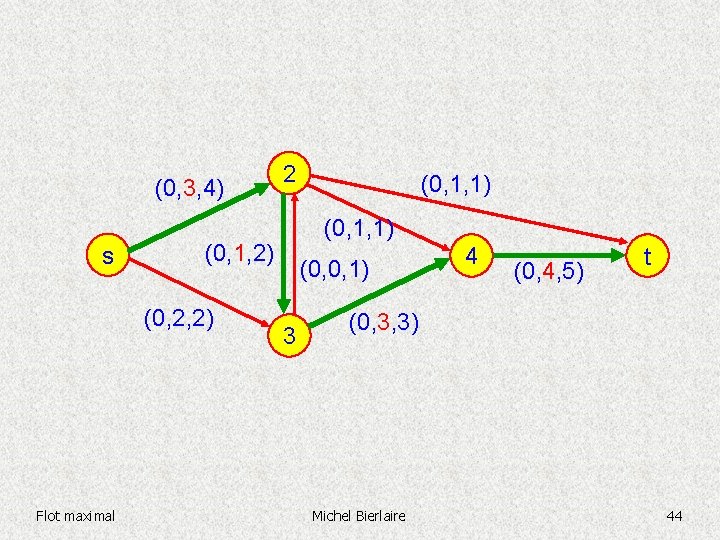
(0, 3, 4) s (0, 1, 1) (0, 1, 2) (0, 2, 2) Flot maximal 2 (0, 0, 1) 3 4 (0, 4, 5) t (0, 3, 3) Michel Bierlaire 44

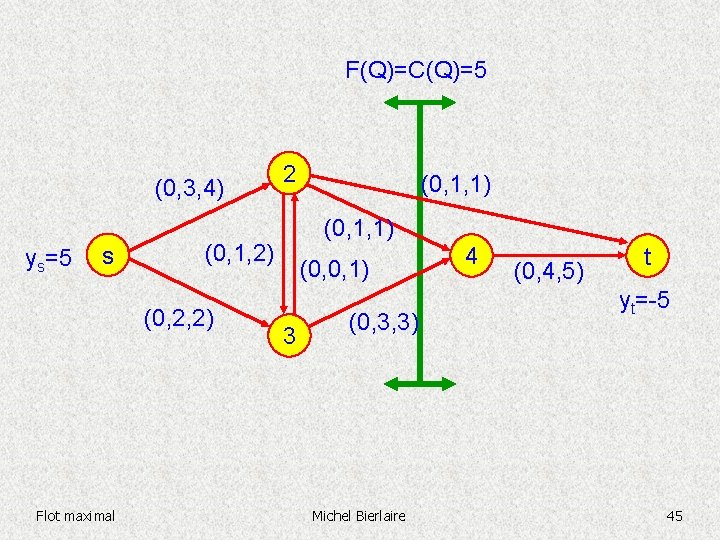
F(Q)=C(Q)=5 (0, 3, 4) ys=5 s (0, 1, 1) (0, 1, 2) (0, 2, 2) Flot maximal 2 (0, 0, 1) 3 (0, 3, 3) Michel Bierlaire 4 (0, 4, 5) t yt=-5 45
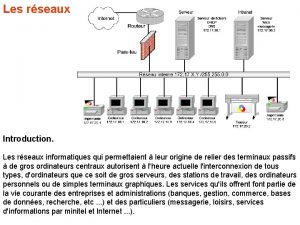
 Rseaux
Rseaux Scurit
Scurit Rseaux sociaux
Rseaux sociaux Rseaux
Rseaux La fiche de lecture la ficelle
La fiche de lecture la ficelle Immobile pendant la vie et je me promène durant ma mort
Immobile pendant la vie et je me promène durant ma mort Optimisation
Optimisation Powerstar
Powerstar Optimisateur de tournée
Optimisateur de tournée Optimisation plateforme logistique
Optimisation plateforme logistique Dynamic scheduling mobile solution
Dynamic scheduling mobile solution Ultrasound image optimisation
Ultrasound image optimisation Dr michael fenton
Dr michael fenton Tetris
Tetris Signe de flot et de glacon
Signe de flot et de glacon Tete de meduse hypertension portale
Tete de meduse hypertension portale Chiffrement par flot
Chiffrement par flot Signe du flot choc rotulien
Signe du flot choc rotulien Coupe minimale
Coupe minimale Signe du flot genou
Signe du flot genou Flot réalisable
Flot réalisable Flot tilburg
Flot tilburg Garder à flot
Garder à flot Les professeurs 1 of 1 dans la salle de classe.
Les professeurs 1 of 1 dans la salle de classe. Le guide de la parfaite epouse
Le guide de la parfaite epouse Texte dopinion
Texte dopinion Quel beau nom paroles
Quel beau nom paroles Les enfers dans la mythologie grecque
Les enfers dans la mythologie grecque Nous sommes les étoiles dans le ciel d'abraham paroles
Nous sommes les étoiles dans le ciel d'abraham paroles Les actions dans un conte
Les actions dans un conte Transmissions ciblées has
Transmissions ciblées has Marque allemande de bonbon
Marque allemande de bonbon Zone chaude zone froide supermarché
Zone chaude zone froide supermarché Echanges des substances dissoutes
Echanges des substances dissoutes Les instructions en classe
Les instructions en classe Situation de passage le dernier jour d'un condamné
Situation de passage le dernier jour d'un condamné Les remerciements dans un rapport de stage
Les remerciements dans un rapport de stage Les fonctions dans le groupe verbal
Les fonctions dans le groupe verbal Recherche dichotomique
Recherche dichotomique Initiation à la recherche en soins infirmiers
Initiation à la recherche en soins infirmiers Zb uzh recherche
Zb uzh recherche Moteur de recherche exemple
Moteur de recherche exemple Recherche photos
Recherche photos Recherche séquentielle
Recherche séquentielle Recherche inversée par image
Recherche inversée par image Nagpupugay kahulugan
Nagpupugay kahulugan