Measures of Central Tendency of Grouped Data Standard

Measures of Central Tendency of Grouped Data, Standard Deviation, Histograms and Frequency Polygons

Univariate Data • Concerned with a single attribute or variable. • Two forms of numerical data – DISCRETE data – collected by counting exact amounts – CONTINUOUS data – values form part of a continuous scale – generally collected by measurement

Grouped frequency Table • • For large volume of data. Group data in frequency table. Groups should not overlap. Use inequality signs for continuous data.

ORGANISING DATA • Can organise data using – a stem and leaf diagram/display – A frequency table – A grouped frequency table • Data could be discrete or continuous

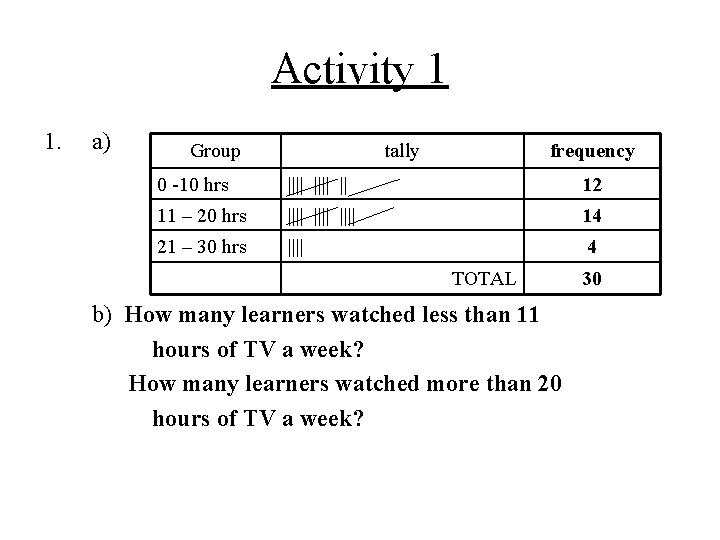
Activity 1 1. a) Group tally frequency 0 -10 hrs |||| || 12 11 – 20 hrs |||| 14 21 – 30 hrs |||| 4 TOTAL b) How many learners watched less than 11 hours of TV a week? How many learners watched more than 20 hours of TV a week? 30

2. a) Continuous data b) Interval Tally c) 11 learners Frequency || 2 ||| 3 |||| | 6 |||| || 7 |||| 5 ||| 3

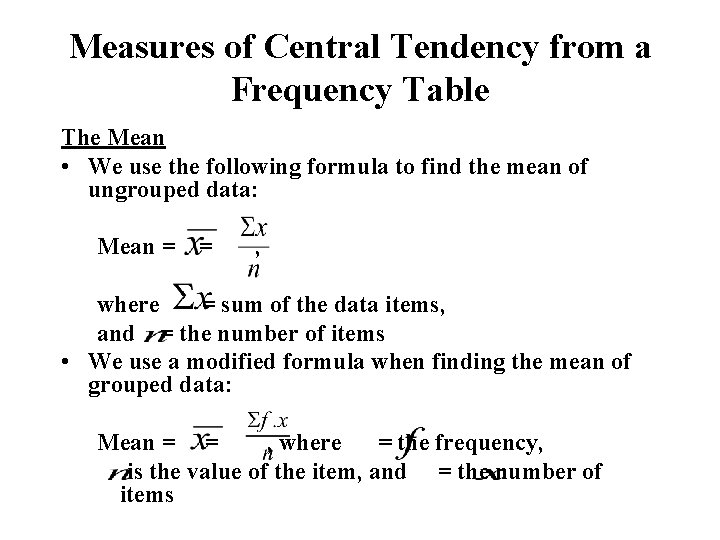
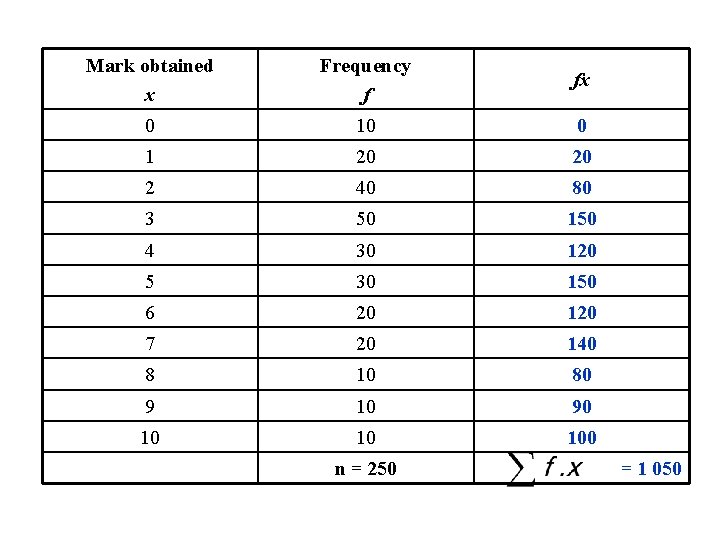
Measures of Central Tendency from a Frequency Table The Mean • We use the following formula to find the mean of ungrouped data: Mean = = , where = sum of the data items, and = the number of items • We use a modified formula when finding the mean of grouped data: Mean = = , where = the frequency, is the value of the item, and = the number of items

The median is the middle data item when the data is listed in order. We sometimes use the formula to find out which item is the middle item, and can also find the median from the frequency table. The mode is the data item with the highest frequency.

Mark obtained x Frequency f fx 0 10 0 1 20 20 2 40 80 3 50 150 4 30 120 5 30 150 6 20 120 7 20 140 8 10 80 9 10 90 10 10 100 n = 250 = 1 050

• Mean = 4, 2 • Median = 4 • Mode = 3

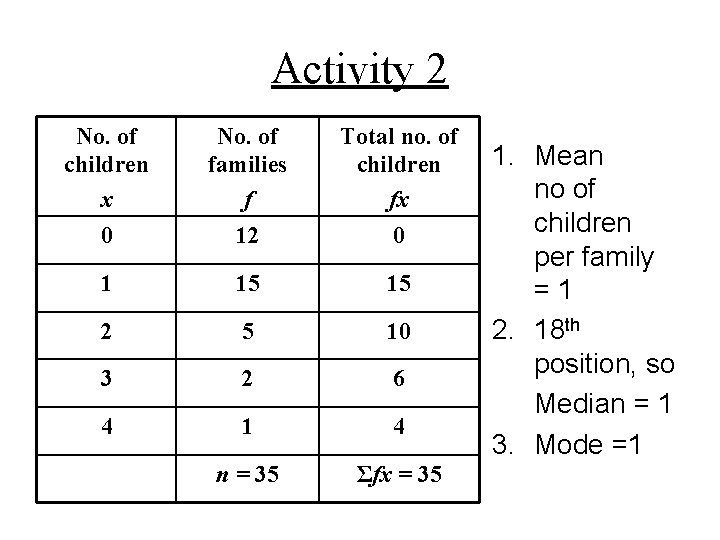
Activity 2 No. of children x 0 No. of families f 12 Total no. of children fx 0 1 15 15 2 5 10 3 2 6 4 1 4 n = 35 Σfx = 35 1. Mean no of children per family =1 2. 18 th position, so Median = 1 3. Mode =1

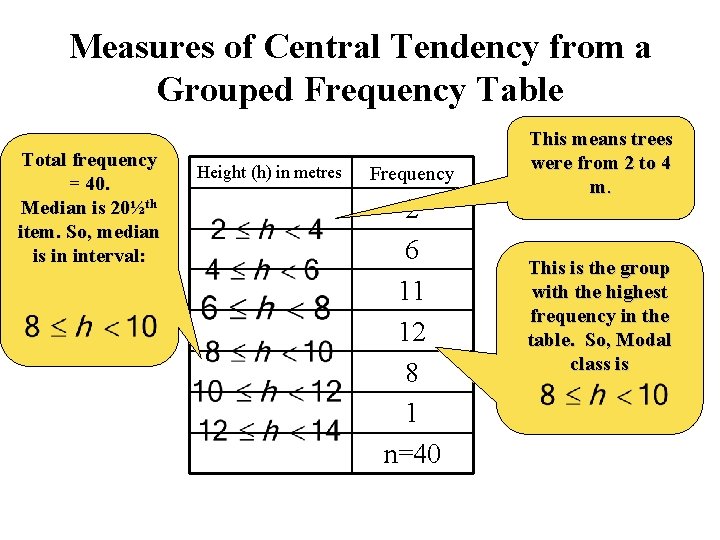
Measures of Central Tendency from a Grouped Frequency Table Total frequency = 40. Median is 20½th item. So, median is in interval: Height (h) in metres Frequency 2 6 11 12 8 1 n=40 This means trees were from 2 to 4 m. This is the group with the highest frequency in the table. So, Modal class is

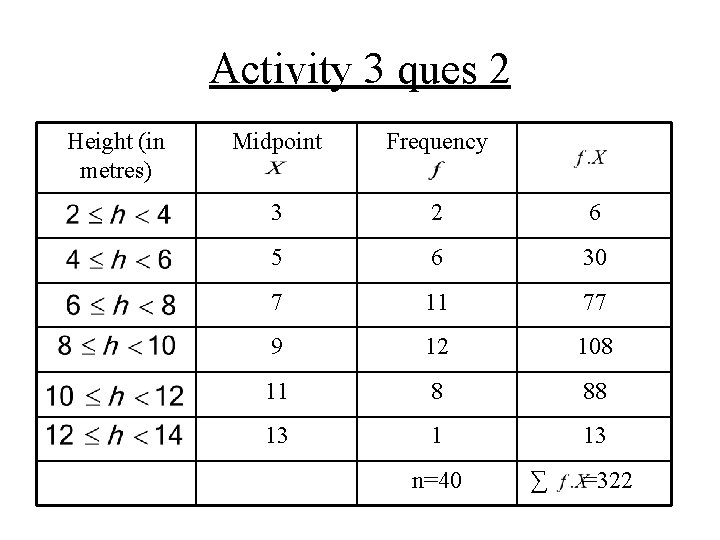
Activity 3 ques 2 Height (in metres) Midpoint Frequency 3 2 6 5 6 30 7 11 77 9 12 108 11 8 88 13 1 13 n=40 ∑ =322

b) Mean ≈ 8, 05 c) Median is 20, 5 th item. Median is in the interval Median ≈ d) Modal class is

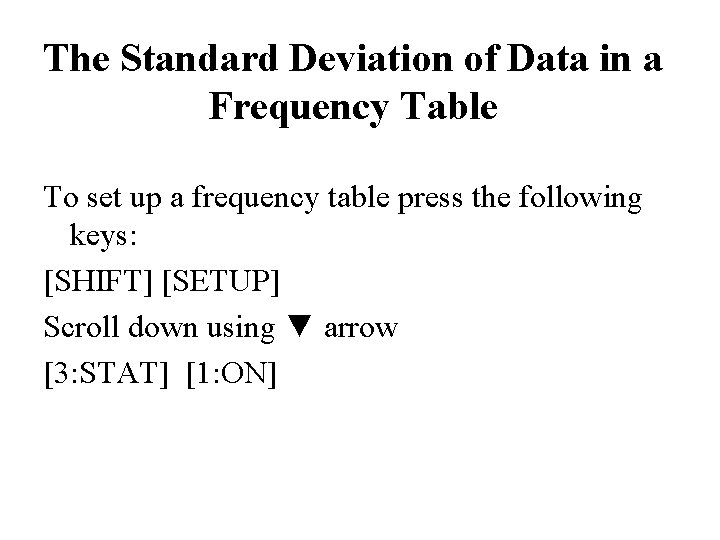
The Standard Deviation of Data in a Frequency Table To set up a frequency table press the following keys: [SHIFT] [SETUP] Scroll down using ▼ arrow [3: STAT] [1: ON]

Activity 4 3 a) Mean = 418 g b) Standard deviation = 21 g 4. (418 ± 21) = (418 – 21; 418 + 21) = (397; 439) 6 cans lie in this interval. = 31%

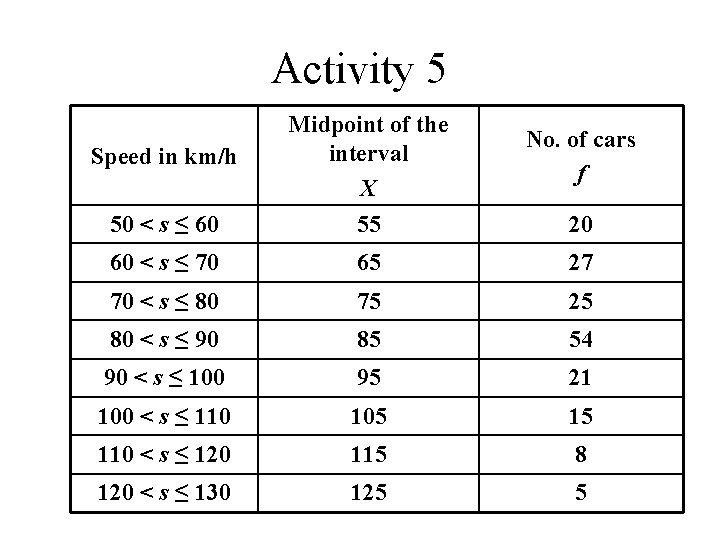
Activity 5 50 < s ≤ 60 Midpoint of the interval X 55 60 < s ≤ 70 65 27 70 < s ≤ 80 75 25 80 < s ≤ 90 85 54 90 < s ≤ 100 95 21 100 < s ≤ 110 105 15 110 < s ≤ 120 115 8 120 < s ≤ 130 125 5 Speed in km/h No. of cars f 20

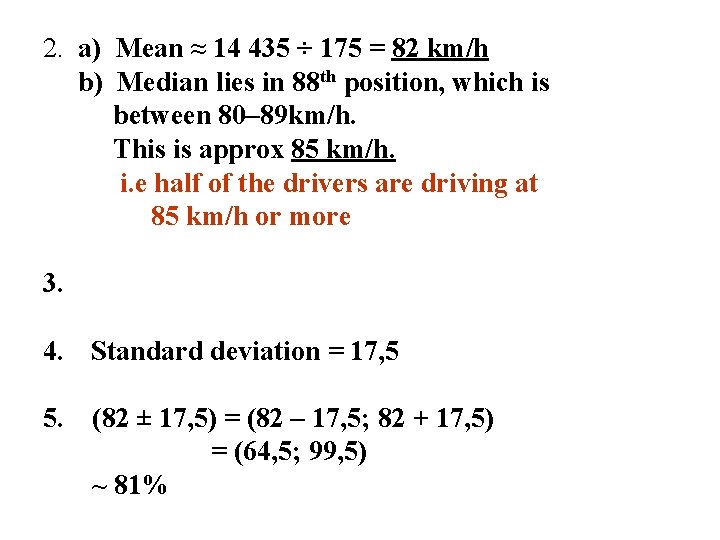
2. a) Mean ≈ 14 435 ÷ 175 = 82 km/h b) Median lies in 88 th position, which is between 80– 89 km/h. This is approx 85 km/h. i. e half of the drivers are driving at 85 km/h or more 3. 4. Standard deviation = 17, 5 5. (82 ± 17, 5) = (82 – 17, 5; 82 + 17, 5) = (64, 5; 99, 5) ~ 81%