Measures Of Central Tendancy cont Geometric Mean Geometric

Measures Of Central Tendancy (cont. ) Geometric Mean: Geometric mean is defined at the nth root of the product of n observations of a distribution. Symbolically, GM =. . . n√x 1……x 2……x 3…… If we have only two observations, say, 4 and 16 then GM = √ 4× 16 = √ 64 = 8. Similarly, if there are three observations, then we have to calculate the cube root of the product of these three observations; and so on. When the number of items is large, it becomes extremely difficult to multiply the numbers and to calculate the root. To simplify calculations, logarithms are used.

Example: If we have to find out the geometric mean of 2, 4 and 8, then we find Log GM = (∑ log xi)N = (Log 2 + Log 4 + Log 8)3 = (0. 3010 + 0. 6021+ 0. 9031)3 = (1. 8062)/3 = 0. 60206 GM = Antilog (0. 60206) =4

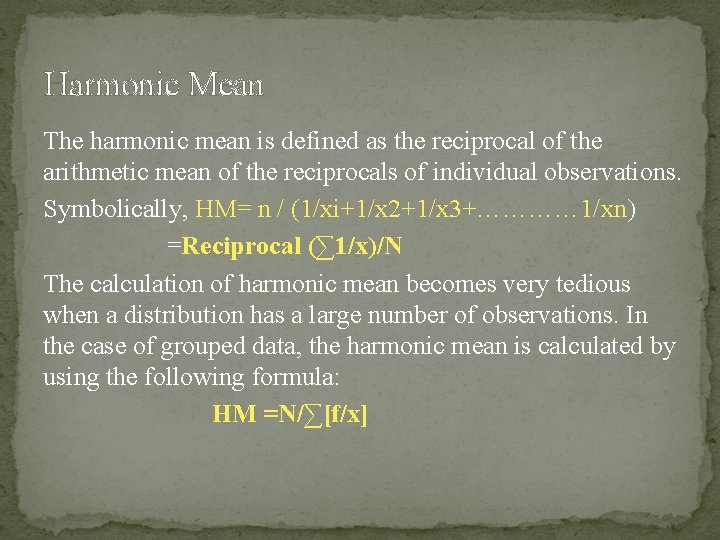
Harmonic Mean The harmonic mean is defined as the reciprocal of the arithmetic mean of the reciprocals of individual observations. Symbolically, HM= n / (1/xi+1/x 2+1/x 3+………… 1/xn) =Reciprocal (∑ 1/x)/N The calculation of harmonic mean becomes very tedious when a distribution has a large number of observations. In the case of grouped data, the harmonic mean is calculated by using the following formula: HM =N/∑[f/x]

Example: Suppose we have three observations 4, 8 and 16. We are required to calculate the harmonic mean. Reciprocals of 4, 8 and 16 are: 1/4 , 1/8 , 16 1 respectively Since HM = N/(1/ x 1+ 1/ x 2+1/ x 3) = 3(1/ 4 +1/ 8 +1/ 16) = 3/(0. 25 +0. 125 +0. 0625 )3 = 6. 857 (approx. )

Example: Consider the following series: Class-interval Frequency 2 -4 20 4 -6 40 6 -8 30 8 -10 10 Calculate Harmonic Mean.

Solution: Class-interval 2 -4 -6 8 10 Mid-value 3 5 7 9 =∑[f*1/x]/N = 100/20. 0641 = 4. 984 approx. Frequency Reciprocal of MV f /x 20 0. 3333 6. 6660 40 0. 2000 8. 0000 30 0. 1429 4. 2870 10 0. 1111 1. 1111 Total 20. 0641 4 68 -