LESSON 11 4 Areas of Regular Polygons 11

LESSON 11. 4 Areas of Regular Polygons 11 -4 Honors Geometry Today: • Bridge Questions • 11. 3 Assignment • Last problems of 11. 3 • 11. 4 Instruction

LESSON 11 -3 Areas of Circles and Sectors Find the area of: a segment of a circle whose chord is 12” and the measure of the intercepted arc is 120°. the checkered region. 6’

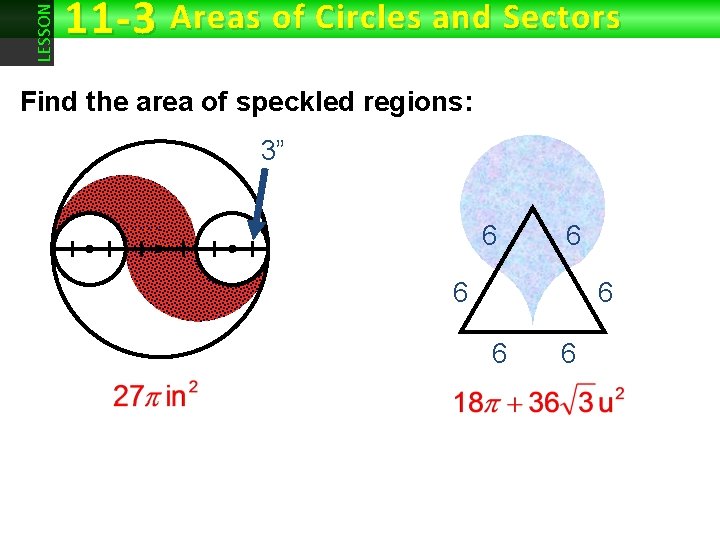
LESSON 11 -3 Areas of Circles and Sectors Find the area of speckled regions: 3” 6 6 6

LESSON 11 -4 11. 4 Areas of Regular Polygons &Composite Figures Objective: 1. Find areas of regular polygons. 2. Find areas of composite figures. Vocabulary: center of a regular polygon, radius of a regular polygon, apothem, central angle of a regular polygon, composite figure

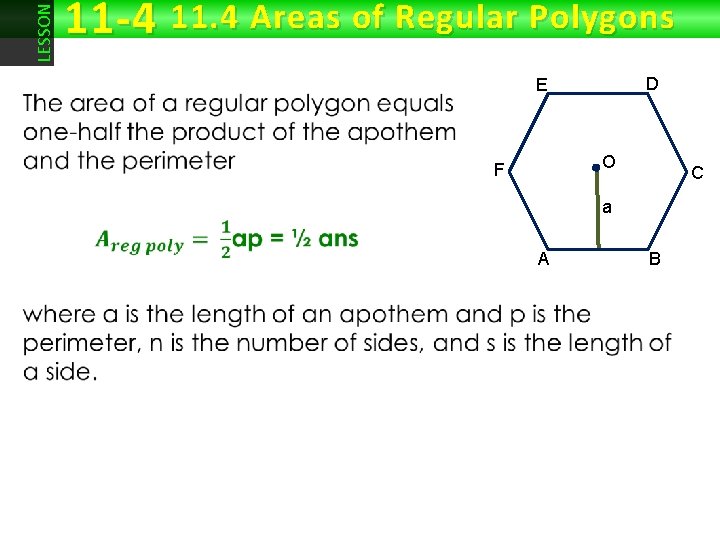
LESSON 11 -4 11. 4 Areas of Regular Polygons radius of a polygon- a segment joining the center of a circle to a point on the circumsribing circle apothem- a segment joining the center of a regular polygon to the midpoint of one of the polygon’s sides, a point on the inscribed circle. D E O F A P C B

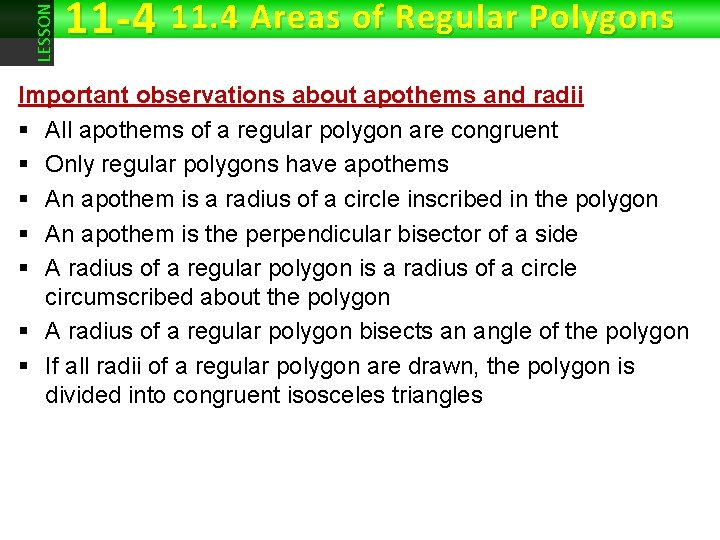
LESSON 11 -4 11. 4 Areas of Regular Polygons Important observations about apothems and radii § All apothems of a regular polygon are congruent § Only regular polygons have apothems § An apothem is a radius of a circle inscribed in the polygon § An apothem is the perpendicular bisector of a side § A radius of a regular polygon is a radius of a circle circumscribed about the polygon § A radius of a regular polygon bisects an angle of the polygon § If all radii of a regular polygon are drawn, the polygon is divided into congruent isosceles triangles

LESSON 11 -4 11. 4 Areas of Regular Polygons D E • O F C a A B

LESSON 11 -4 11. 4 Areas of Regular Polygons Advice: Find the area of a pentagon with sides of 5 m to the nearest tenth of a meter. 1. 2. 3. 4. 5. 6. Draw figure. Split into triangles. Find the central angle. Split triangle with apothem. Find missing measure. Calculate area. ≈ 43. 0 m 2

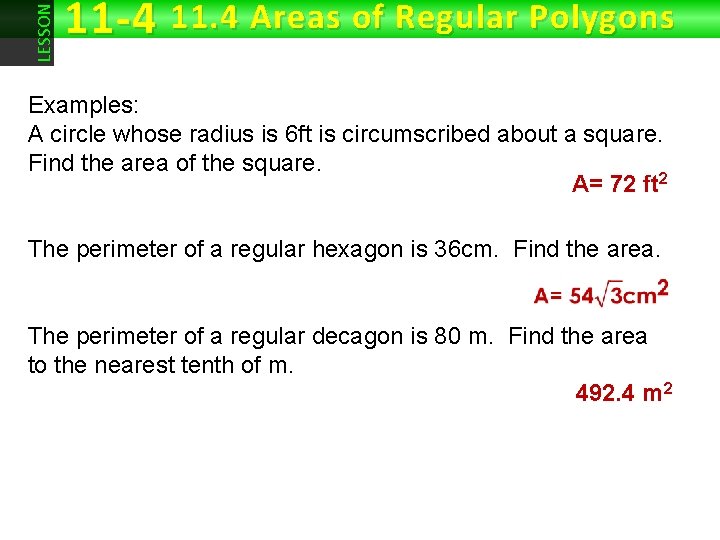
LESSON 11 -4 11. 4 Areas of Regular Polygons Examples: A circle whose radius is 6 ft is circumscribed about a square. Find the area of the square. A= 72 ft 2 The perimeter of a regular hexagon is 36 cm. Find the area. The perimeter of a regular decagon is 80 m. Find the area to the nearest tenth of m. 492. 4 m 2

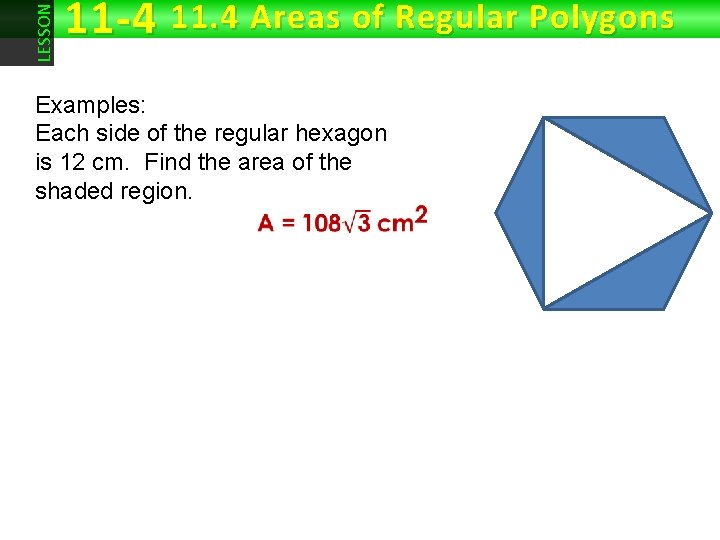
LESSON 11 -4 11. 4 Areas of Regular Polygons Examples: Each side of the regular hexagon is 12 cm. Find the area of the shaded region.

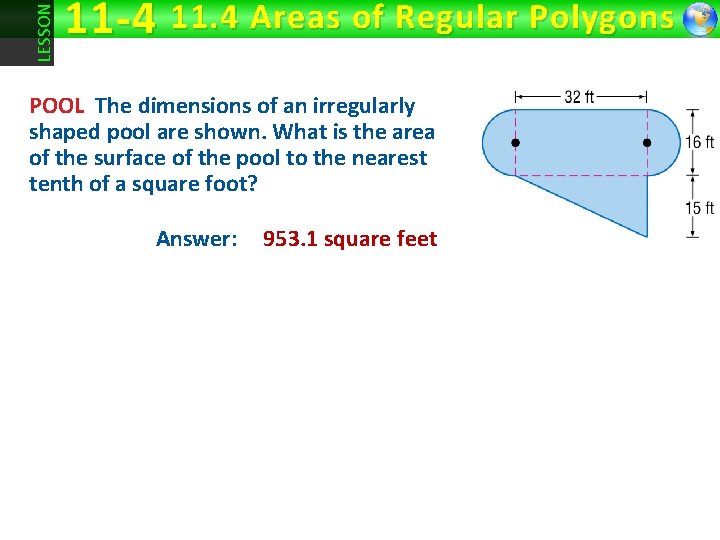
LESSON 11 -4 11. 4 Areas of Regular Polygons POOL The dimensions of an irregularly shaped pool are shown. What is the area of the surface of the pool to the nearest tenth of a square foot? Answer: 953. 1 square feet

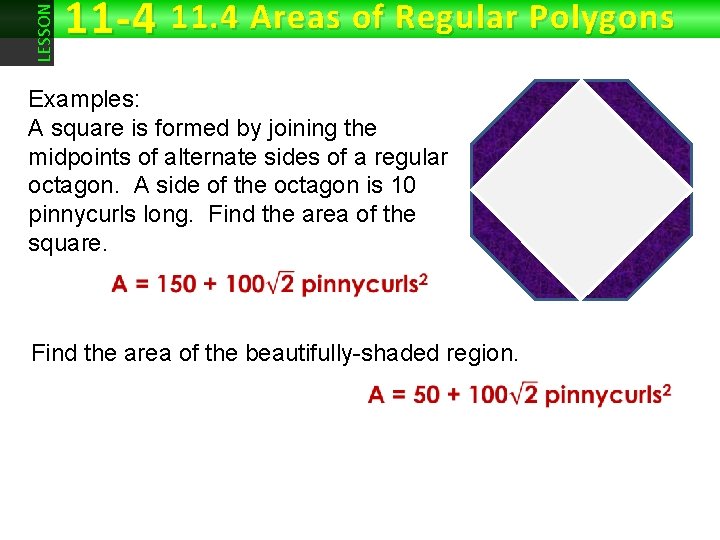
LESSON 11 -4 11. 4 Areas of Regular Polygons Examples: A square is formed by joining the midpoints of alternate sides of a regular octagon. A side of the octagon is 10 pinnycurls long. Find the area of the square. Find the area of the beautifully-shaded region.

LESSON 11 -4 11. 4 Areas of Regular Polygons Assignment Due tomorrow: • 11. 4 P. 811 #9 -29 odd, 32