KINETIC MOLECULAR THEORY Kinetic Molecular Theory A theory

KINETIC MOLECULAR THEORY

Kinetic Molecular Theory A theory that explains the physical properties of gases by describing the behavior of subatomic particles

Physical Properties of Gases: • Gases have mass • Gases are easily compressed • Gases completely fill their containers • Gases diffuse rapidly • Gases exert pressure

KMT has 5 postulates:

#1 A gas consists of very small particles that have mass. (molecules or atoms)

#2 Gas particles are very far apart from each other. (therefore gases are easily compressed)

#3 Gas particles are in continuous, rapid, random motion.

#4 Collisions of gas particles (with each other and with container) are perfectly elastic (no energy lost)

#5 The average energy of the gas particles depends on the temperature of the gas.

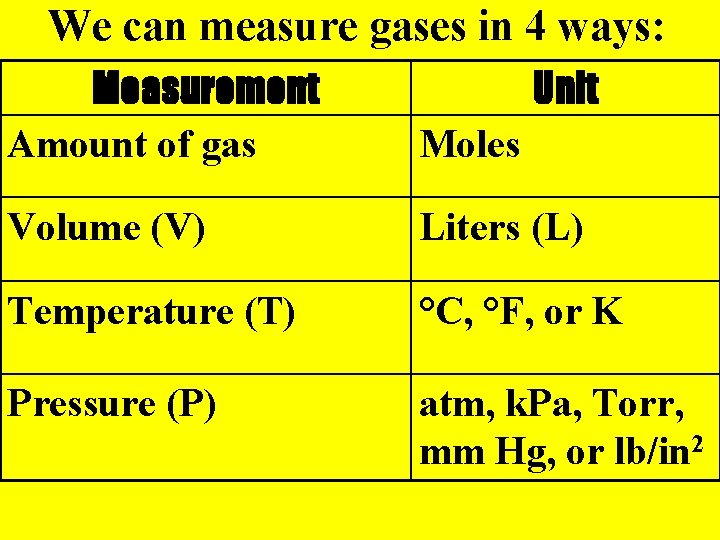
We can measure gases in 4 ways: Measurement Amount of gas Unit Moles Volume (V) Liters (L) Temperature (T) °C, °F, or K Pressure (P) atm, k. Pa, Torr, mm Hg, or lb/in 2

Temperature (T) A measurement of the average kinetic energy of a substance

Higher temperatures cause gas particles to move faster

K = °C + 273 14°C = ? K 14°C + 273 = 287 K To solve gas problems, the temp must always be in Kelvin (K) !!!

Pressure (P) The force per unit area on a surface

Pressure is caused by gas particles slamming into the container’s walls.

Units of Pressure: 1) atmosphere (atm) 2) kilopascal (k. Pa) 3) millimeters of Mercury (mm Hg) 4) Torr 5) Pounds per square inch (lb/in 2) 1 atm = 101. 3 k. Pa = 760 mm Hg = 760 torr = 14. 7 lb/in 2

STOP… collaborate and listen

Boyle’s Law P 1 V 1 = P 2 V 2 1 = before 2 = after MUST BE AT CONSTANT TEMPERATURE!!

A gas occupies a volume of 0. 458 L at a pressure of 1. 01 k. Pa and temperature of 295 Kelvin. Although the temperature stays the same, the volume is increased to 0. 477 L. What is the new pressure? 0. 970 k. Pa

Charles’ Law V 1 T 2 = V 2 T 1 1 = before 2 = after MUST BE AT CONSTANT PRESSURE!!

What will be the volume of a gas sample at 309 K if its volume at 215 K is 3. 42 L? Assume that pressure is constant. 4. 92 L

Gay-Lussac’s Law 1 = before 2 = after MUST BE AT CONSTANT VOLUME!!

A balloon with a pressure of 0. 900 atm is heated from 105 K to 155 K. If volume is held constant, what is the new pressure? 1. 33 atm

Confused yet? ? ? There’s an easier way…

Combined Gas Law 1 = before 2 = after JUST HOLD ONE QUANTITY CONSTANT!! (take it out of the equation)

The gas in a 0. 010 L container has a pressure of 1. 39 atmospheres. When the gas is transferred to a 0. 017 L container at the same temperature, what is the pressure of the gas? 0. 82 atm

The gas inside a 70. 8 m. L piston has a temperature of 35. 0°C. If the temperature is raised to 100. 0°C, what is the new volume of the gas? 85. 7 m. L

An elastic container is inflated to a volume of 18. 0 liters at a pressure of 1. 26 atm. The container then expands to a new volume at a pressure of 1. 05 atm. What is the new volume? 21. 6 liters

Avagadro’s Law Equal volumes of gas (at same P and T) contain the same amount of particles

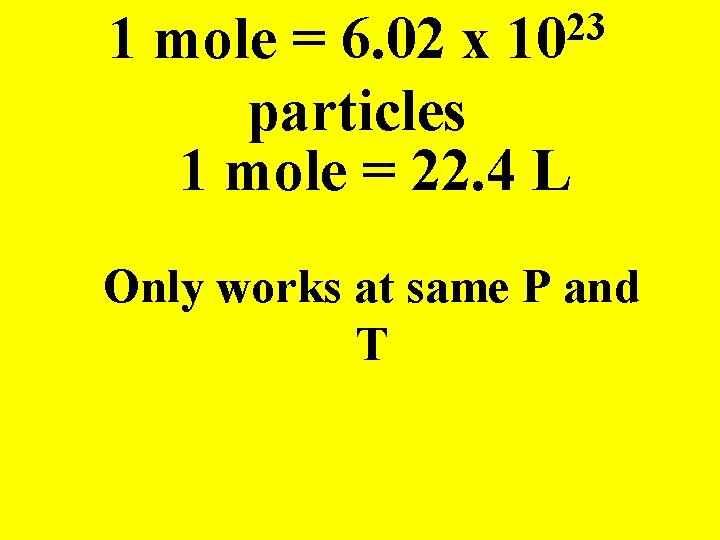
23 10 1 mole = 6. 02 x particles 1 mole = 22. 4 L Only works at same P and T

Ideal Gas Law The mother of all gas laws. It includes everything! PV = n. RT

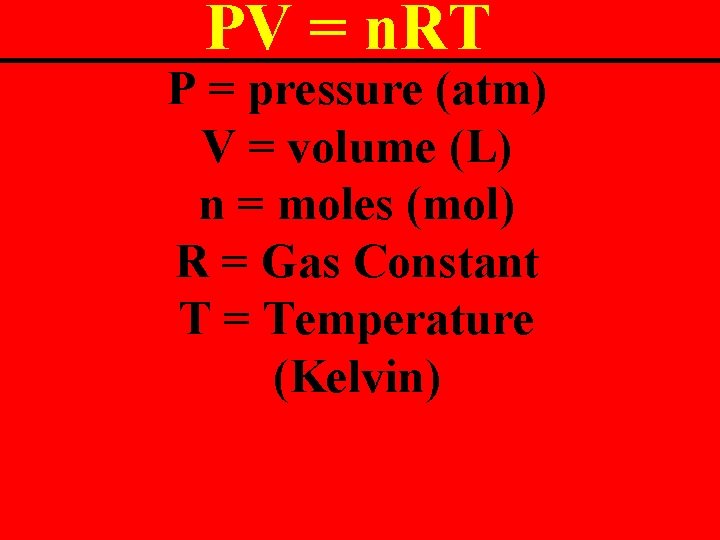
PV = n. RT P = pressure (atm) V = volume (L) n = moles (mol) R = Gas Constant T = Temperature (Kelvin)

PV = n. RT MUST USE THESE UNITS!!!

If the pressure exerted by a gas at 0. 00°C in a volume of 0. 0010 L is 5. 00 atm, how many moles of gas are present? 2. 2 x -4 10 moles

Ideal gases don’t really exist… …real gases do!

Real Gas All gases are real gases. DUH!!

Ideal Gas A gas that is described by the KMT postulates.

Ideal Gas Law works most of the time.

It does NOT work at very low temperatures and very high pressures.

Dalton’s Law of Partial Pressures The sum of the partial pressures of the gases is equal to the total pressure

Dalton’s Law of Partial Pressures Ptotal = P 1 + P 2 + P 3 + …

A balloon contains O 2 and N 2 gas. If the partial pressure of the O 2 is 0. 75 atm and the partial pressure of the N 2 is 0. 55 atm, what is the total pressure of the balloon? 1. 30 atm

The gas inside a 70. 8 m. L can contains H 2 O(g) and CO 2(g) and has a total pressure of 760 mm Hg. If the partial pressure of the H 2 O is 350 mm Hg, what is the partial pressure of the CO 2? 410 mm Hg

Graham’s Law Gases with smaller masses move faster than gases with large masses (like a kid in Walmart)

H 2 moves faster than N 2. Which of the following gases moves the fastest? O 2 Cl 2 Ar CO 2 I 2 NH 3 H 2 O Br 2

STP Standard Temperature and Pressure: 0°C and 1 atm

How many moles of N 2 gas are in a 4. 5 L balloon at STP? 0. 20 moles

A gas at STP is heated to 55°C. What is the new pressure if volume is held constant? 1. 2 atm

A gas tank contains CO 2 and O 2 gas. What is the total pressure in the tank if the partial pressure of CO 2 is 0. 45 atm and the partial pressure of O 2 is 0. 55? 1. 00 atm