Indeterminate Forms 00 We want to take the

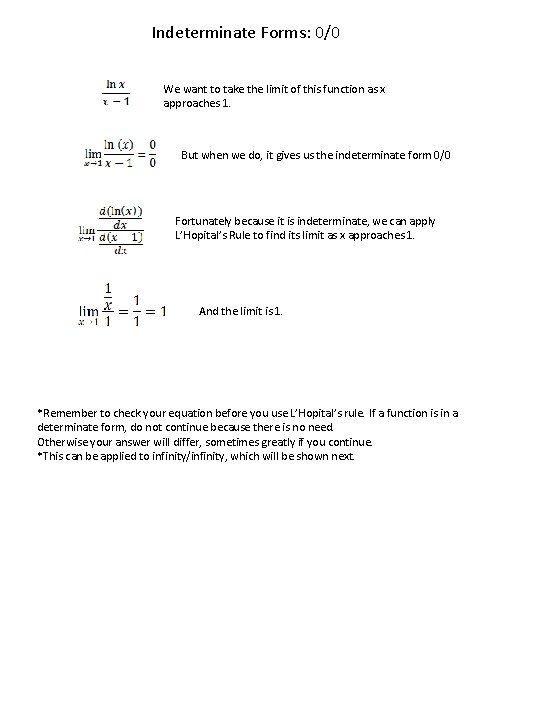
Indeterminate Forms: 0/0 We want to take the limit of this function as x approaches 1. But when we do, it gives us the indeterminate form 0/0 Fortunately because it is indeterminate, we can apply L’Hopital’s Rule to find its limit as x approaches 1. And the limit is 1. *Remember to check your equation before you use L’Hopital’s rule. If a function is in a determinate form, do not continue because there is no need. Otherwise your answer will differ, sometimes greatly if you continue. *This can be applied to infinity/infinity, which will be shown next.

Indeterminate Forms: Infinity/Infinity We want to find out what this function’s limit is as it approaches infinity. When we do, it is infinity/infinity. Fortunately because it is infinity/infinity, we can use L’Hopital’s rule straight up. We use L’Hopital’s Rule, and we get an indeterminate of 0/0. Now we take the bottom part of the fraction, 1/((x+3)ln 3) and multiply the entire fraction by its reciprocal as shown above. We then take the limit of the function that is remaining, and we get our limit ln 3/ln 2.

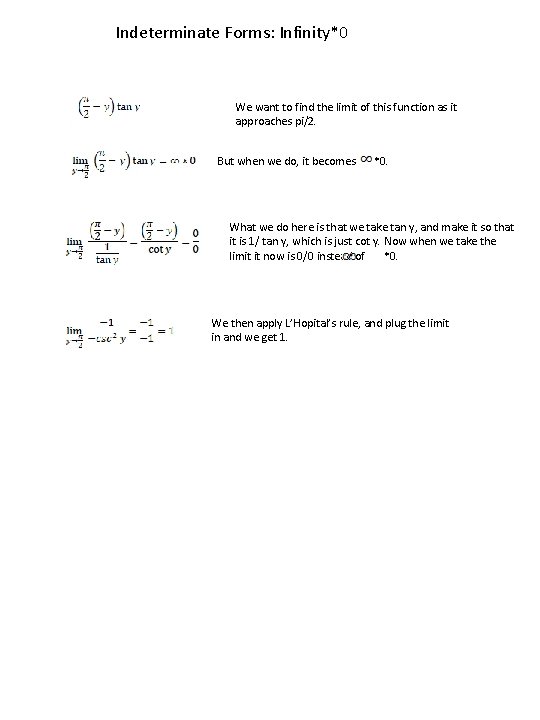
Indeterminate Forms: Infinity*0 We want to find the limit of this function as it approaches pi/2. But when we do, it becomes *0. What we do here is that we take tan y, and make it so that it is 1/ tan y, which is just cot y. Now when we take the limit it now is 0/0 instead of *0. We then apply L’Hopital’s rule, and plug the limit in and we get 1.

Indeterminate Form: Infinity-Infinity We want to find the limit of this function as it approaches 0, but it is the indeterminate form infinity – infinity. So what we do is we try to get a common denominator, and fuse three separate fractions into one giant fraction and take its limit, but it is still indeterminate. This time we can apply L’Hopital’s Rule directly. We derive the functions, and it turns out it is still indeterminate. So we use L’Hopital’s Rule again, and the limit is 1.

Indeterminate Forms: 0^0, 1^infinity, etc. We take the limit of our function, but it is another of the indeterminate forms, 0^0. So we put this function under e^ln(u), and move the tan x at the top to the bottom, giving us cot x. Then we take the limit, but it gives us infinity/infinity. Then we apply L’Hopital’s rule to get the limit, but it still is infinity over infinity. So now we multiply the fraction by sin^2 (x) , which gives us cos x sin x. We then take the limit of the function, which gives us 0. Then it is applied to the exponential function, giving us the true limit of one.