Grade DE Problems involving ratios Solve problems involving

Grade D/E Problems involving ratios Solve problems involving ratios, e. g. conversion, comparison, scaling, mixing, concentrations If you have any questions regarding these resources or come across any errors, please contact helpful-report@pixl. org. uk

Key Vocabulary Ratio Parts Common units

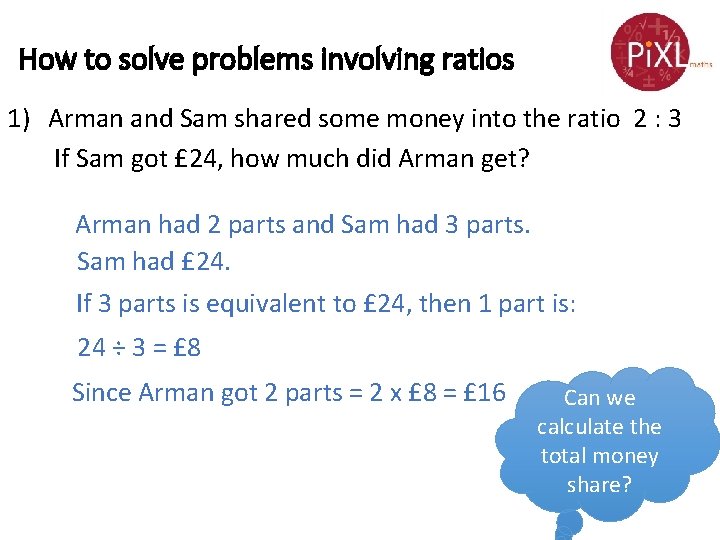
How to solve problems involving ratios 1) Arman and Sam shared some money into the ratio 2 : 3 If Sam got £ 24, how much did Arman get? Arman had 2 parts and Sam had 3 parts. Sam had £ 24. If 3 parts is equivalent to £ 24, then 1 part is: 24 ÷ 3 = £ 8 Since Arman got 2 parts = 2 x £ 8 = £ 16 Can we calculate the total money share?

How to solve problems involving ratios 2) Here are the ingredients for 8 cupcakes. Jennie has 1. 2 kg flour and plenty of other ingredients. How many cupcakes can she make? 200 g flour 150 g sugar 125 g butter 3 eggs 50 g cocoa powder For every 8 cupcakes, she uses 200 g flour 200 ÷ 8 = 25 g flour needed for each cupcake 1. 2 kg = 1200 g ÷ 25 g = 48 cupcakes can be made by Jennie.

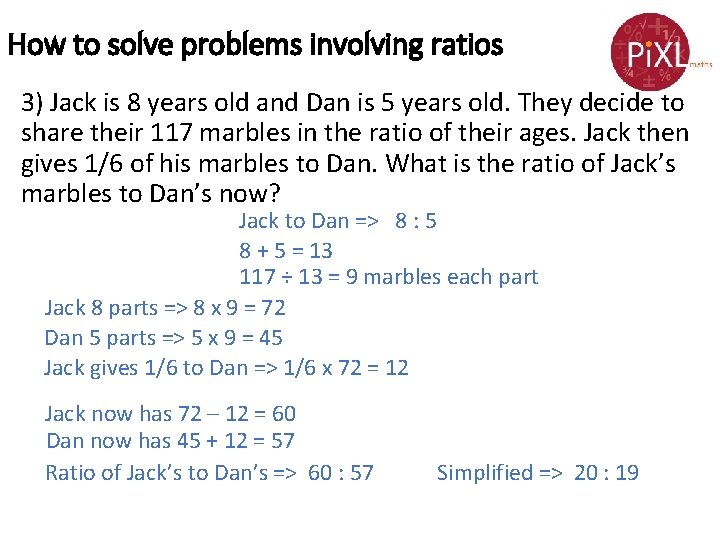
How to solve problems involving ratios 3) Jack is 8 years old and Dan is 5 years old. They decide to share their 117 marbles in the ratio of their ages. Jack then gives 1/6 of his marbles to Dan. What is the ratio of Jack’s marbles to Dan’s now? Jack to Dan => 8 : 5 8 + 5 = 13 117 ÷ 13 = 9 marbles each part Jack 8 parts => 8 x 9 = 72 Dan 5 parts => 5 x 9 = 45 Jack gives 1/6 to Dan => 1/6 x 72 = 12 Jack now has 72 – 12 = 60 Dan now has 45 + 12 = 57 Ratio of Jack’s to Dan’s => 60 : 57 Simplified => 20 : 19

Solve problems involving ratios – Now you try… 1) The cost of 14 pens is £ 38. 50. Find the cost of 5 pens. 2) A recipe for 15 people requires 150 g butter, 70 g sugar, 250 g flour and 10 g cocoa powder. Adapt the recipe for 9 people. 3) Andy drove to a shopping centre 12 km away at an average speed of 40 km/h. It took her 15 minutes to come back home. In which part of the journey was she faster? The speed limit was 30 mph. Did she go over the speed limit?

Solve problems involving ratios – Now you try… 1) Cost of 14 pens is £ 38. 50. Find the cost of 5 pens. 1) 13. 75 2) Recipe for 15 people requires 150 g butter, 70 g sugar, 250 g flour and 10 g cocoa powder. Adapt the recipe for 9 people. 2) 90 g butter, 42 g sugar, 150 g flour, 6 g cocoa powder 3) Andy drove to a shopping centre 12 km away at an average speed of 40 km/h. It took her 15 minutes to come back home. In which part of the journey she was faster? Speed limit was 30 mph. Did she go over the speed limit? 3) To shopping centre: 40 km/h=24. 8 mph under speed limit Going home: 60 km/h = 37 mph – faster but over the speed limit

Problem Solving and Reasoning Lucy wants to buy an economical car. Which car should Lucy choose- orange advert or green advert? With our car you can do 315 miles with 10 gallons of petrol. With our car you can do 210 miles with 28 litres of petrol.

Problem Solving and Reasoning Lucy wants to buy an economical car. Which car should Lucy choose- orange advert or green advert? With our car you can do 315 miles with 10 gallons of petrol. With our car you can do 210 miles with 28 litres of petrol. Orange advert: 315 miles per 10 gallons. 315 ÷ 10 = 31. 5 miles per gallon Green advert: 210 miles per 28 litres. 210 ÷ 28 = 7. 5 miles per litre We know that 1 gallon is approximately 4. 5 litres For every 4. 5 litres, the orange advert car goes 31. 5 miles. 31. 5 ÷ 4. 5= 7 miles Lucy should choose the car in the green advert as it travels more miles per litre of petrol.

Reason and explain • List the possible methods that can be used when calculating recipes. • What does best buy mean? Where can you use it in real life? • Which of the below is best buy 25 teabags for £ 1. 35 70 tea bags for £ 2. 55 100 teabags for £ 3. 70