Grade 12 AP Calculus MCV 4 UE Chapter












- Slides: 23

Grade 12 (AP Calculus -MCV 4 UE) Chapter 4: Applications of Derivatives Linearization and Newton’s Method Mr. Choi © 2019 E. Choi – MCV 4 UE - All Rights Reserved

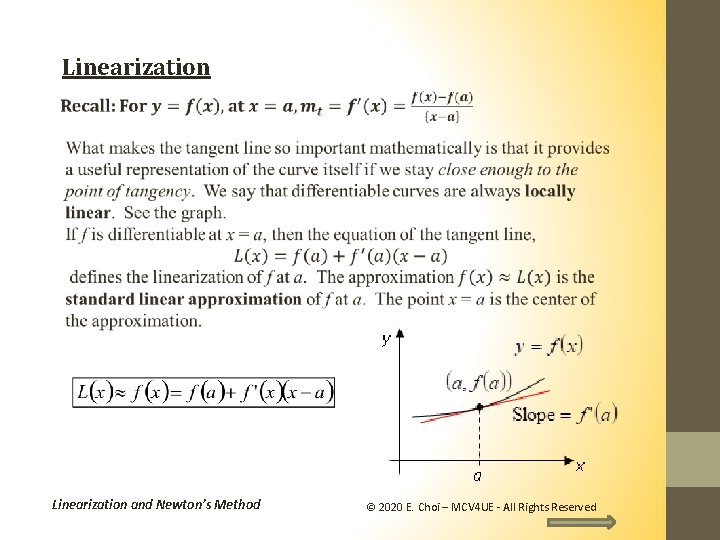
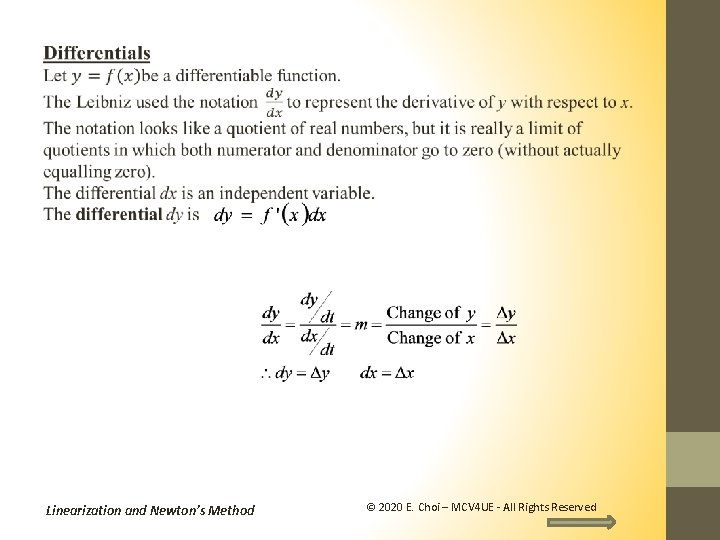
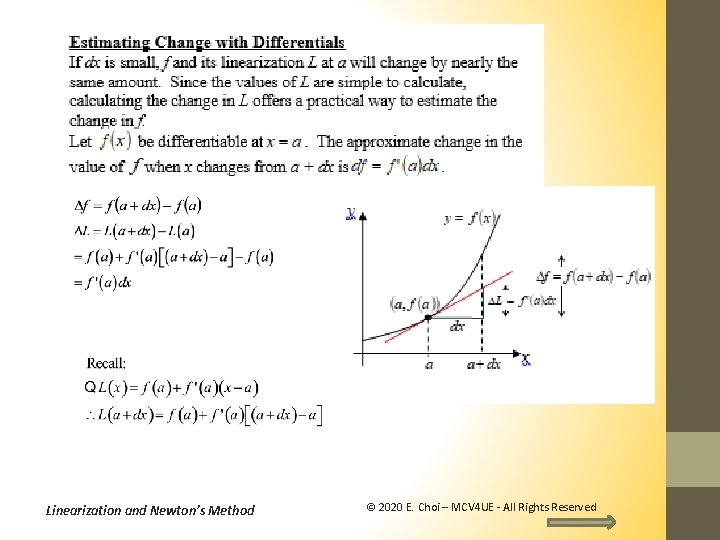
Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Why Linearization? Real systems are inherently nonlinear. (Linear systems do not exist!) Ex. f(t)=Kx(t), v(t)=Ri(t) Nonlinear systems are difficult to deal with mathematically. Many control analysis/design techniques are available for linear systems. Linear approximation is often good enough for control system analysis and design purposes. Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

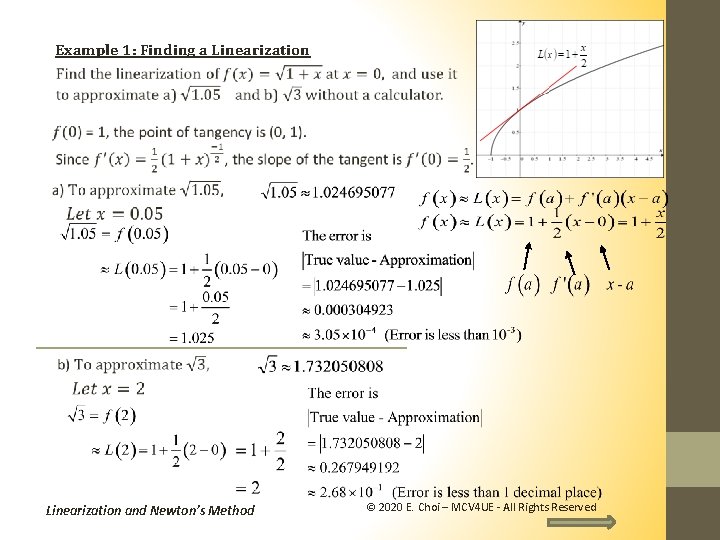
Example 1: Finding a Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization can never replace a calculator when it comes to find square roots. As we move away from zero (the center of the approximation), we lose accuracy and the approximation becomes less useful. Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

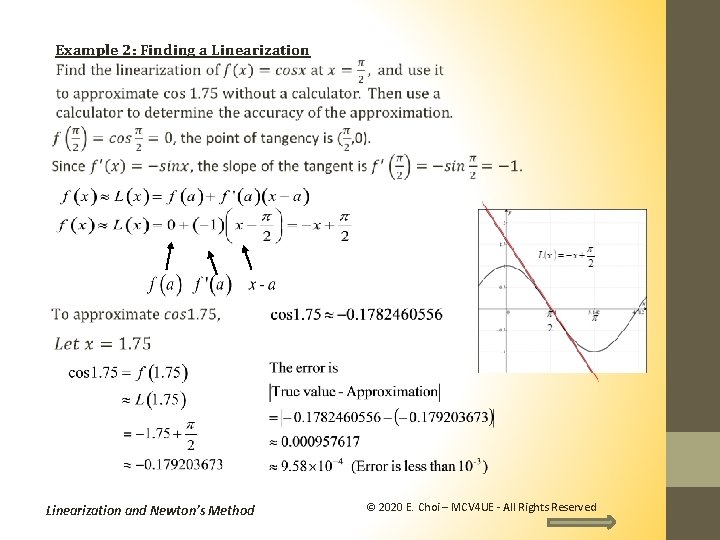
Example 2: Finding a Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

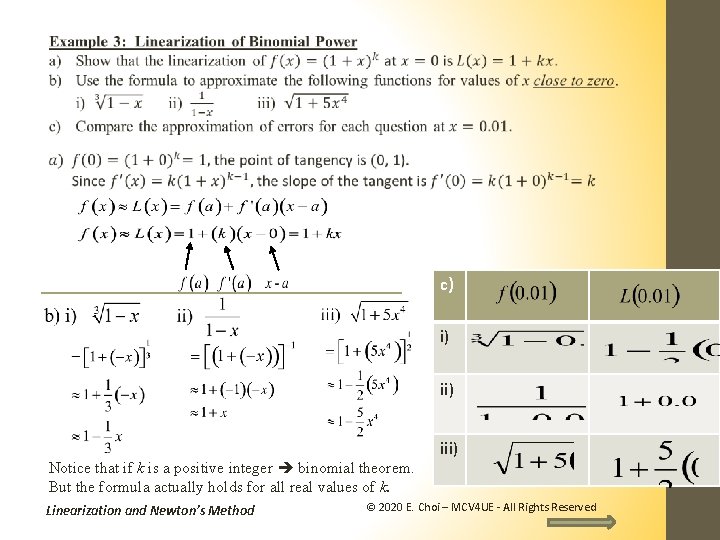
c) i) ii) Notice that if k is a positive integer binomial theorem. But the formula actually holds for all real values of k. Linearization and Newton’s Method iii) © 2020 E. Choi – MCV 4 UE - All Rights Reserved

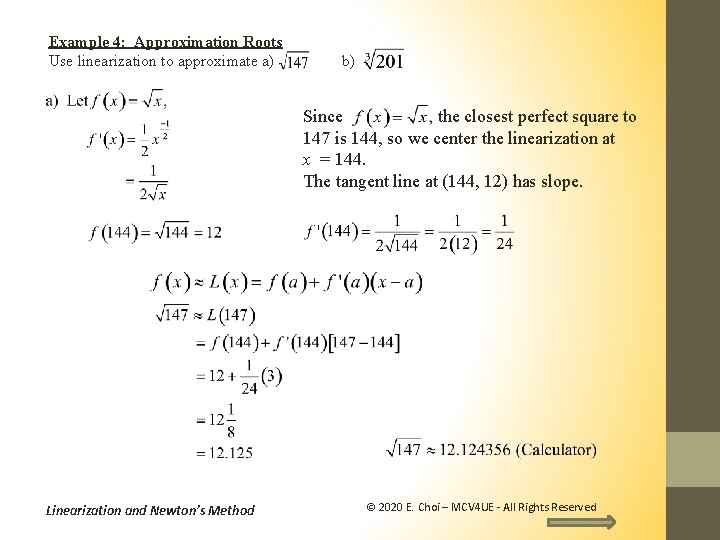
Example 4: Approximation Roots Use linearization to approximate a) b) Since the closest perfect square to 147 is 144, so we center the linearization at x = 144. The tangent line at (144, 12) has slope. Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Example 4: Approximation Roots Use linearization to approximate a) b) Since the closest cubic root to 201 is 216, so we center the linearization at x = 216. The tangent line at (216, 6) has slope. Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

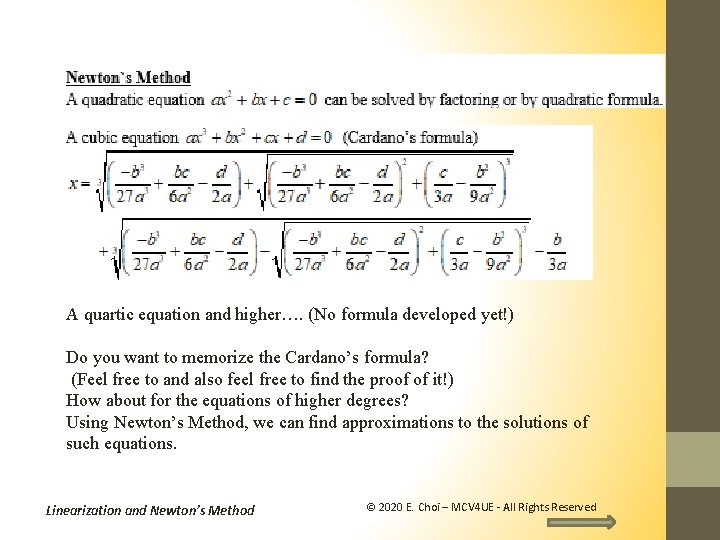
A quartic equation and higher…. (No formula developed yet!) Do you want to memorize the Cardano’s formula? (Feel free to and also feel free to find the proof of it!) How about for the equations of higher degrees? Using Newton’s Method, we can find approximations to the solutions of such equations. Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

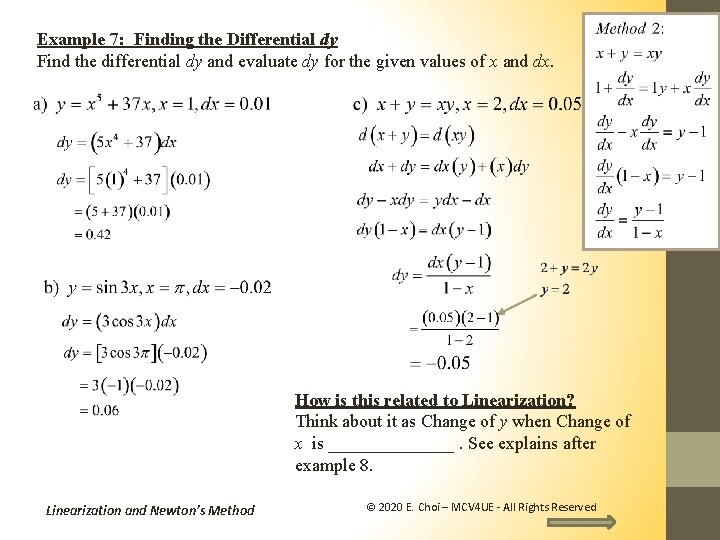
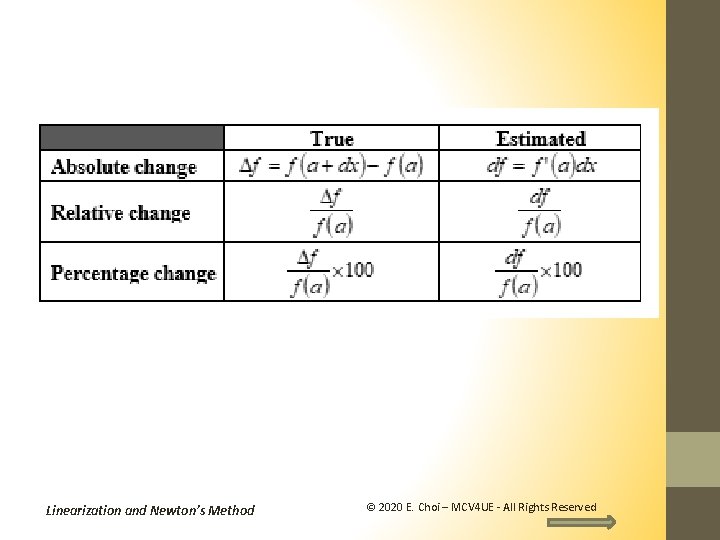
Example 7: Finding the Differential dy Find the differential dy and evaluate dy for the given values of x and dx. How is this related to Linearization? Think about it as Change of y when Change of x is _______. See explains after example 8. Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Example 8: Finding Differentials of Functions Find the differentials of the followings Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Tolerance is a permissible differences, allowing some freedom to move within limits. Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

GTA: 2751 square mile (About 3. 6 GTA) Richmond Hill: 39 square mile (About 255 Richmond Hill) Therefore the effect of the tolerance is approx. . 9950 square mile. (25770 km 2) Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

Homework Textbook: P. 242 #1 -7, 9 -13, 19 -33, 37 -40, 46 -54 Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved

End of lesson Linearization and Newton’s Method © 2020 E. Choi – MCV 4 UE - All Rights Reserved
 Seuil d'indifférence calcul
Seuil d'indifférence calcul Trapped plasma
Trapped plasma Menghitung mcv mch mchc
Menghitung mcv mch mchc Schilling
Schilling Trombosit histogram
Trombosit histogram Bmp blood test
Bmp blood test How to measure mcv
How to measure mcv Qualitative reduction
Qualitative reduction Advia hematology analyzer
Advia hematology analyzer Rumus mcv mch mchc
Rumus mcv mch mchc Pemeriksaan darah perifer lengkap
Pemeriksaan darah perifer lengkap Indici eritrocitari mcv
Indici eritrocitari mcv Eos baso
Eos baso Normochromic
Normochromic Calculus chapter 5
Calculus chapter 5 Stewart calculus chapter 11
Stewart calculus chapter 11 Limits
Limits Ap calc ab chapter 5
Ap calc ab chapter 5 Precalculus honors chapter 1 test
Precalculus honors chapter 1 test Calculus chapter 3
Calculus chapter 3 Calculus chapter 12
Calculus chapter 12 Chapter p preparation for calculus
Chapter p preparation for calculus What is calculus for
What is calculus for College grade equivalent
College grade equivalent