Golden Number and Arts Ars sine Scientia Nihil

Golden Number and Arts Ars sine Scientia Nihil! Gheorghe Samoilă, researcher Click to advance, or let it run by itself!

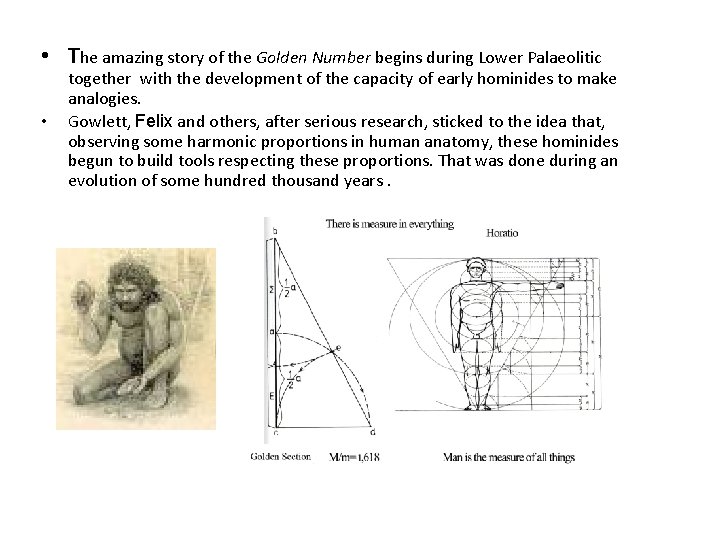
• The amazing story of the Golden Number begins during Lower Palaeolitic • together with the development of the capacity of early hominides to make analogies. Gowlett, Felix and others, after serious research, sticked to the idea that, observing some harmonic proportions in human anatomy, these hominides begun to build tools respecting these proportions. That was done during an evolution of some hundred thousand years.

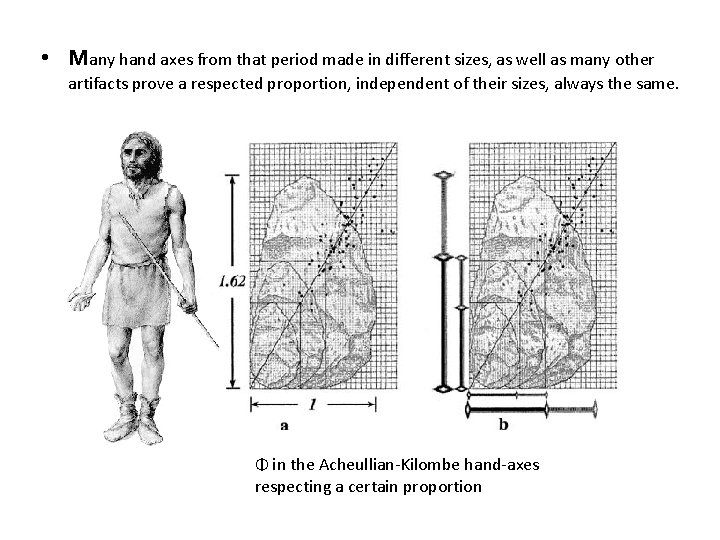
• Many hand axes from that period made in different sizes, as well as many other artifacts prove a respected proportion, independent of their sizes, always the same. Φ in the Acheullian-Kilombe hand-axes respecting a certain proportion

• Much later, the Egyptians include in their architectural design this Number. We • know this from the analyse of their monuments, tombs, ritual objects. Giza Pyramide, Ramesses Tomb, cult objects. But the mathematics formalizing this ratio has been not discovered in a written form.

• The formulation of this proportion has been made later by the old Greeks. The Pythagorean School, Plato later, they laid down the bases for geometric studies of this, calling the fascinating proportion “the number” which put order into the whole Universe (Plato-Timaeus). And Euclid wrote in his Geometry about this, letting the first written document about. Vitruvius called this number "analogia" giving a beautiful definition for the Symmetry: • “Symmetry, said he, resides in the correlation by measurement between various elements of the plan, and between each of these elements and the whole… As in the human body…It proceeds from proportion and it achieves consonance between every part and the whole…This symmetry is regulated by the modulus, the standard of common measure which the Greeks called the number” [1].

• • • What is in fact this “Golden Number”, called in this way later and having being given by the old Greeks the symbol Φ : Let’s try a geometrical approach dividing a segment of a straight line into two unequal parts in such a way that the ratio between the whole segment AB and the greater segment AC, equals the ratio between the same greater segment AC and the smaller segment CB. This ratio is always (no matter the segments size) 1, 618… cu 1, 618. . . A________C_____B This division is called the division between extreme and mean ratio. It is an irrational number calculated applying Pithagora’s formulae equalling (1+√ 5)/2=1, 618 and noted as Φ. Luca Pacioli called him later “The Divine Proportion”, and Johannes Keppler “one of the Two Jewels of Geometry”

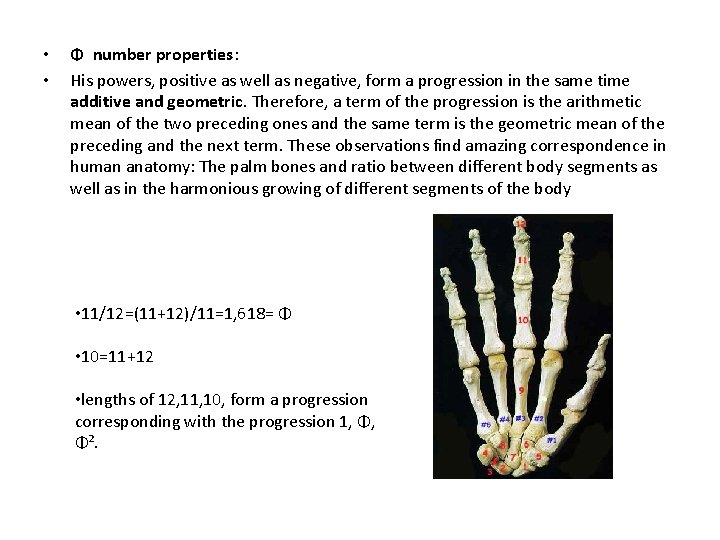
• • Φ number properties: His powers, positive as well as negative, form a progression in the same time additive and geometric. Therefore, a term of the progression is the arithmetic mean of the two preceding ones and the same term is the geometric mean of the preceding and the next term. These observations find amazing correspondence in human anatomy: The palm bones and ratio between different body segments as well as in the harmonious growing of different segments of the body • 11/12=(11+12)/11=1, 618= Φ • 10=11+12 • lengths of 12, 11, 10, form a progression corresponding with the progression 1, Φ, Φ².

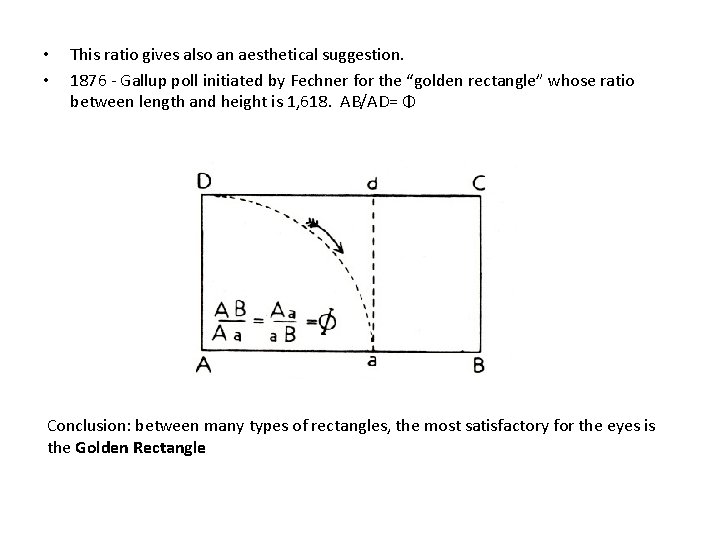
• • This ratio gives also an aesthetical suggestion. 1876 - Gallup poll initiated by Fechner for the “golden rectangle” whose ratio between length and height is 1, 618. AB/AD= Φ Conclusion: between many types of rectangles, the most satisfactory for the eyes is the Golden Rectangle

The Golden Rectangle can be divided building a square on his smaller side and the rest from the area will be also a golden rectangle. This division can be repeted infinitely getting a smaller and smaller serie of rectangles having the area diminished constantly with 1/Φ 2.

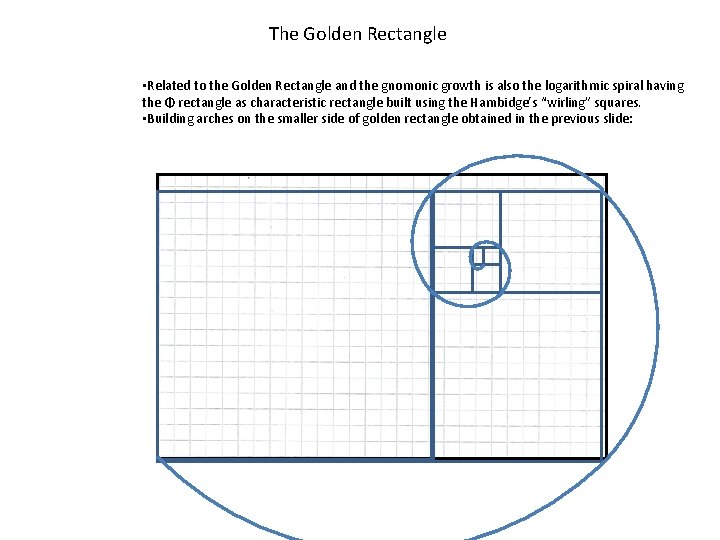
The Golden Rectangle • Related to the Golden Rectangle and the gnomonic growth is also the logarithmic spiral having the Φ rectangle as characteristic rectangle built using the Hambidge’s “wirling” squares. • Building arches on the smaller side of golden rectangle obtained in the previous slide:

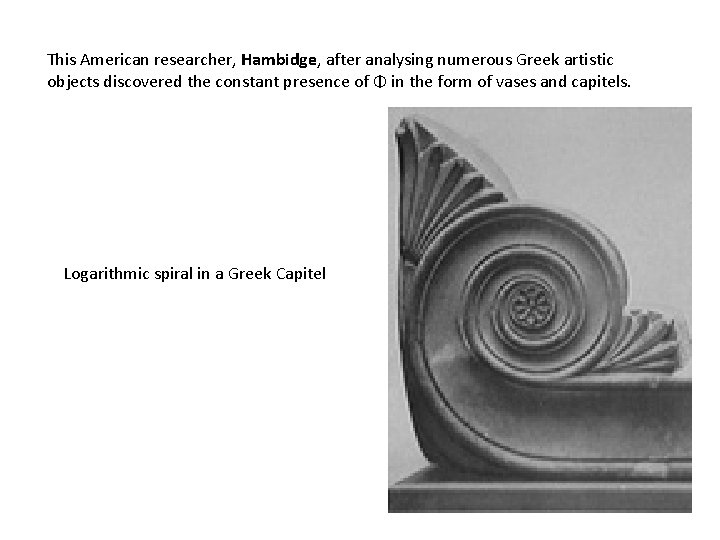
This American researcher, Hambidge, after analysing numerous Greek artistic objects discovered the constant presence of Φ in the form of vases and capitels. Logarithmic spiral in a Greek Capitel

Zeissing, in 1850 noted the presence of the Golden Number in the frontal view of the Parthenon. AC/AD=DC/AC= Φ

Φ is also present in Painting of the Renaissance and here Leonardo da Vinci has to be mentioned, Durrer and many others. Here we have the harmonic analyse of Mona Lisa Internet image

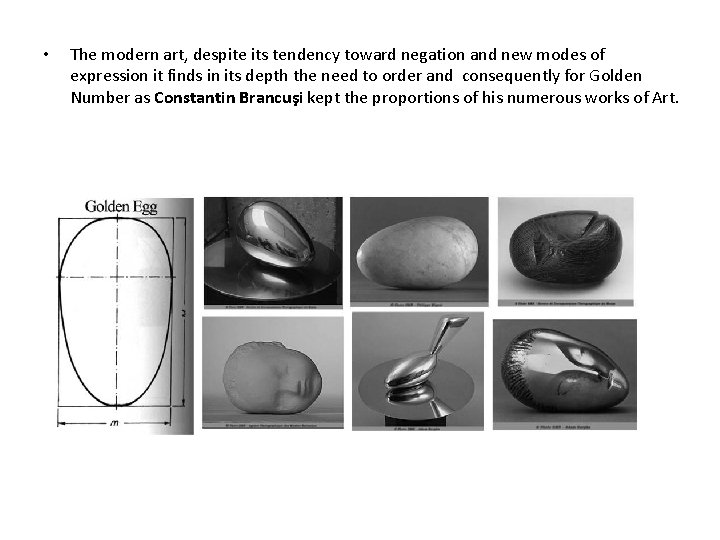
• The modern art, despite its tendency toward negation and new modes of expression it finds in its depth the need to order and consequently for Golden Number as Constantin Brancuşi kept the proportions of his numerous works of Art.

Seurat divisionism-Harmonic analysis of Le pont de Courbevoie Innovation in composition: using the Golden Number in composition (more important than the painting technique (pointillism))

Salvador Dali, an authentic modernist applied in his Leda and the Swan the rules of Golden Number Internet image

• Matila Ghyka, the amazing Romanian who has given an exceptional synthesis on the Golden Number, especially through his book The Geometry of Art and Life, exercised a considerable influence toward some modern artists as we have seen with Salvador Dali in the previous slide.

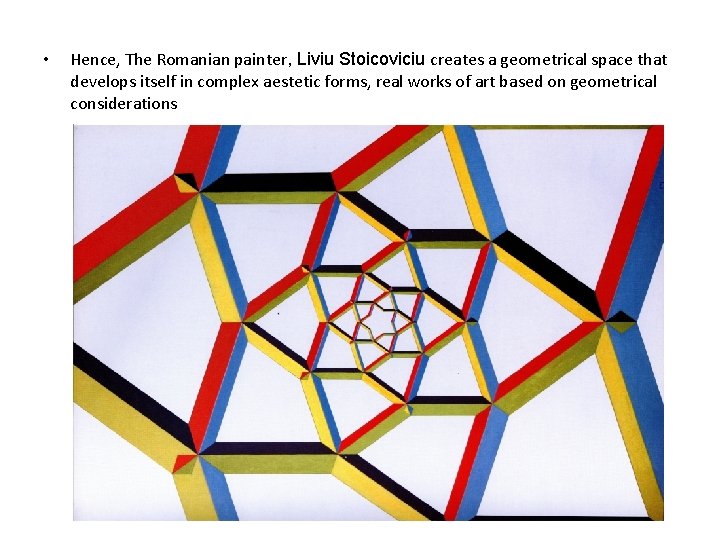
• Hence, The Romanian painter, Liviu Stoicoviciu creates a geometrical space that develops itself in complex aestetic forms, real works of art based on geometrical considerations

See his works in the exhibition!