Establishing the Recurrence Relation What is to be

Establishing the Recurrence Relation

What is to be learned n How to find the formulae for linear recurrence relations that are not arithmetic or geometric

4 8 20 56…. U 1 U 2 U 3 U 4 Is of form Un+1 = m. Un + c Un+1 = 3 Un – 4 so U 2 = m. U 1 + c 8 = m(4) + c U 5 = 164 U 3 = m. U 2 + c 20= m(8) + c 4 m + c = 8 8 m + c = 20 4 m = 12 m=3 c=-4

An ant colony are suffering from an outbreak of disease and worker ants are reducing by a certain percentage weekly. Being resourceful creatures they are capturing an extra ants to work for them each week A 0 There were initially 1400 worker ants. A week later there are 1220. A 1 The following week, there are 1076. A 2 What percentage of ants are dying? How many ants are they capturing? An+1 = m. An + c

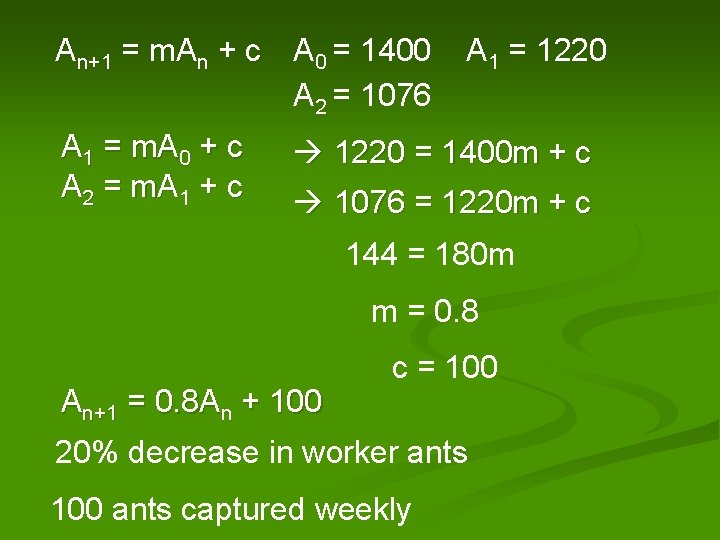
An+1 = m. An + c A 0 = 1400 A 2 = 1076 A 1 = m. A 0 + c A 2 = m. A 1 + c A 1 = 1220 = 1400 m + c 1076 = 1220 m + c 144 = 180 m m = 0. 8 An+1 = 0. 8 An + 100 c = 100 20% decrease in worker ants 100 ants captured weekly

2 U 1 Finding Recurrence Relation 6 26…. U 2 U 3 Is of form Un+1 = m. Un + c so U 2 = m. U 1 + c 6 = m(2) + c U 3 = m. U 2 + c 26= m(6) + c 2 m + c = 6 6 m + c = 26 4 m = 20 Un+1 = 5 Un – 4 m=5 c=-4

Sandra has borrowed money from Lenny the loan shark. The original loan was £ 3000. L 0 He is paying back £ 200 each week. She is shocked to find that 2 weeks later she now owes £ 3, 210!!!!!! L 2 What are Lenny’s rates of interest? Ln+1 = m. Ln + c - 200

Ln+1 = m. Ln – 200 Equation with L 0? L 1 = m. L 0 – 200 L 1 = m(3000) – 200 Equation with L 2? L 2 = m. L 1 – 200 3210 = m. L 1 – 200 L 1 = 3000 m – 200 3210 = m. L 1 – 200 L 0 = 3000 L 2= 3210

L 1 = 3000 m – 200 3210 = m. L 1 – 200 3210 = m(3000 m – 200) – 200 3210 = 3000 m 2 – 200 m – 200 0 = 3000 m 2 – 200 m – 3410 Using Big Nasty Formula m = 1. 1 Ln+1 = 1. 1 Ln – 200 Interest Rate 10% per week.