ESSENTIAL CALCULUS CH 12 Multiple integrals In this





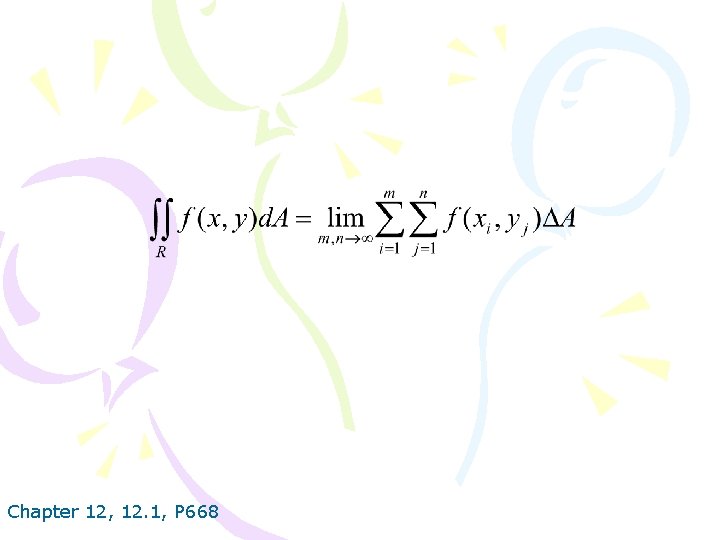
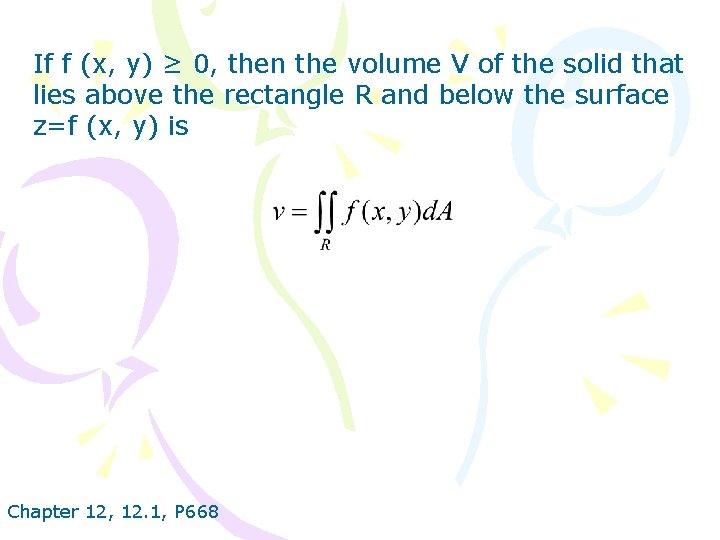
![MIDPOINT RULE FOR DOUBLE INTEGRALS where xi is the midpoint of [xi-1, xi] and MIDPOINT RULE FOR DOUBLE INTEGRALS where xi is the midpoint of [xi-1, xi] and](https://slidetodoc.com/presentation_image_h/2576218f11d5a804c893f00259634037/image-9.jpg)


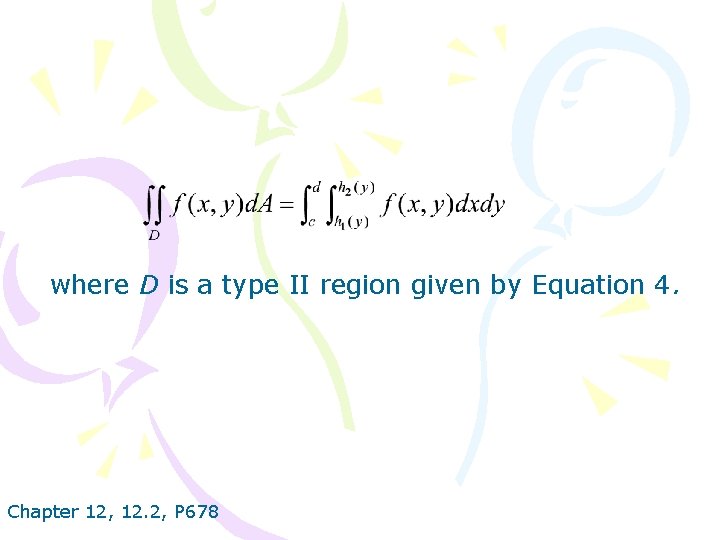

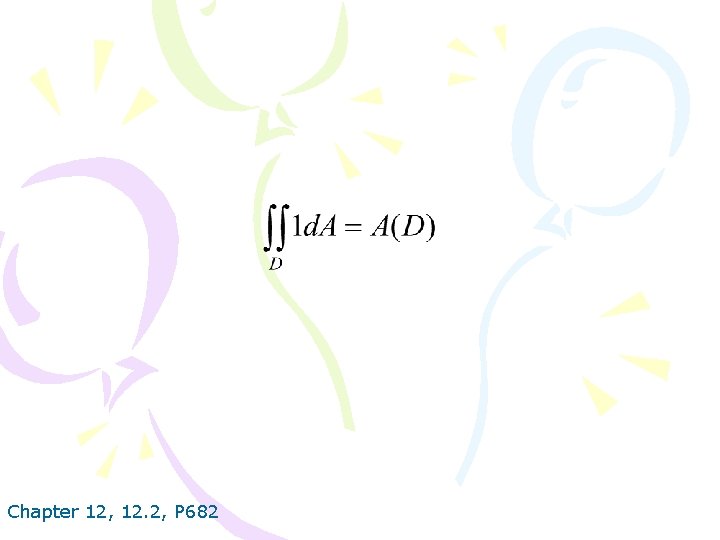
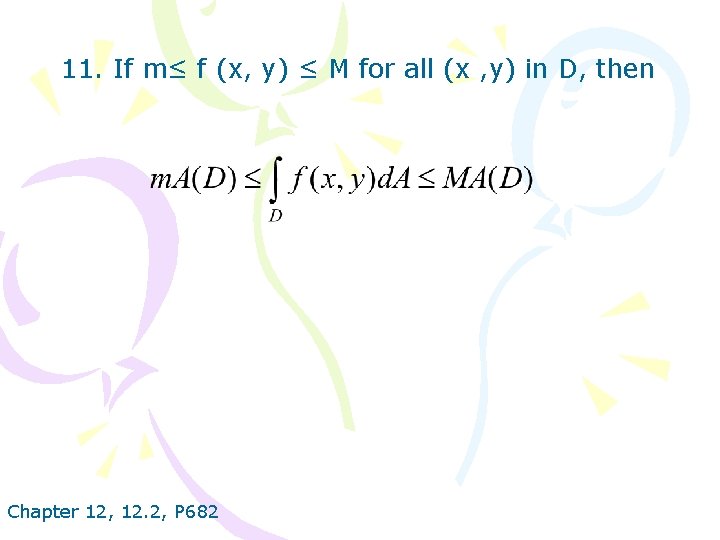







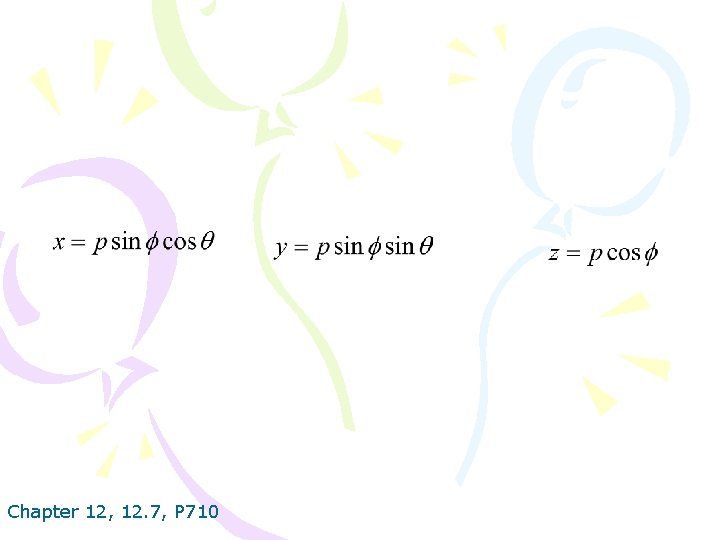


- Slides: 59

ESSENTIAL CALCULUS CH 12 Multiple integrals

In this Chapter: n 12. 1 Double Integrals over Rectangles n 12. 2 Double Integrals over General Regions n 12. 3 Double Integrals in Polar Coordinates n 12. 4 Applications of Double Integrals n 12. 5 Triple Integrals n 12. 6 Triple Integrals in Cylindrical Coordinates n 12. 7 Triple Integrals in Spherical Coordinates n 12. 8 Change of Variables in Multiple Integrals Review

Chapter 12, 12. 1, P 665 (See Figure 2. ) Our goal is to find the volume of S.

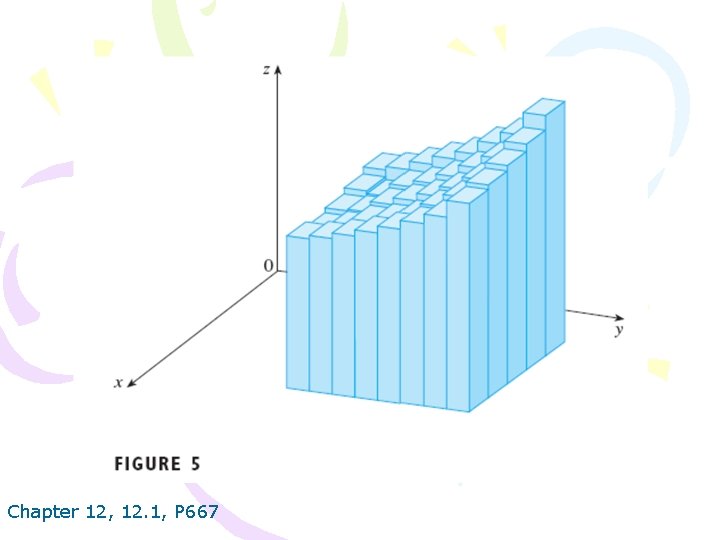
Chapter 12, 12. 1, P 667

Chapter 12, 12. 1, P 667

5. DEFINITION The double integral of f over the rectangle R is if this limit exists. Chapter 12, 12. 1, P 667
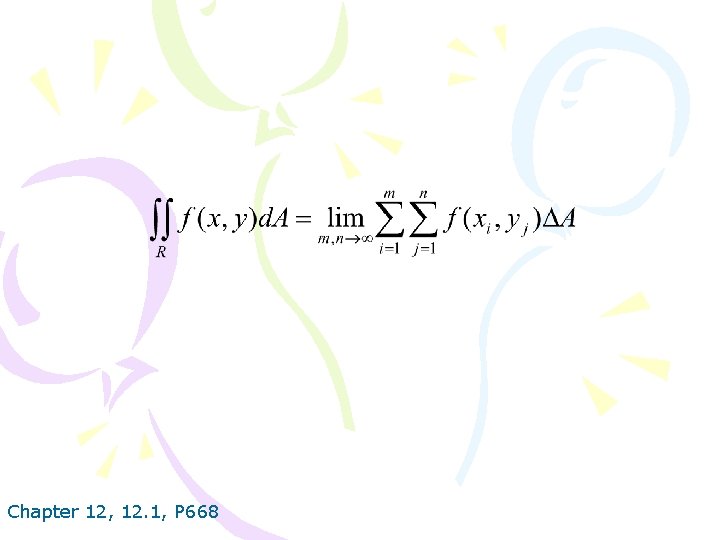
Chapter 12, 12. 1, P 668
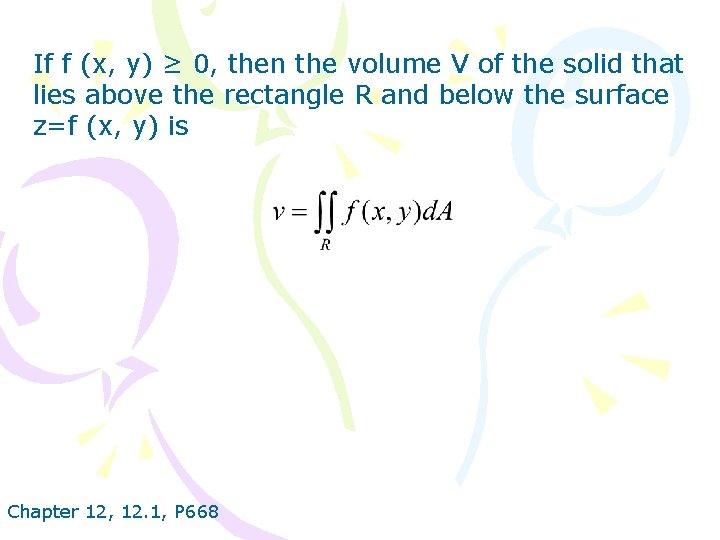
If f (x, y) ≥ 0, then the volume V of the solid that lies above the rectangle R and below the surface z=f (x, y) is Chapter 12, 12. 1, P 668
![MIDPOINT RULE FOR DOUBLE INTEGRALS where xi is the midpoint of xi1 xi and MIDPOINT RULE FOR DOUBLE INTEGRALS where xi is the midpoint of [xi-1, xi] and](https://slidetodoc.com/presentation_image_h/2576218f11d5a804c893f00259634037/image-9.jpg)
MIDPOINT RULE FOR DOUBLE INTEGRALS where xi is the midpoint of [xi-1, xi] and yj is the midpoint of [yj-1, yj ]. Chapter 12, 12. 1, P 669

10. FUBINI’S THEOREM If f is continuous on the rectangle R={(x, y} │a ≤ x≤ b, c ≤ y ≤d} , then More generally, this is true if we assume that f is bounded on R, is discontinuous only on a finite number of smooth curves, and the iterated integrals exist. Chapter 12, 12. 1, P 672

where R Chapter 12, 12. 1, P 673

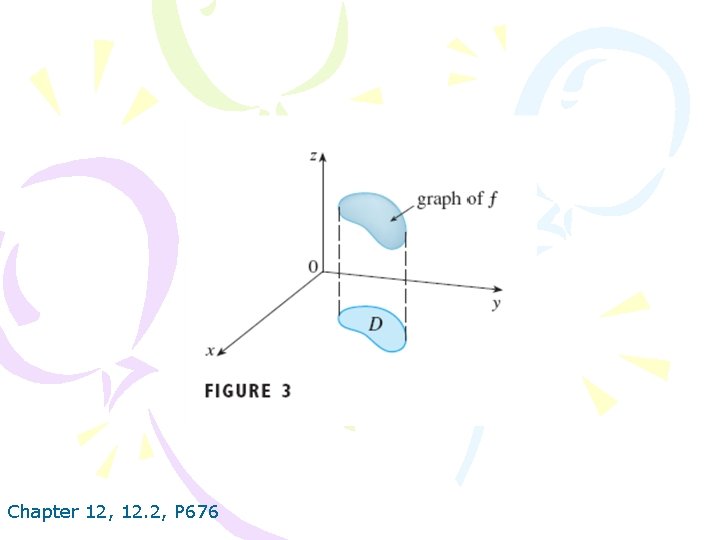
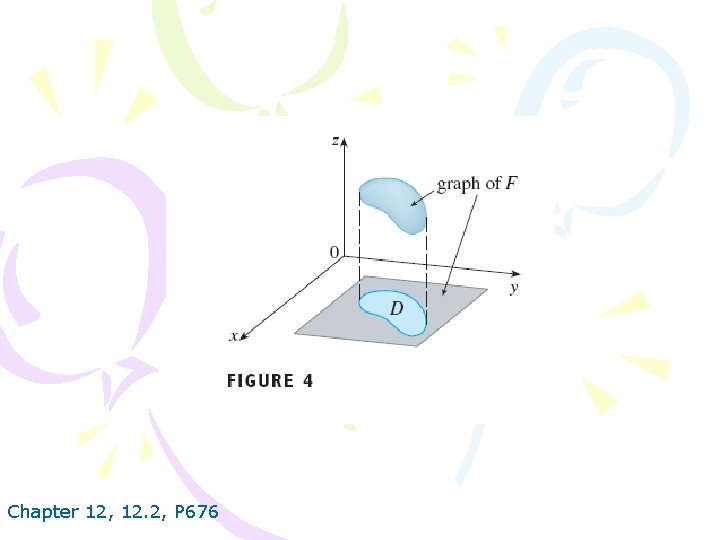
Chapter 12, 12. 2, P 676

Chapter 12, 12. 2, P 676

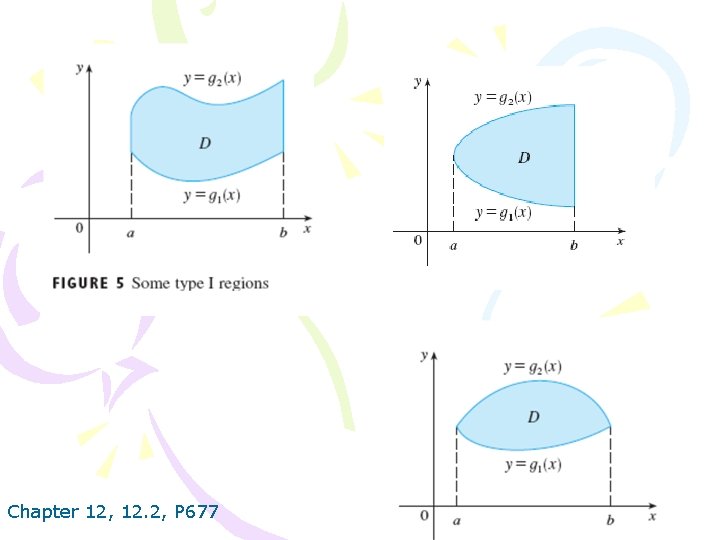
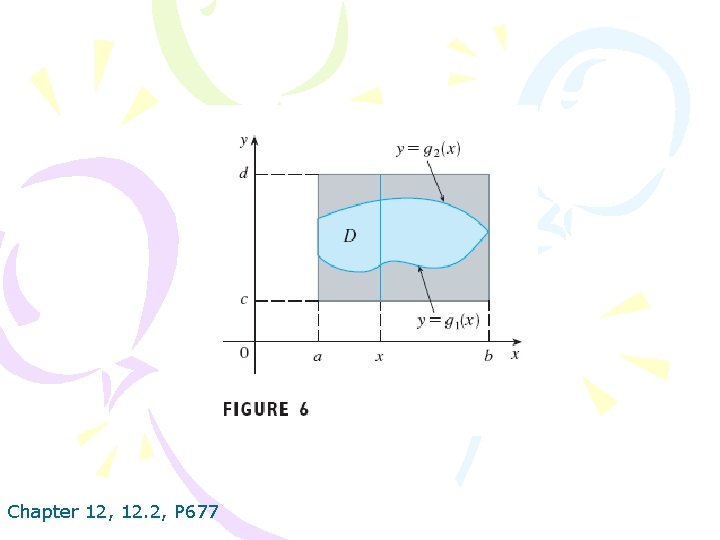
Chapter 12, 12. 2, P 677

Chapter 12, 12. 2, P 677

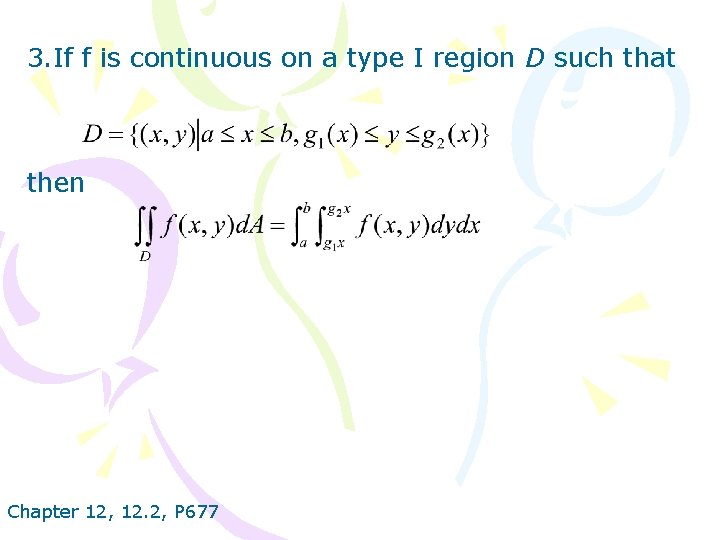
3. If f is continuous on a type I region D such that then Chapter 12, 12. 2, P 677

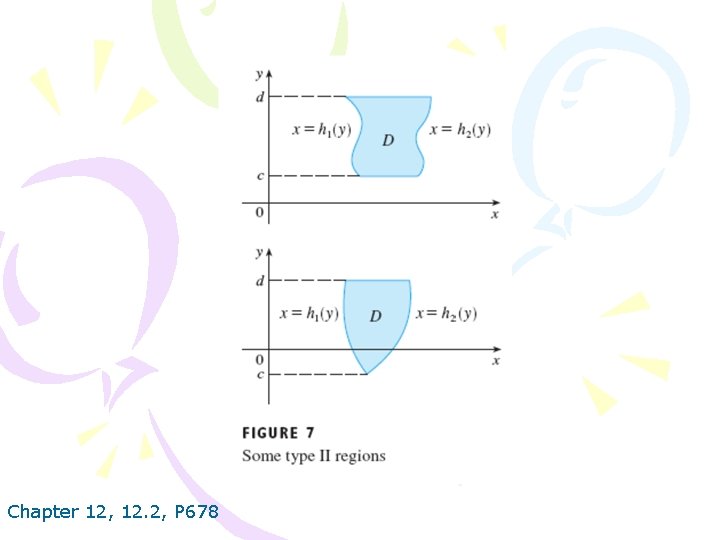
Chapter 12, 12. 2, P 678
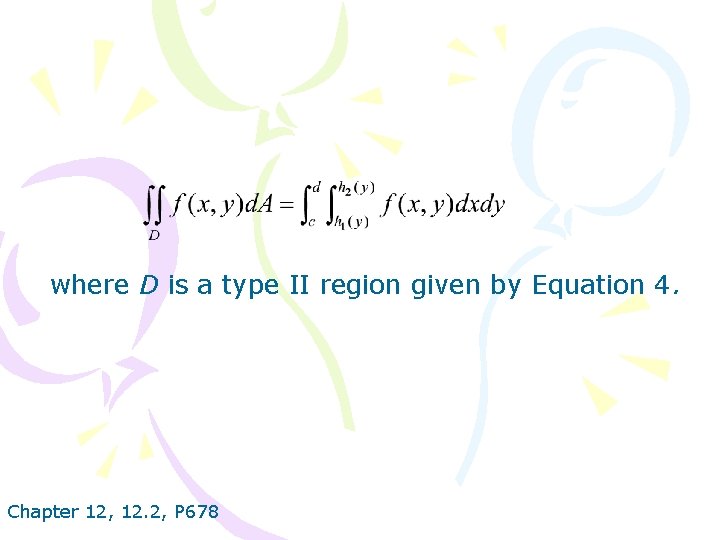
where D is a type II region given by Equation 4. Chapter 12, 12. 2, P 678

Chapter 12, 12. 2, P 681

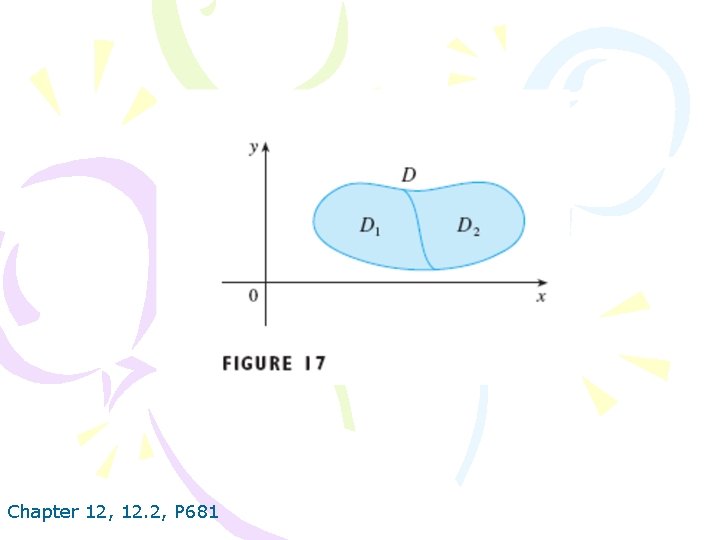
6. 7. If f (x, y) ≥ g (x, y) for all (x, y) in D, then 8. If D=D 1 U D 2, where D 1 and D 2 don’t overlap except perhaps on their boundaries (see Figure 17), then Chapter 12, 12. 2, P 681
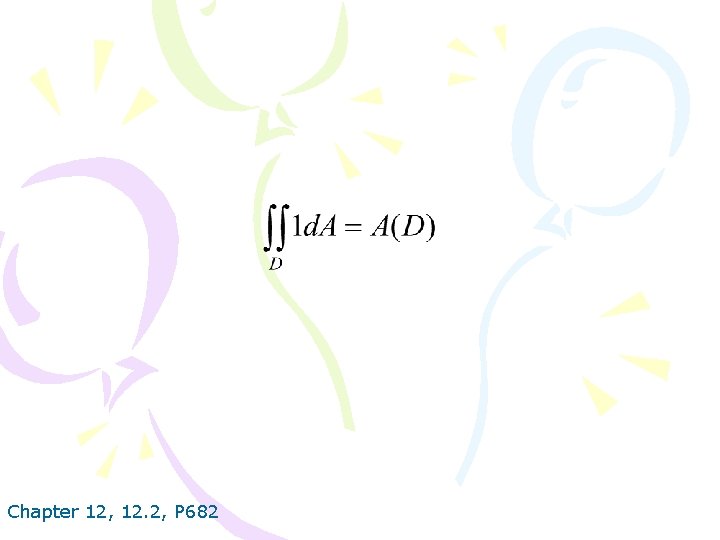
Chapter 12, 12. 2, P 682
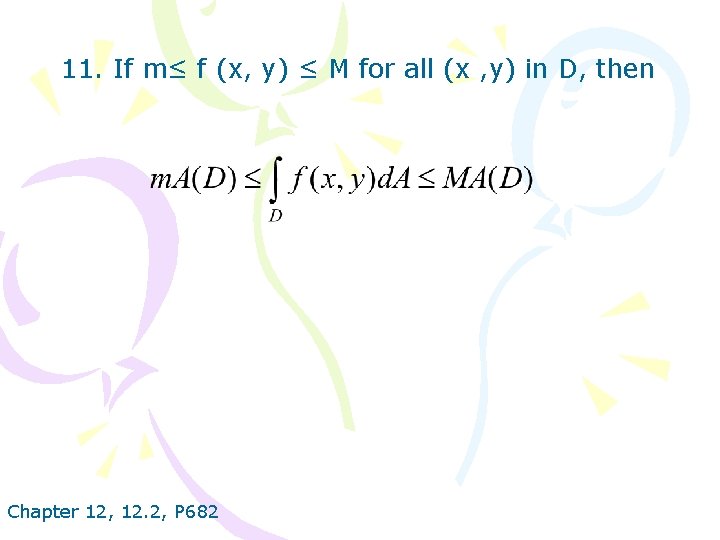
11. If m≤ f (x, y) ≤ M for all (x , y) in D, then Chapter 12, 12. 2, P 682

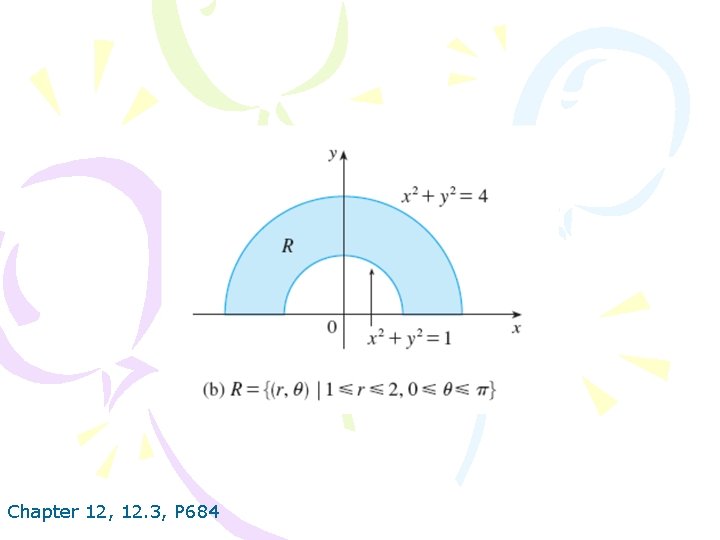
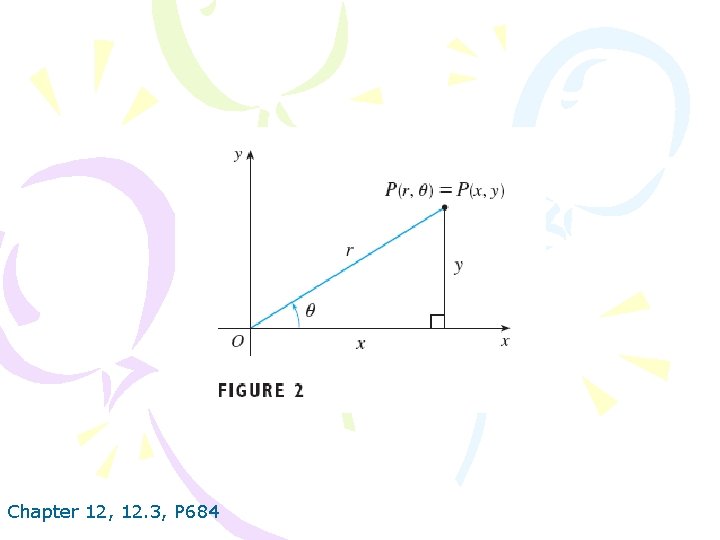
Chapter 12, 12. 3, P 684

Chapter 12, 12. 3, P 684

Chapter 12, 12. 3, P 684

r 2=x 2+y 2 Chapter 12, 12. 3, P 684 x=r cosθ y=r sinθ

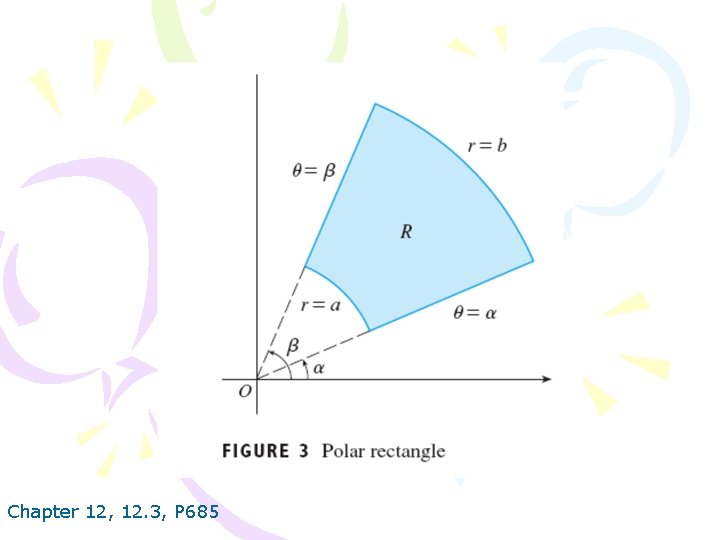
Chapter 12, 12. 3, P 685

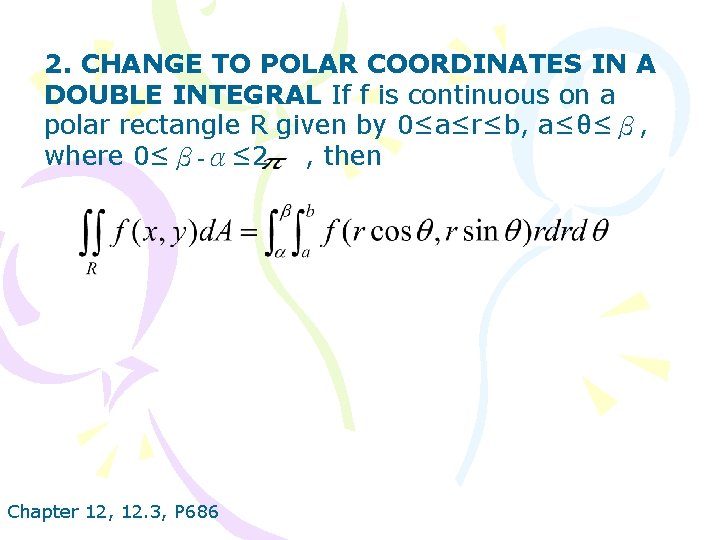
2. CHANGE TO POLAR COORDINATES IN A DOUBLE INTEGRAL If f is continuous on a polar rectangle R given by 0≤a≤r≤b, a≤θ≤β, where 0≤β-α≤ 2 , then Chapter 12, 12. 3, P 686

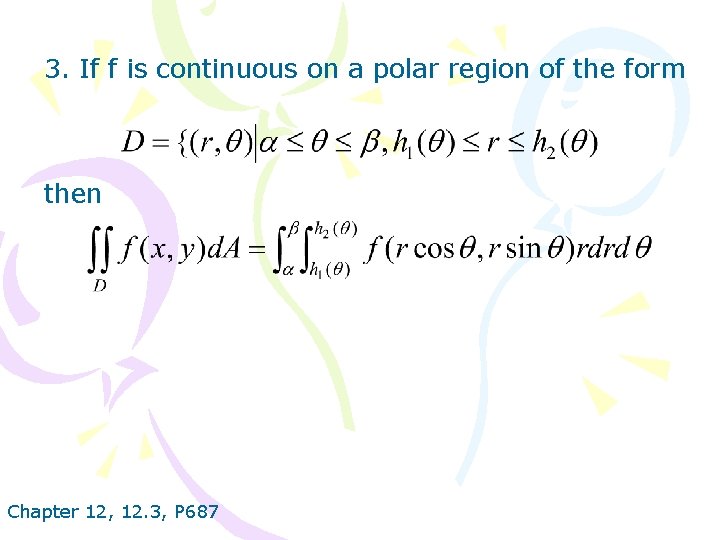
3. If f is continuous on a polar region of the form then Chapter 12, 12. 3, P 687

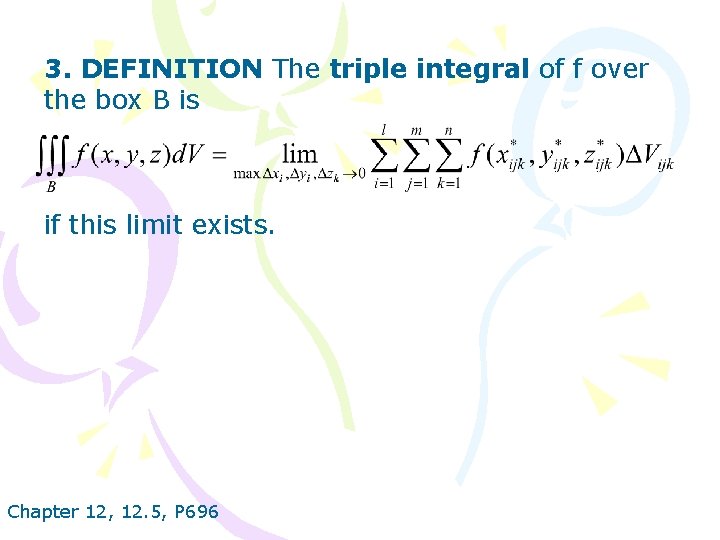
3. DEFINITION The triple integral of f over the box B is if this limit exists. Chapter 12, 12. 5, P 696

Chapter 12, 12. 5, P 696

4. FUBINI’S THEOREM FOR TRIPLE INTEGRALS If f is continuous on the rectangular box B=[a, b]╳ [c, d ]╳[r, s], then Chapter 12, 12. 5, P 696

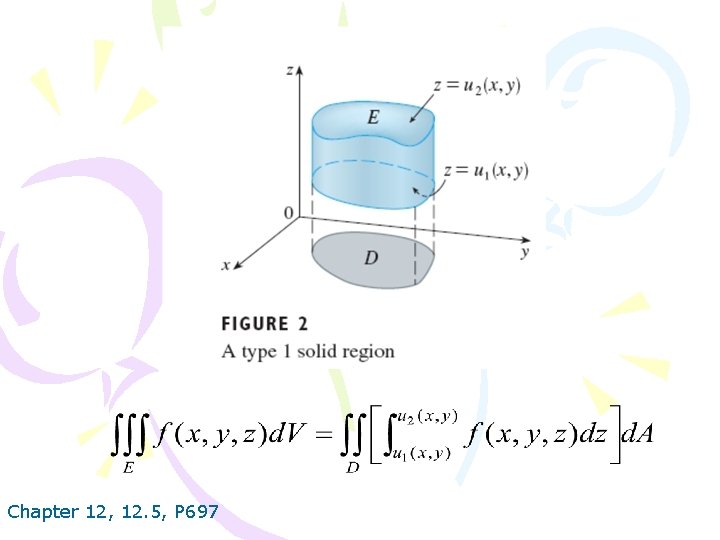
Chapter 12, 12. 5, P 697

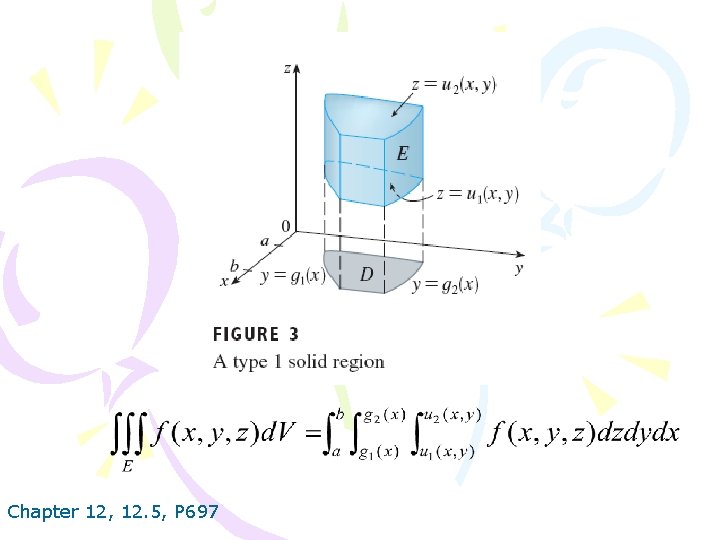
Chapter 12, 12. 5, P 697

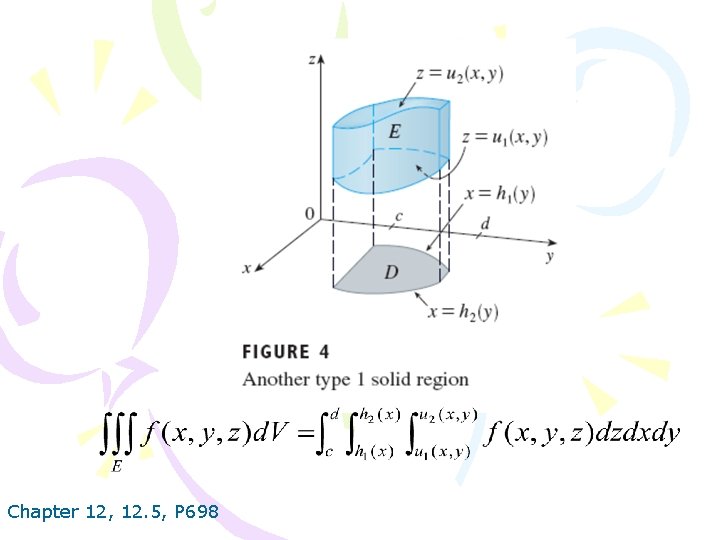
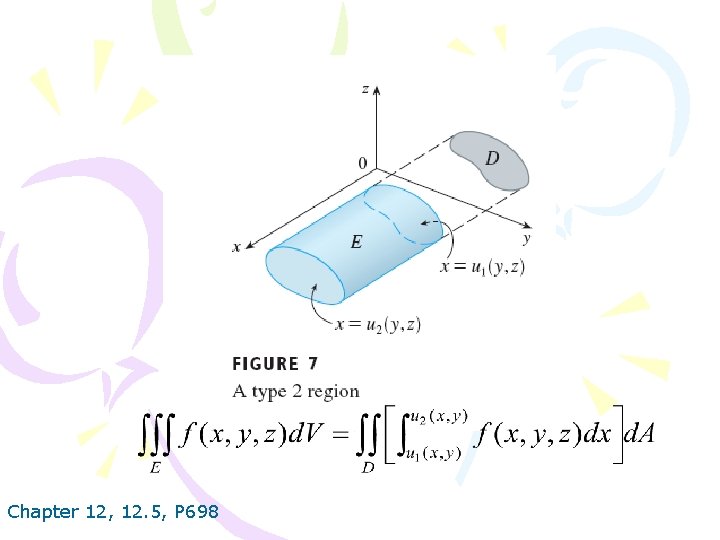
Chapter 12, 12. 5, P 698

Chapter 12, 12. 5, P 698

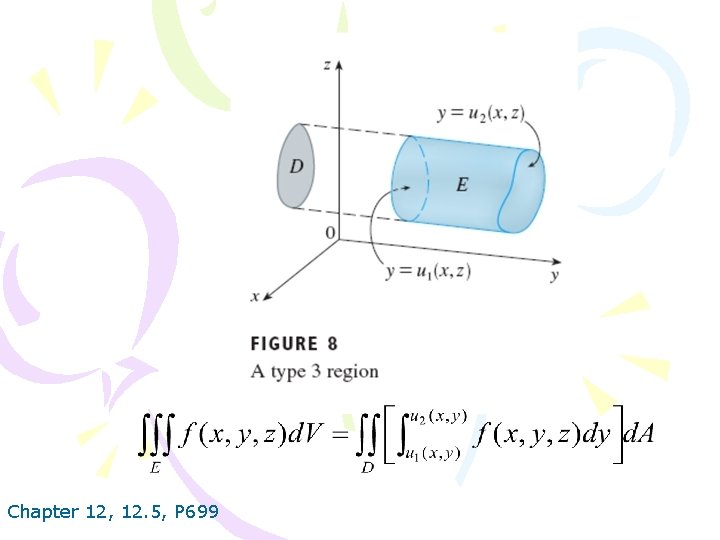
Chapter 12, 12. 5, P 699

Chapter 12, 12. 5, P 700

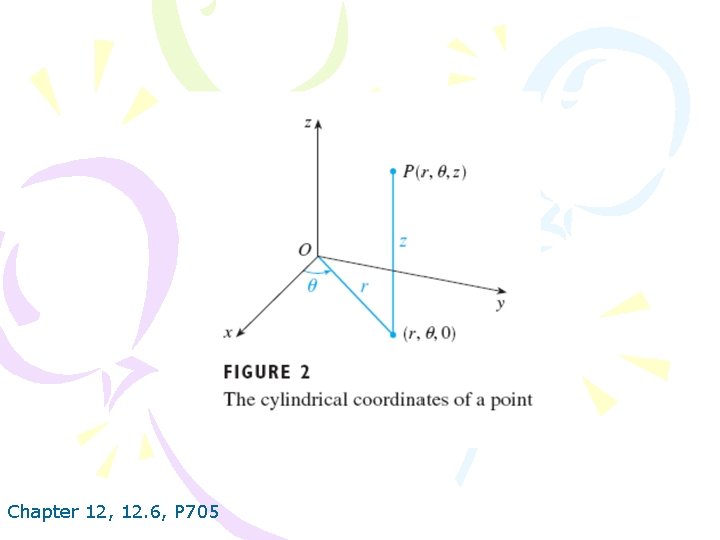
Chapter 12, 12. 6, P 705

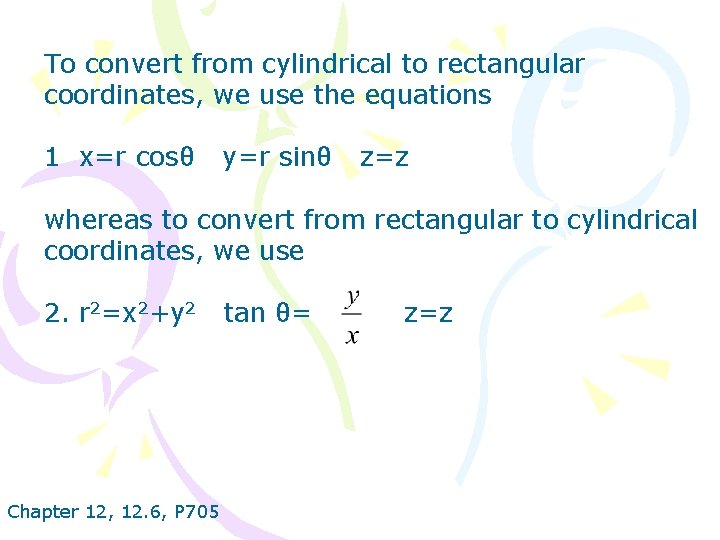
To convert from cylindrical to rectangular coordinates, we use the equations 1 x=r cosθ y=r sinθ z=z whereas to convert from rectangular to cylindrical coordinates, we use 2. r 2=x 2+y 2 Chapter 12, 12. 6, P 705 tan θ= z=z

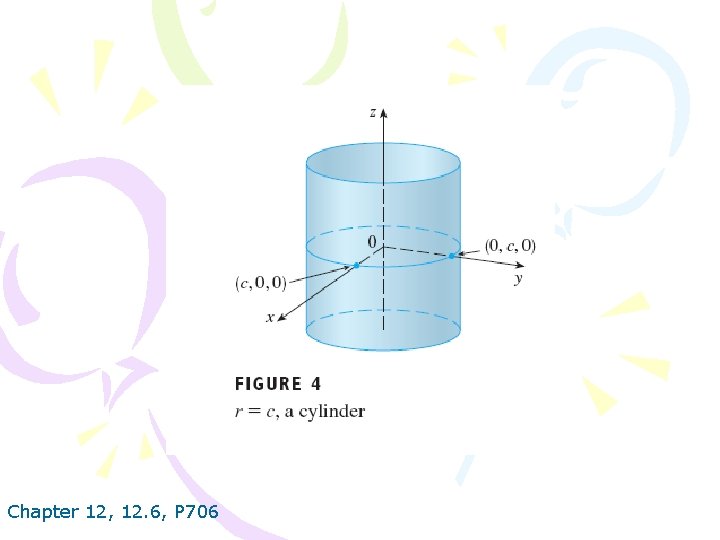
Chapter 12, 12. 6, P 706

Chapter 12, 12. 6, P 706

Chapter 12, 12. 6, P 706

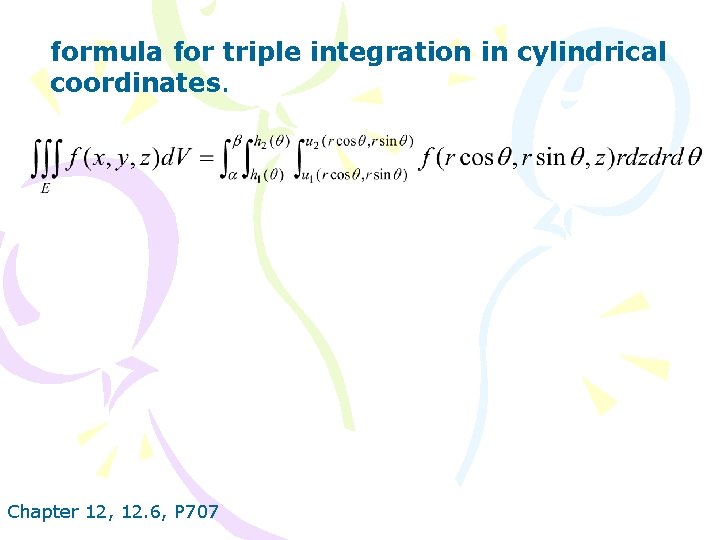
formula for triple integration in cylindrical coordinates. Chapter 12, 12. 6, P 707

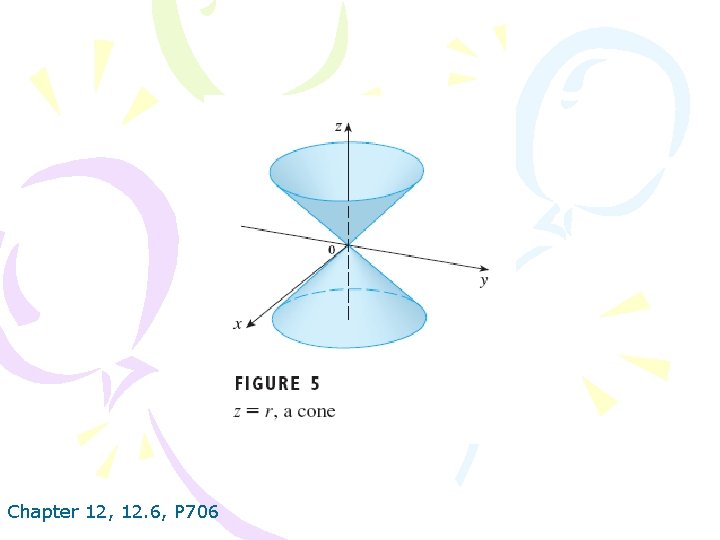
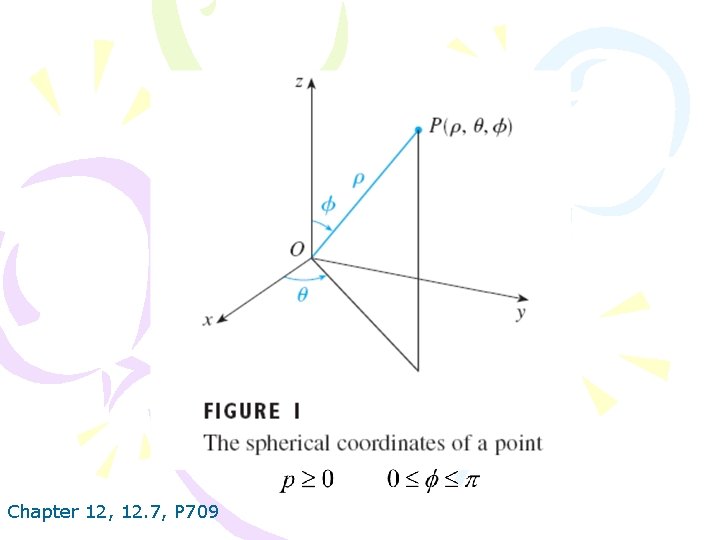
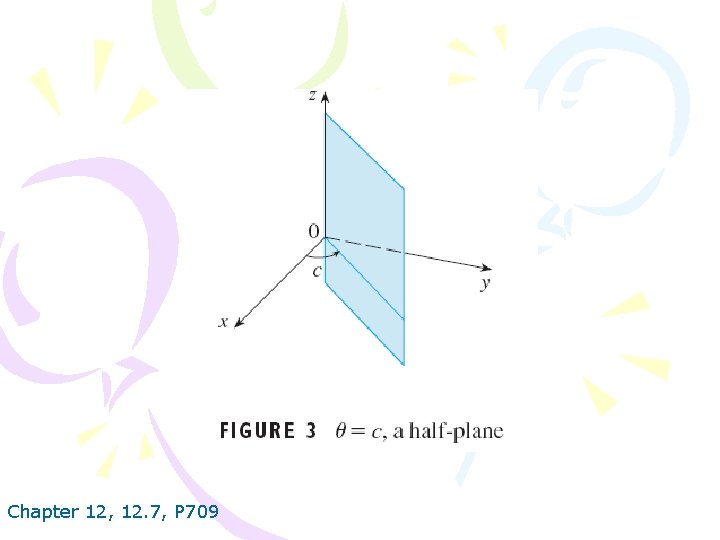
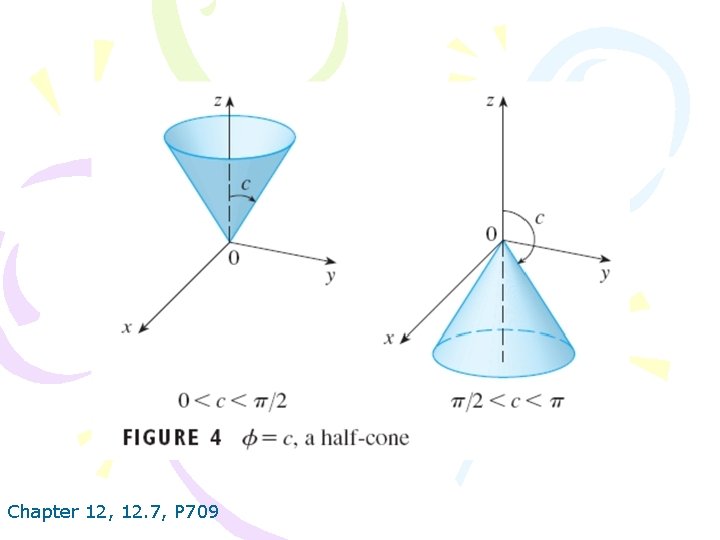
Chapter 12, 12. 7, P 709

Chapter 12, 12. 7, P 709

Chapter 12, 12. 7, P 709

Chapter 12, 12. 7, P 709

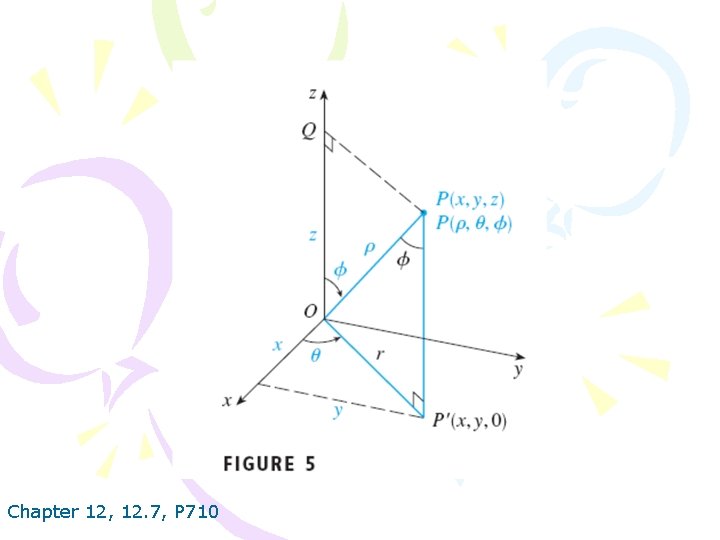
Chapter 12, 12. 7, P 710
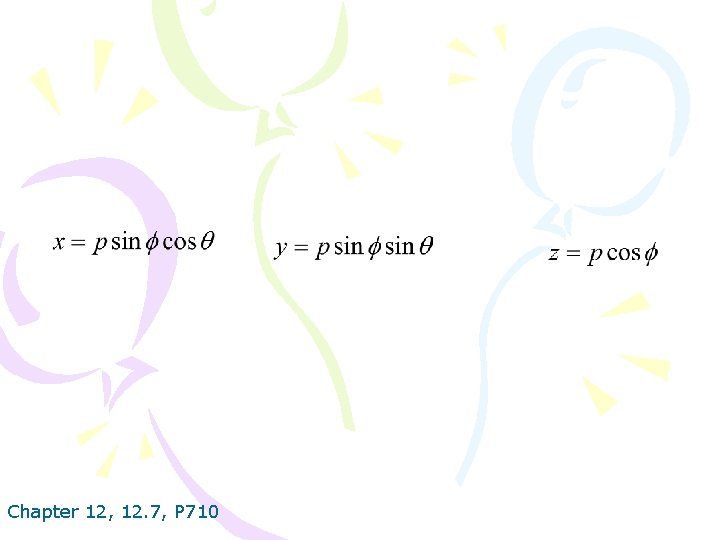
Chapter 12, 12. 7, P 710

Chapter 12, 12. 7, P 710

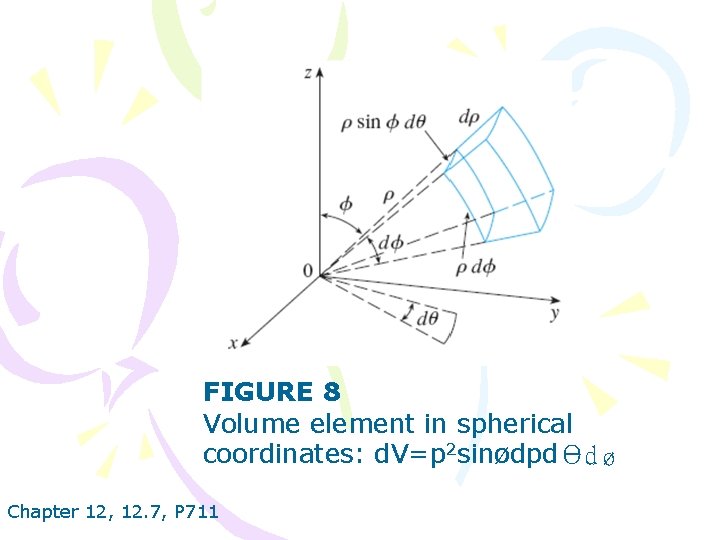
FIGURE 8 Volume element in spherical coordinates: d. V=p 2 sinødpdΘd ø Chapter 12, 12. 7, P 711

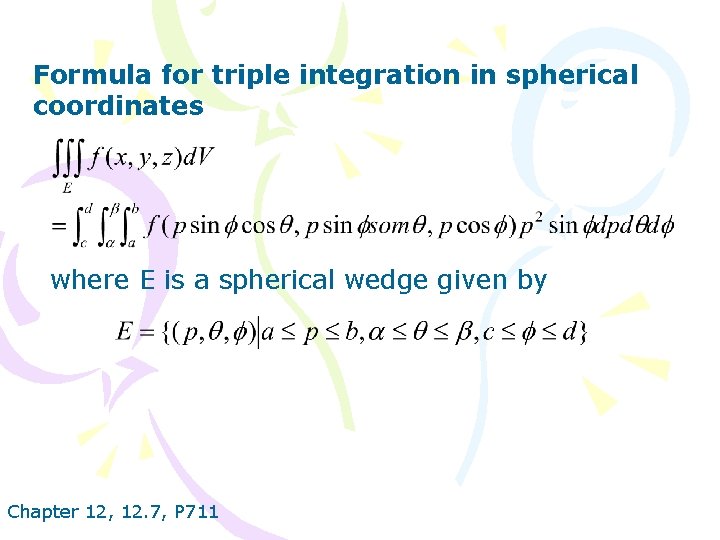
Formula for triple integration in spherical coordinates where E is a spherical wedge given by Chapter 12, 12. 7, P 711

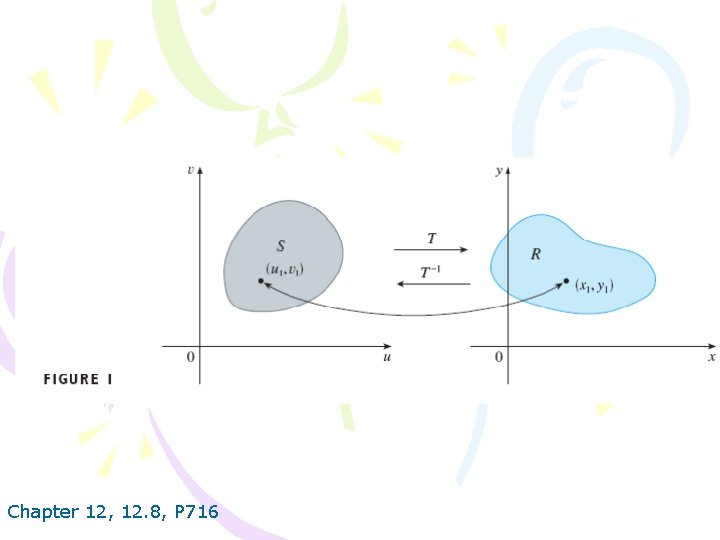
Chapter 12, 12. 8, P 716

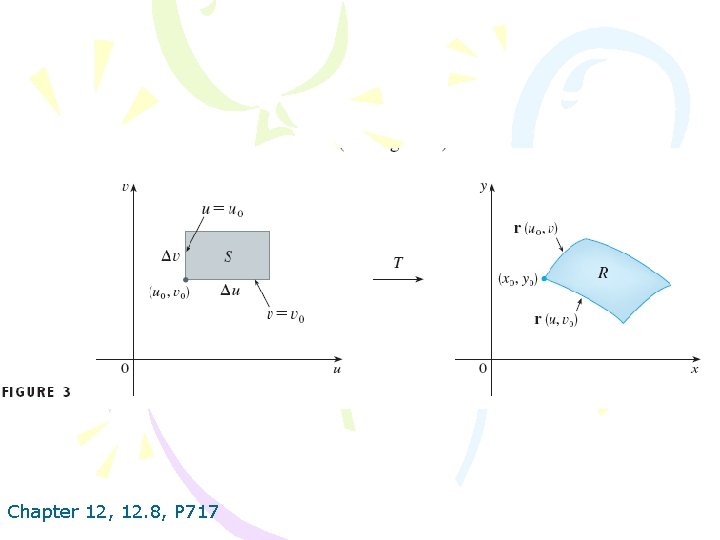
Chapter 12, 12. 8, P 717

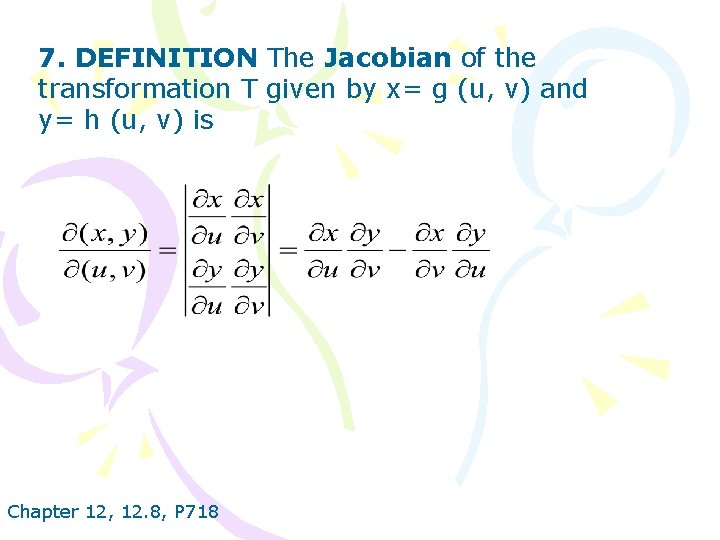
7. DEFINITION The Jacobian of the transformation T given by x= g (u, v) and y= h (u, v) is Chapter 12, 12. 8, P 718

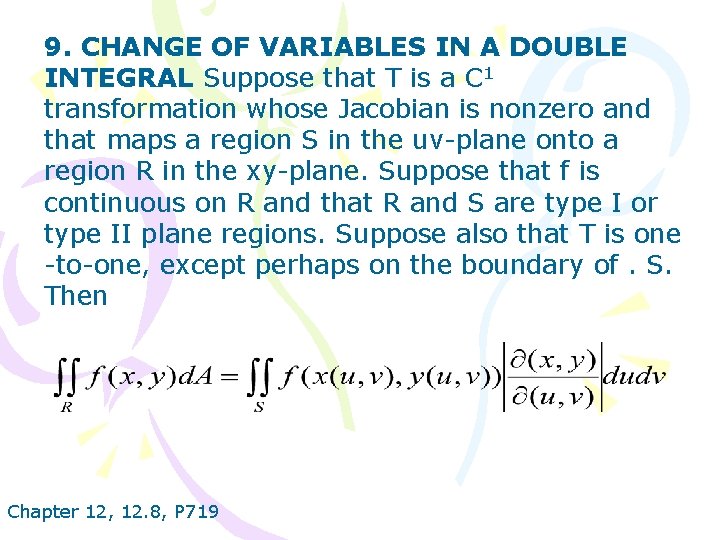
9. CHANGE OF VARIABLES IN A DOUBLE INTEGRAL Suppose that T is a C 1 transformation whose Jacobian is nonzero and that maps a region S in the uv-plane onto a region R in the xy-plane. Suppose that f is continuous on R and that R and S are type I or type II plane regions. Suppose also that T is one -to-one, except perhaps on the boundary of. S. Then Chapter 12, 12. 8, P 719

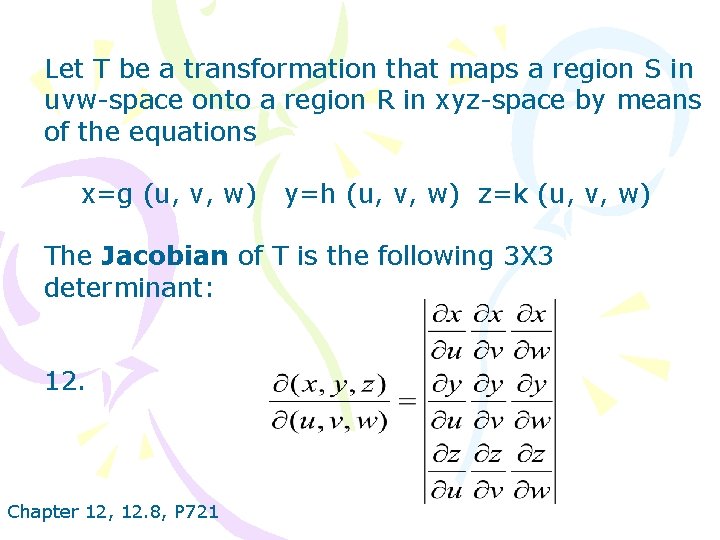
Let T be a transformation that maps a region S in uvw-space onto a region R in xyz-space by means of the equations x=g (u, v, w) y=h (u, v, w) z=k (u, v, w) The Jacobian of T is the following 3 X 3 determinant: 12. Chapter 12, 12. 8, P 721

13. Chapter 12, 12. 8, P 722
 Calculus chapter 5
Calculus chapter 5 Change of variables multiple integrals
Change of variables multiple integrals Plamatic acid
Plamatic acid Arctan integral
Arctan integral The fundamental theorem of calculus
The fundamental theorem of calculus Radiation integrals and auxiliary potential functions
Radiation integrals and auxiliary potential functions Integrals involving powers of secant and tangent
Integrals involving powers of secant and tangent Fourier series of even and odd functions
Fourier series of even and odd functions Integrals
Integrals Hard trig integrals
Hard trig integrals General power rule
General power rule Curl formula in spherical coordinates
Curl formula in spherical coordinates Integration by substitution
Integration by substitution Integral rule
Integral rule Additive interval property
Additive interval property Sigma notation to integral
Sigma notation to integral Average rate of change integrals
Average rate of change integrals Area enclosed by curve and x axis
Area enclosed by curve and x axis Fubini's theorem
Fubini's theorem Properties of indefinite integrals
Properties of indefinite integrals Double integral over rectangle
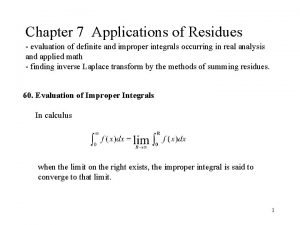
Double integral over rectangle Application of residue theorem to evaluate real integrals
Application of residue theorem to evaluate real integrals Double integral volume
Double integral volume X=tcost y=tsint
X=tcost y=tsint Integral permukaan adalah
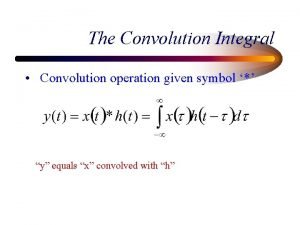
Integral permukaan adalah Integral convolution
Integral convolution Polar integrals
Polar integrals Maurits haverkort
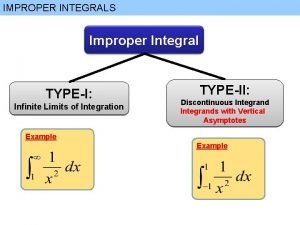
Maurits haverkort Improper integrals
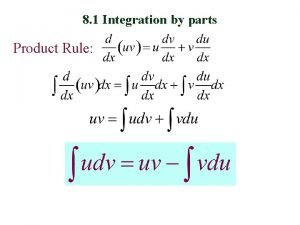
Improper integrals Product rule in integration
Product rule in integration Signals and systems
Signals and systems Triple integrals
Triple integrals Kompetensi dasar integral
Kompetensi dasar integral Substitution rule
Substitution rule Improper integral
Improper integral Integral garis
Integral garis Trig derivatives
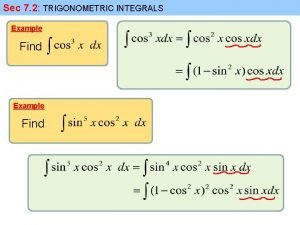
Trig derivatives Trigonemtric integrals
Trigonemtric integrals Is integral the area under a curve
Is integral the area under a curve Application of residue theorem to evaluate real integrals
Application of residue theorem to evaluate real integrals Circuit training properties of definite integrals
Circuit training properties of definite integrals Easy integrals
Easy integrals Exploration 1-3a introduction to definite integrals
Exploration 1-3a introduction to definite integrals Multiple instruction multiple data
Multiple instruction multiple data Multiple baseline across settings
Multiple baseline across settings Honors precalculus chapter 1 test
Honors precalculus chapter 1 test Reciprocal rule calculus
Reciprocal rule calculus Calculus 1 college
Calculus 1 college Greg kelly calculus
Greg kelly calculus Hedonic calculus scenarios
Hedonic calculus scenarios Calculus latin
Calculus latin Calculus critical points
Calculus critical points Section 3 introduction to functions
Section 3 introduction to functions Calculus 9
Calculus 9 Slope fields ap calculus
Slope fields ap calculus Sequence calculus
Sequence calculus Matriks hessian 3 variabel
Matriks hessian 3 variabel Relational algebra and calculus
Relational algebra and calculus Calculus book
Calculus book Pre calculus unit 1 review
Pre calculus unit 1 review