ECE 576 POWER SYSTEM DYNAMICS AND STABILITY Lecture

ECE 576 POWER SYSTEM DYNAMICS AND STABILITY Lecture 35 Direct Methods for Stability Analysis Professor M. A. Pai Department of Electrical and Computer Engineering © 2000 University of Illinois Board of Trustees, All Rights Reserved

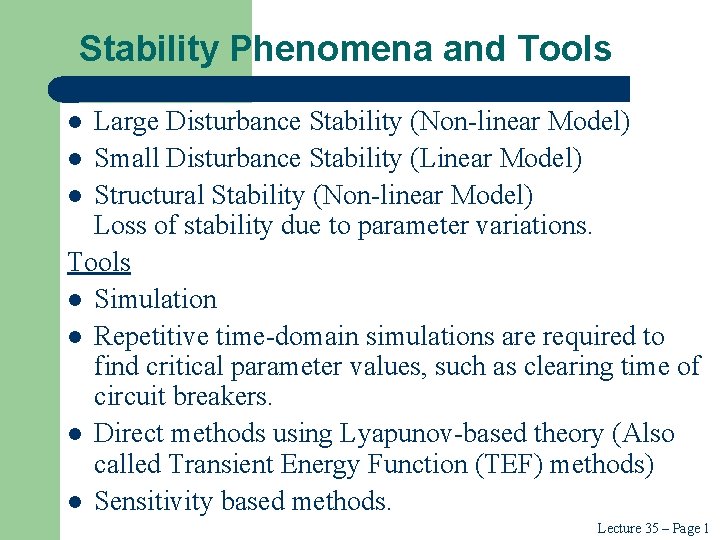
Stability Phenomena and Tools Large Disturbance Stability (Non-linear Model) l Small Disturbance Stability (Linear Model) l Structural Stability (Non-linear Model) Loss of stability due to parameter variations. Tools l Simulation l Repetitive time-domain simulations are required to find critical parameter values, such as clearing time of circuit breakers. l Direct methods using Lyapunov-based theory (Also called Transient Energy Function (TEF) methods) l Sensitivity based methods. l Lecture 35 – Page 1

TEF Techniques l No repeated simulations are involved. l Limited somewhat by modeling complexity. l Energy of the system used as Lyapunov function. l Computing energy at the “controlling” unstable equilibrium point (CUEP) (critical energy). l CUEP defines the mode of instability for a particular fault. l Computing critical energy is not easy. Lecture 35 – Page 2

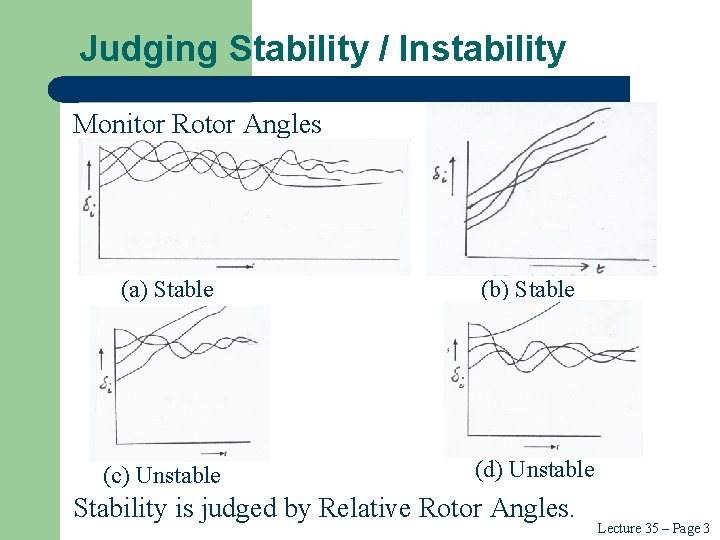
Judging Stability / Instability Monitor Rotor Angles (a) Stable (b) Stable (c) Unstable (d) Unstable Stability is judged by Relative Rotor Angles. Lecture 35 – Page 3

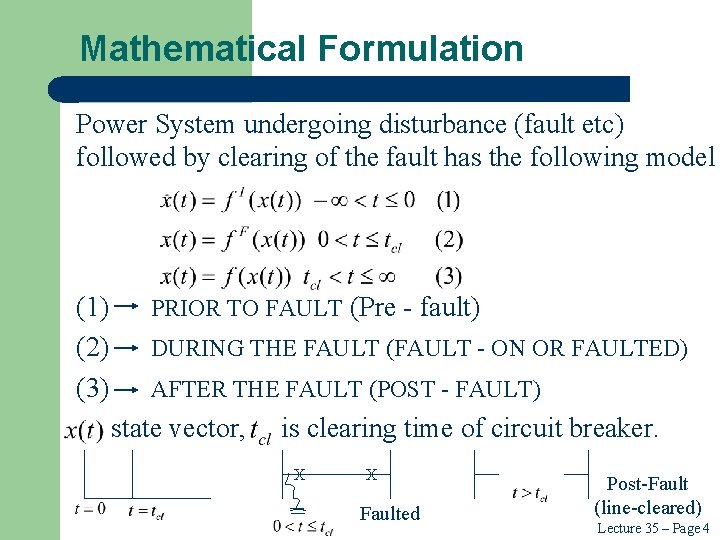
Mathematical Formulation Power System undergoing disturbance (fault etc) followed by clearing of the fault has the following model (1) (2) (3) PRIOR TO FAULT (Pre - fault) DURING THE FAULT (FAULT - ON OR FAULTED) AFTER THE FAULT (POST - FAULT) state vector, is clearing time of circuit breaker. X X Faulted Post-Fault (line-cleared) Lecture 35 – Page 4

Critical Clearing Time l l l In the pre fault state the system would have reached a steady state. Hence is known. The pre fault dynamics are of no interest. . Two systems FAULTED (1) AND POST-FAULT (2). Initial Conditions for (2) are provided by the solution of (1) evaluated at Lecture 35 – Page 5

Critical Clearing Time (contd) l l l Assume post - fault system has a stable equilibrium point All possible values of for differing clearing times, provide initial conditions for the post - fault system. Will the trajectory of the post fault system starting at. converge to Largest value of (called ) for which this is true is called critical clearing time. is different for different faults. Let us view this pictorially: Lecture 35 – Page 6

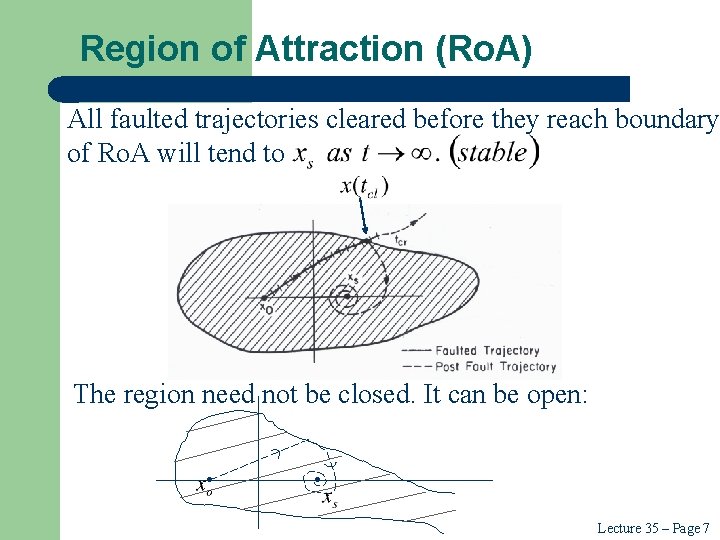
Region of Attraction (Ro. A) All faulted trajectories cleared before they reach boundary of Ro. A will tend to The region need not be closed. It can be open: . . Lecture 35 – Page 7

Methods to Compute Ro. A l l Topic of intense research in P. S. literature since early 60’s. stable equilibrium point (s. e. p. ) of post - fault system l is generally close to pre-fault s. e. p Surrounding this s. e. p there a number of unstable equilibrium points (u. e. p). l Boundary of Ro. A is characterized via these u. e. p’s Lecture 35 – Page 8

Characterization of Ro. A l l l l Define an energy function of the post-fault system. Compute over all i is one possible choice for Ro. A is defined by Extremely conservative result. Alternative method: Depending on the fault, identify THE towards which faulted trajectory is headed. Call it (controlling u. e. p) Then is a good estimate of Ro. A. Lecture 35 – Page 9

Lyapunov’s Method l If there exists a scalar function such that and around equilibrium point ‘ 0’ and then equilibrium ‘ 0’ is asymptotically stable. . l Thus l enters directly in the computation of Lecture 35 – Page 10

Lyapunov’s Method in P. S. l condition can be relaxed to provided along any other solution except x=0. l This is an important aspect of the Lyapunov theory. l Early application of Lyapunov’s Method in Power Systems – – Gless Magnusson – Aylett – El - Abiad - Nagappan l Many research papers after 1964. l Transient Energy Function (TEF). 1940 – 1960 Lecture 35 – Page 11

Multimachine internal node model Lecture 35 – Page 12

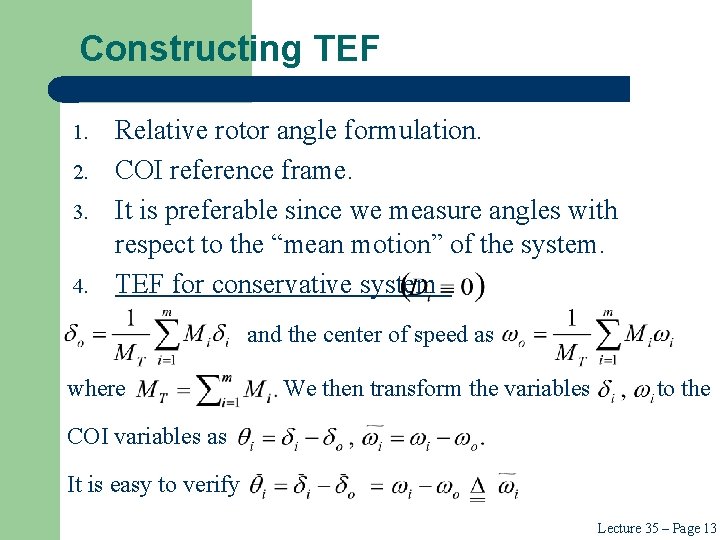
Constructing TEF 1. 2. 3. 4. Relative rotor angle formulation. COI reference frame. It is preferable since we measure angles with respect to the “mean motion” of the system. TEF for conservative system and the center of speed as where We then transform the variables to the COI variables as It is easy to verify Lecture 35 – Page 13

TEF (contd) The swing equations with l l l become (omitting the algebra): If one of the machines is an infinite bus, say, m whose inertia constant is very large, then and also and. The COI variables become In the literature is simply taken as zero. Equation is modified accordingly, and there will be only (m-1) equations after omitting the equation for machine m. Lecture 35 – Page 14

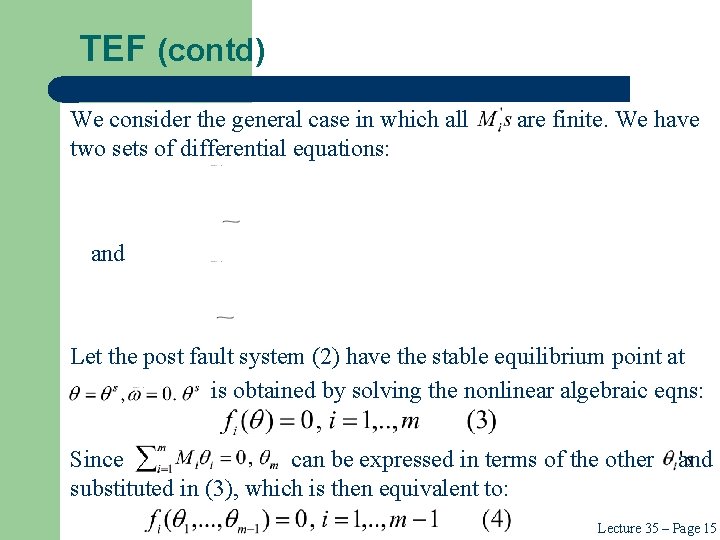
TEF (contd) We consider the general case in which all two sets of differential equations: are finite. We have and Let the post fault system (2) have the stable equilibrium point at is obtained by solving the nonlinear algebraic eqns: Since can be expressed in terms of the other substituted in (3), which is then equivalent to: and Lecture 35 – Page 15

TEF (contd) Steps for computing the critical clearing time are: 1. 2. 3. l Construct an energy or Lyapunov function for the post-fault system. Find the critical value of for a given fault denoted by Integrate the faulted equations, until This instant of time is called the critical clearing time. Most of the methods differ as to how to implement steps 2 and 3. Lecture 35 – Page 16

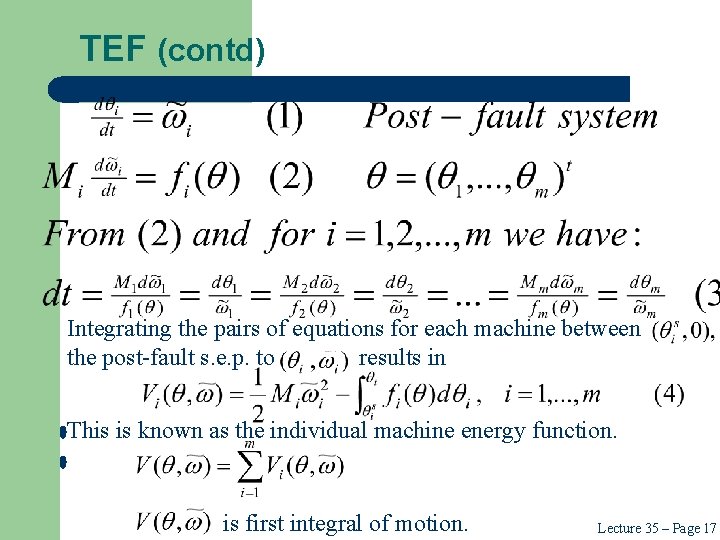
TEF (contd) Integrating the pairs of equations for each machine between the post-fault s. e. p. to results in This is known as the individual machine energy function. is first integral of motion. Lecture 35 – Page 17

TEF (contd) since Lecture 35 – Page 18

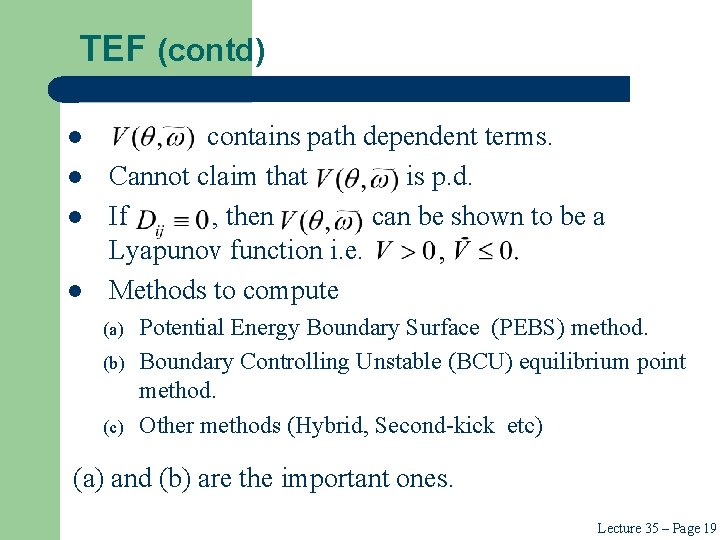
TEF (contd) l l contains path dependent terms. Cannot claim that is p. d. If , then can be shown to be a Lyapunov function i. e. Methods to compute (a) (b) (c) Potential Energy Boundary Surface (PEBS) method. Boundary Controlling Unstable (BCU) equilibrium point method. Other methods (Hybrid, Second-kick etc) (a) and (b) are the important ones. Lecture 35 – Page 19

Equal Area Criterion and TEF Single-machine infinite-bus system l l l A three-phase fault occurs at the middle of one of the lines at t=0, and is subsequently cleared at by opening the circuit breakers at both ends of the faulted line. The pre-fault, faulted, and post-fault configurations and their reduction to a two-machine equivalent are constructed. The electric power during pre-fault, faulted, and post-fault states are respectively. ~ > > > Single-machine infinite-bus system Lecture 35 – Page 20

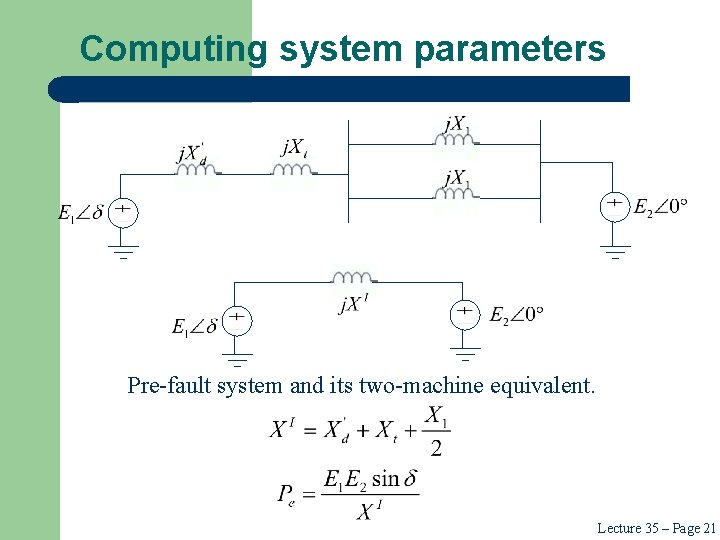
Computing system parameters Pre-fault system and its two-machine equivalent. Lecture 35 – Page 21

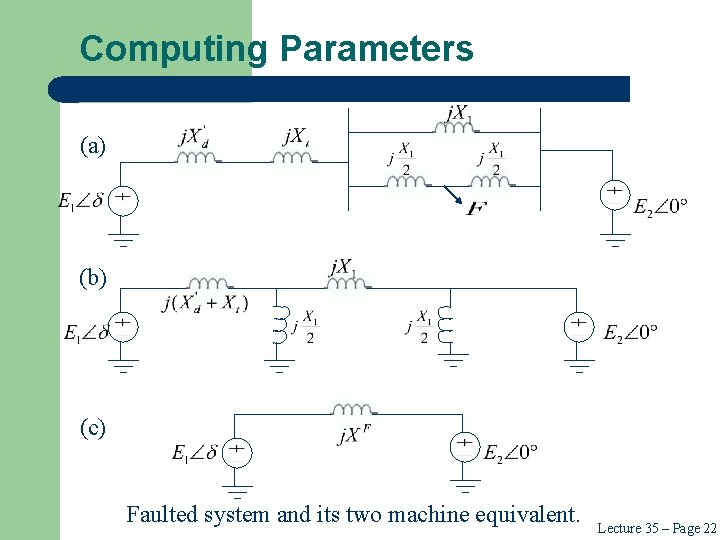
Computing Parameters (a) (b) (c) Faulted system and its two machine equivalent. Lecture 35 – Page 22

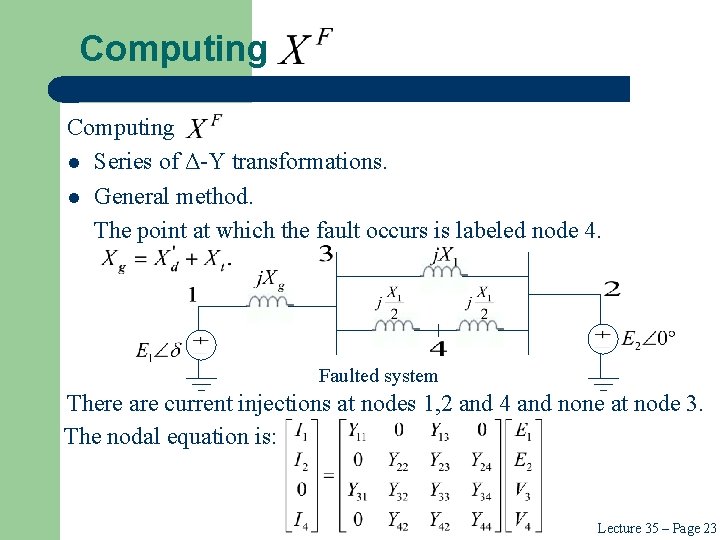
Computing l Series of Δ-Υ transformations. l General method. The point at which the fault occurs is labeled node 4. Faulted system There are current injections at nodes 1, 2 and 4 and none at node 3. The nodal equation is: Lecture 35 – Page 23

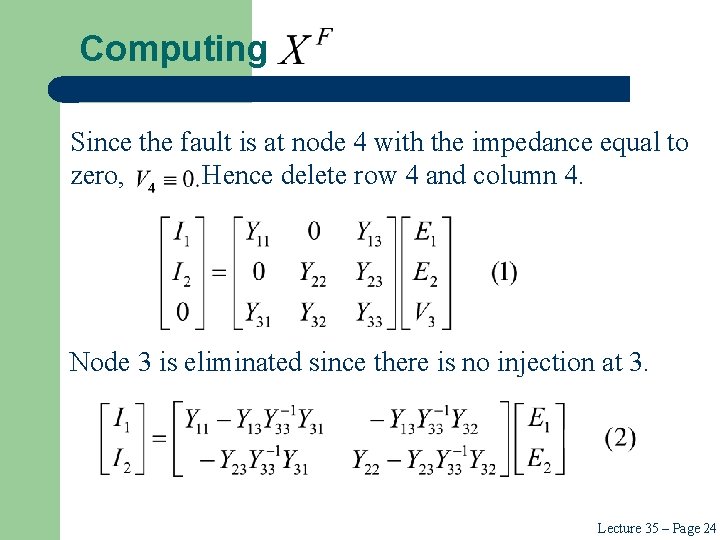
Computing Since the fault is at node 4 with the impedance equal to zero, Hence delete row 4 and column 4. Node 3 is eliminated since there is no injection at 3. Lecture 35 – Page 24

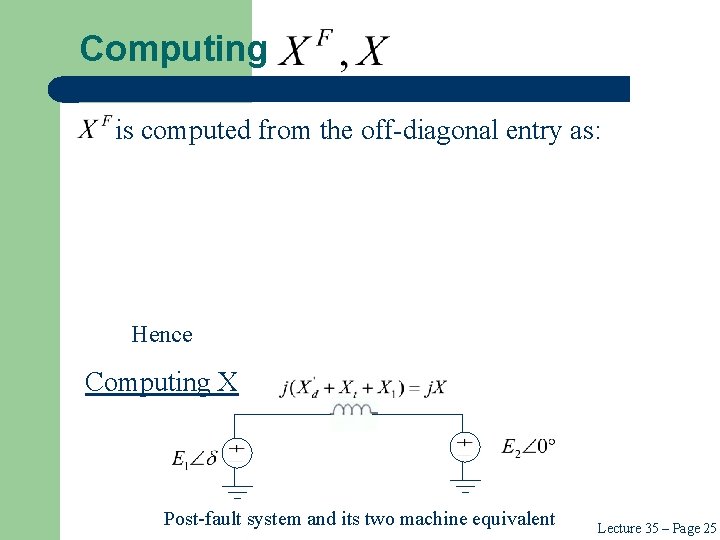
Computing is computed from the off-diagonal entry as: Hence Computing X Post-fault system and its two machine equivalent Lecture 35 – Page 25