EC 941 Game Theory Lecture 5 Prof Francesco

EC 941 - Game Theory Lecture 5 Prof. Francesco Squintani Email: f. squintani@warwick. ac. uk 1

Structure of the Lecture n Definition of Extensive-Form Games n Subgame Perfection and Backward Induction n Applications: Stackelberg Duopoly, Harris-Vickers Race. 2

Extensive Form Games n The strategic form representation is appropriate to describe simultaneous move games. n In order to account for the sequence of moves in a game, we introduce the extensive form representation. n This will allow to refine the predictions of the Nash Equilibrium concept, and formulate more precise and reasonable predictions. 3

An Entry Game n A potential entrant chooses whether to enter a market controlled by a monopolist. n If the entrant enters the market, the monopolist can either start a price war, or share the market with the entrant. n Intuitively, the incumbent cannot credibly commit to a price war the entrant, and hence cannot deter entry. 4

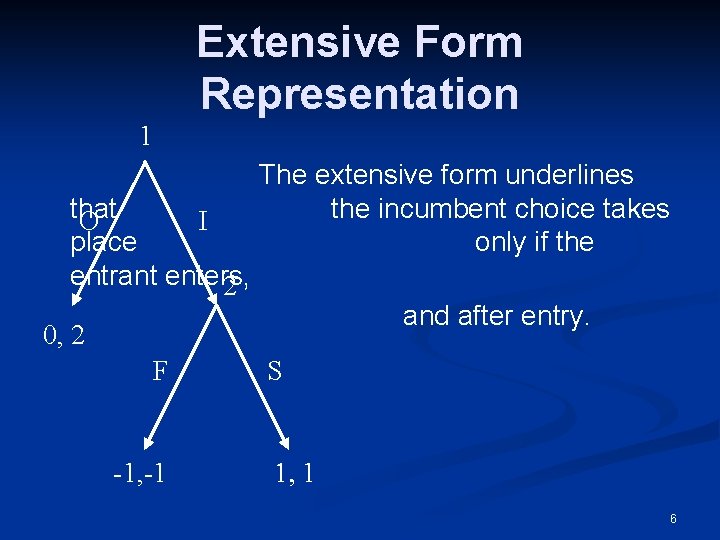
Let us model the entry game in strategic form. Fight In Out Share -1, -1 1, 1 0, 2 The game has two Nash Equilibria: (In, Share) and (Out, Fight). Only the first one is “reasonable”. The second one contains a non-credible threat. 5

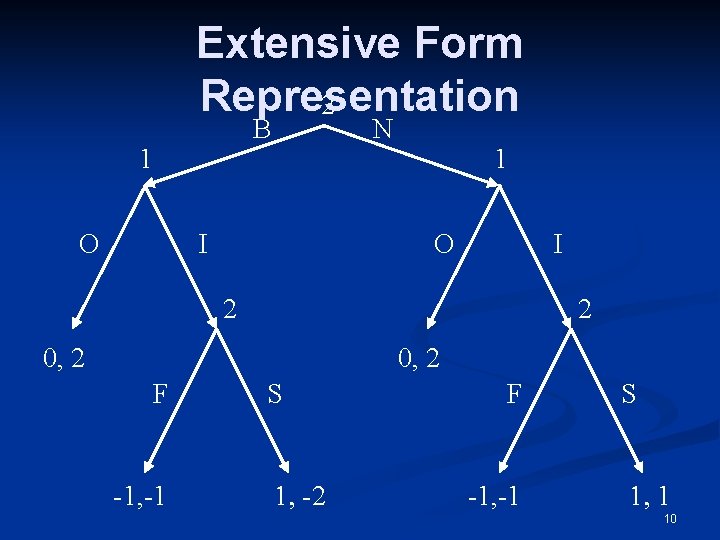
Extensive Form Representation 1 that O I place entrant enters, 2 The extensive form underlines the incumbent choice takes only if the and after entry. 0, 2 F -1, -1 S 1, 1 6

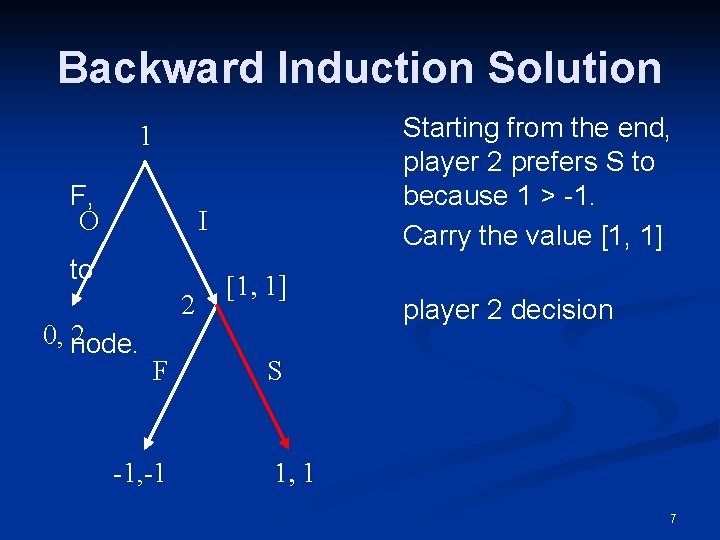
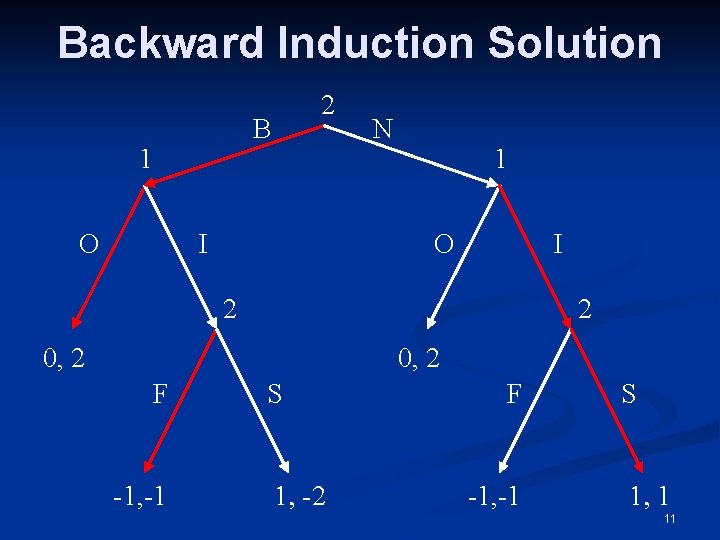
Backward Induction Solution Starting from the end, player 2 prefers S to because 1 > -1. Carry the value [1, 1] 1 F, O I to 0, node. 2 2 F -1, -1 [1, 1] player 2 decision S 1, 1 7

1 [1, 1] plays I, Oplayer over O, I 2 0, to 2 node. F -1, -1 [1, 1] Given that player 2 S if player plays 1 chooses I because 1 > 0. Carry the value [1, 1] S player 1 decision 1, 1 This is the BI value of the game. The BI solution is (I, 8

The Value of Commitment n n Before a potential competitor chooses whether to enter a market or not, the incumbent may or may not “burn bridges”, i. e. make an investment that reduces her payoff if sharing the market with the competitor. By burning bridges, she successfully deters entry. The competitor correctly anticipates that if entering the market, she will be fought by the incumbent. As a result, the competitor chooses not to enter the market. 9

Extensive Form Representation 2 B 1 O N I 1 O I 2 2 0, 2 F -1, -1 S 1, -2 F -1, -1 S 1, 1 10

Backward Induction Solution B 1 O 2 I N 1 O I 2 2 0, 2 F -1, -1 S 1, -2 F -1, -1 S 1, 1 11

General Definitions Definition An extensive game with perfect information G is: n a set of players I, n a set of sequences E (terminal histories) with the property that no sequence is a proper subhistory of any other sequence, n a function P (the player function) that assigns a player to every sequence that is a proper subhistory of some terminal history, n for each player i, preferences ui over the set of terminal histories. 12

For example, in the entry game, n n Players: The challenger and the incumbent. Terminal histories: (In, Share), (In, Fight), and Out. Player function: P(∅) =Challenger, P(In) = Incumbent. Preferences The challenger’s preferences are given by the payoff function u 1 with u 1(I, S) = 1, u 1(O) = 0, u 1(I, F) = -1. The incumbent’s preferences are given by payoff function u 2 with u 2(O) = 2, u 2(I, S) = 1, u 2(I, F) = -1. 13

Strategies and Outcomes Definition A strategy si of player i in an extensive game with perfect information is a function that assigns to each history h after which it is player i’s turn to move (i. e. , P(h) = i) an action in A(h), the set of actions available after h. n A player’s strategy specifies the action the player chooses for every history after which it is her turn to move. 14

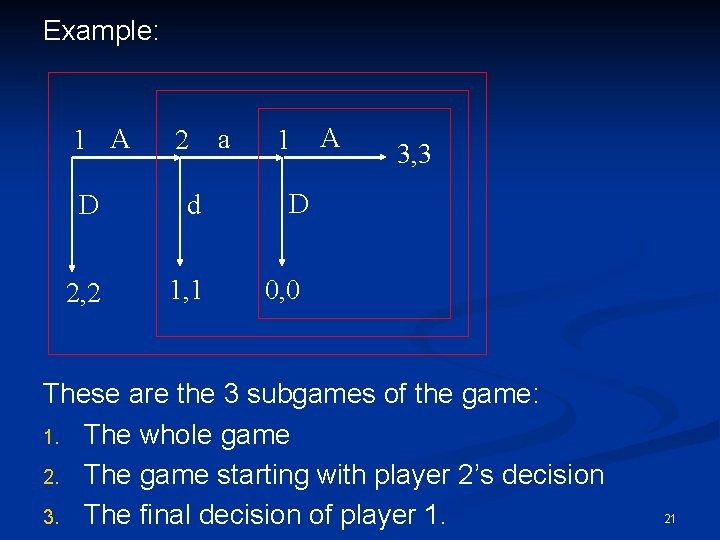
n For example, in the game below, 1 A 2 D d 2, 2 1, 1 a 1 A 3, 3 D 0, 0 the strategies of player 1 are {AA, AD, DA, DD}, and the strategies of player 2 are {a, d}. 15

n Each strategy profile s uniquely determines a terminal history e that is reached through the strategy s. n Because each terminal history e is associated with payoffs u(e), from each extensive form game G = (I, E, P, u), we obtain a normal form game G=(I, S, u), by assigning the utility u(e) to the strategy profiles s identifying terminal node e. 16

n The strategic form representation is: a DD are DA AD AA d 2, 2 0, 0 1, 1 3, 3 1, 1 The Nash Equilibrium (AA, a) and any mix between DA and DD, with player 2 playing d. 17

n In terms of outcomes in the extensive form, they correspond to: 1 A [3, 3] n 2 a [3, 3] D d 2, 2 1, 1 1 A [3, 3] 3, 3 D 0, 0 The backward-induction solution is (AA, a). 18

Theorem In any extensive form game of perfect information, the backward-induction solution is a Nash Equilibrium. Proposition In any extensive form game of perfect information in which there are no ties in payoffs, the backward-induction solution is unique. Proposition There exists Nash Equilibria of extensive form games of perfect information that do not correspond to a backward induction solution. 19

Subgames Definition Let Γ be an extensive game with perfect information, with player function P. For any nonterminal history h of Γ, the subgame Γ(h) following the history h is the following extensive form game: n Players The players in Γ. n Terminal histories The set of all sequences h’ of actions such that (h, h’ ) is a terminal history of Γ. n Player function The player P(h, h’ ) is assigned to each proper subhistory h’ of a terminal history. n Preferences Each player prefers h’ to h’’ if and only if she prefers (h, h’ ) to (h, h’’ ) in Γ. 20

Example: 1 A 2 D d 2, 2 1, 1 a 1 A 3, 3 D 0, 0 These are the 3 subgames of the game: 1. The whole game 2. The game starting with player 2’s decision 3. The final decision of player 1. 21

Subgame Perfect Equilibrium Definition The strategy profile s∗ is a subgame perfect equilibrium if it induces a Nash equilibrium in every subgame. Theorem The strategy profile s∗ is a subgame perfect equilibrium if, for every player i, every history h after which it is player i’s turn to move (i. e. P(h) = i), and every strategy ri of player i, the terminal history Oh(s∗) generated by s∗ after the history h yields utility to player i no less than the 22

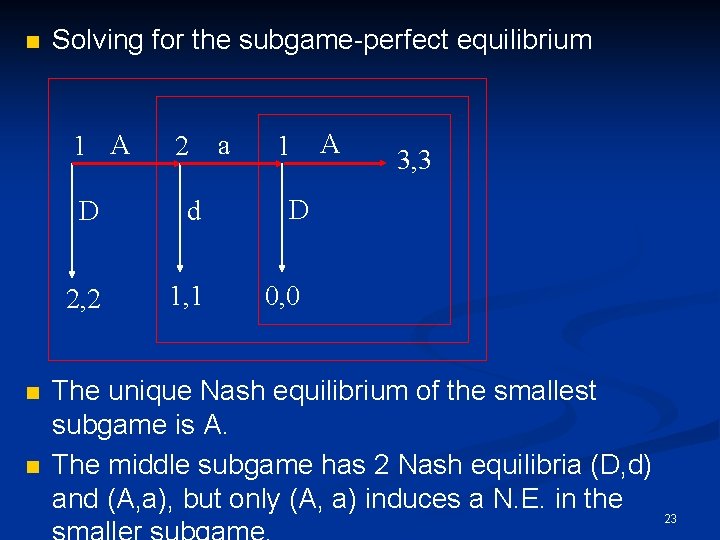
n Solving for the subgame-perfect equilibrium 1 A n n 2 D d 2, 2 1, 1 a 1 A 3, 3 D 0, 0 The unique Nash equilibrium of the smallest subgame is A. The middle subgame has 2 Nash equilibria (D, d) and (A, a), but only (A, a) induces a N. E. in the 23

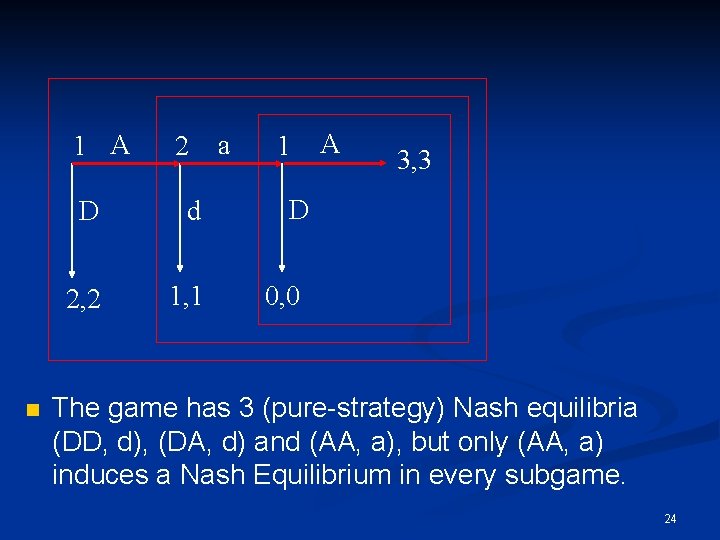
1 A n 2 D d 2, 2 1, 1 a 1 A 3, 3 D 0, 0 The game has 3 (pure-strategy) Nash equilibria (DD, d), (DA, d) and (AA, a), but only (AA, a) induces a Nash Equilibrium in every subgame. 24

n In games of perfect information, the subgame perfect equilibrium coincides with the backwardinduction solution. n But subgame-perfect equilibrium is a more general concept, defined also for extensive form games without perfect information. 25

Application: Stackelberg duopoly There are two firms in the market. n Firm i’ s cost of producing qi units of the good is Ci (qi); the price at which output is sold when the total output is Q is P(Q). n Each firm’s strategy is the output, as in Cournot model. n But the firms make their decisions sequentially, rather than simultaneously. n One firm chooses its output, then the other firm does so, knowing the output chosen by the first 26 n

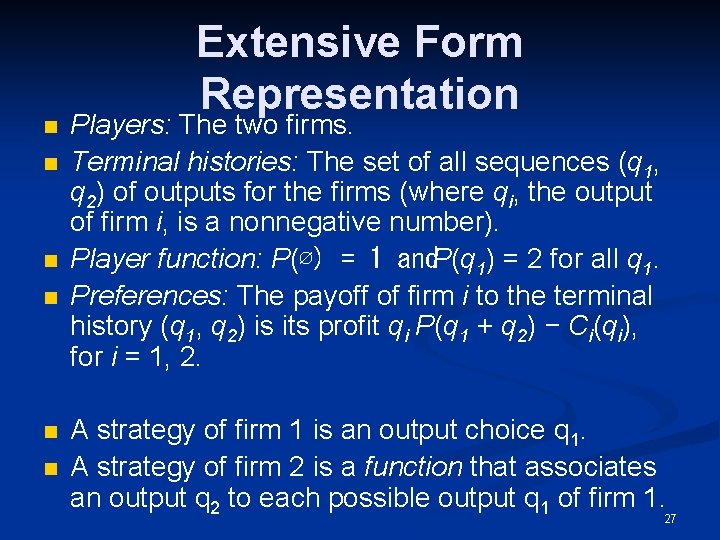
n n n Extensive Form Representation Players: The two firms. Terminal histories: The set of all sequences (q 1, q 2) of outputs for the firms (where qi, the output of firm i, is a nonnegative number). Player function: P(∅) = 1 and. P(q 1) = 2 for all q 1. Preferences: The payoff of firm i to the terminal history (q 1, q 2) is its profit qi P(q 1 + q 2) − Ci(qi), for i = 1, 2. A strategy of firm 1 is an output choice q 1. A strategy of firm 2 is a function that associates an output q 2 to each possible output q 1 of firm 1. 27

Backward Induction Solution n For any q 1 output of firm 1, we find the output b 2(q 1) of firm 2 that maximize its profit q 2 P(q 1 + q 2) – C 2 (q 2). In any subgame perfect equilibrium, firm 2’s strategy is b 2. n We then find the output q 1 of firm 1 that maximize its profit, given the strategy b 2(q 1) of firm 2. Firm 1’s output in a subgame perfect equilibrium is the value q 1 that maximizes q 1 P(q 1 + b 2(q 1)) − C 1 (q 1). 28

n n Suppose that Ci(qi) = cqi for i = 1, 2, and P(Q) = a − Q if Q ≤ a, P(Q) = 0 if Q > a, where c > 0 and c < a. We know that firm 2’s best response to output q 1 of firm 1 is b 2(q 1) = (a − c − q 1)/2 if q 1 ≤ a − c, b 2(q 1) = 0 if q 1 > a − c. In a subgame perfect equilibrium of Stackelberg’s game firm 2’s strategy is this function b 2 and firm 1’s strategy q 1* maximizes q 1 (a − c − (q 1 + (a − c − q 1)/2)) = q 1 (a − c − q 1)/2. The first order condition yields q 1* =(a − c)/2. 29

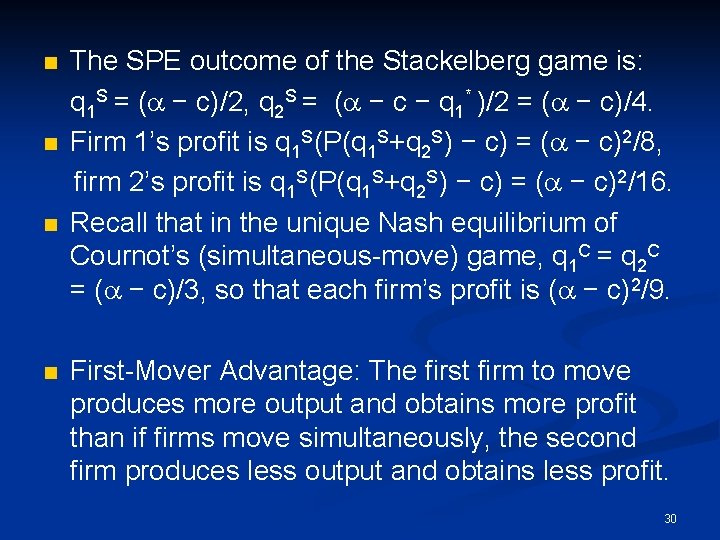
n n The SPE outcome of the Stackelberg game is: q 1 S = (a − c)/2, q 2 S = (a − c − q 1* )/2 = (a − c)/4. Firm 1’s profit is q 1 S(P(q 1 S+q 2 S) − c) = (a − c)2/8, firm 2’s profit is q 1 S(P(q 1 S+q 2 S) − c) = (a − c)2/16. Recall that in the unique Nash equilibrium of Cournot’s (simultaneous-move) game, q 1 C = q 2 C = (a − c)/3, so that each firm’s profit is (a − c)2/9. First-Mover Advantage: The first firm to move produces more output and obtains more profit than if firms move simultaneously, the second firm produces less output and obtains less profit. 30

Application: Harris-Vickers Race n Two firms compete to develop new technologies. n How does competition affect the pace of activity? n How does a leading advantage translates into a final outcome? n We model this race as an extensive game where players alternately choose how many “steps” to take towards a finish line. 31

n Player i = 1, 2 is initially ki > 0 steps from the finish line. n On each of her turns, a player can either take 0 steps (at zero cost), 1 step, at a cost of c(1), or 2 steps, at a cost of c(2) > 2 c(1). n The first player to reach the finish line wins a prize, worth vi > 0 to player i; the losing player’s payoff is 0. n If, on successive turns, neither player takes any step, the game ends and neither player obtains the prize. 32

Extensive Form Representation n The game G 1(k 1, k 2) is defined as follows. n Players: The two parties. n Player function: P(∅) = 1, P(x 1) = 2 for all x 1, P(x 1, y 1) = 1 for all (x 1, y 1), P(x 1, y 1, x 2) = 2 for all (x 1, y 1, x 2), etc. For all t, xt is the number of steps taken by player 1 on her tth turn, and yt are the steps taken by 2 on 33

Terminal histories: The set of sequences of the form (x 1, y 1, x 2, y 2, . . . , x. T) or (x 1, y 1, x 2, y 2, . . . , y. T) for some integer T, where xt and yt are 0, 1, or 2, there are never two successive 0’s except possibly at the end of a sequence, and either x 1+…+x. T = k 1, y 1+…+y. T < k 2 (1 wins the race), or x 1+…+x. T < k 1 and y 1+…+y. T = k 2 (2 wins the race). n n Preferences: For an end history in which player i 34

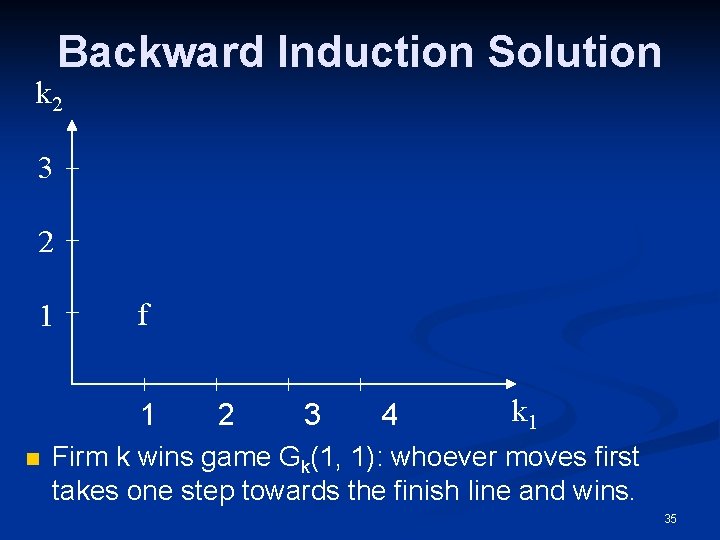
Backward Induction Solution k 2 3 2 1 f 1 n 2 3 4 k 1 Firm k wins game Gk(1, 1): whoever moves first takes one step towards the finish line and wins. 35

4 k 2 3 2 f 1 n f 2 3 4 k 1 Firm 1 wins game G 1(1, 2) by taking 1 step, and wins game G 1(2, 1) by taking 2 steps. (If she took 1 only step, the next round would be equivalent to game G 2(1, 1), where 2 wins. ) 36

4 k 2 3 2 f f 1 n 2 3 4 k 1 Firm 1 wins game G 1(2, 2) by taking 2 steps. (If she took 1 step, the next round would be equivalent to game G 2(1, 2), where 2 wins. ) Similarly, firm 2 wins game G 2(2, 2). 37

4 k 2 1 3 1 2 f f 1 n n Firm 1 wins games G 1(1, 3) and G 1(1, 4) with 1 step. 2 3 4 k 1 In games G 2(1, 3) and G 2(1, 4), firm 2 loses. With 1 step, the next round is G 1(1, 2) and G 1(1, 3), with 2 steps, the next round is G 1(1, 1) and G 1(1, 2): in all such games, firm 1 wins. Hence 2 takes 0 38

k 2 1 1 3 1 1 2 f f 1 f f 4 1 n n 2 3 4 k 1 Firm 1 wins games G 1(2, 3) and G 1(2, 4): with 1 step, the next round is G 2(1, 3) and G 2(1, 4), where firm 2 loses. Firm 2 loses in G 2(2, 3) and G 2(2, 4): with j > 0 steps, 39

4 k 2 1 1 3 1 1 2 f f 2 2 1 n 2 3 4 k 1 Analogously to the previous steps, firm 2 wins games Gk(3, 1), Gk(4, 1), Gk(3, 2) and Gk(4, 2) for k=1, 2. 40

4 k 2 1 1 f f 3 1 1 f f 2 2 1 n n 2 3 4 k 1 Consider games Gk(3, 3), Gk(3, 4), Gk(4, 3) and Gk(4, 4). Reaching “regions” where one player wins for sure is the same as reaching the finish line. 41

k 2 5 1 1 f f 2 2 4 1 1 f f 2 2 3 1 1 f f 2 2 2 1 f f 2 2 2 k 1 1 n 2 3 4 5 6 7 Hence, the matrix can then be completed inductively. 8 42

Summary of the Lecture n Definition of Extensive-Form Games n Subgame Perfection and Backward Induction n Applications: Stackelberg Duopoly, Harris-Vickers Race. 43

Preview of the Next Lecture n Extensive-Form Games with Imperfect Information n Spence Signalling Game n Crawford and Sobel Cheap Talk 44