Dr Scheme Dr Scheme PLT Scheme Dr Scheme


















![; ; sort: list-of-numbers -> list-of-numbers (define (sort alon) (cond [(empty? alon) empty] [else ; ; sort: list-of-numbers -> list-of-numbers (define (sort alon) (cond [(empty? alon) empty] [else](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-26.jpg)


![インサーションソート • sort の内部に sort が登場 (define (sort alon) (cond [(empty? alon) empty] [else インサーションソート • sort の内部に sort が登場 (define (sort alon) (cond [(empty? alon) empty] [else](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-29.jpg)











![「例題5.大きな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (larger-items alon threshold) (cond [(empty? alon) empty] 「例題5.大きな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (larger-items alon threshold) (cond [(empty? alon) empty]](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-45.jpg)







![「例題6.小さな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (smaller-items alon threshold) (cond [(empty? alon) empty] 「例題6.小さな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (smaller-items alon threshold) (cond [(empty? alon) empty]](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-55.jpg)









![繰り返し処理 • quick-sort の内部に quick-sort が登場 (define (quick-sort alon) 終了(cond [(empty? alon) empty] 自明な解 繰り返し処理 • quick-sort の内部に quick-sort が登場 (define (quick-sort alon) 終了(cond [(empty? alon) empty] 自明な解](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-69.jpg)













![住所録構造体のクイックソート (2/2) (define (smaller-items a-list threshold) (cond [(empty? a-list) empty] [else (cond [(string<? (address-record-name 住所録構造体のクイックソート (2/2) (define (smaller-items a-list threshold) (cond [(empty? a-list) empty] [else (cond [(string<? (address-record-name](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-83.jpg)

- Slides: 84











Dr. Scheme の使用 • Dr. Scheme の起動 プログラム → PLT Scheme → Dr. Scheme • 今日の演習では「Intermediate Student 」 に設定 Language → Choose Language → Intermediate Student





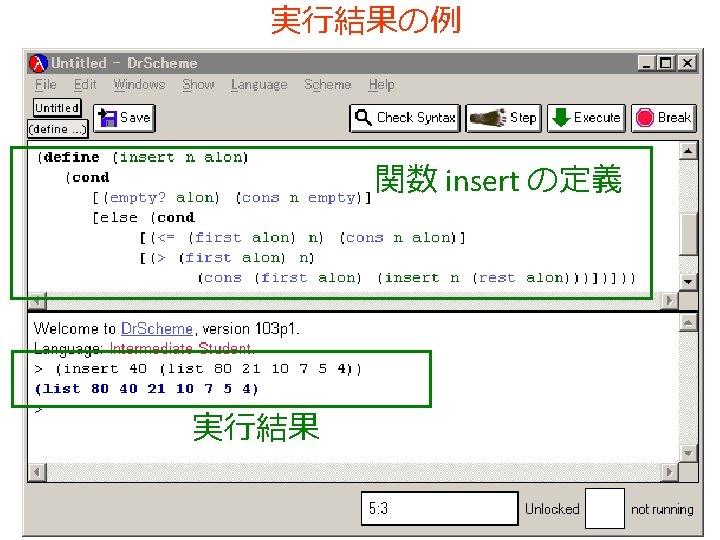
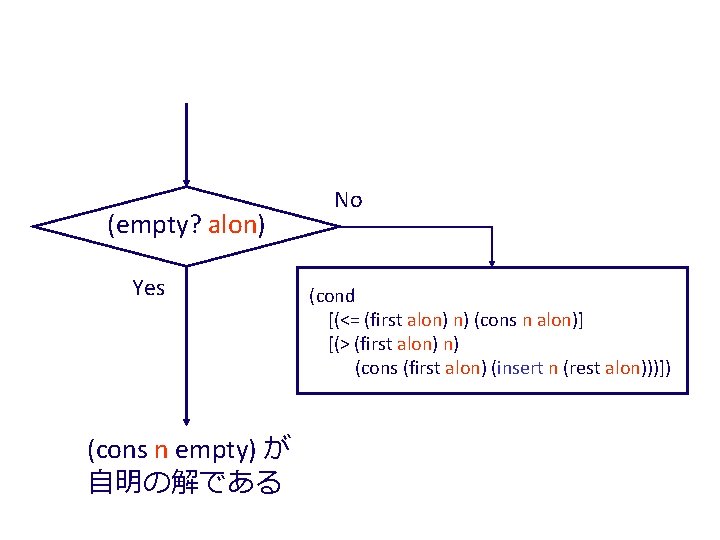
; ; insert: number list-of-numbers->list-of-numbers ; ; to create a list of numbers from n and the numbers ; ; on alon that is sorted in descending order; alon is ; ; already sorted ; ; insert: number list-of-numbers(sorted) ; ; -> list-of-numbers(sorted) (define (insert n alon) (cond [(empty? alon) (cons n empty)] [else (cond [(<= (first alon) n) (cons n alon)] [(> (first alon) n) (cons (first alon) (insert n (rest alon)))])]))


(empty? alon) Yes (cons n empty) が 自明の解である No (cond [(<= (first alon) n) (cons n alon)] [(> (first alon) n) (cons (first alon) (insert n (rest alon)))])

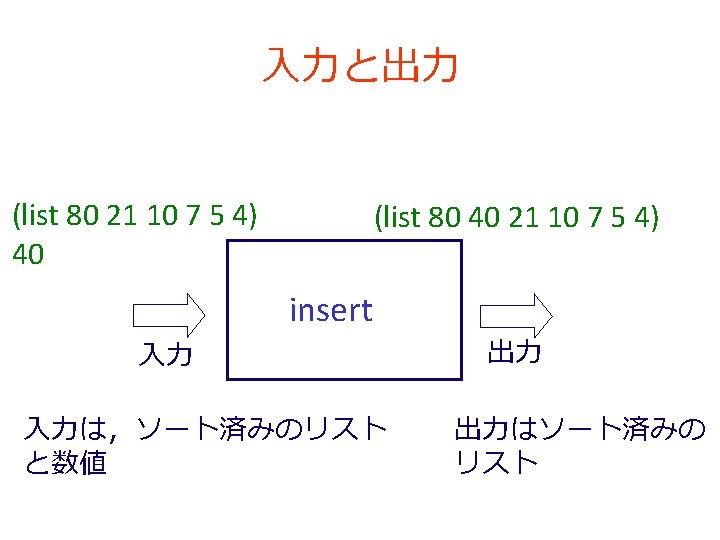
要素の挿入 • insert の内部に insert が登場 (define (insert n alon) (cond [(empty? alon) (cons n empty)] [else (cond [(<= (first alon) n) (cons n alon)] [(> (first alon) n) (cons (first alon) (insert n (rest alon)))])])) • insert の実行が繰り返される 例: (insert 40 (list 80 21 10 7 5 4)) = (cons 80 (insert 40 (list 21 10 7 5 4))) ⇒ まさに「再帰」である

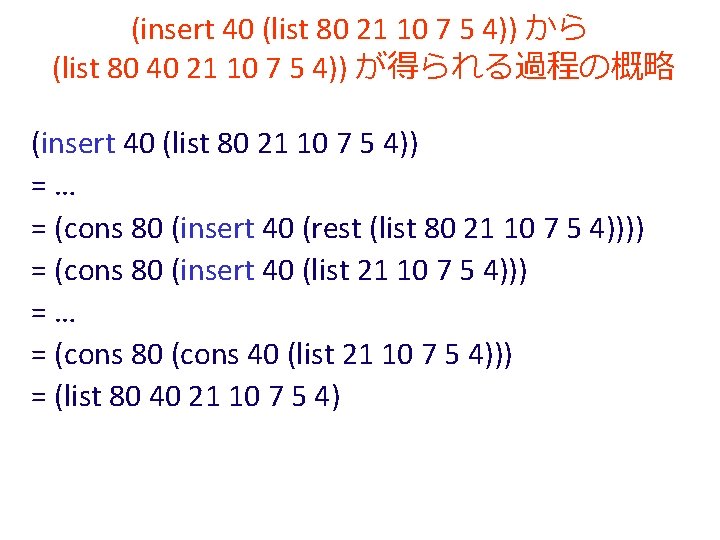
(insert 40 (list 80 21 10 7 5 4)) から (list 80 40 21 10 7 5 4)) が得られる過程の概略 (insert 40 (list 80 21 10 7 5 4)) =… = (cons 80 (insert 40 (rest (list 80 21 10 7 5 4)))) = (cons 80 (insert 40 (list 21 10 7 5 4))) =… = (cons 80 (cons 40 (list 21 10 7 5 4))) = (list 80 40 21 10 7 5 4)

(insert 40 (list 80 21 10 7 5 4)) から (list 80 40 21 10 7 5 4)) が得られる過程の概略 (insert 40 (list 80 21 10 7 5 4)) =… = (cons 80 (insert 40 (rest (list 80 21 10 7 5 4)))) =これは, (cons 80 (insert 40 (list 21 10 7 5 4))) =(define … (insert n alon) (cond 80 (cons 40 (list 21 10 7 5 4))) = (cons [(empty? alon) (cons n empty)] = (list 80(cond 40 21 10 7 5 4) [else [(<= (first alon) n) (cons n alon)] [(> (first alon) n) (cons (first alon) (insert n (rest alon)))])])) の alon を (list 80 21 10 7 5 4) で,n を 40 で置き換えたもの


「例題2.インサーションソート」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す ; ; sort: list-of-numbers -> list-of-numbers (define (sort alon) (cond [(empty? alon) empty] [else (insert (first alon) (sort (rest alon)))])) (define (insert n alon) (cond [(empty? alon) (cons n empty)] [else (cond [(<= (first alon) n) (cons n alon)] [(> (first alon) n) (cons (first alon) (insert n (rest alon)))])])) 例題1 と同じ 2. その後,次を「実行用ウインドウ」で実行しなさい (sort (list 3 5 1 4)) ☆ 次は,例題3に進んでくだ


![sort listofnumbers listofnumbers define sort alon cond empty alon empty else ; ; sort: list-of-numbers -> list-of-numbers (define (sort alon) (cond [(empty? alon) empty] [else](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-26.jpg)
; ; sort: list-of-numbers -> list-of-numbers (define (sort alon) (cond [(empty? alon) empty] [else (insert (first alon) (sort (rest alon)))])) ; ; insert: number list-of-numbers(sorted) -> list-of-numbers(sorted) (define (insert n alon) (cond [(empty? alon) (cons n empty)] [else (cond [(<= (first alon) n) (cons n alon)] [(> (first alon) n) (cons (first alon) (insert n (rest alon)))])]))


(empty? alon) No Yes (insert (first alon) (sort (rest alon))) empty が自明の解である
![インサーションソート sort の内部に sort が登場 define sort alon cond empty alon empty else インサーションソート • sort の内部に sort が登場 (define (sort alon) (cond [(empty? alon) empty] [else](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-29.jpg)
インサーションソート • sort の内部に sort が登場 (define (sort alon) (cond [(empty? alon) empty] [else (insert (first alon) (sort (rest alon)))])) • sort の実行が繰り返される 例: (sort (list 3 5 1 4)) = (insert 3 (sort (list 5 1 4))) ⇒ まさに「再帰」である

(sort (list 3 5 1 4)) から (list 5 4 3 1)) が得られる過程の概略 (1/2) (sort (list 3 5 1 4)) =… = (insert 3 (sort (rest (list 3 5 1 4)))) = (insert 3 (sort (list 5 1 4))) =… = (insert 3 (insert 5 (sort (rest (list 5 1 4))))) = (insert 3 (insert 5 (sort (list 1 4)))) =… = (insert 3 (insert 5 (insert 1 (sort (rest (list 1 4)))))) = (insert 3 (insert 5 (insert 1 (sort (list 4))))) =… = (insert 3 (insert 5 (insert 1 (insert 4 (sort (rest (list 4))))))) = (insert 3 (insert 5 (insert 1 (insert 4 (sort empty))))) =… = (insert 3 (insert 5 (insert 1 (insert 4 empty)))) 次ページへ

(sort (list 3 5 1 4)) から (list 5 4 3 1)) が得られる過程の概略 (1/2) (sort (list 3 5 1 4)) =… = (insert 3 (sort (rest (list 3 5 1 4)))) = (insert 3 (sort (list 5 1 4))) =… これは, = (insert 3 (insert 5 (sort (rest (list 5 1 4))))) alon) = (insert(define 3 (insert(sort 5 (sort (list 1 4)))) =… (cond = (insert 3 (insert 5 (insert 1 (sort (rest (list 1 4)))))) [(empty? alon) empty] = (insert 3 (insert 5 (insert 1 (sort (list 4))))) [else (insert (first alon) (sort (rest alon)))])) =… の alon を (list 3 5 1 4) で置き換えたもの = (insert 3 (insert 5 (insert 1 (insert 4 (sort (rest (list 4))))))) = (insert 3 (insert 5 (insert 1 (insert 4 (sort empty))))) =… = (insert 3 (insert 5 (insert 1 (insert 4 empty)))) 次ページへ

(sort (list 3 5 1 4)) から (list 5 4 3 1)) が得られる過程の概略 (2/2) = (insert 3 (insert 5 (insert 1 (insert 4 empty)))) =… = (insert 3 (insert 5 (cons 4 (insert 1 (rest (list 4)))))) = (insert 3 (insert 5 (cons 4 (insert 1 empty)))) =… = (insert 3 (insert 5 (cons 4 (cons 1 empty)))) =… = (insert 3 (cons 5 (list 4 1))) =… = (cons 5 (insert 3 (list 4 1))) =… = (cons 5 (cons 4 (insert 3 (list 1))) =… = (cons 5 (cons 4 (cons 3 (list 1))) = (list 5 4 3 1)



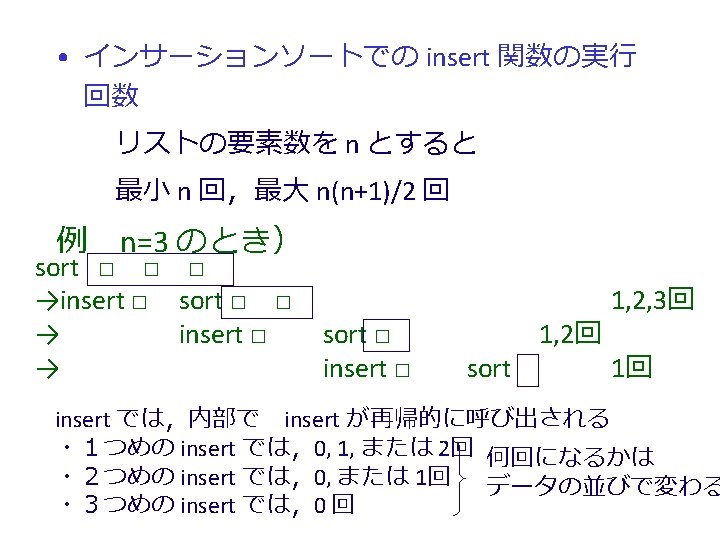
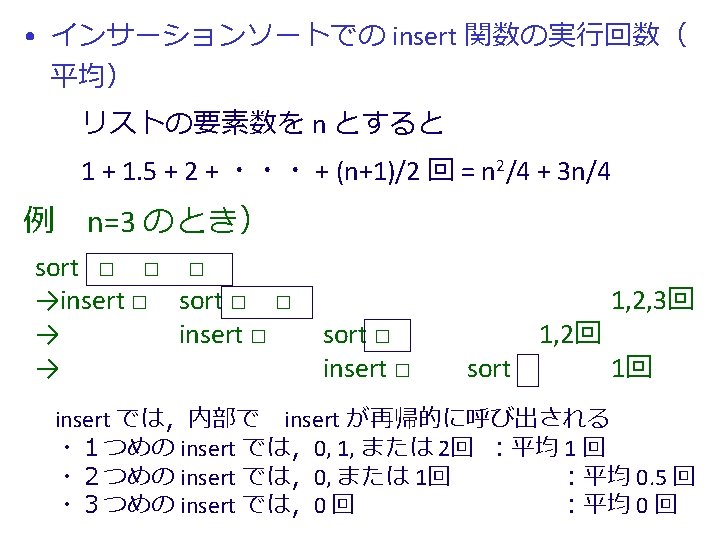
• インサーションソートでの sort 関数の実行 回数 リストの要素数を n とすると n+1 回 例 n=3 のとき) (sort (list 80 30 50)) = (insert 80 (sort (list 30 50))) = (insert 80 (insert 30 (sort (list 50)))) = (insert 80 (insert 30 (insert 50 (sort empty))))



• インサーションソートでの sort 関数の実行 回数 リストの要素数を n とすると n+1 回 例 n=3 のとき) (sort (list 80 30 50)) = (insert 80 (sort (list 30 50))) = (insert 80 (insert 30 (sort (list 50)))) = (insert 80 (insert 30 (insert 50 (sort empty))))


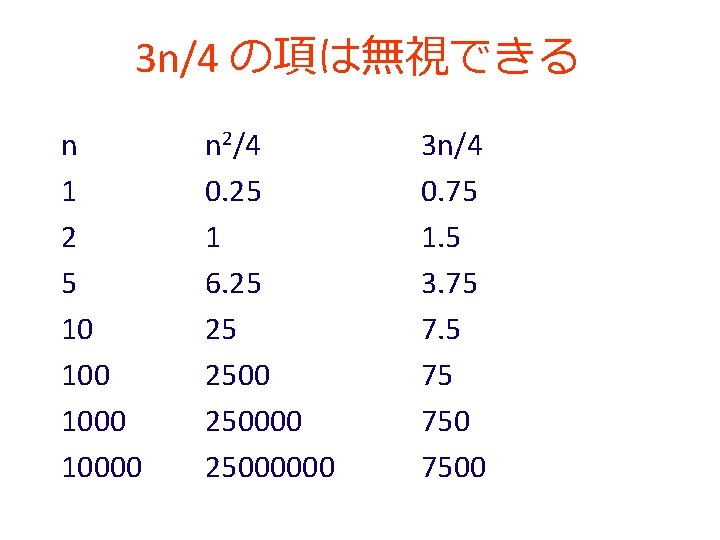
3 n/4 の項は無視できる n 1 2 5 10 10000 n 2/4 0. 25 1 6. 25 25 25000000 3 n/4 0. 75 1. 5 3. 75 7. 5 75 7500




![例題5大きな要素の選択の手順 1 次を定義用ウインドウで実行しなさい 入力した後にExecute ボタンを押す define largeritems alon threshold cond empty alon empty 「例題5.大きな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (larger-items alon threshold) (cond [(empty? alon) empty]](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-45.jpg)
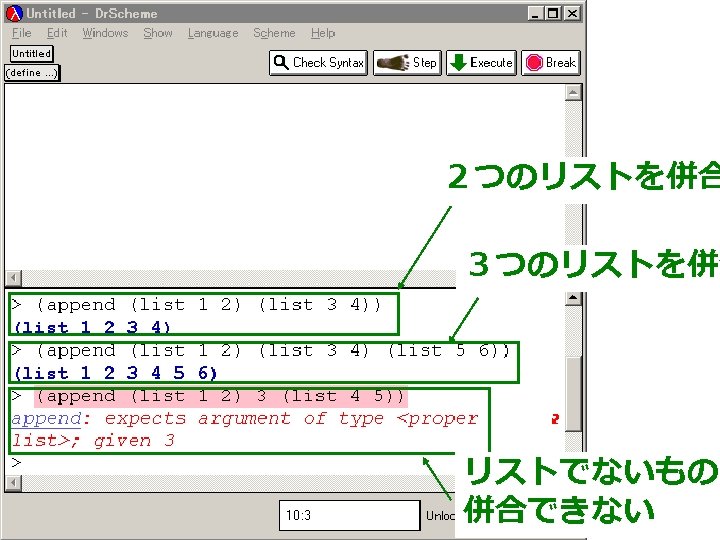
「例題5.大きな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (larger-items alon threshold) (cond [(empty? alon) empty] [else (cond [(>= (first alon) threshold) (cons (first alon) (larger-items (rest alon) threshold))] [else (larger-items (rest alon) threshold)])])) 2. その後,次を「実行用ウインドウ」で実行しなさい (larger-items (list 1 2 3 10 11 12 4 5 6) 6) ☆ 次は,例題6に進んでくだ


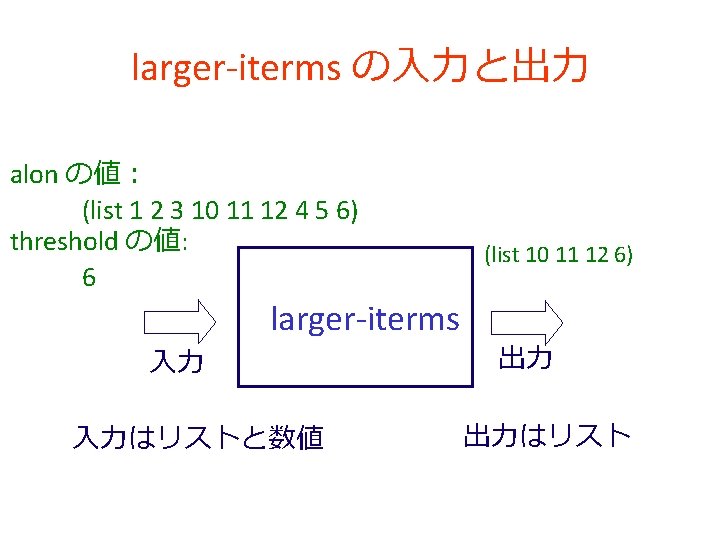
larger-iterms の入力と出力 alon の値: (list 1 2 3 10 11 12 4 5 6) threshold の値: 6 larger-iterms 入力 入力はリストと数値 (list 10 11 12 6) 出力 出力はリスト

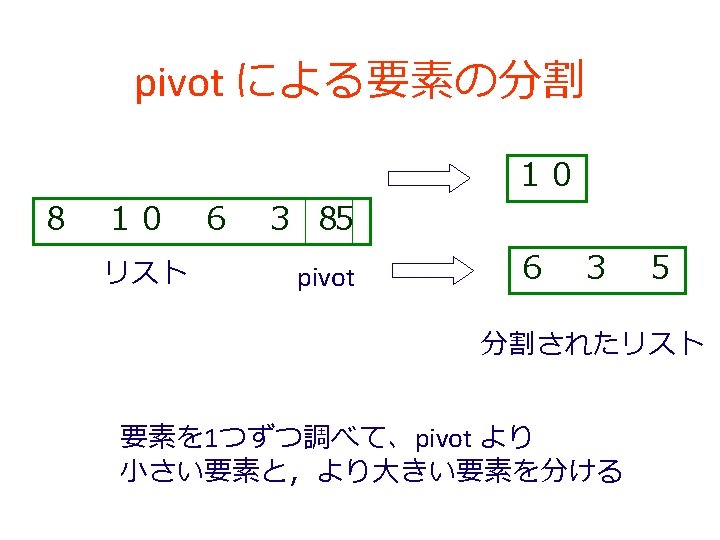
; ; larger-items: list-of-numbers number -> list-of-numbers ; ; alon から threshold 以上の数を選びリストを作る (define (larger-items alon threshold) (cond [(empty? alon) empty] [else (cond [(>= (first alon) threshold) (cons (first alon) (larger-items (rest alon) threshold))] [else (larger-items (rest alon) threshold)])]))


繰り返し処理 (empty? alon) No Yes empty が解 (cond [(>= (first alon) threshold) (cons (first alon) (larger-items (rest alon) threshold))] [else (larger-items (rest alon) threshold)])

繰り返し処理 • larger-items の内部に larger-items が登場 (define (larger-items alon threshold) (cond 終了 [(empty? alon) empty] 自明な解 条件 [else (cond [(>= (first alon) threshold) (cons (first alon) (larger-items (rest alon) threshold))] [else (larger-items (rest alon) threshold)])])) • larger-items の実行が繰り返される 例: (larger-items (list 6 4 2) 3) = (cons 6 (larger-items (list 4 2) 3)) ⇒ まさに「再帰」である

(larger-items (list 6 2 4) 3) から (list 6 4) が得られる過程の概略 (larger-items (list 6 2 4) 3) =… = (cons 6 (larger-items (list 2 4) 3)) =. . . = (cons 6 (larger-items (list 4) 3)) =. . . = (cons 6 (cons 4 (larger-items empty 3))) =. . . = (cons 6 (cons 4 empty)) = (list 6 4)

(larger-items (list 6 2 4) 3) から (list 6 4) が得られる過程の概略 (larger-items (list 6 2 4) 3) =… = (cons 6 (larger-items (list 2 4) 3)) =. . . これは, = (cons 6(define (larger-items (list alon 4) 3)) (larger-items threshold) (cond =. . . [(empty? alon) empty] [else = (cons 6 (cons 4 (larger-items empty 3))) (cond [(>= (first alon) threshold) =. . . (cons (first alon) = (cons 6 (cons 4 empty)) (larger-items (rest alon) threshold))] [else (larger-items (rest alon) threshold)])])) = (list 6 4) の alon を (list 6 2 4) で,threshold を 3 で置き換えたもの

![例題6小さな要素の選択の手順 1 次を定義用ウインドウで実行しなさい 入力した後にExecute ボタンを押す define smalleritems alon threshold cond empty alon empty 「例題6.小さな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (smaller-items alon threshold) (cond [(empty? alon) empty]](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-55.jpg)
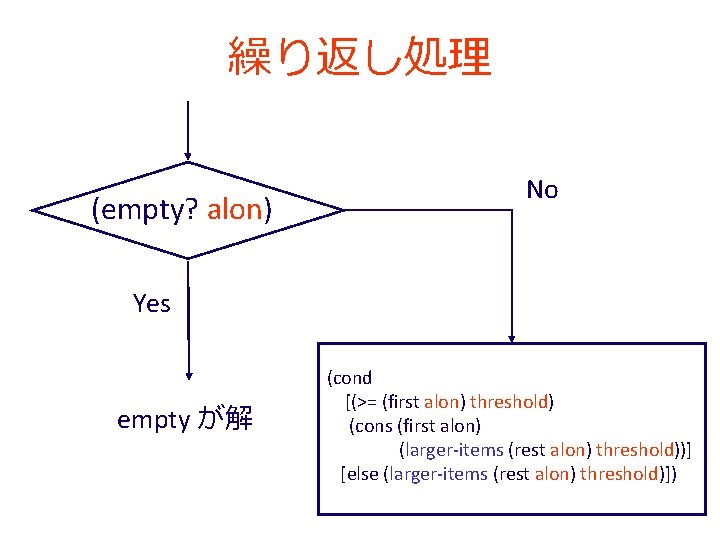
「例題6.小さな要素の選択」の手順 1. 次を「定義用ウインドウ」で,実行しなさい • 入力した後に,Execute ボタンを押す (define (smaller-items alon threshold) (cond [(empty? alon) empty] [else (cond [(< (first alon) threshold) (cons (first alon) (smaller-items (rest alon) threshold))] [else (smaller-items (rest alon) threshold)])])) 2. その後,次を「実行用ウインドウ」で実行しなさい (smaller-items (list 1 2 3 10 11 12 4 5 6) 6) ☆ 次は,例題7に進んでくだ


; ; smaller-items: list-of-numbers number -> list-of-numbers ; ; alon から threshold より小さな数を選びリストを作 る (define (smaller-items alon threshold) (cond [(empty? alon) empty] [else (cond [(< (first alon) threshold) (cons (first alon) (smaller-items (rest alon) threshold))]


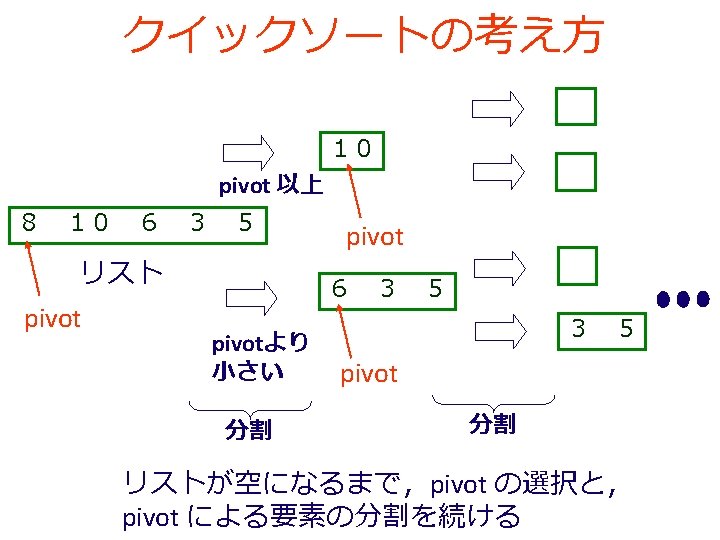
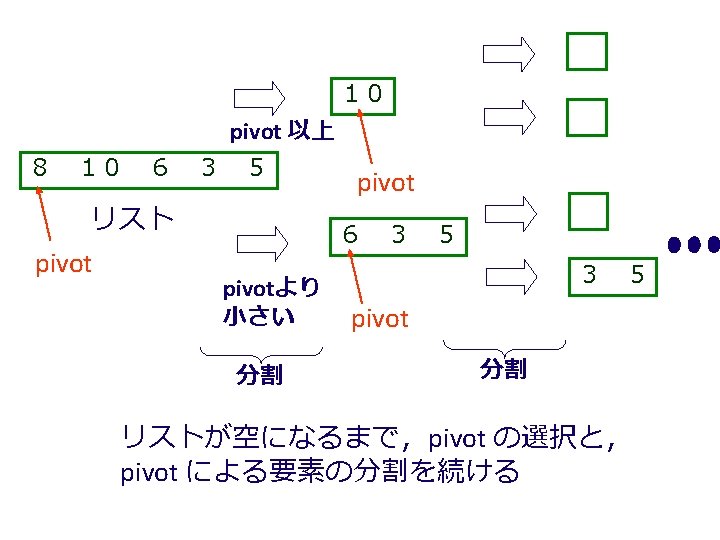
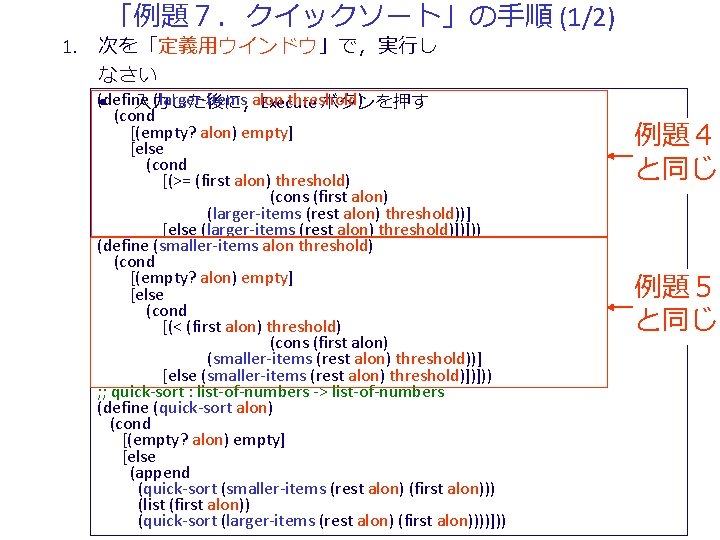
「例題7.クイックソート」の手順 (1/2) 1. 次を「定義用ウインドウ」で,実行し なさい (define (larger-items alon threshold) • 入力した後に,Execute ボタンを押す (cond [(empty? alon) empty] [else (cond [(>= (first alon) threshold) (cons (first alon) (larger-items (rest alon) threshold))] [else (larger-items (rest alon) threshold)])])) (define (smaller-items alon threshold) (cond [(empty? alon) empty] [else (cond [(< (first alon) threshold) (cons (first alon) (smaller-items (rest alon) threshold))] [else (smaller-items (rest alon) threshold)])])) ; ; quick-sort : list-of-numbers -> list-of-numbers (define (quick-sort alon) (cond [(empty? alon) empty] [else (append (quick-sort (smaller-items (rest alon) (first alon))) (list (first alon)) (quick-sort (larger-items (rest alon) (first alon))))])) 例題4 と同じ 例題5 と同じ




クイックソートのプログラム ; ; quick-sort : list-of-numbers -> list-of-numbers (define (quick-sort alon) (cond [(empty? alon) empty] [else (append (quick-sort (smaller-items (rest alon) (first alon))) (list (first alon)) (quick-sort (larger-items (rest alon) (first alon))))]))





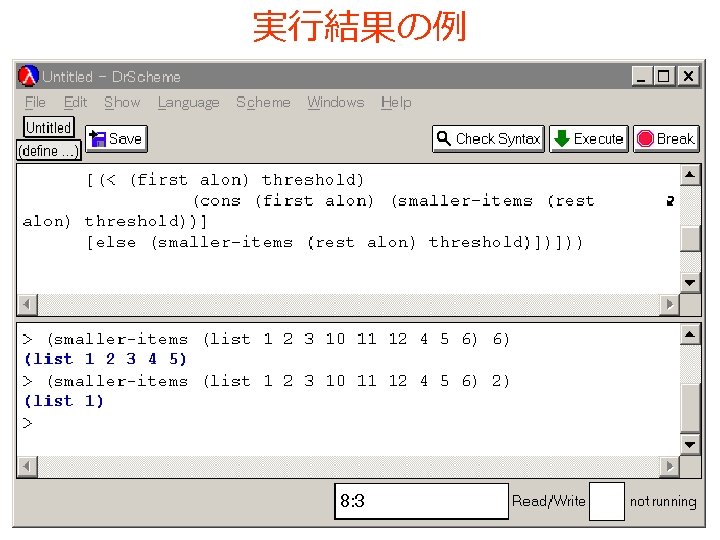
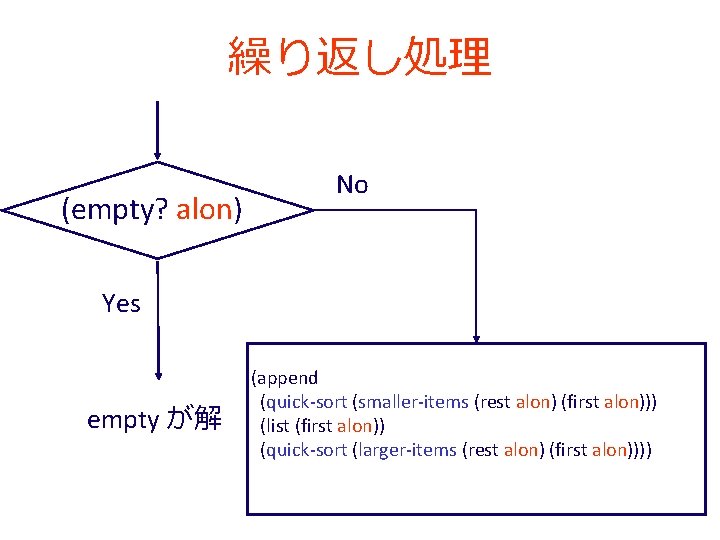
繰り返し処理 (empty? alon) No Yes empty が解 (append (quick-sort (smaller-items (rest alon) (first alon))) (list (first alon)) (quick-sort (larger-items (rest alon) (first alon))))
![繰り返し処理 quicksort の内部に quicksort が登場 define quicksort alon 終了cond empty alon empty 自明な解 繰り返し処理 • quick-sort の内部に quick-sort が登場 (define (quick-sort alon) 終了(cond [(empty? alon) empty] 自明な解](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-69.jpg)
繰り返し処理 • quick-sort の内部に quick-sort が登場 (define (quick-sort alon) 終了(cond [(empty? alon) empty] 自明な解 条件 [else (append (quick-sort (smaller-items (rest alon) (first alon))) (list (first alon)) (quick-sort (larger-items (rest alon) (first alon))))])) • quick-sort の実行が繰り返される 例: ⇒ まさに「再帰」である

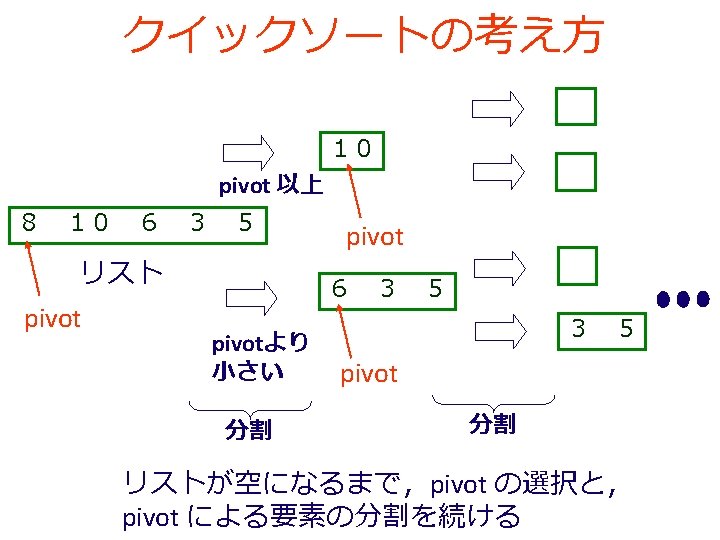
(quick-sort (list 6 2 4)) からの過程 (quick-sort (list 6 2 4)) =… = (append (quick-sort (smaller-items (rest (list 6 2 4)) (first (list 6 2 4)))) (list (first (list 6 2 4))) (quick-sort (larger-items (rest (list 6 2 4)) (first (list 6 2 4)))))])) 要するに、3つのリスト (quick-sort (smaller-items (list 2 4) 6) (list 6) (quick-sort (larger-items (list 2 4) 6) に分割されている → 6 以上 → (list 6) → 6 未満





; ; larger-items: list of address-note, number -> listof numbers ; ; a-list から threshold 以上の数を選びリストを作る (define (larger-items a-list threshold) (cond [(empty? a-list) empty] [else (cond [(string>=? (address-record-name (first a-list)) threshold) (cons (first a-list) (larger-items (rest a-list) threshold))] [else (larger-items (rest a-list) threshold)])]))

; ; smaller-items: list of data, number -> listof numbers ; ; a-list から threshold より小さな数を選びリストを作る (define (smaller-items a-list threshold) (cond [(empty? a-list) empty] [else (cond [(string<? (address-record-name (first a-list)) threshold) (cons (first a-list) (smaller-items (rest a-list) threshold))] [else (smaller-items (rest a-list) threshold)])]))

クイックソートのプログラム ; ; quick-sort : list of data -> list of numbers (define (quick-sort a-list) (cond [(empty? a-list) empty] [else (append (quick-sort (smaller-items (rest a-list) (address-record-name (first a-list)))) (list (first a-list)) (quick-sort (larger-items (rest a-list) (address-record-name (first a-list)))))]))

(quick-sort book) からの過程の概略 (quick-sort book) = (quick-sort (list (make-address-record "Mike" 10 "Fukuoka") (make-address-record "Bill" 20 "Saga") (make-address-record "Ken" 30 "Nagasaki"))) =. . . = (append (quick-sort (smaller-items (list (make-address-record "Bill" 20 "Saga") (make-address-record "Ken" 30 "Nagasaki")) "Mike")) (list (make-address-record "Mike" 10 "Fukuoka")) (quick-sort (larger-items (list (make-address-record "Bill" 20 "Saga") (make-address-record "Ken" 30 "Nagasaki")) "Mike"))


課題1 • 関数 quick-sort (授業の例題5)について の問題 – (quick-sort (list 8 10 6 3 5)) から (list 3 5 6 8 10) が 得られる過程の概略を数行程度で説明しなさ い ; ; quick-sort : list-of-numbers -> list-of-numbers (define (quick-sort alon) (cond [(empty? alon) empty] [else (append (quick-sort (smaller-items (rest alon) (first alon))) (list (first alon)) (quick-sort (larger-items (rest alon) (first alon))))]))


住所録構造体のクイックソート (1/2) (define-struct address-record (name age address)) (define book (list (make-address-record "Mike" 10 "Fukuoka") (make-address-record "Bill" 20 "Saga") (make-address-record "Ken" 30 "Nagasaki"))) (define (larger-items a-list threshold) (cond [(empty? a-list) empty] [else (cond [(string>=? (address-record-name (first a-list)) threshold) (cons (first a-list) (larger-items (rest a-list) threshold))] [else (larger-items (rest a-list) threshold)])]))
![住所録構造体のクイックソート 22 define smalleritems alist threshold cond empty alist empty else cond string addressrecordname 住所録構造体のクイックソート (2/2) (define (smaller-items a-list threshold) (cond [(empty? a-list) empty] [else (cond [(string<? (address-record-name](https://slidetodoc.com/presentation_image_h/8fc4c5d7c6707fa1e10715aa66e33056/image-83.jpg)
住所録構造体のクイックソート (2/2) (define (smaller-items a-list threshold) (cond [(empty? a-list) empty] [else (cond [(string<? (address-record-name (first a-list)) threshold) (cons (first a-list) (smaller-items (rest a-list) threshold))] [else (smaller-items (rest a-list) threshold)])])) (define (quick-sort a-list) (cond [(empty? a-list) empty] [else (append (quick-sort (smaller-items (rest a-list) (address-record-name (first a-list)))) (list (first a-list)) (quick-sort (larger-items (rest a-list) (address-record-name (first a-list)))))]))

 Plt scheme
Plt scheme Plt morphology
Plt morphology Plt fuzzy set
Plt fuzzy set Communication model
Communication model Fcps my plt
Fcps my plt Kewenangan plt dan plh dalam aspek keuangan
Kewenangan plt dan plh dalam aspek keuangan Sysmex xe2100
Sysmex xe2100 Plt morphology
Plt morphology Plt electronics
Plt electronics Billy b and plt
Billy b and plt Cbc with plt
Cbc with plt Emilim
Emilim Mdm hydra
Mdm hydra Anu mlp
Anu mlp Import numpy as np import matplotlib.pyplot as plt
Import numpy as np import matplotlib.pyplot as plt Plt 106
Plt 106 Plt skills
Plt skills Uts student admin
Uts student admin Principio impedenziometrico
Principio impedenziometrico Plt
Plt Introduction to high voltage technology
Introduction to high voltage technology Plt skills
Plt skills Wbc ip message blasts/abn lympho
Wbc ip message blasts/abn lympho Stata graph schemes download
Stata graph schemes download Consumer financial services pensacola fl
Consumer financial services pensacola fl 3 domain scheme and 5 kingdom scheme
3 domain scheme and 5 kingdom scheme Keywords past simple
Keywords past simple Love is not all about
Love is not all about Zero deposit scheme pros and cons
Zero deposit scheme pros and cons Computer games teach us nothing
Computer games teach us nothing Scheme of postembryonic hematopoiesis - lymphocytopoiesis
Scheme of postembryonic hematopoiesis - lymphocytopoiesis Much madness is divinest sense line by line analysis
Much madness is divinest sense line by line analysis Salsa scheme
Salsa scheme Iambic pentameter rhyme scheme
Iambic pentameter rhyme scheme Continuous progression scheme
Continuous progression scheme Indiareit fund scheme v
Indiareit fund scheme v Francesco petrarca 1304 1374
Francesco petrarca 1304 1374 A devoted son comprehension marking scheme
A devoted son comprehension marking scheme Tetrad color scheme interior design
Tetrad color scheme interior design Parish buying scheme
Parish buying scheme Humpty dumpty rhyme scheme
Humpty dumpty rhyme scheme Balance in plating
Balance in plating Internal rhyme examples
Internal rhyme examples When in disgrace with fortune and men's eyes summary
When in disgrace with fortune and men's eyes summary Dulce et decorum est techniques
Dulce et decorum est techniques Zed scheme
Zed scheme General loader scheme
General loader scheme Standards rhyme
Standards rhyme Arbitrary color scheme
Arbitrary color scheme Prologue for romeo and juliet
Prologue for romeo and juliet Spenserian sonnet
Spenserian sonnet Vlsm design
Vlsm design Employment guarantee scheme 1972, *
Employment guarantee scheme 1972, * Pickety fence poem
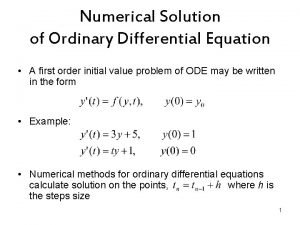
Pickety fence poem Modified euler's method formula
Modified euler's method formula Personality test color wheel
Personality test color wheel Aqa english language paper 2 rail disasters model answers
Aqa english language paper 2 rail disasters model answers Nsw procure it
Nsw procure it Ehtp scheme
Ehtp scheme Gell mann nishijima
Gell mann nishijima 2017 sats mark scheme
2017 sats mark scheme Grandmother's song nellie wong
Grandmother's song nellie wong Internal rhyme
Internal rhyme Chromatic color scheme
Chromatic color scheme And as he stands in victory sin's curse
And as he stands in victory sin's curse Glen davies
Glen davies Exact organization scheme
Exact organization scheme Ehew manual
Ehew manual Creation of backward and forward linkages
Creation of backward and forward linkages J384/02 mark scheme
J384/02 mark scheme Aqa english literature paper 1 inspector calls
Aqa english literature paper 1 inspector calls Words that rhyme with relax
Words that rhyme with relax Prmbf
Prmbf Ac3.1 examine information for validity
Ac3.1 examine information for validity The incentive scheme that rules sumo is intricate
The incentive scheme that rules sumo is intricate Pharmacy quality scheme
Pharmacy quality scheme Ams(https://mdm.gujarat.gov.in
Ams(https://mdm.gujarat.gov.in London 1802 rhyme scheme
London 1802 rhyme scheme Aswas plus
Aswas plus How to prepare scheme of work
How to prepare scheme of work Single user contiguous scheme
Single user contiguous scheme Shall i compare thee to a summer's day romeo and juliet
Shall i compare thee to a summer's day romeo and juliet Andy warhol complementary colors
Andy warhol complementary colors Positive list scheme
Positive list scheme In this scheme cpu execution waits while i/o proceeds.
In this scheme cpu execution waits while i/o proceeds.