Dimensional Analysis The Dimensional Analysis method A way

Dimensional Analysis

The Dimensional Analysis method • A way to solve math problems in science • Used to convert km to miles, m to km, mol to g, g to mol, etc. • To use this we need: 1) desired quantity, 2) given quantity, 3) conversion factors • Conversion factors are valid relationships or equities expressed as a fraction E. g. for 1 km=0. 6 miles the conversion factor is Q. write conversion factors for 1 foot =12 inches Q. what conversion factors can you think of that involve meters?

Conversion factors for 1 ft = 12 in There almost an infinite number of conversion factors that include meters:

Conversion factors • We have looked at conversion factors that are always true. There are conversion factors that are only true for specific questions • E. g. A recipe calls for 2 eggs, 1 cup of flour and 0. 5 cups of sugar • We can use these conversion factors • Q - the chemical equation between H 2 and O 2 involves 2 H 2 molecules combining with 1 O 2 molecule to make 2 H 2 O molecules. Write all possible conversion factors

The steps to follow Now we are ready to solve problems using the dimensional analysis method. The steps involved are: 1. Write down the desired quantity/units 2. Equate the desired quantity to given quantity 3. Determine what conversion factors you can use (both universal and question specific) 4. Multiply given quantity by the appropriate conversion factors to eliminate units you don’t want and leave units you do want 5. Complete the math

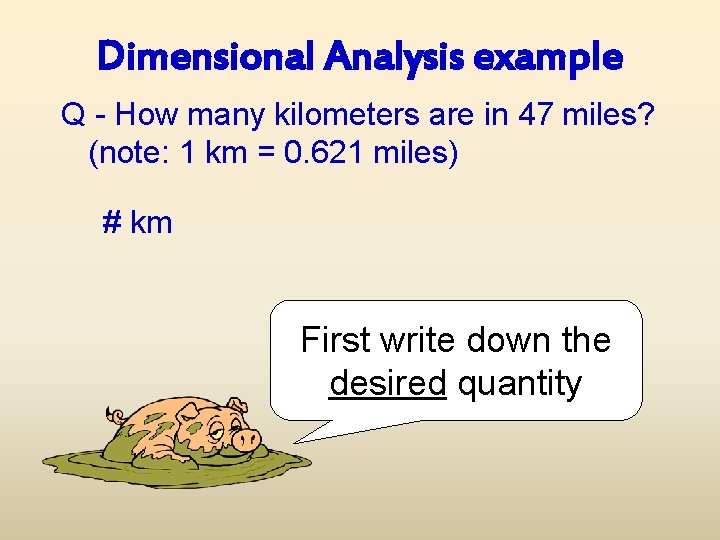
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km First write down the desired quantity

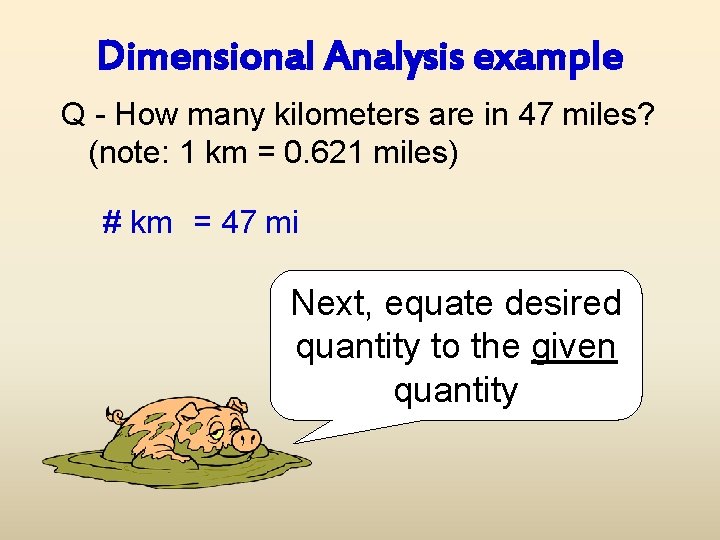
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi Next, equate desired quantity to the given quantity

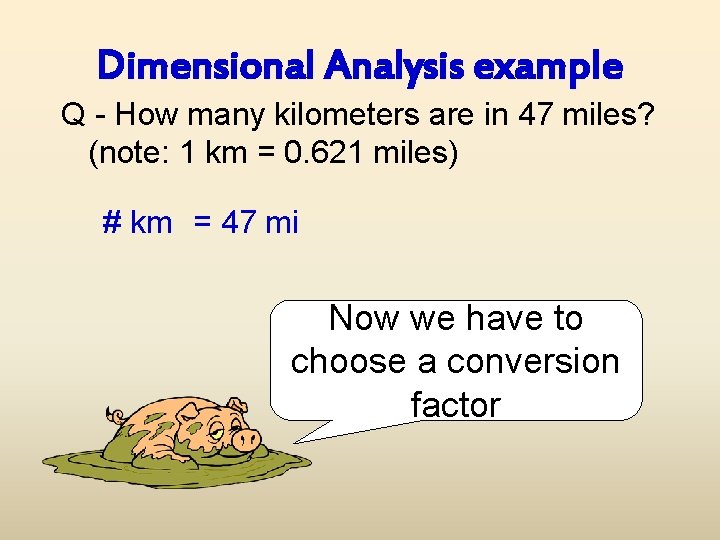
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi Now we have to choose a conversion factor

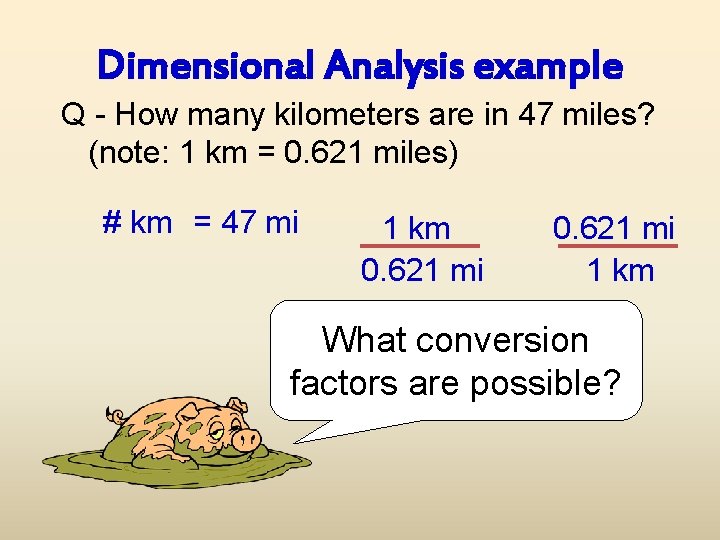
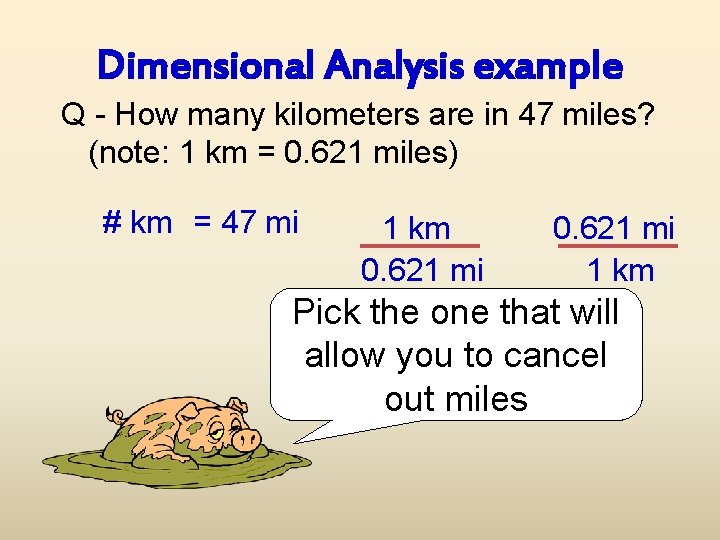
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi 1 km 0. 621 mi 1 km What conversion factors are possible?

Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi 1 km 0. 621 mi 1 km Pick the one that will allow you to cancel out miles

Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi 1 km 0. 621 mi 1 km Pick the one that will allow you to cancel out miles

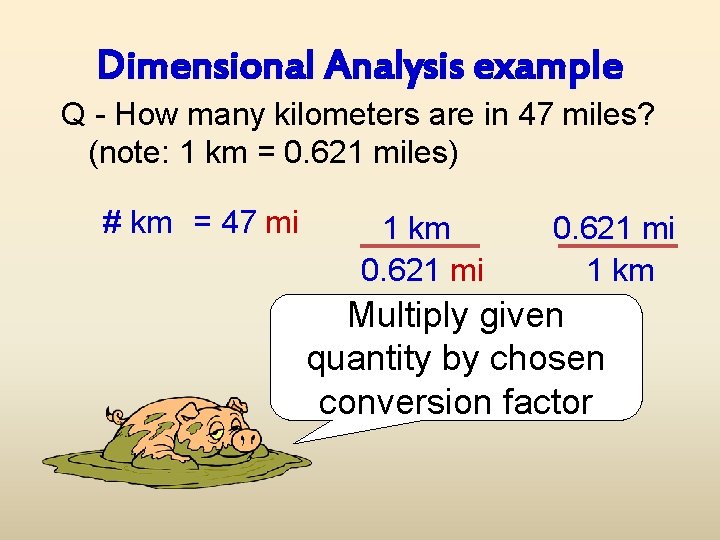
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi 1 km 0. 621 mi 1 km Multiply given quantity by chosen conversion factor

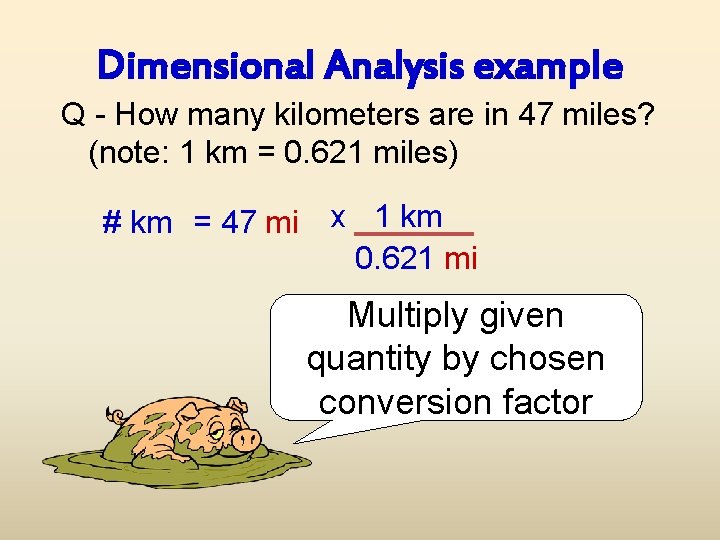
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi x 1 km 0. 621 mi Multiply given quantity by chosen conversion factor

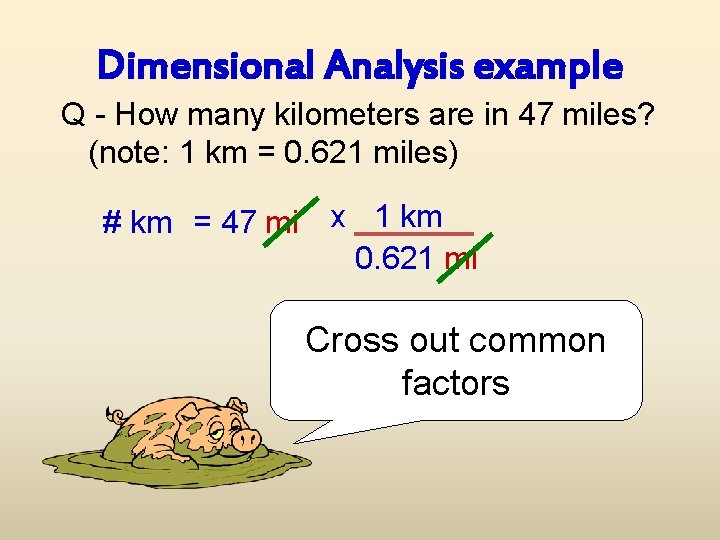
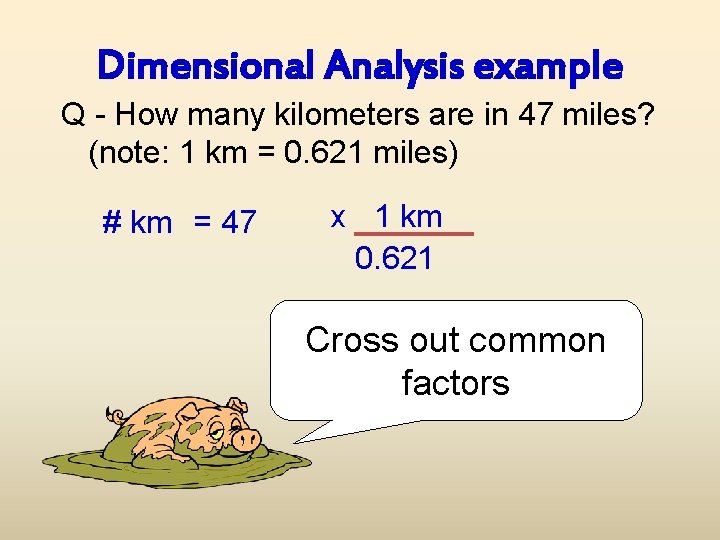
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 mi x 1 km 0. 621 mi Cross out common factors

Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 x 1 km 0. 621 Cross out common factors

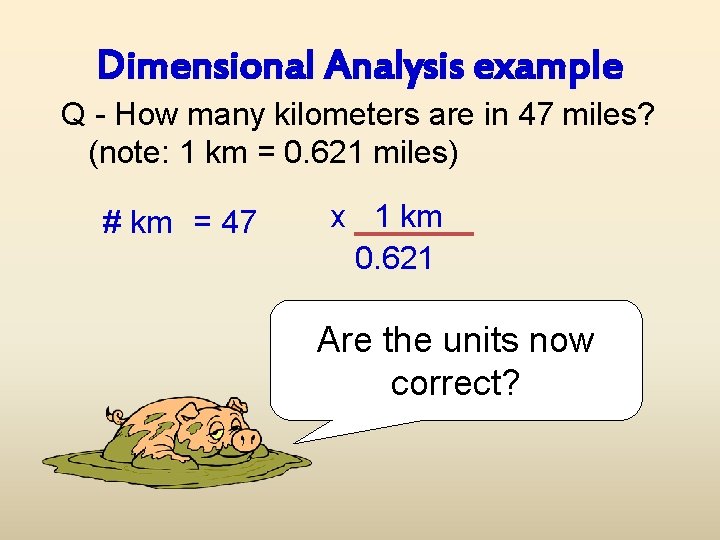
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 x 1 km 0. 621 Are the units now correct?

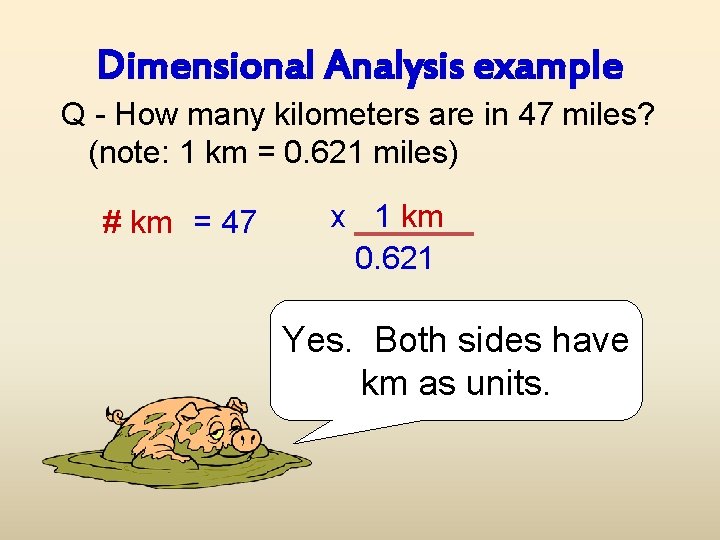
Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 x 1 km 0. 621 Yes. Both sides have km as units.

Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 x 1 km 0. 621 Yes. Both sides have km as units.

Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 x 1 km 0. 621 = 75. 7 km Now finish the math.

Dimensional Analysis example Q - How many kilometers are in 47 miles? (note: 1 km = 0. 621 miles) # km = 47 x 1 km 0. 621 = 75. 7 km The final answer is 75. 7 km

Summary The previous problem was not that hard In other words, you probably could have done it faster using a different method However, for harder problems the factor label method is easiest

More examples 1. You want to buy 100 U. S. dollars. If the exchange rate is 1 Can$ = 0. 65 US$, how much will it cost? # Can$ = 100 US$ x 1 Can$ = 153. 85 Can$ 0. 65 US$ 2. One mole of a gas has a volume of 22. 4 L. How many L will 300 grams of CO 2 occupy? (hint: the molar mass of CO 2 is 44. 01 ____ g/mol). # L CO 2 = 300 g CO 2 x 1 mol CO 2 x 22. 4 L CO 2 = 152. 7 L 44. 01 g CO 2 1 mol CO 2

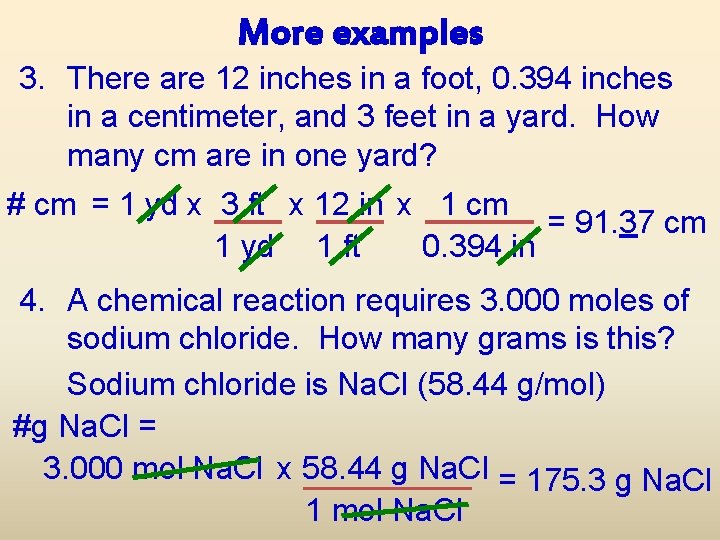
More examples 3. There are 12 inches in a foot, 0. 394 inches in a centimeter, and 3 feet in a yard. How many cm are in one yard? # cm = 1 yd x 3 ft x 12 in x 1 cm = 91. 37 cm 1 yd 1 ft 0. 394 in 4. A chemical reaction requires 3. 000 moles of sodium chloride. How many grams is this? Sodium chloride is Na. Cl (58. 44 g/mol) #g Na. Cl = 3. 000 mol Na. Cl x 58. 44 g Na. Cl = 175. 3 g Na. Cl 1 mol Na. Cl

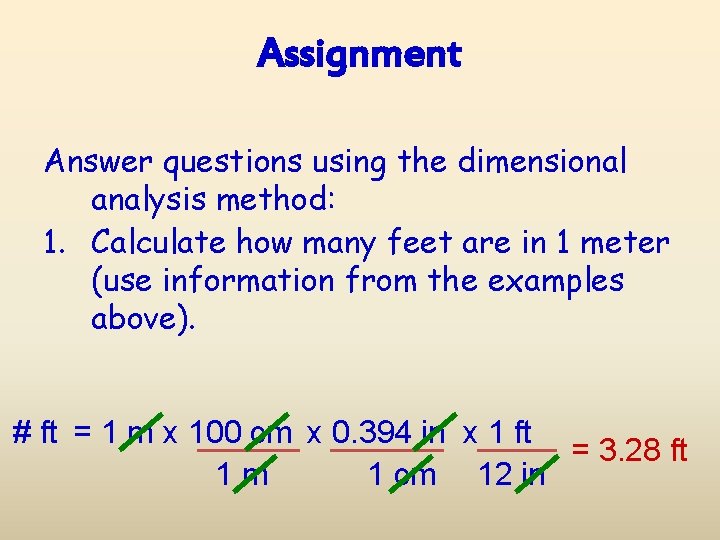
Assignment Answer questions using the dimensional analysis method: 1. Calculate how many feet are in 1 meter (use information from the examples above). # ft = 1 m x 100 cm x 0. 394 in x 1 ft = 3. 28 ft 1 m 1 cm 12 in

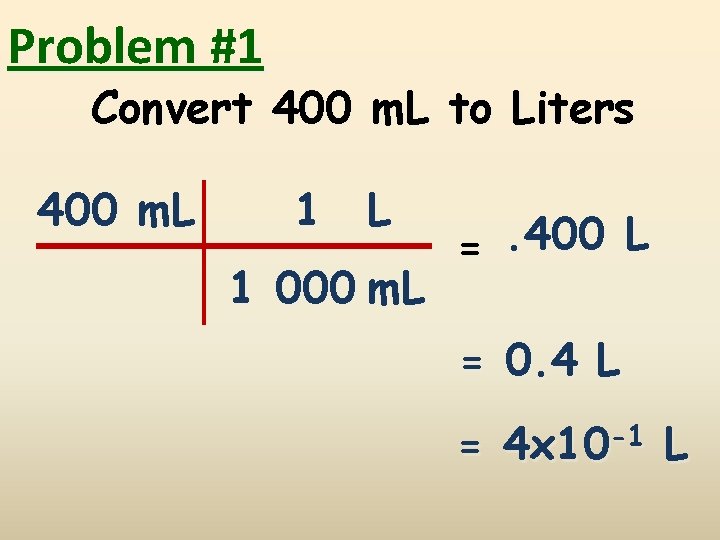
Problem #1 Convert 400 m. L to Liters 400 m. L 1 000 m. L =. 400 L = 0. 4 L = 4 x 10 -1 L

Problem #2 Convert 10 meters to mm 10 m 1 000 mm 1 m = 10 000 mm = 1 x 104 mm

Problem #3 Convert 73 grams to kg 73 g 1 kg 1 000 g = 0. 073 kg = 7. 3 x 10 -2 kg

Problem #4 Convert 0. 02 kilometers to m 0. 02 km 1 000 m 1 km = 20 m = 2 x 101 m

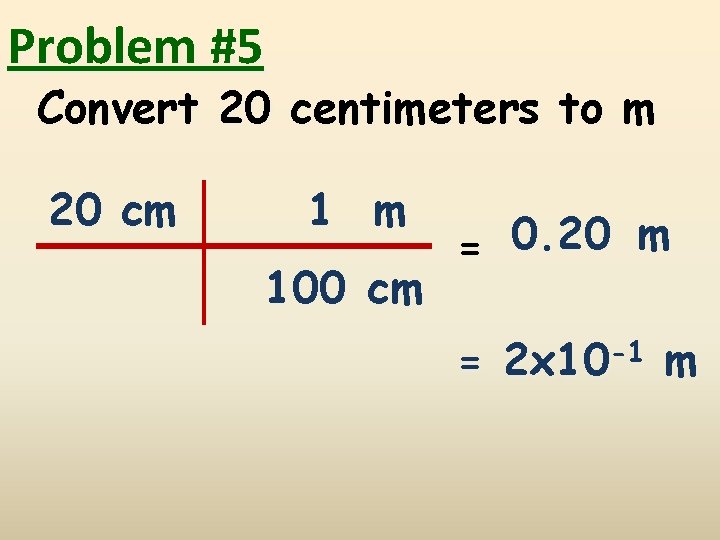
Problem #5 Convert 20 centimeters to m 20 cm 1 m 100 cm = 0. 20 m = 2 x 10 -1 m

Problem #6 Convert 450 milliliters to d. L 450 m. L 1 d. L 100 m. L = 4. 5 d. L

Problem #7 Convert 10 kilograms to grams 10 kg 1 000 g 1 kg = 10 000 g = 1 x 104 g

Problem #8 Convert 935 mg to cg 935 mg 1 cg 10 mg = 93. 5 cg = 9. 35 x 101 cg

Problem #9 Convert 5. 2 kg to mg 5. 2 kg 1 000 mg 1 kg = mg = 5 200 000 mg = 5. 2 x 106 mg

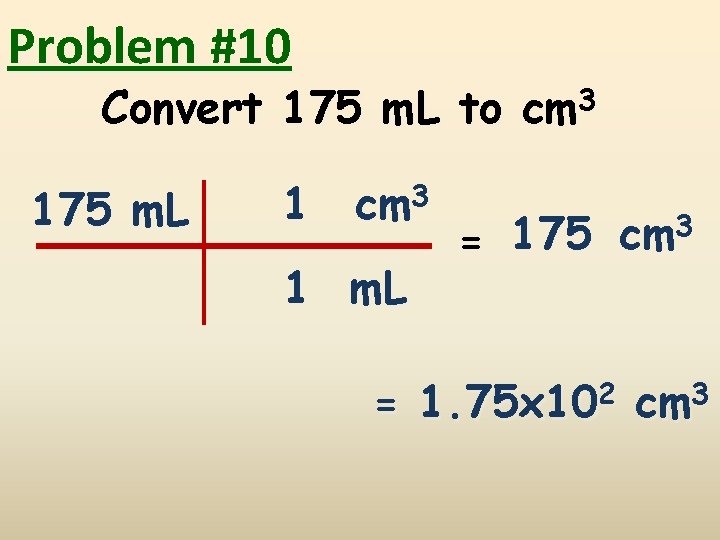
Problem #10 Convert 175 m. L to cm 3 175 m. L 1 cm 3 1 m. L 3 175 cm = = 1. 75 x 102 cm 3