Differential Equations SecondOrder Linear DEs Variation of Parameters













- Slides: 36

Differential Equations Second-Order Linear DEs Variation of Parameters Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

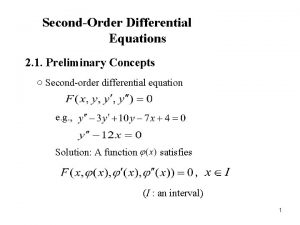
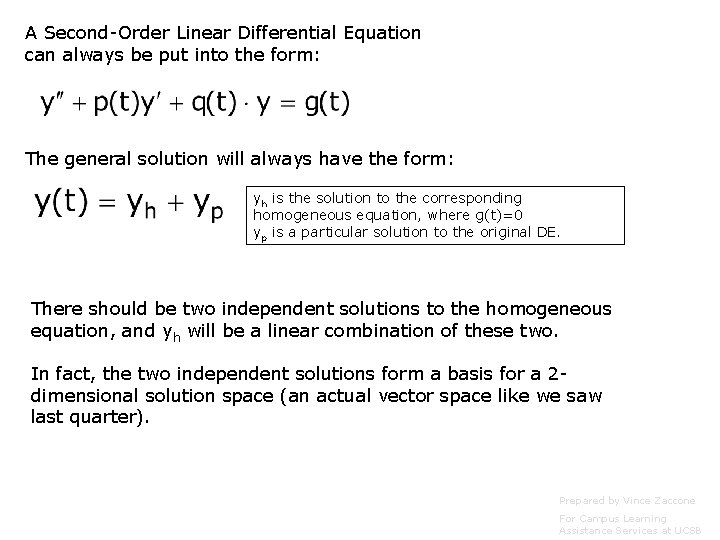
A Second-Order Linear Differential Equation can always be put into the form: The general solution will always have the form: yh is the solution to the corresponding homogeneous equation, where g(t)=0 yp is a particular solution to the original DE. There should be two independent solutions to the homogeneous equation, and yh will be a linear combination of these two. In fact, the two independent solutions form a basis for a 2 dimensional solution space (an actual vector space like we saw last quarter). Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters To solve a non-homogeneous equation, find a solution to the corresponding homogeneous equation first, then find a particular solution. The method of VARIATION of PARAMETERS will modify the homogeneous solution to find the particular solution. Below is the method applied to a general equation, and later will be a few specific examples. Above is the general linear 2 nd order D. E. , below is the associated homogeneous equation Find a fundamental solution set for this homogeneous equation (we know how to do this if p(t) and q(t) are constants). Call these solutions y 1(t) and y 2(t). So the solution to the homogeneous equation is: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

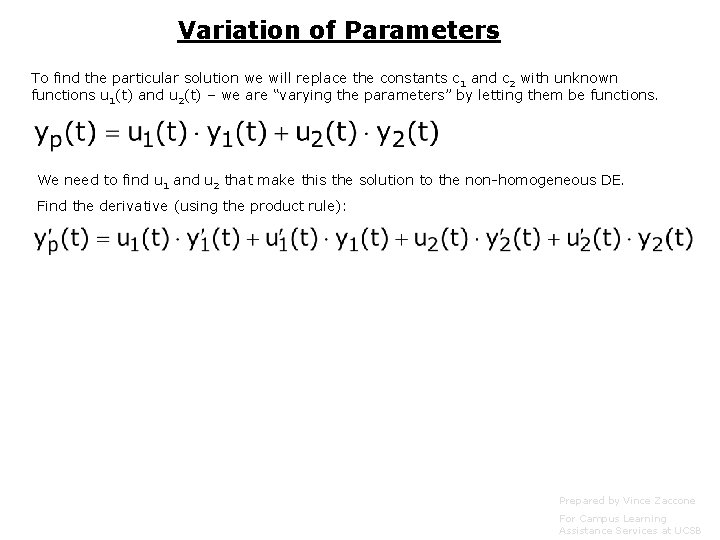
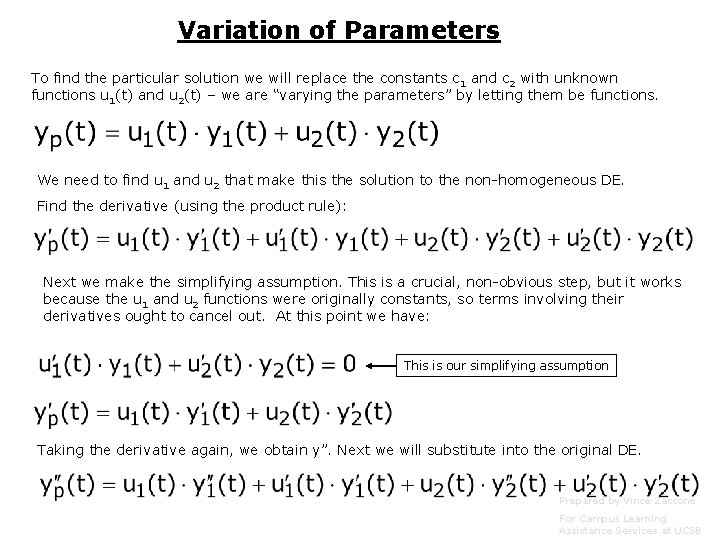
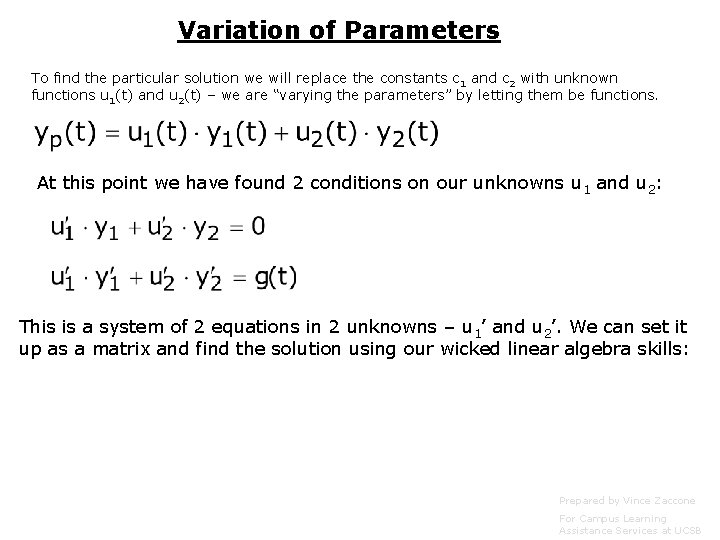
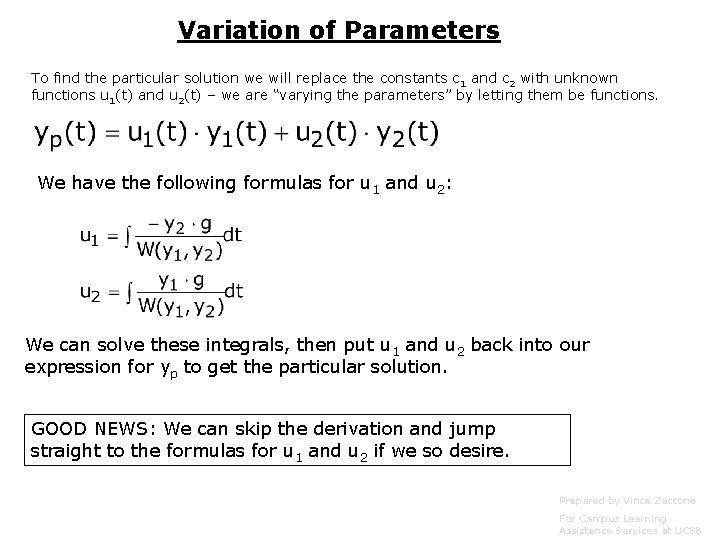
Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

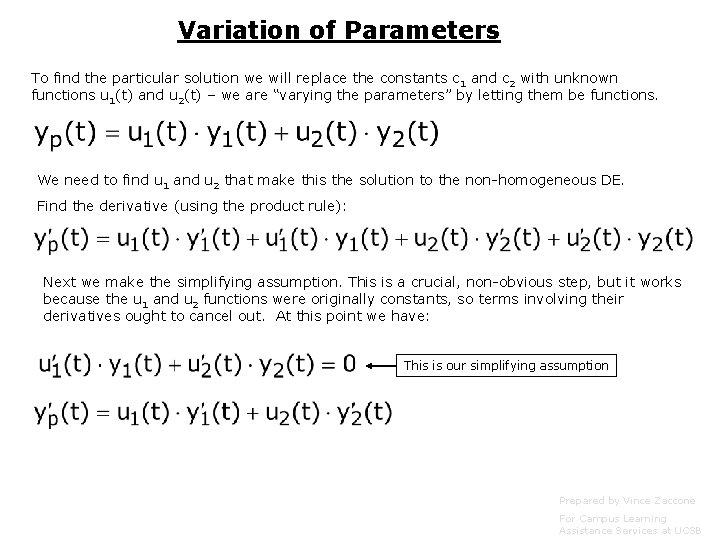
Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. We need to find u 1 and u 2 that make this the solution to the non-homogeneous DE. Find the derivative (using the product rule): Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. We need to find u 1 and u 2 that make this the solution to the non-homogeneous DE. Find the derivative (using the product rule): Next we make the simplifying assumption. This is a crucial, non-obvious step, but it works because the u 1 and u 2 functions were originally constants, so terms involving their derivatives ought to cancel out. At this point we have: This is our simplifying assumption Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. We need to find u 1 and u 2 that make this the solution to the non-homogeneous DE. Find the derivative (using the product rule): Next we make the simplifying assumption. This is a crucial, non-obvious step, but it works because the u 1 and u 2 functions were originally constants, so terms involving their derivatives ought to cancel out. At this point we have: This is our simplifying assumption Taking the derivative again, we obtain y”. Next we will substitute into the original DE. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

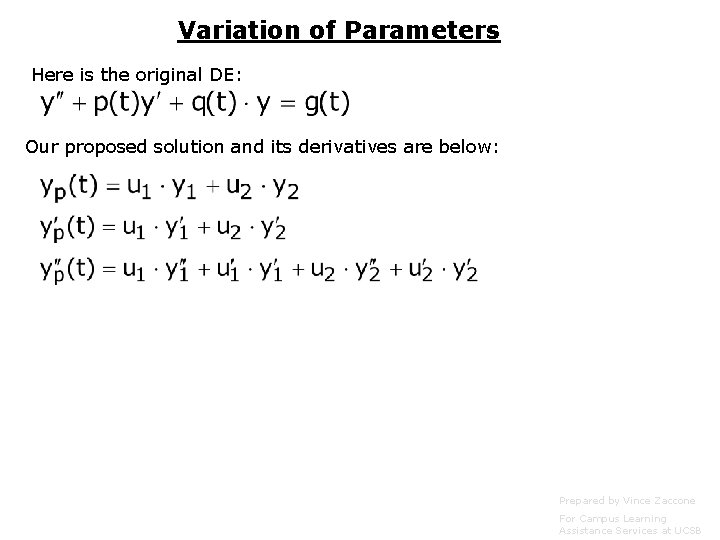
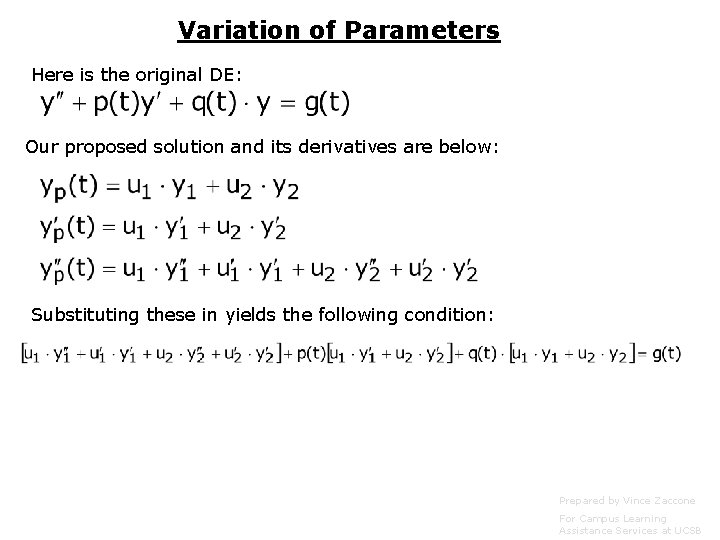
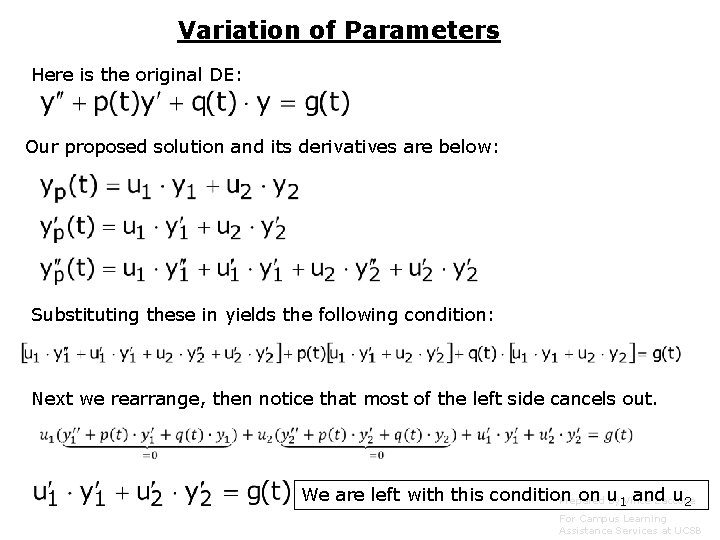
Variation of Parameters Here is the original DE: Our proposed solution and its derivatives are below: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters Here is the original DE: Our proposed solution and its derivatives are below: Substituting these in yields the following condition: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters Here is the original DE: Our proposed solution and its derivatives are below: Substituting these in yields the following condition: Next we rearrange, then notice that most of the left side cancels out. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters Here is the original DE: Our proposed solution and its derivatives are below: Substituting these in yields the following condition: Next we rearrange, then notice that most of the left side cancels out. We are left with this condition on u and u 2 Prepared by 1 Vince Zaccone For Campus Learning Assistance Services at UCSB

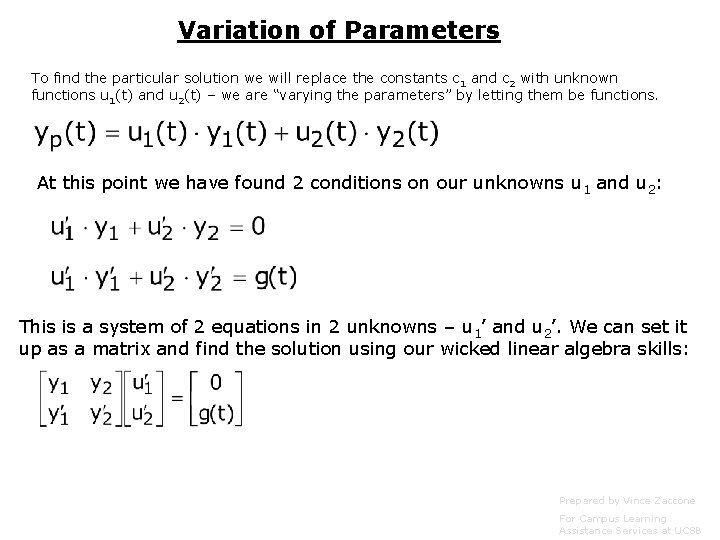
Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. At this point we have found 2 conditions on our unknowns u 1 and u 2: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

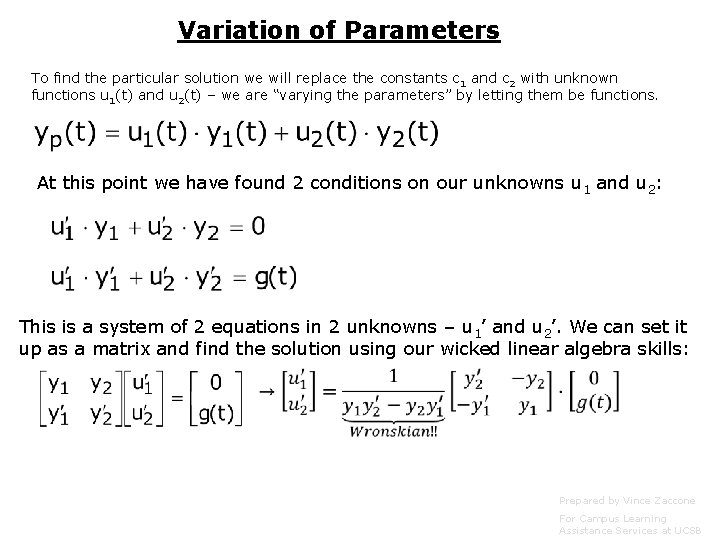
Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. At this point we have found 2 conditions on our unknowns u 1 and u 2: This is a system of 2 equations in 2 unknowns – u 1’ and u 2’. We can set it up as a matrix and find the solution using our wicked linear algebra skills: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. At this point we have found 2 conditions on our unknowns u 1 and u 2: This is a system of 2 equations in 2 unknowns – u 1’ and u 2’. We can set it up as a matrix and find the solution using our wicked linear algebra skills: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

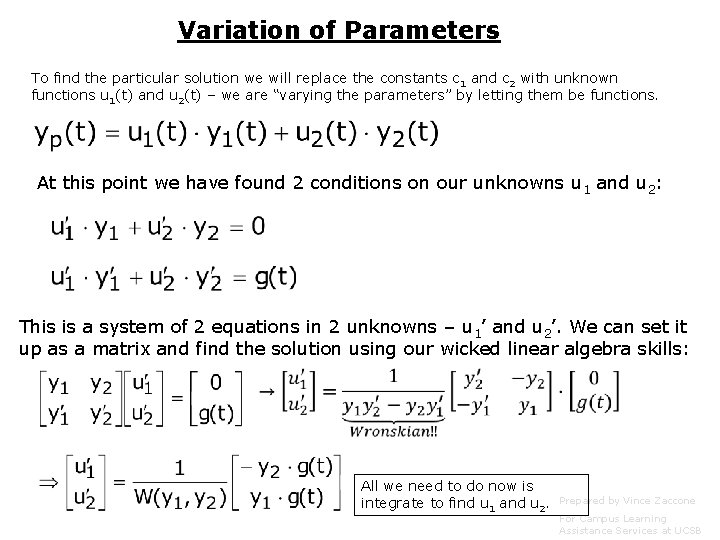
Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. At this point we have found 2 conditions on our unknowns u 1 and u 2: This is a system of 2 equations in 2 unknowns – u 1’ and u 2’. We can set it up as a matrix and find the solution using our wicked linear algebra skills: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. At this point we have found 2 conditions on our unknowns u 1 and u 2: This is a system of 2 equations in 2 unknowns – u 1’ and u 2’. We can set it up as a matrix and find the solution using our wicked linear algebra skills: All we need to do now is integrate to find u 1 and u 2. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Variation of Parameters To find the particular solution we will replace the constants c 1 and c 2 with unknown functions u 1(t) and u 2(t) – we are “varying the parameters” by letting them be functions. We have the following formulas for u 1 and u 2: We can solve these integrals, then put u 1 and u 2 back into our expression for yp to get the particular solution. GOOD NEWS: We can skip the derivation and jump straight to the formulas for u 1 and u 2 if we so desire. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

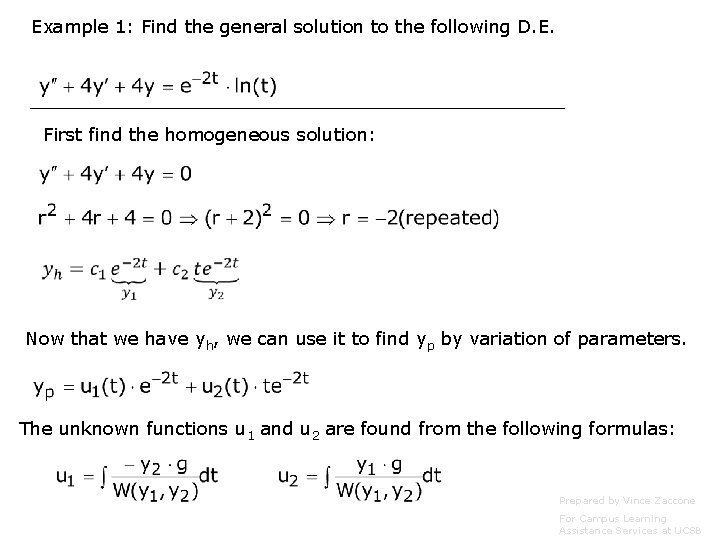
Example 1: Find the general solution to the following D. E. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 1: Find the general solution to the following D. E. First find the homogeneous solution: Now that we have yh, we can use it to find yp by variation of parameters. The unknown functions u 1 and u 2 are found from the following formulas: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

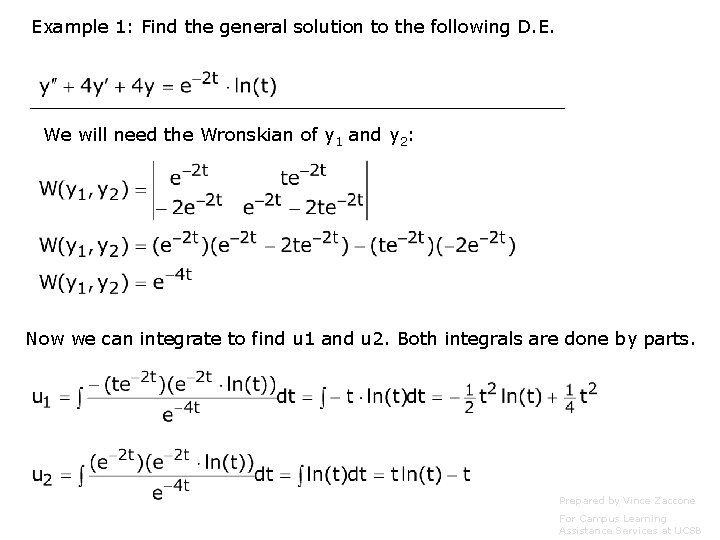
Example 1: Find the general solution to the following D. E. We will need the Wronskian of y 1 and y 2: Now we can integrate to find u 1 and u 2. Both integrals are done by parts. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 1: Find the general solution to the following D. E. Putting these functions into our expression for y p, we get: Finally we can write down the general solution by adding y h and yp. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 2: Find the general solution to the following D. E. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 2: Find the general solution to the following D. E. First find the homogeneous solution: Find the particular solution by assuming it has the form: The unknown functions u 1 and u 2 are found by integrating: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 2: Find the general solution to the following D. E. We need to find the Wronskian of y 1 and y 2: Now we can find u 1 and u 2 : Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

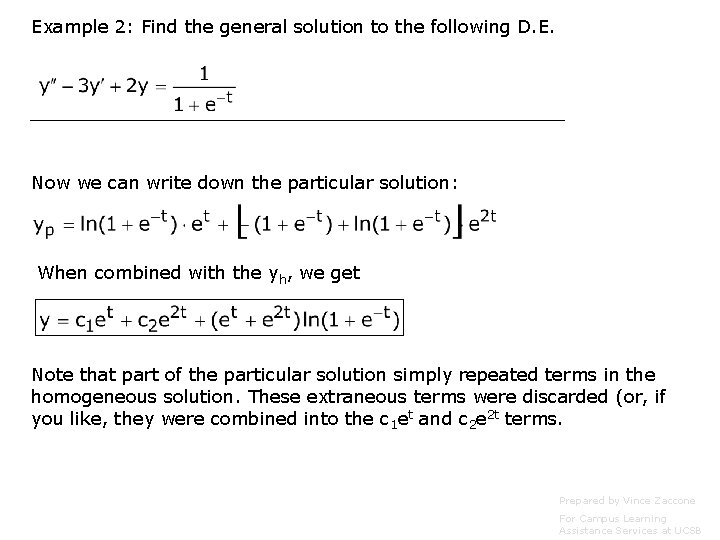
Example 2: Find the general solution to the following D. E. Now we can write down the particular solution: When combined with the yh, we get Note that part of the particular solution simply repeated terms in the homogeneous solution. These extraneous terms were discarded (or, if you like, they were combined into the c 1 et and c 2 e 2 t terms. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

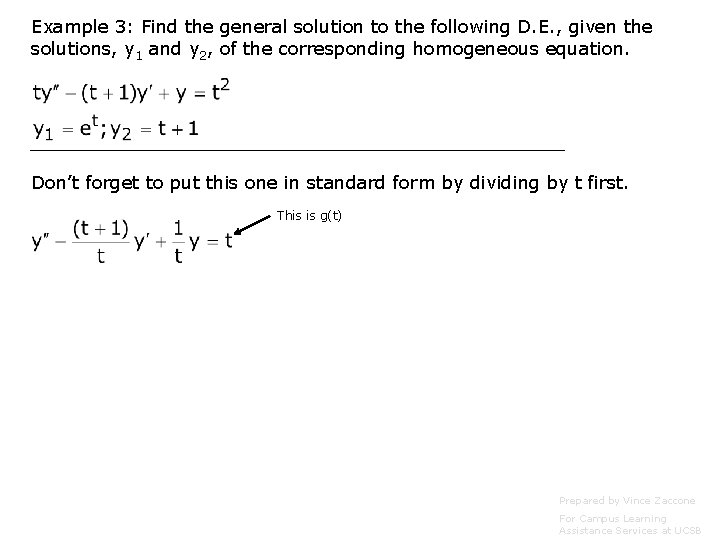
Example 3: Find the general solution to the following D. E. , given the solutions, y 1 and y 2, of the corresponding homogeneous equation. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 3: Find the general solution to the following D. E. , given the solutions, y 1 and y 2, of the corresponding homogeneous equation. Don’t forget to put this one in standard form by dividing by t first. This is g(t) Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

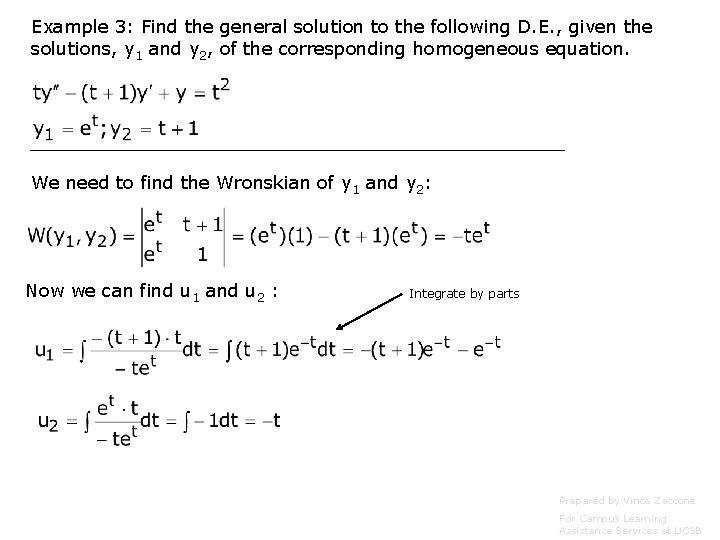
Example 3: Find the general solution to the following D. E. , given the solutions, y 1 and y 2, of the corresponding homogeneous equation. Don’t forget to put this one in standard form by dividing by t first. This is g(t) Find the particular solution by assuming it has the form: The unknown functions u 1 and u 2 are found by integrating: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 3: Find the general solution to the following D. E. , given the solutions, y 1 and y 2, of the corresponding homogeneous equation. We need to find the Wronskian of y 1 and y 2: Now we can find u 1 and u 2 : Integrate by parts Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

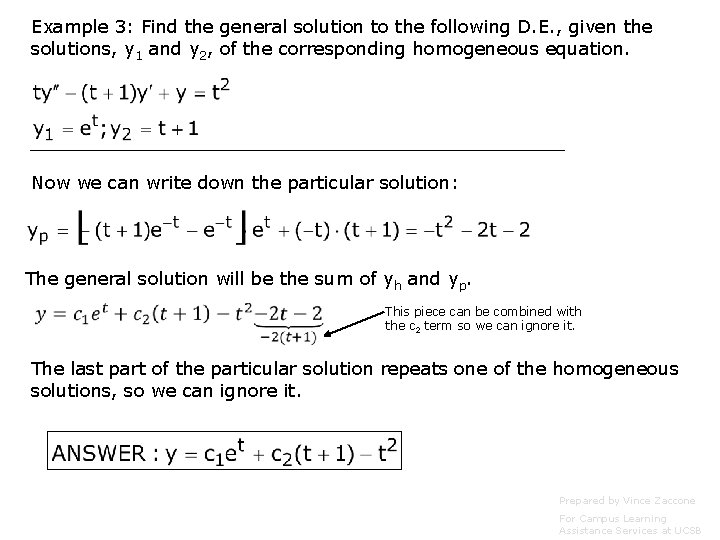
Example 3: Find the general solution to the following D. E. , given the solutions, y 1 and y 2, of the corresponding homogeneous equation. Now we can write down the particular solution: The general solution will be the sum of yh and yp. This piece can be combined with the c 2 term so we can ignore it. The last part of the particular solution repeats one of the homogeneous solutions, so we can ignore it. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

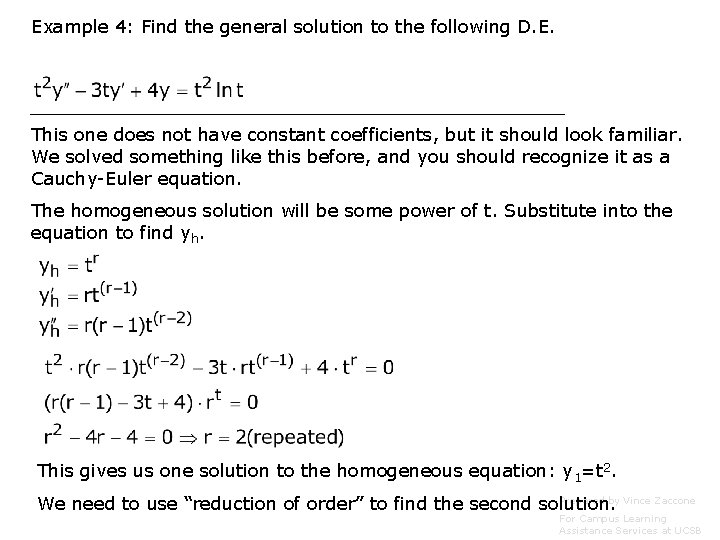
Example 4: Find the general solution to the following D. E. Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

Example 4: Find the general solution to the following D. E. This one does not have constant coefficients, but it should look familiar. We solved something like this before, and you should recognize it as a Cauchy-Euler equation. The homogeneous solution will be some power of t. Substitute into the equation to find yh. This gives us one solution to the homogeneous equation: y 1=t 2. Prepared by Vince Zaccone We need to use “reduction of order” to find the second solution. For Campus Learning Assistance Services at UCSB

Example 4: Find the general solution to the following D. E. “Reduction of Order” modifies our existing solution by multiplying by an unknown function of t. This should yield a 2 nd independent solution to our DE. Find derivatives and plug in to find a condition for v(t). This simplifies to: We need to solve this equation for v(t). Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

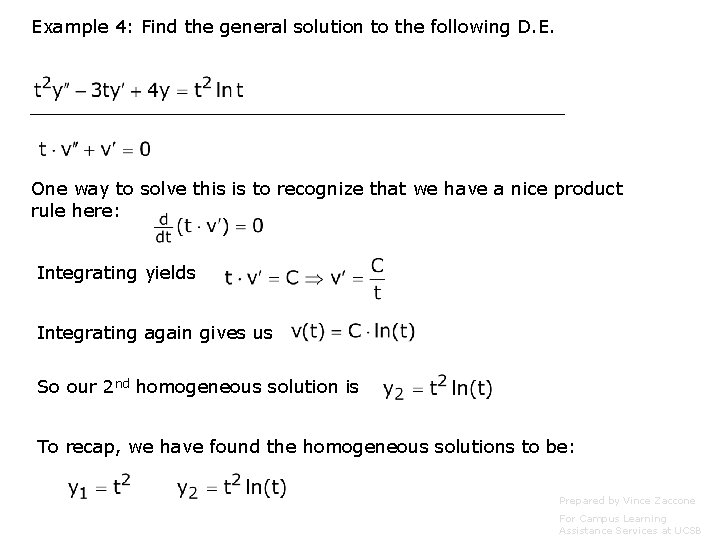
Example 4: Find the general solution to the following D. E. One way to solve this is to recognize that we have a nice product rule here: Integrating yields Integrating again gives us So our 2 nd homogeneous solution is To recap, we have found the homogeneous solutions to be: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

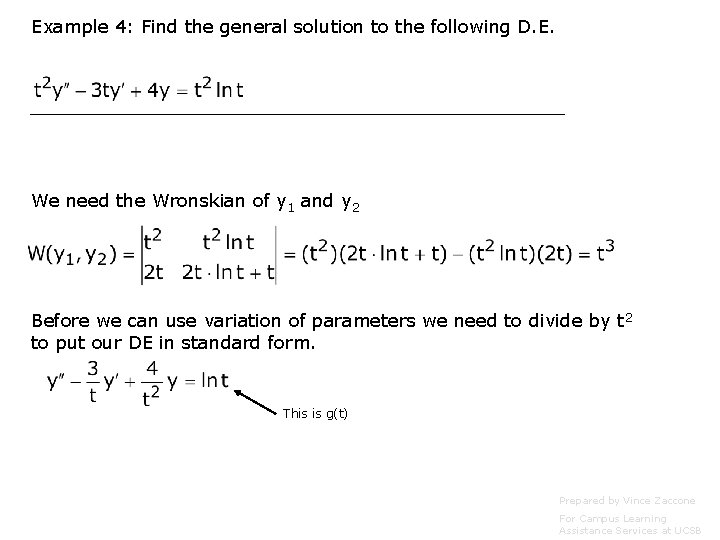
Example 4: Find the general solution to the following D. E. We need the Wronskian of y 1 and y 2 Before we can use variation of parameters we need to divide by t 2 to put our DE in standard form. This is g(t) Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB

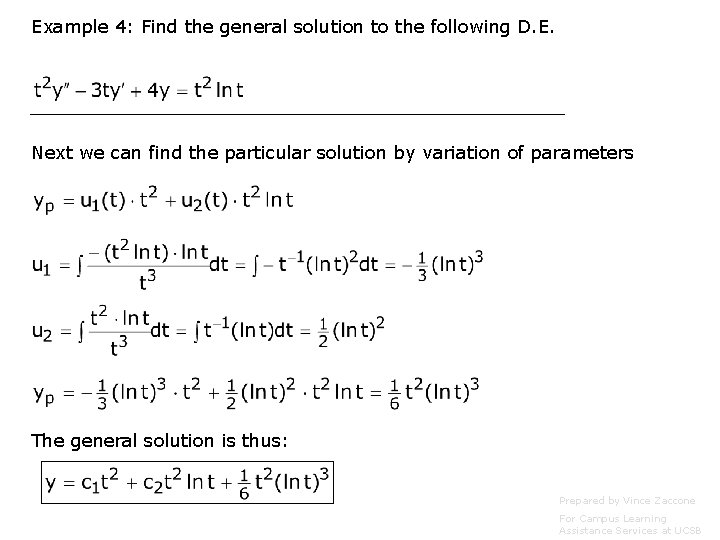
Example 4: Find the general solution to the following D. E. Next we can find the particular solution by variation of parameters The general solution is thus: Prepared by Vince Zaccone For Campus Learning Assistance Services at UCSB
 Differential equations variation of parameters
Differential equations variation of parameters Method of characteristics pde second order
Method of characteristics pde second order L
L Introduction to linear algebra strang
Introduction to linear algebra strang Higher order linear differential equations
Higher order linear differential equations Variation of parameters
Variation of parameters Method of variation of parameters
Method of variation of parameters Des des des
Des des des Constant of variation
Constant of variation Examples of direct variation graphs
Examples of direct variation graphs Prediction interval formula
Prediction interval formula Persamaan linear simultan
Persamaan linear simultan Difference between linear and nonlinear
Difference between linear and nonlinear Eliminasi gauss naif
Eliminasi gauss naif Constant of proportionality
Constant of proportionality Is a direct variation a linear function
Is a direct variation a linear function Differential equations projects
Differential equations projects Bernoulli differential equation ppt
Bernoulli differential equation ppt Classification of pde
Classification of pde Cengage differential equations
Cengage differential equations Non linear ode
Non linear ode Euler midpoint method
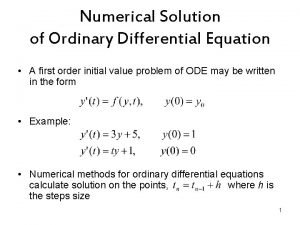
Euler midpoint method Differential equations calculus
Differential equations calculus Differential equation examples
Differential equation examples Separation of variables
Separation of variables What is a first order equation
What is a first order equation Integrating factor of differential equation
Integrating factor of differential equation Separation of variables differential equations
Separation of variables differential equations Natural solution
Natural solution How to solve second order differential equations
How to solve second order differential equations Solving 1st order differential equations
Solving 1st order differential equations Classification of differential equations
Classification of differential equations Traffic flow differential equations
Traffic flow differential equations Midpoint method formula
Midpoint method formula Modified euler formula
Modified euler formula First-order linear equations
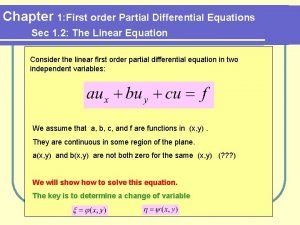
First-order linear equations First order linear equation
First order linear equation