Continuity Essential discontinuity Any other type of the






![Example No maximum or minimum value on the domain. However, on [-3, 3], it Example No maximum or minimum value on the domain. However, on [-3, 3], it](https://slidetodoc.com/presentation_image/357ad410c99c8279c17fa7ab60f26e62/image-22.jpg)
![Conclusions about hypotheses l l Conclude that hypothesis that interval be closed, [a, b], Conclusions about hypotheses l l Conclude that hypothesis that interval be closed, [a, b],](https://slidetodoc.com/presentation_image/357ad410c99c8279c17fa7ab60f26e62/image-23.jpg)















- Slides: 63

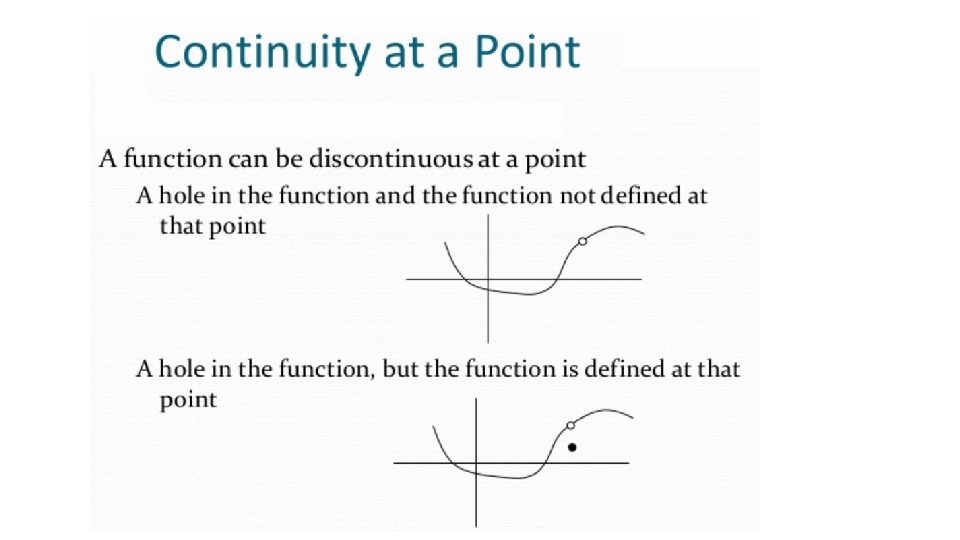
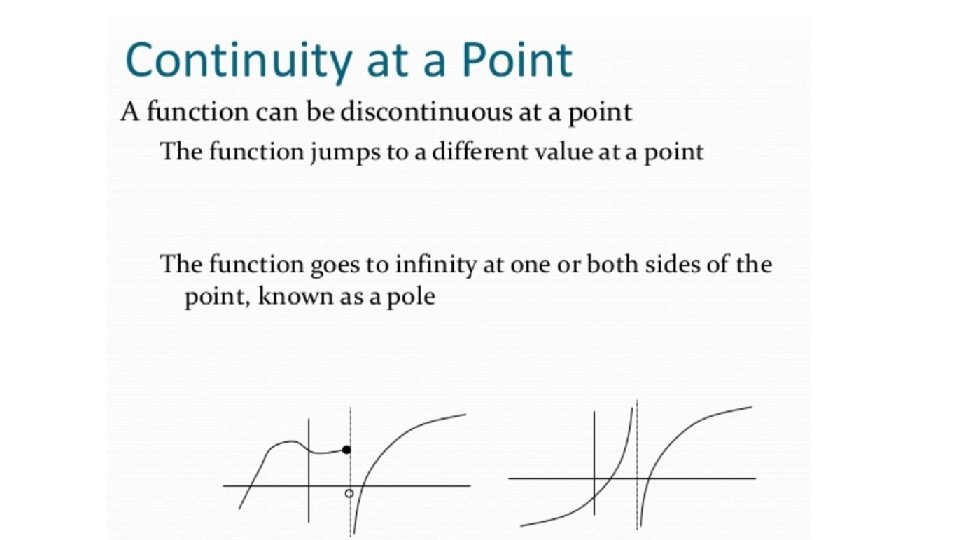
Continuity











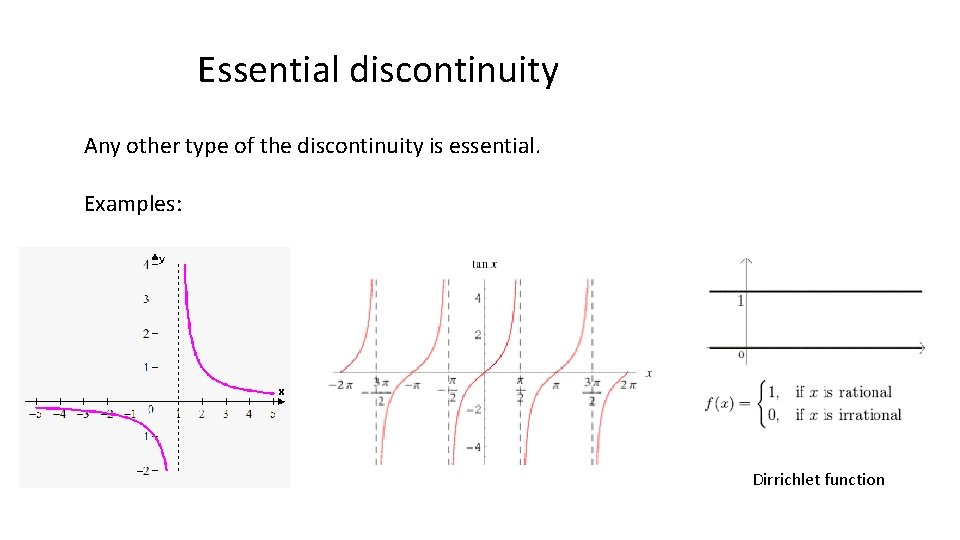
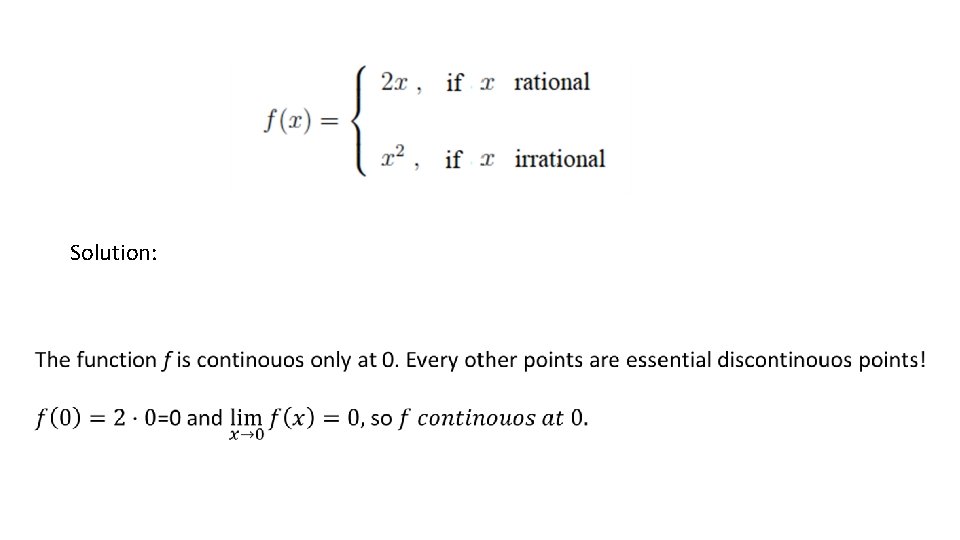
Essential discontinuity Any other type of the discontinuity is essential. Examples: Dirrichlet function

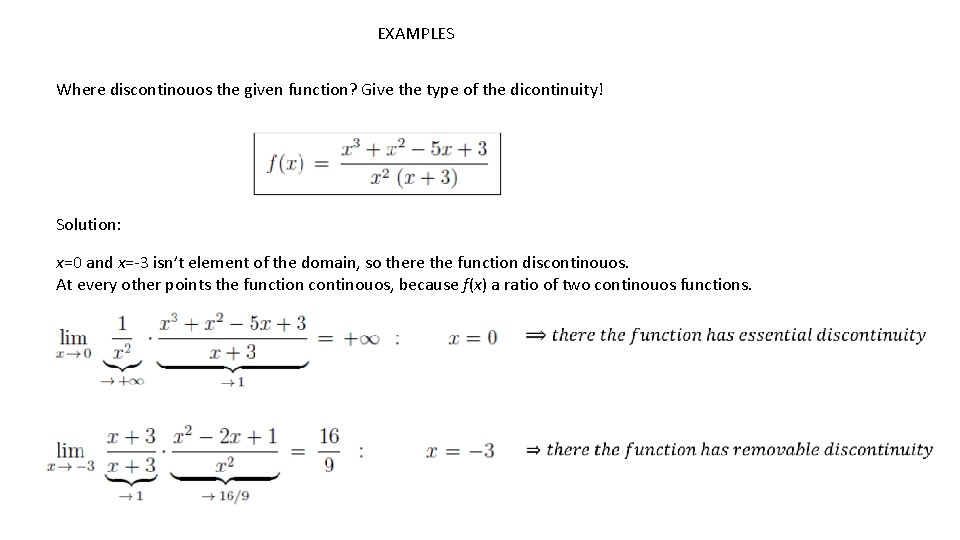
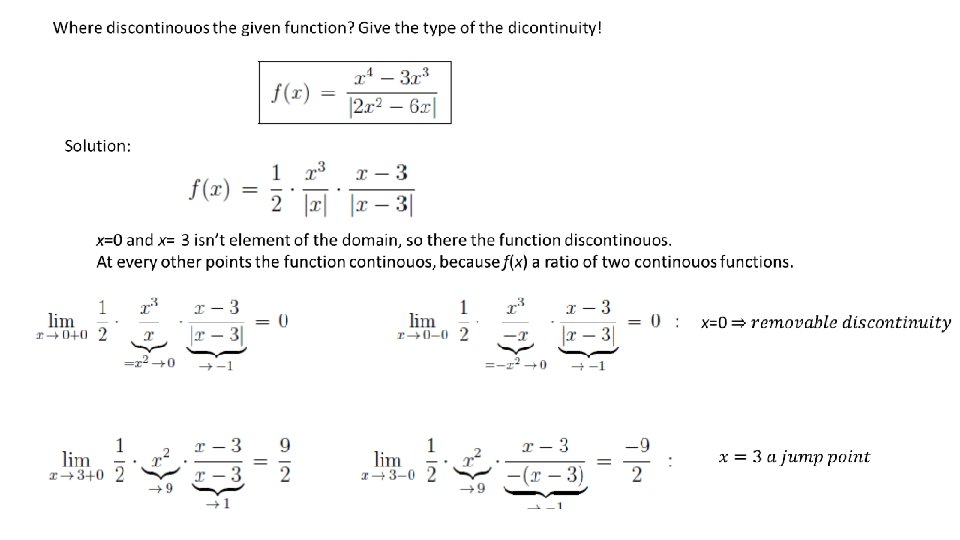
EXAMPLES Where discontinouos the given function? Give the type of the dicontinuity! Solution: x=0 and x=-3 isn’t element of the domain, so there the function discontinouos. At every other points the function continouos, because f(x) a ratio of two continouos functions.


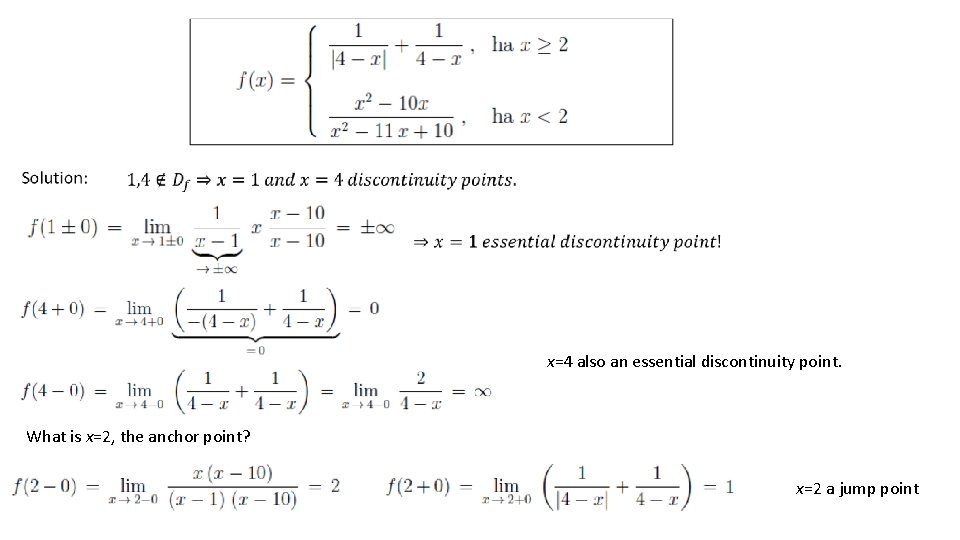
x=4 also an essential discontinuity point. What is x=2, the anchor point? x=2 a jump point

Solution:

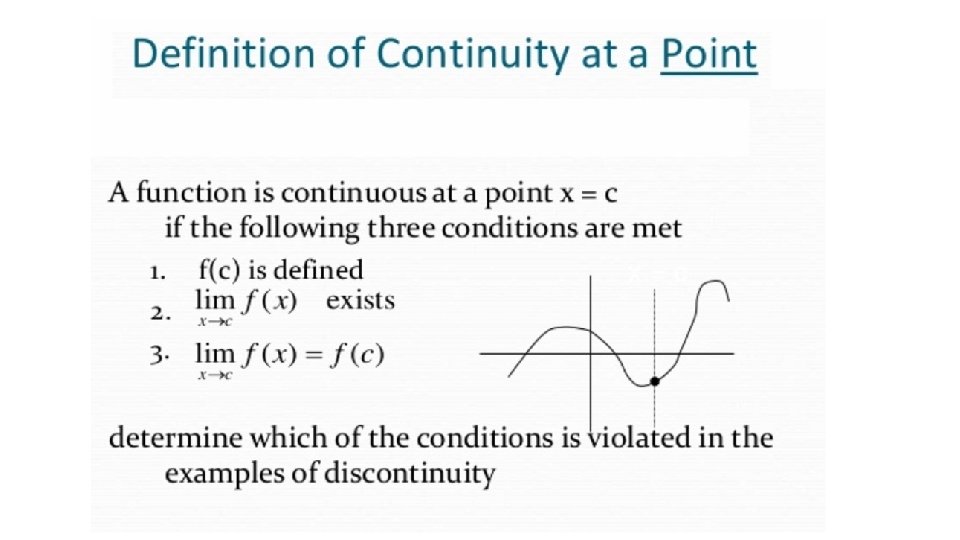
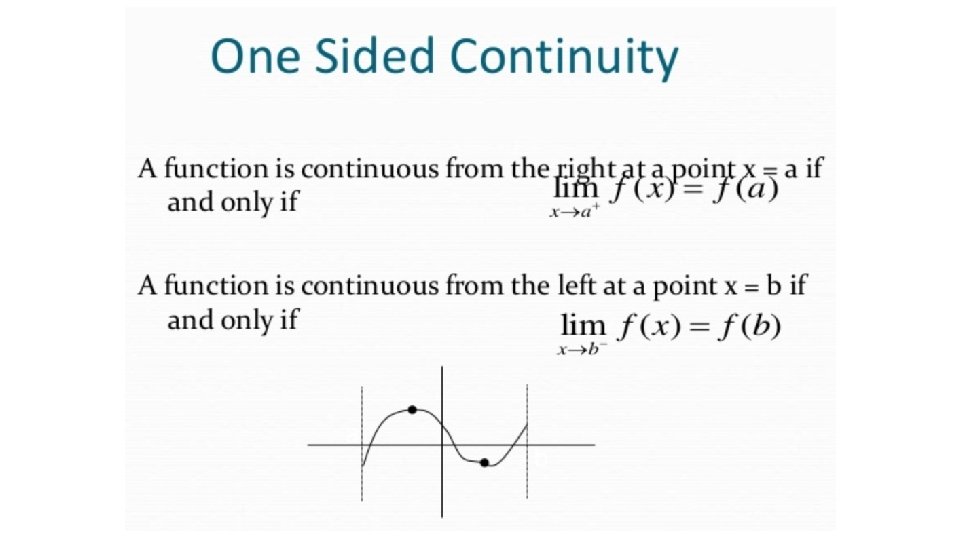
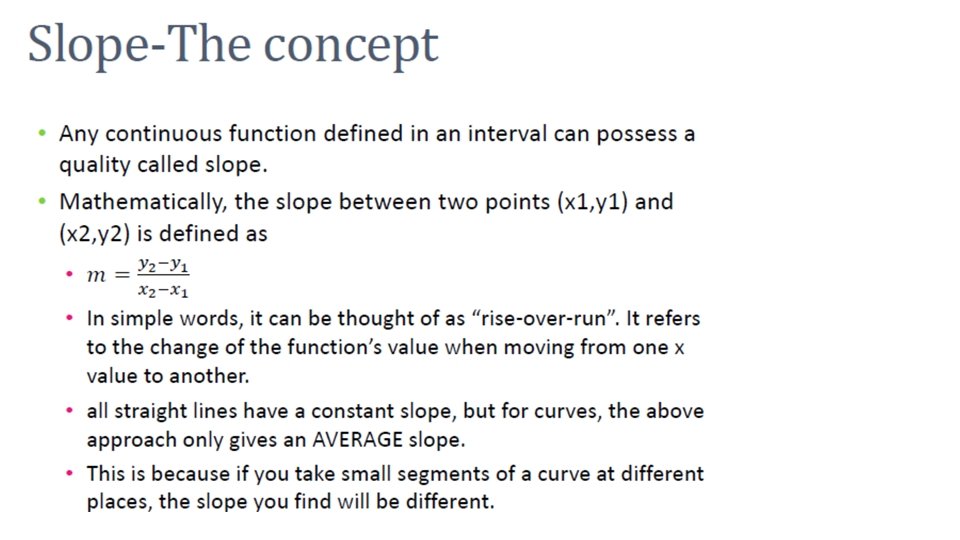
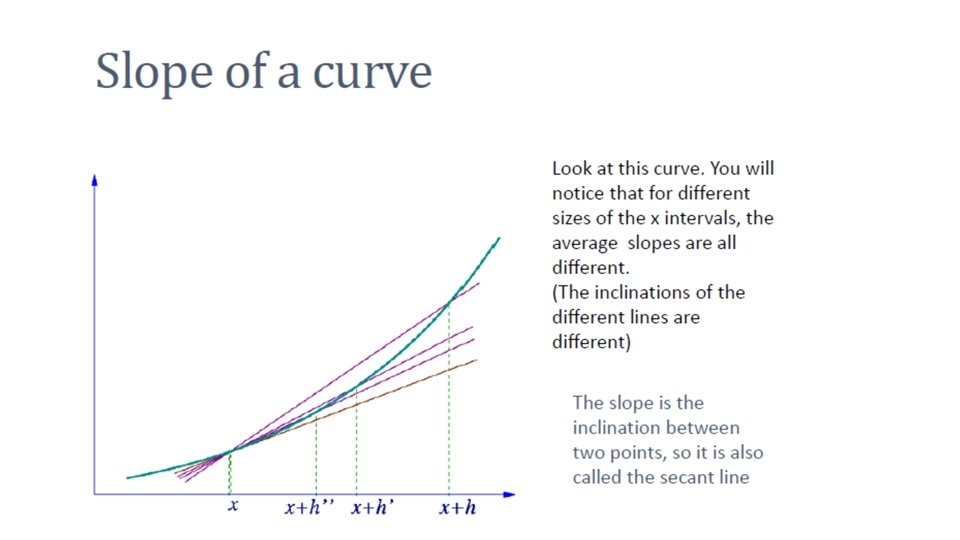
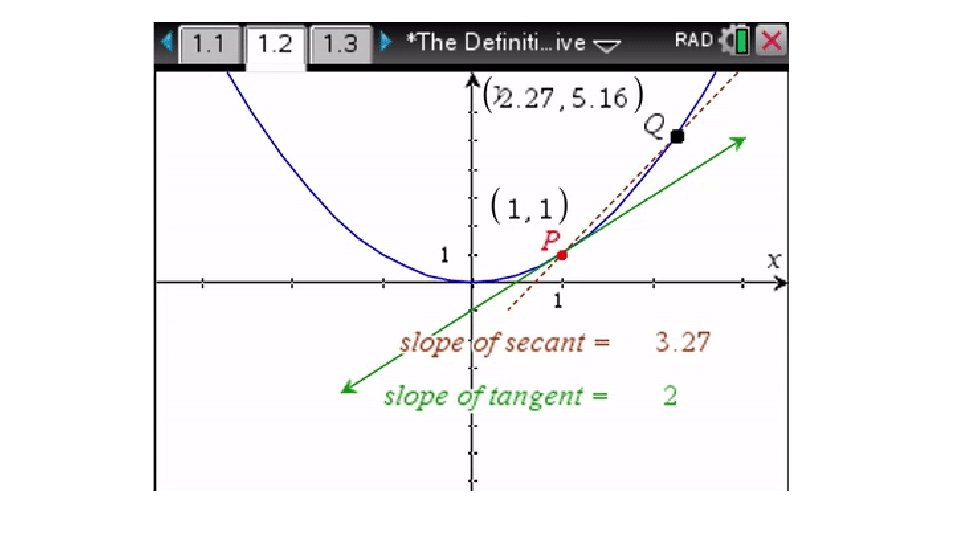
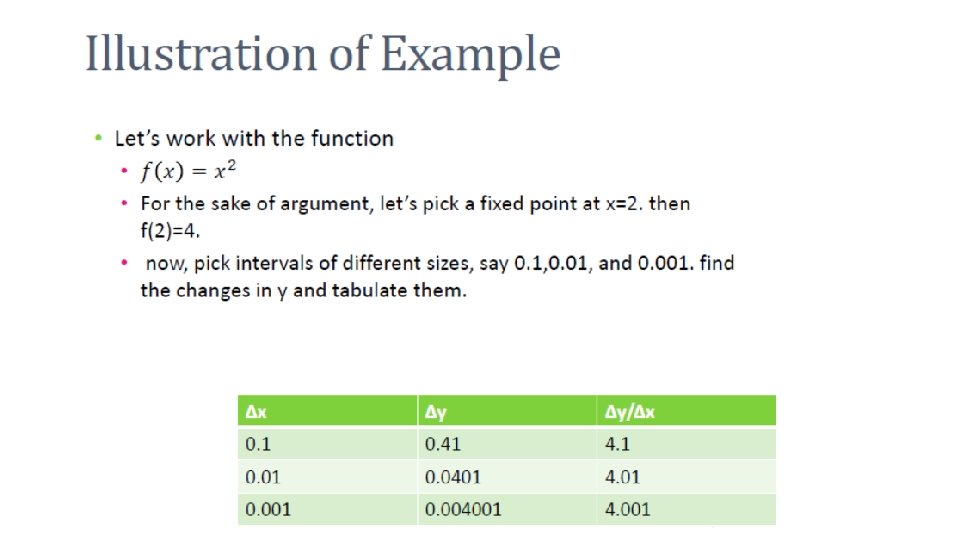
Continuity on an Interval • The function f is said to be continuous on an open interval (a, b) if – It is continuous at each number/point of the interval • It is said to be continuous on a closed interval [a, b] if – It is continuous at each number/point of the interval and – It is continuous from the right at a and continuous from the left at b 17

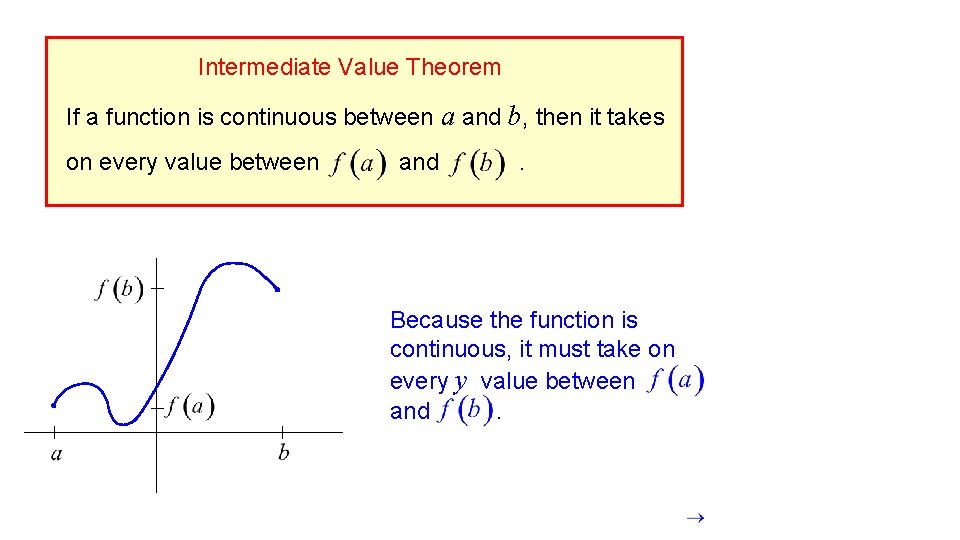
Intermediate Value Theorem If a function is continuous between a and b, then it takes on every value between and . Because the function is continuous, it must take on every y value between and.

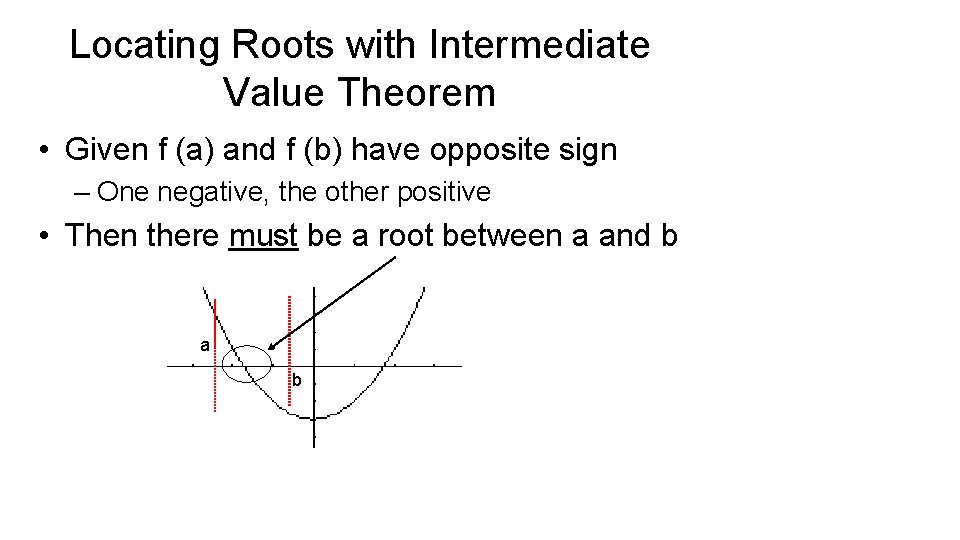
Locating Roots with Intermediate Value Theorem • Given f (a) and f (b) have opposite sign – One negative, the other positive • Then there must be a root between a and b a b

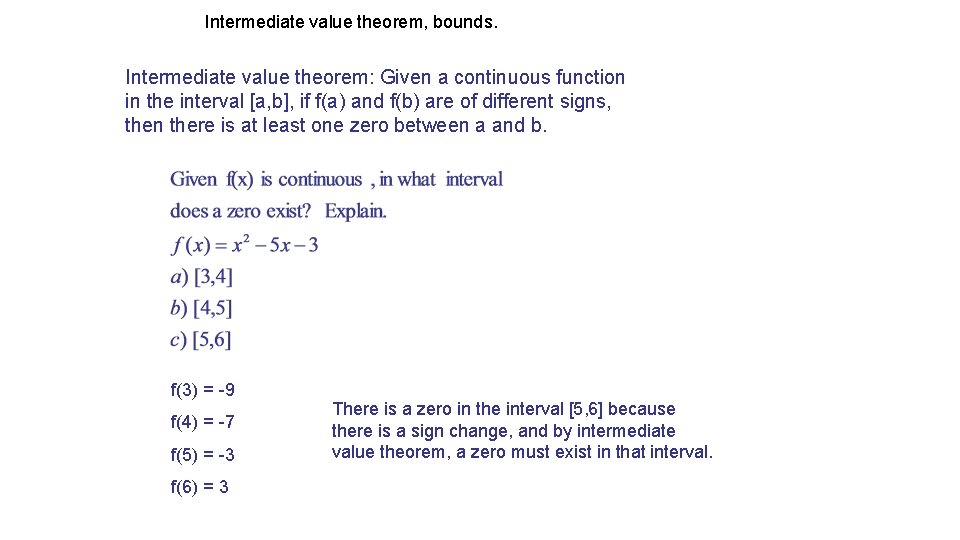
Intermediate value theorem, bounds. Intermediate value theorem: Given a continuous function in the interval [a, b], if f(a) and f(b) are of different signs, then there is at least one zero between a and b. f(3) = -9 f(4) = -7 f(5) = -3 f(6) = 3 There is a zero in the interval [5, 6] because there is a sign change, and by intermediate value theorem, a zero must exist in that interval.

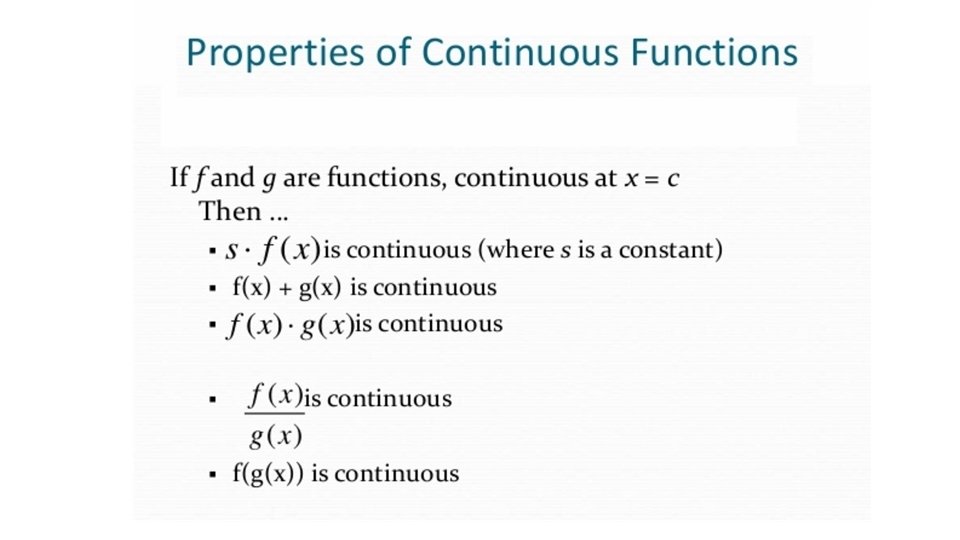
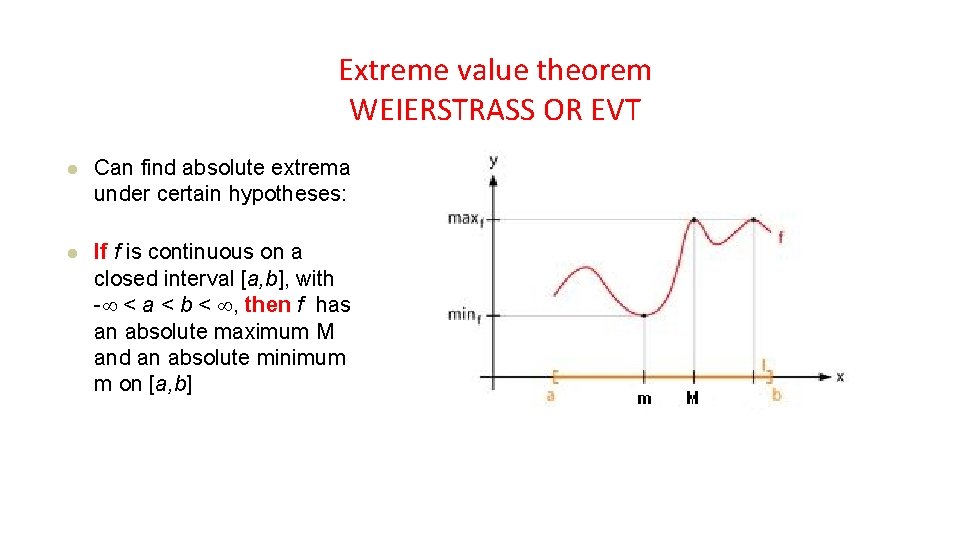
Extreme value theorem WEIERSTRASS OR EVT l Can find absolute extrema under certain hypotheses: l If f is continuous on a closed interval [a, b], with - < a < b < , then f has an absolute maximum M and an absolute minimum m on [a, b]
![Example No maximum or minimum value on the domain However on 3 3 it Example No maximum or minimum value on the domain. However, on [-3, 3], it](https://slidetodoc.com/presentation_image/357ad410c99c8279c17fa7ab60f26e62/image-22.jpg)
Example No maximum or minimum value on the domain. However, on [-3, 3], it has both. Question: does function f fullfil EVT?
![Conclusions about hypotheses l l Conclude that hypothesis that interval be closed a b Conclusions about hypotheses l l Conclude that hypothesis that interval be closed, [a, b],](https://slidetodoc.com/presentation_image/357ad410c99c8279c17fa7ab60f26e62/image-23.jpg)
Conclusions about hypotheses l l Conclude that hypothesis that interval be closed, [a, b], essential Conclusion that f is continuous also essential:

Examples fulfilling hypotheses l f(x) = 2 - 3 x where -5 < x < 8 l g(x) = sin(x) where 0 < x < 2 p

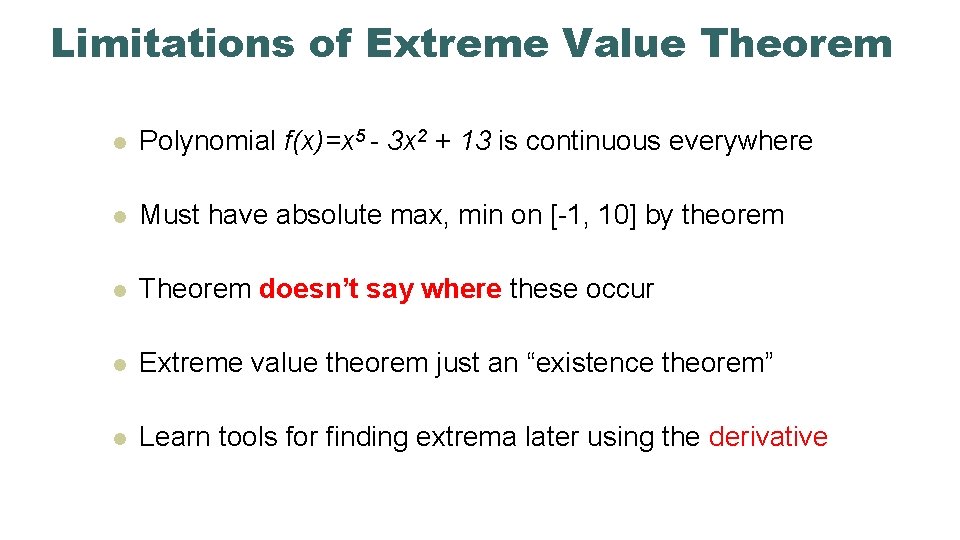
Limitations of Extreme Value Theorem l Polynomial f(x)=x 5 - 3 x 2 + 13 is continuous everywhere l Must have absolute max, min on [-1, 10] by theorem l Theorem doesn’t say where these occur l Extreme value theorem just an “existence theorem” l Learn tools for finding extrema later using the derivative















Derivative of a produce

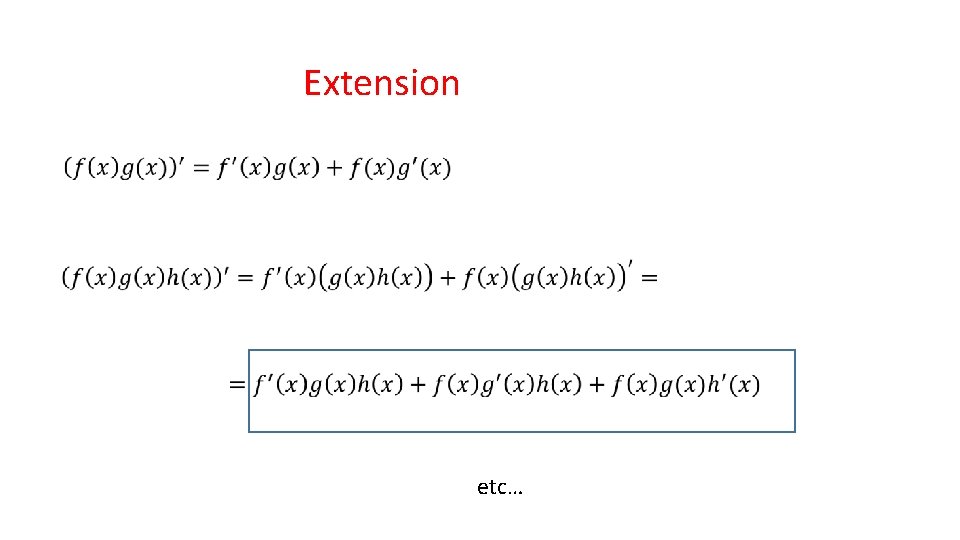
Extension etc…

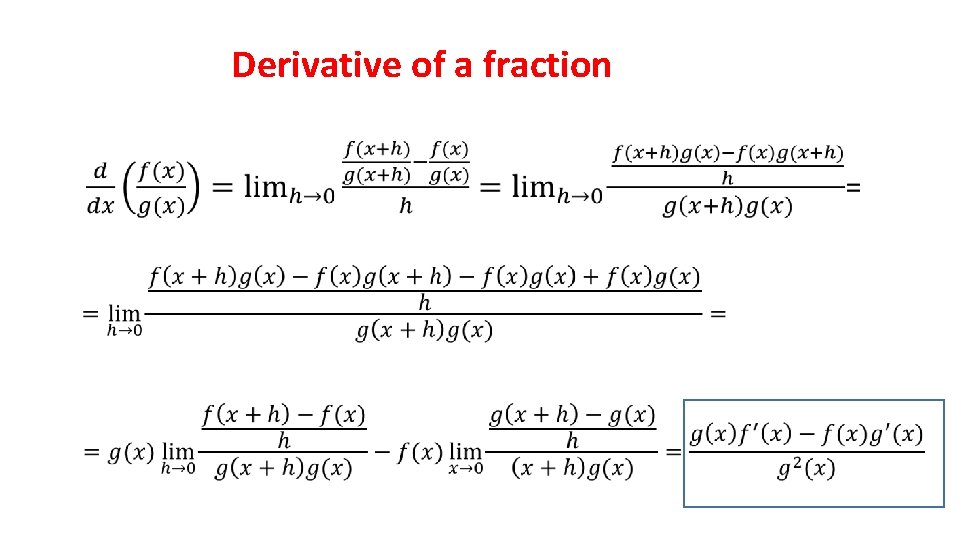
Derivative of a fraction

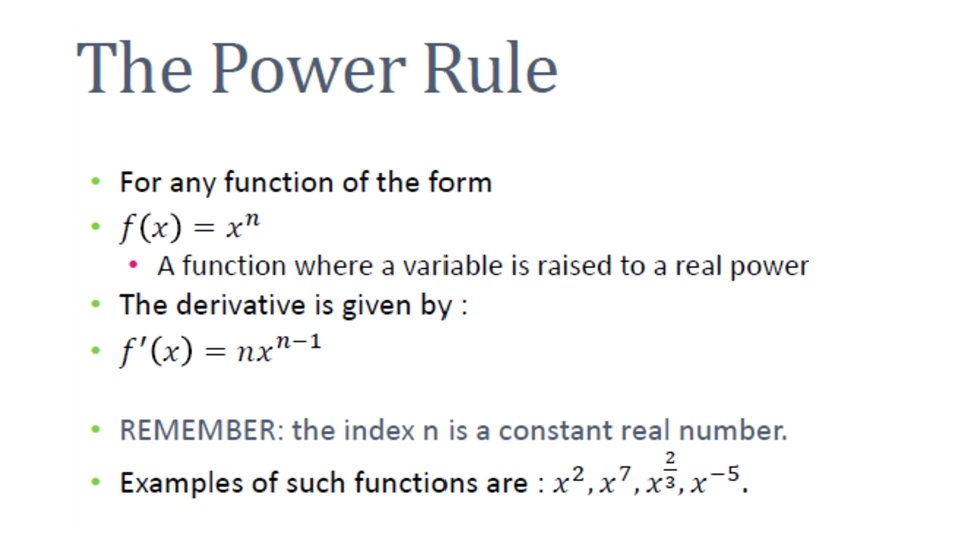
Derivative of the power function

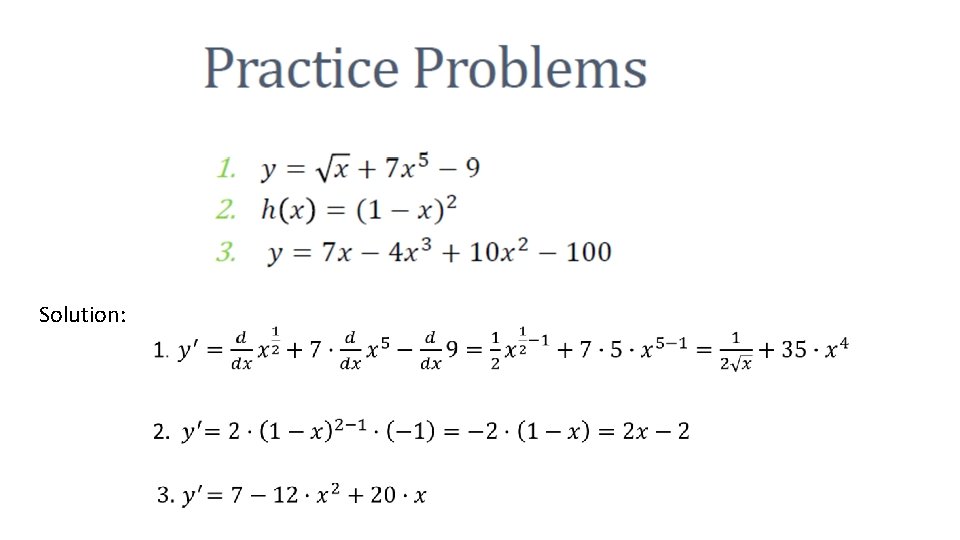
Derivative of the power function


Solution:

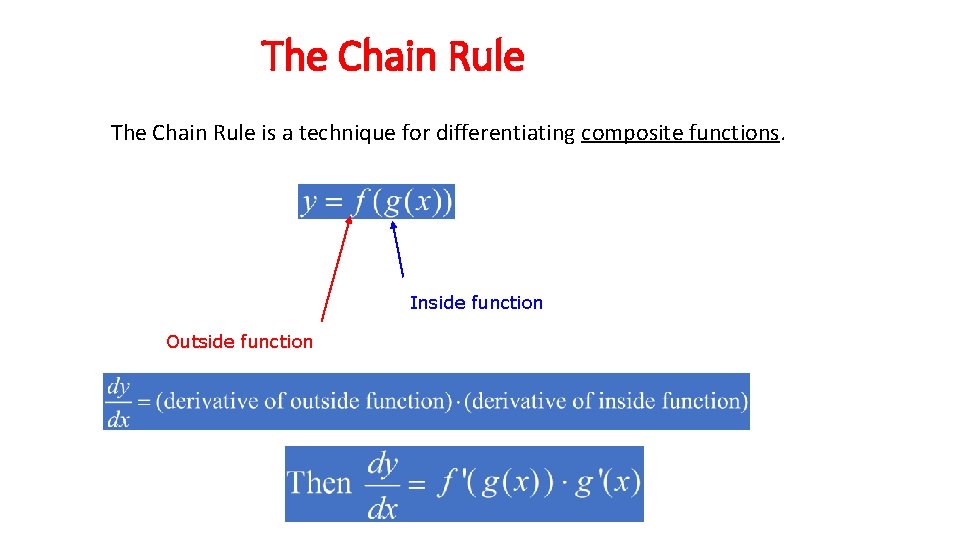
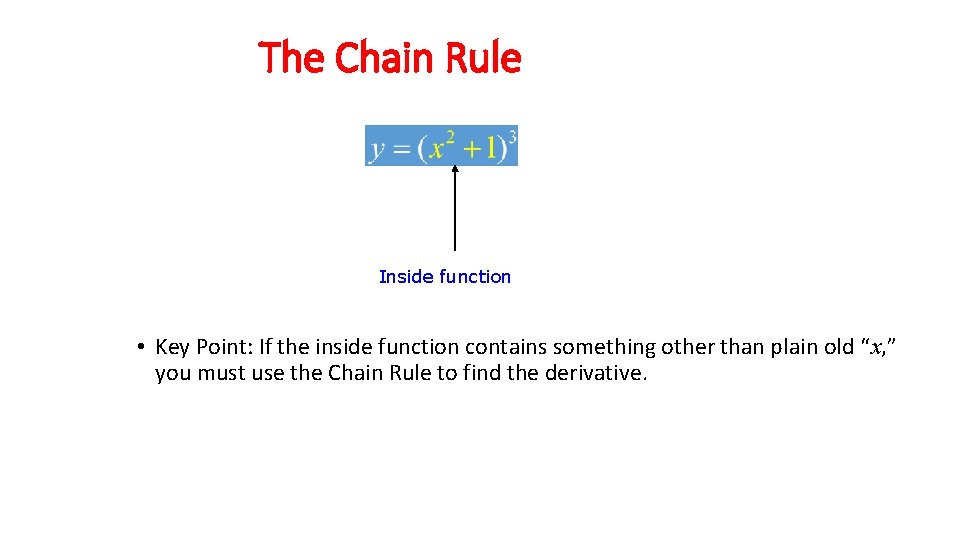
The Chain Rule is a technique for differentiating composite functions. Inside function Outside function

The Chain Rule 1. Identify inner and outer functions. 2. Derive outer function, leaving the inner function alone. 3. Derive the inner function.

The Chain Rule Inside function • Key Point: If the inside function contains something other than plain old “x, ” you must use the Chain Rule to find the derivative.

The Chain Rule Outside function

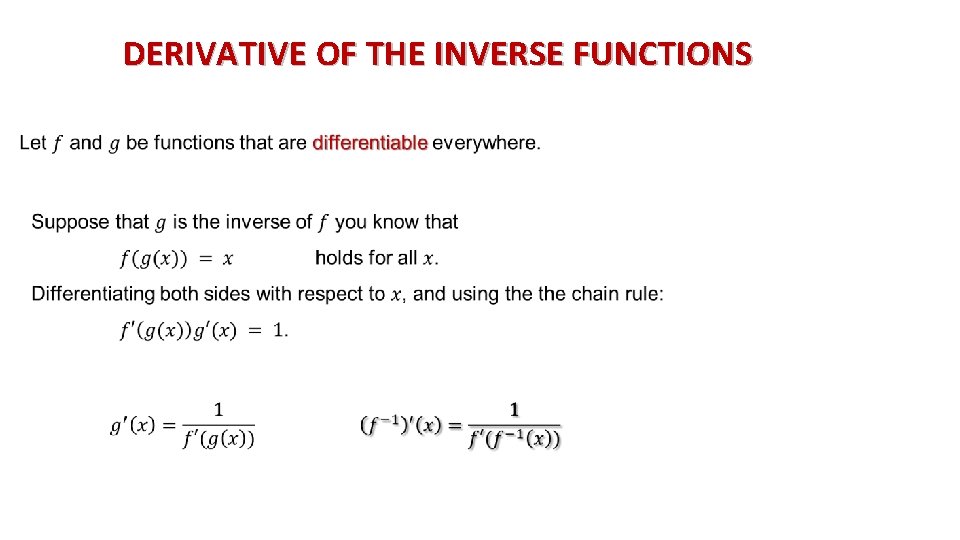
DERIVATIVE OF THE INVERSE FUNCTIONS

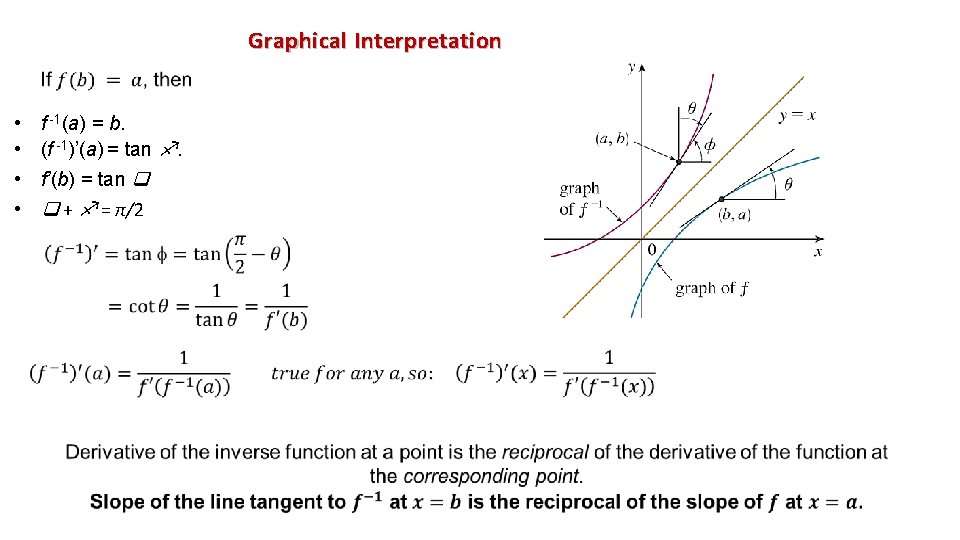
Graphical Interpretation • • f -1(a) = b. (f -1)’(a) = tan . f’(b) = tan + = π/2

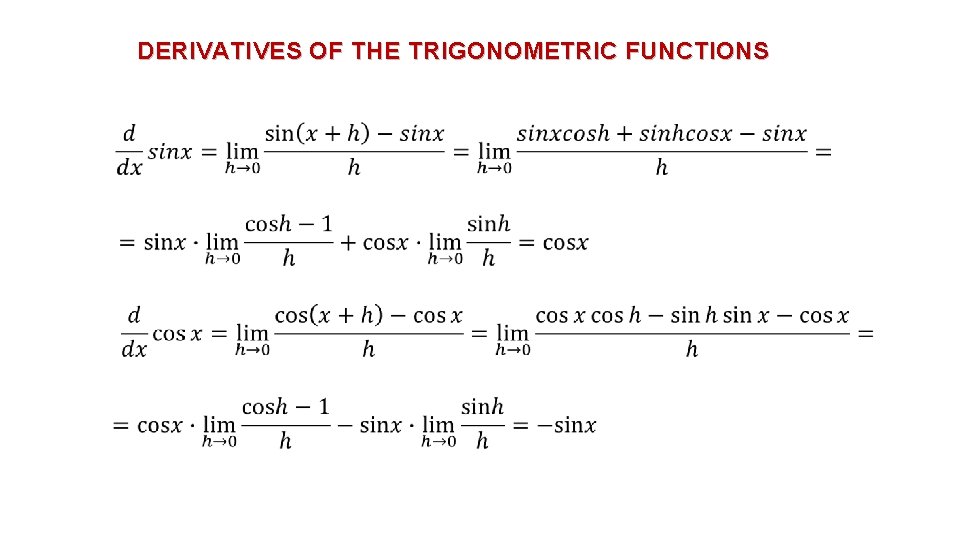
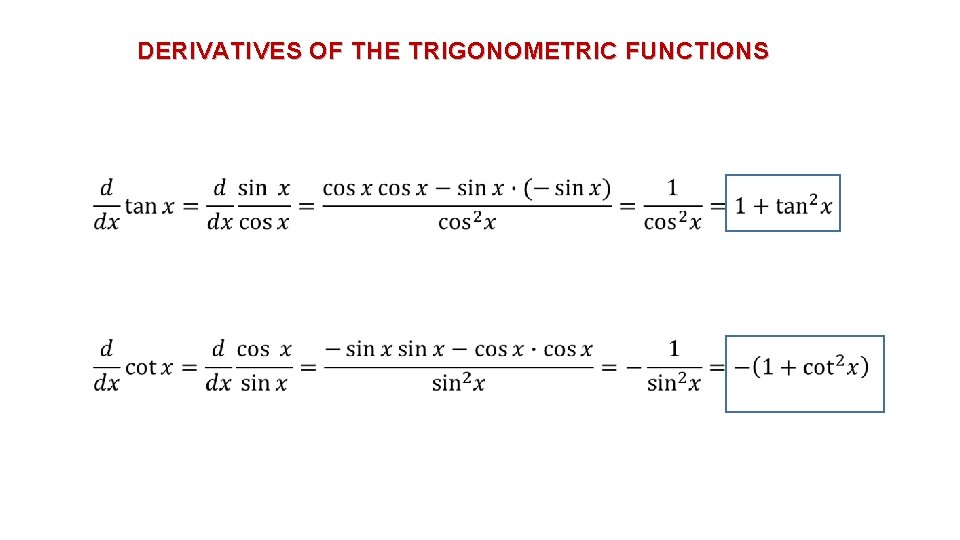
DERIVATIVES OF THE TRIGONOMETRIC FUNCTIONS

DERIVATIVES OF THE TRIGONOMETRIC FUNCTIONS

DERIVATIVES OF THE TRIGONOMETRIC FUNCTIONS

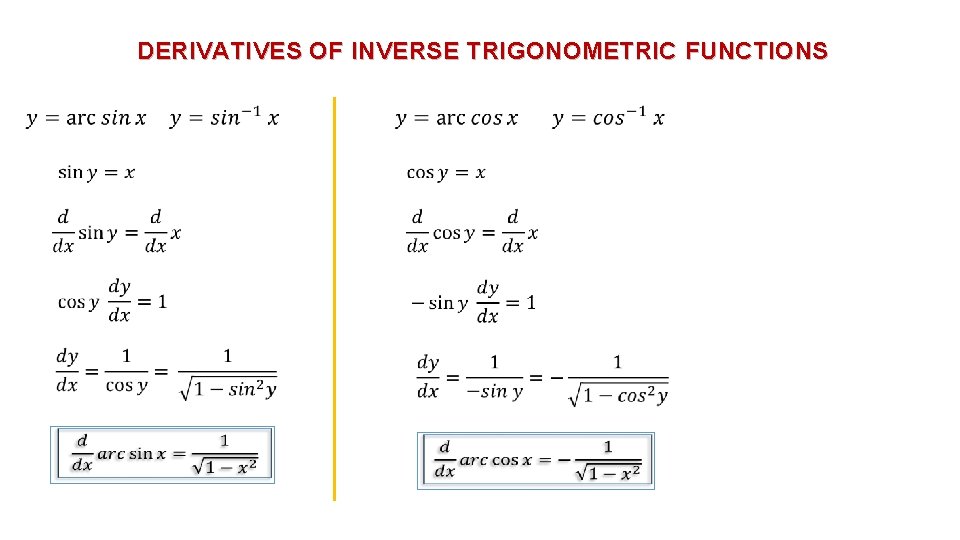
DERIVATIVES OF INVERSE TRIG ONOMETRIC FUNCTIONS

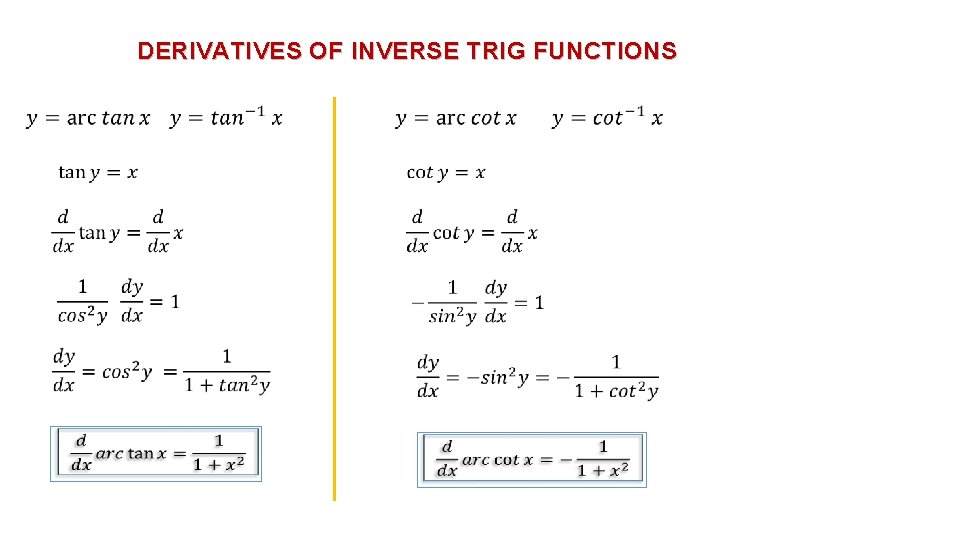
DERIVATIVES OF INVERSE TRIG FUNCTIONS

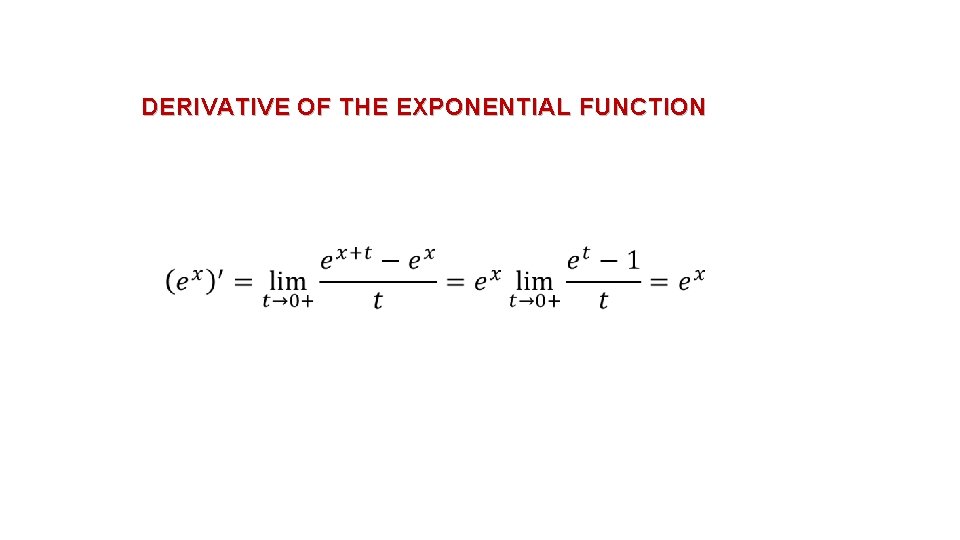
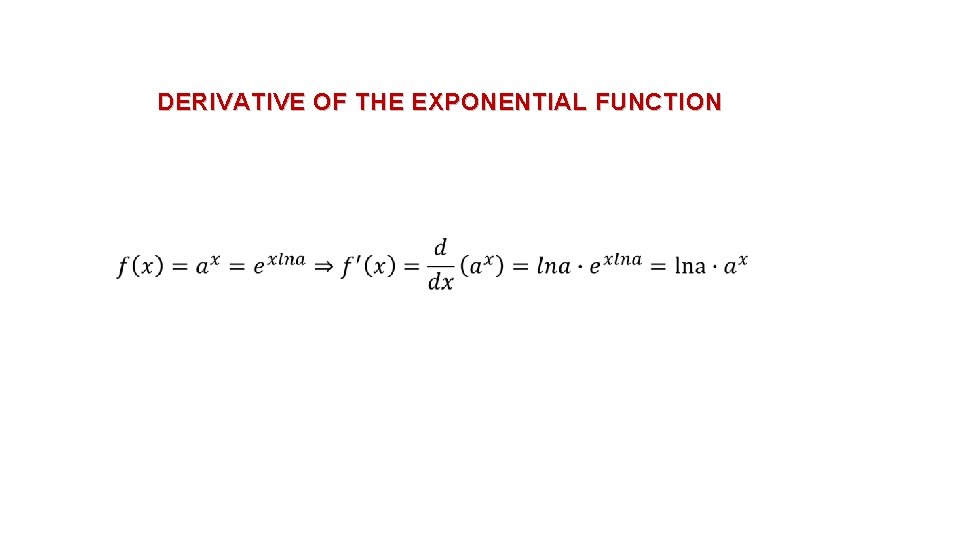
DERIVATIVE OF THE EXPONENTIAL FUNCTION

DERIVATIVE OF THE EXPONENTIAL FUNCTION

DERIVATIVE OF THE EXPONENTIAL FUNCTION

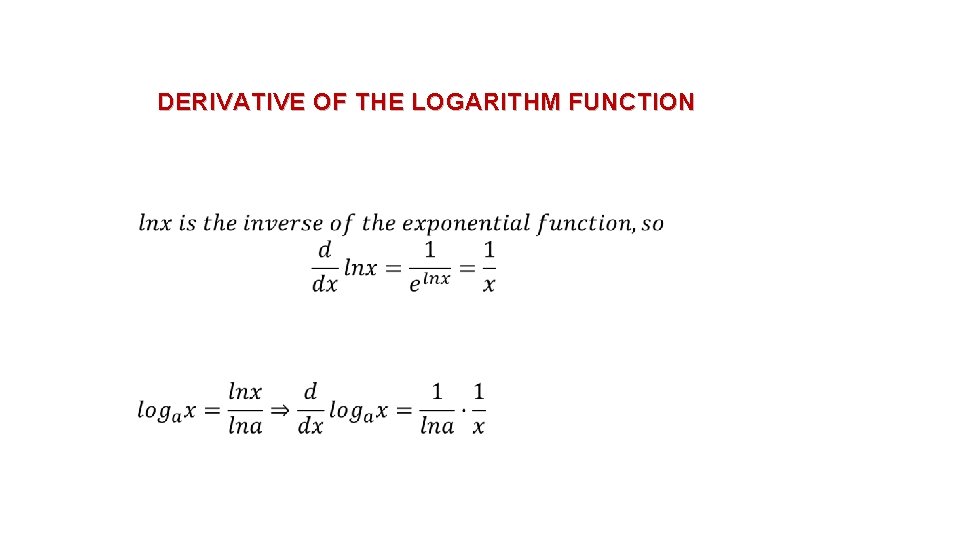
DERIVATIVE OF THE LOGARITHM FUNCTION

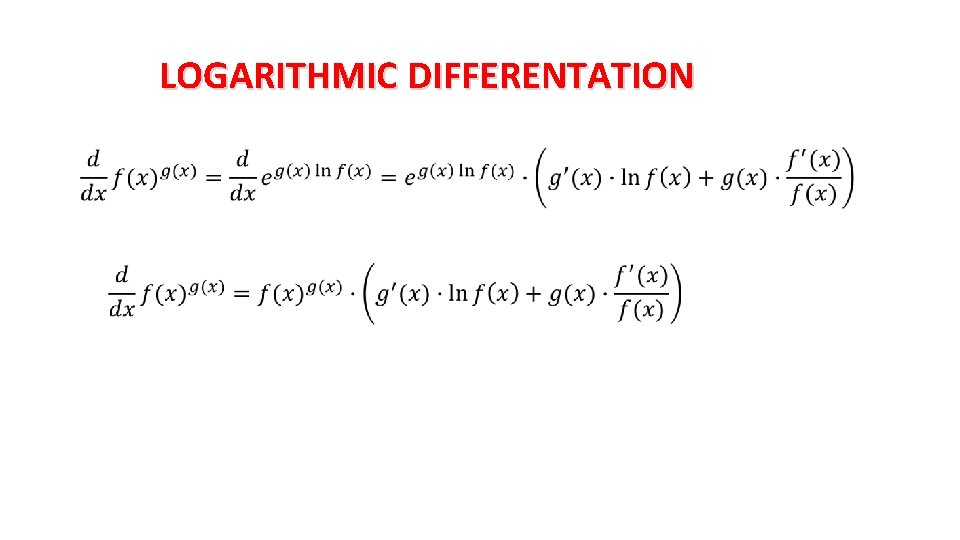
LOGARITHMIC DIFFERENTATION

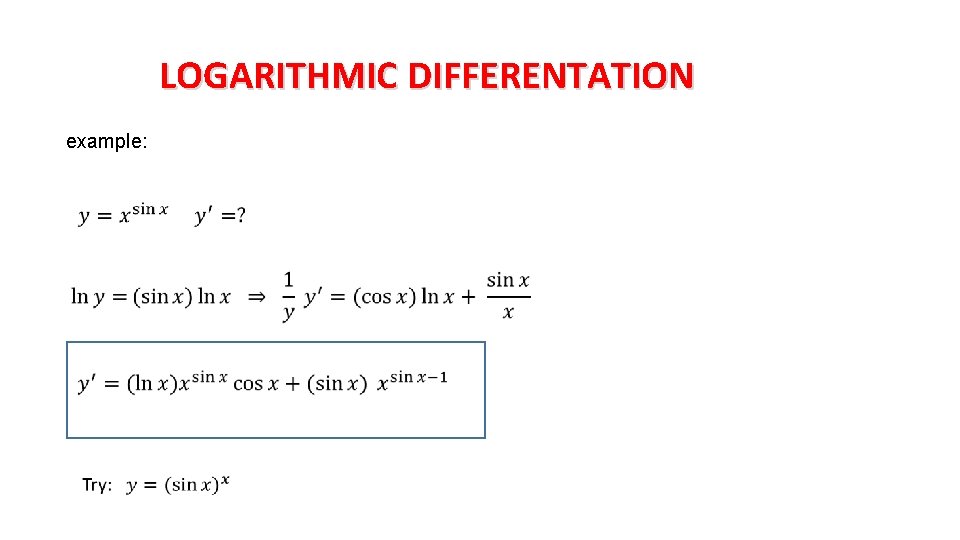
LOGARITHMIC DIFFERENTATION example:
 Discontinuous development psychology definition
Discontinuous development psychology definition Early experience vs. later experience
Early experience vs. later experience Jump essential discontinuity
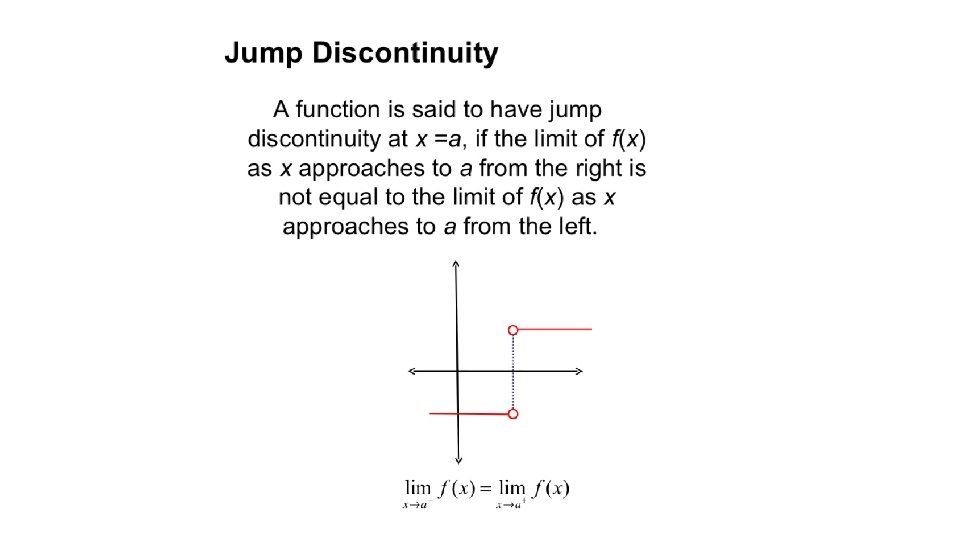
Jump essential discontinuity How to find the point of discontinuity
How to find the point of discontinuity Absolute continuity implies uniform continuity
Absolute continuity implies uniform continuity Characteristics of lipids
Characteristics of lipids There isn't any burger
There isn't any burger Any to any connectivity
Any to any connectivity Any question atau any questions
Any question atau any questions Main actors and forces in a modern marketing system
Main actors and forces in a modern marketing system A cat by any other name worksheet answers
A cat by any other name worksheet answers Marketing more than any other business function deals with
Marketing more than any other business function deals with Marketing more than any other business function deals with
Marketing more than any other business function deals with Any substance other than food
Any substance other than food Types of repair
Types of repair Discontinuous development psychology
Discontinuous development psychology Regression discontinuity
Regression discontinuity Technology discontinuity
Technology discontinuity Regression discontinuity design
Regression discontinuity design Piaget second stage
Piaget second stage Discontinuity of development
Discontinuity of development Infants, children, and adolescents 8th edition
Infants, children, and adolescents 8th edition Mohorovicic discontinuity
Mohorovicic discontinuity Mohorovicic discontinuity
Mohorovicic discontinuity Mohorovicic discontinuity
Mohorovicic discontinuity Cultural discontinuity
Cultural discontinuity Oscillating discontinuity definition
Oscillating discontinuity definition How to find discontinuity of a function
How to find discontinuity of a function Increasing intervals
Increasing intervals What is conrad discontinuity
What is conrad discontinuity 8-3 rational functions and their graphs
8-3 rational functions and their graphs Infinite discontinuity
Infinite discontinuity Contoh pendekatan discontinuity yaitu...
Contoh pendekatan discontinuity yaitu... Equation of a rational function
Equation of a rational function Removable discontinuity algebraically
Removable discontinuity algebraically Secondary processing discontinuities
Secondary processing discontinuities Discontinuity functions beam
Discontinuity functions beam Interrupted time series vs regression discontinuity
Interrupted time series vs regression discontinuity Uniform building code 1997
Uniform building code 1997 Language
Language Site class seismic design category
Site class seismic design category Repetti discontinuity
Repetti discontinuity Hình ảnh bộ gõ cơ thể búng tay
Hình ảnh bộ gõ cơ thể búng tay Bổ thể
Bổ thể Tỉ lệ cơ thể trẻ em
Tỉ lệ cơ thể trẻ em Chó sói
Chó sói Tư thế worms-breton
Tư thế worms-breton Chúa yêu trần thế
Chúa yêu trần thế Môn thể thao bắt đầu bằng chữ đua
Môn thể thao bắt đầu bằng chữ đua Thế nào là hệ số cao nhất
Thế nào là hệ số cao nhất Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Cong thức tính động năng
Cong thức tính động năng Trời xanh đây là của chúng ta thể thơ
Trời xanh đây là của chúng ta thể thơ Cách giải mật thư tọa độ
Cách giải mật thư tọa độ 101012 bằng
101012 bằng độ dài liên kết
độ dài liên kết Các châu lục và đại dương trên thế giới
Các châu lục và đại dương trên thế giới Thơ thất ngôn tứ tuyệt đường luật
Thơ thất ngôn tứ tuyệt đường luật Quá trình desamine hóa có thể tạo ra
Quá trình desamine hóa có thể tạo ra Một số thể thơ truyền thống
Một số thể thơ truyền thống Bàn tay mà dây bẩn
Bàn tay mà dây bẩn Vẽ hình chiếu vuông góc của vật thể sau
Vẽ hình chiếu vuông góc của vật thể sau Biện pháp chống mỏi cơ
Biện pháp chống mỏi cơ