Contents The inverse trigonometric functions The reciprocal trigonometric


- Slides: 11

Contents The inverse trigonometric functions The reciprocal trigonometric functions Trigonometric identities Examination-style question 1 of 35 © Boardworks Ltd 2006

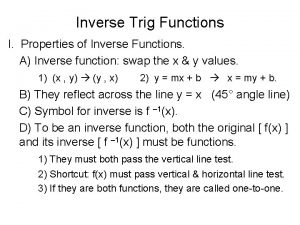
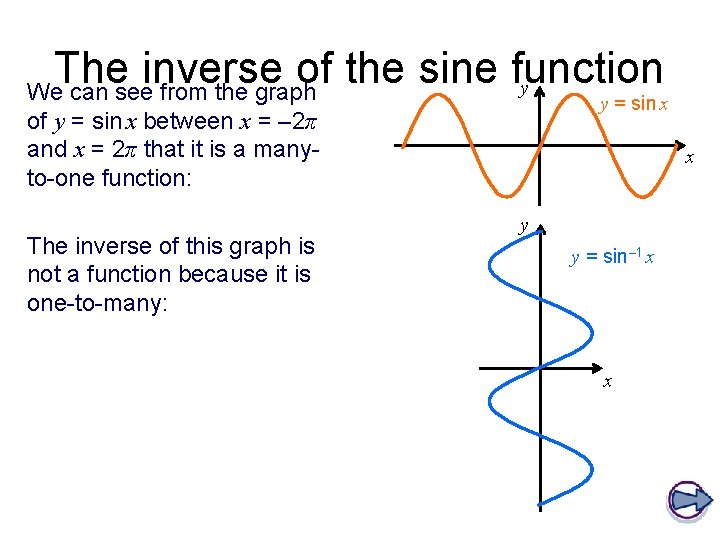
The inverse of the sine function Suppose we wish to find θ such that sin θ = x In other words, we want to find the angle whose sine is x. This is written as θ = sin– 1 x or θ = arcsin x In this context, sin– 1 x means the inverse of sin x. This is not the same as (sin x)– 1 which is the reciprocal of sin x, . Is y = sin– 1 x a function?

The inverse of the sine function y We can see from the graph y = sin x of y = sin x between x = – 2π and x = 2π that it is a manyto-one function: The inverse of this graph is not a function because it is one-to-many: x y y = sin– 1 x x

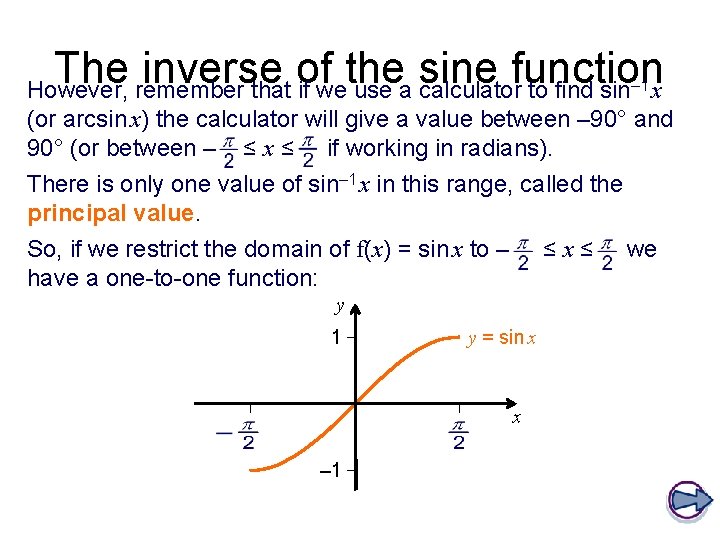
The inverse of the sine function However, remember that if we use a calculator to find sin x – 1 (or arcsin x) the calculator will give a value between – 90° and 90° (or between – ≤ x ≤ if working in radians). There is only one value of sin– 1 x in this range, called the principal value. So, if we restrict the domain of f(x) = sin x to – ≤ x ≤ we have a one-to-one function: y 1 y = sin x x – 1

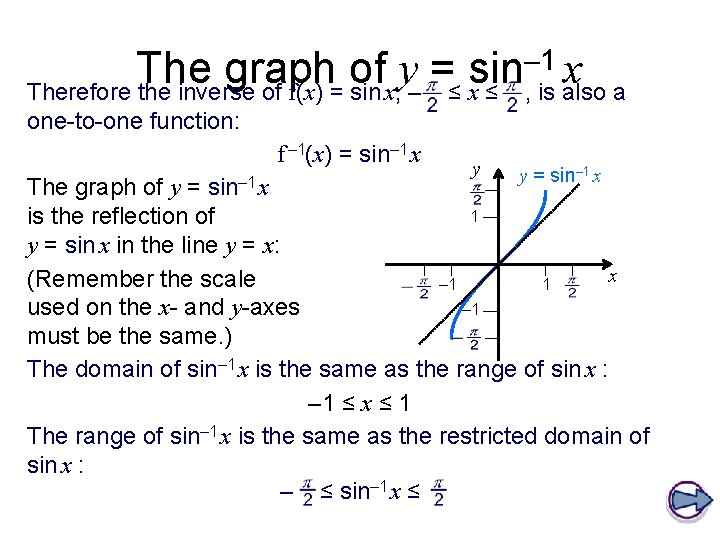
– 1 x The graph of y = sin Therefore the inverse of f(x) = sin x, – ≤ x ≤ , is also a one-to-one function: f – 1(x) = sin– 1 x y y = sin– 1 x The graph of y = 1 is the reflection of y = sin x in the line y = x: x (Remember the scale – 1 1 – 1 used on the x- and y-axes must be the same. ) The domain of sin– 1 x is the same as the range of sin x : – 1 ≤ x ≤ 1 The range of sin– 1 x is the same as the restricted domain of sin x : – ≤ sin– 1 x ≤

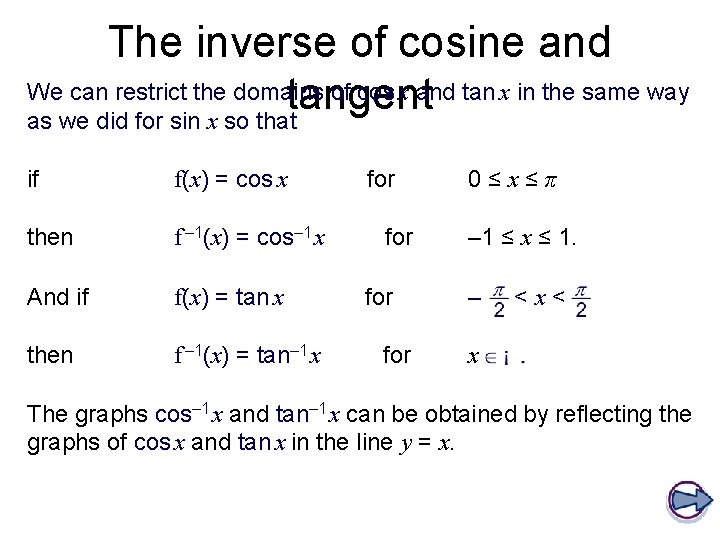
The inverse of cosine and We can restrict the domains of cos x and tan x in the same way tangent as we did for sin x so that if f(x) = cos x then f – 1(x) = cos– 1 x And if f(x) = tan x then f – 1(x) = tan– 1 x for for 0≤x≤π – 1 ≤ x ≤ 1. – <x< x The graphs cos– 1 x and tan– 1 x can be obtained by reflecting the graphs of cos x and tan x in the line y = x.

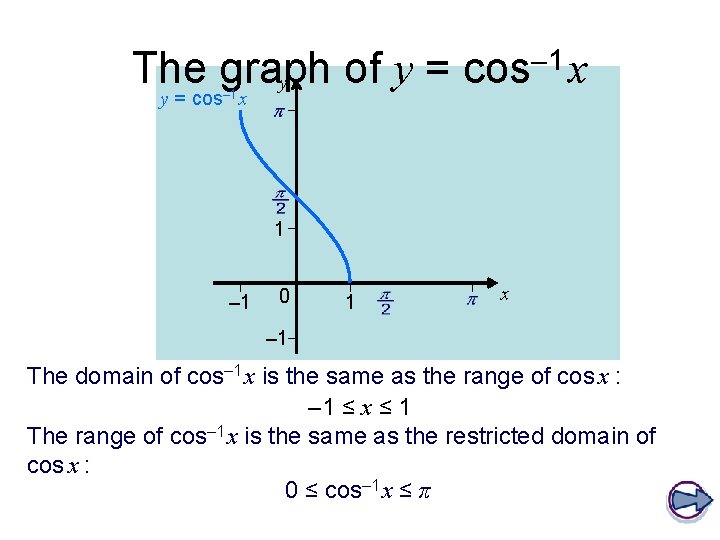
– 1 x The graph of y = cos y y = cos– 1 x 1 – 1 0 – 1 1 x y = cosx The domain of cos– 1 x is the same as the range of cos x : – 1 ≤ x ≤ 1 The range of cos– 1 x is the same as the restricted domain of cos x : 0 ≤ cos– 1 x ≤ π

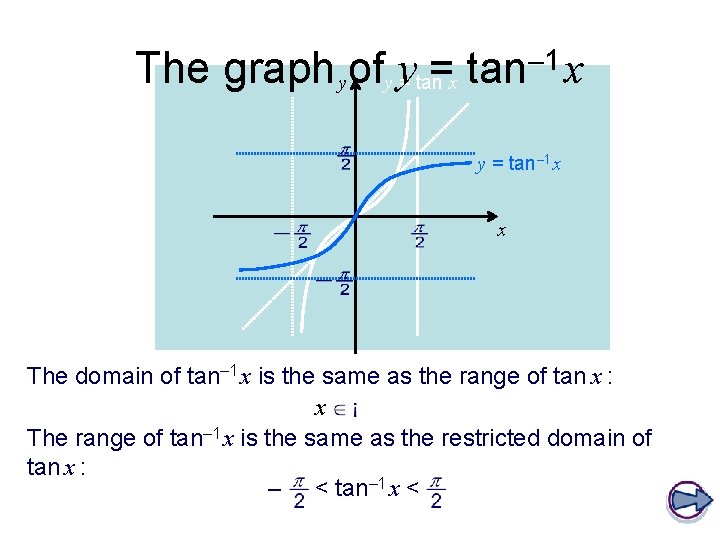
– 1 x The graph yofy y= tanx = tan x y = tan– 1 x x The domain of tan– 1 x is the same as the range of tan x : x The range of tan– 1 x is the same as the restricted domain of tan x : – < tan– 1 x <

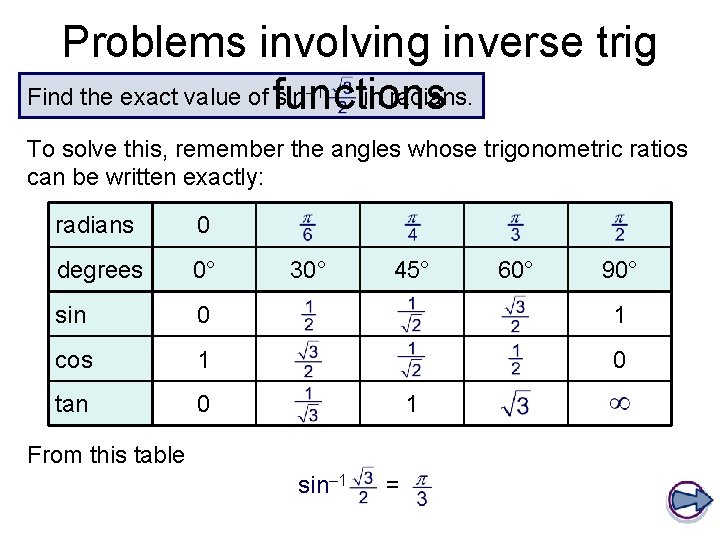
Problems involving inverse trig Find the exact value of functions sin in radians. – 1 To solve this, remember the angles whose trigonometric ratios can be written exactly: radians 0 degrees 0° sin 0 1 cos 1 0 tan 0 30° 45° 1 From this table sin– 1 = 60° 90°

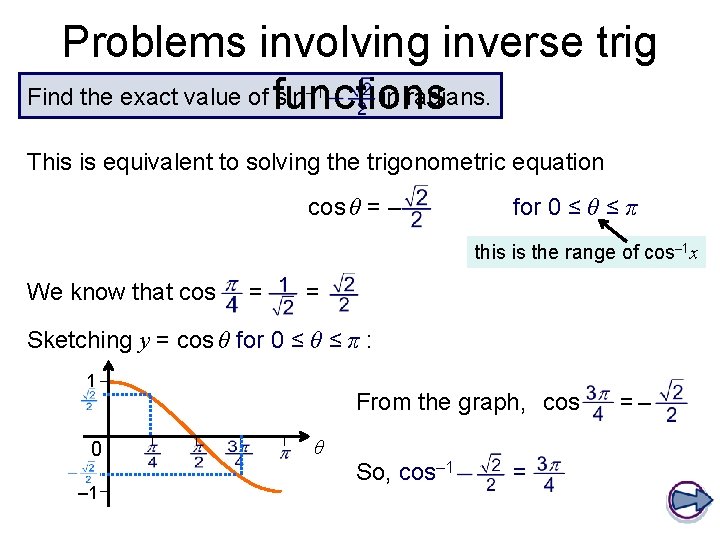
Problems involving inverse trig Find the exact value of functions sin in radians. – 1 This is equivalent to solving the trigonometric equation cos θ = – for 0 ≤ θ ≤ π this is the range of cos– 1 x We know that cos = = Sketching y = cos θ for 0 ≤ θ ≤ π : 1 0 – 1 From the graph, cos θ So, cos– 1 = =–

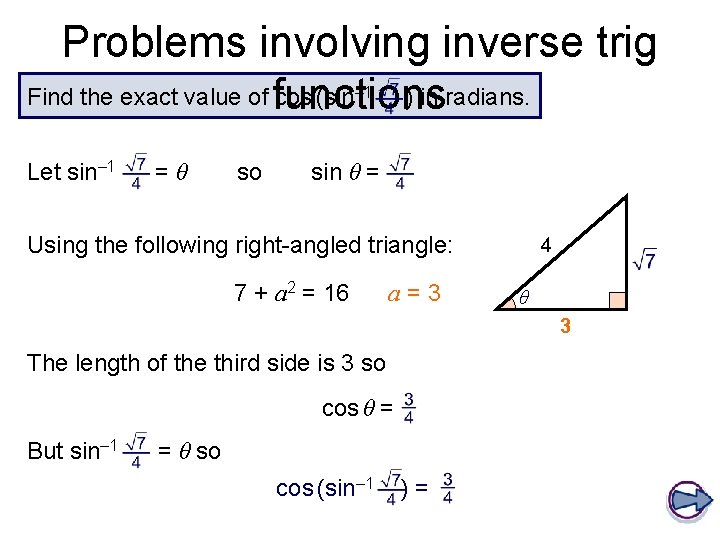
Problems involving inverse trig Find the exact value of functions cos (sin ) in radians. – 1 Let sin– 1 =θ so sin θ = Using the following right-angled triangle: 7 + a 2 = 16 a=3 4 θ 3 The length of the third side is 3 so cos θ = But sin– 1 = θ so cos (sin– 1 )=
 Evaluating inverse trig functions
Evaluating inverse trig functions How is tangent used in real life
How is tangent used in real life Trigonometry derivatives
Trigonometry derivatives Integration of inverse trigonometric functions
Integration of inverse trigonometric functions Inverse circular functions and trigonometric equations
Inverse circular functions and trigonometric equations Integral of exponential
Integral of exponential Domain of the inverse cosine function
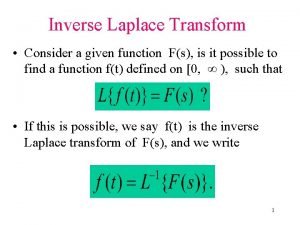
Domain of the inverse cosine function Inverse laplace transform examples
Inverse laplace transform examples Summary of inverse trigonometric functions
Summary of inverse trigonometric functions Arcsin
Arcsin How to graph a reciprocal function
How to graph a reciprocal function Parent constant function
Parent constant function